Chapter 7.2, Problem 84AYU

Problems 79 and 80 require the following discussion: When granular materials are allowed to fall freely, they form conical (cone-shaped) piles. The naturally occurring angle of slope, measured from the horizontal, at which the loose material comes to rest is called the angle of repose and varies for different materials. The angle of repose $\theta$ is related to the height $h$ and base radius $r$ of the conical pile by the equation $\theta ={\cot }^{-1}\frac{r}{h}$. See the illustration.

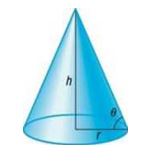
Angle of Repose: Bunker Sand The steepness of sand bunkers on a golf course is affected by the angle of repose of the sand (a larger angle of repose allows for steeper bunkers). A freestanding pile of loose sand from a United States Golf Association (USGA) bunker had a height of 4 feet and a base diameter of approximately $\text{6}\text{.68}$ feet.

(a) Find the angle of repose for USGA bunker sand.

(b) What is the height of such a pile if the diameter of the base is 8 feet?

(c) A 6-foot-high pile of loose Tour Grade 50/50 sand has a base diameter of approximately $\text{8}\text{.44}$ feet. Which type of sand (USGA or Tour Grade 50/50) would be better suited for steep bunkers?