
To approximate the distance from the Earth to stars relatively close by, astronomers often use the method of parallax. Parallax is the apparent displacement of an object caused by a change in the observer’s point of view. As the Earth orbits the Sun, a nearby star will appear to move against the more distant background stars. Astronomers measure a star’s position at times exactly 6 months apart when the Earth is at opposite points in its orbit around the Sun. The Sun, Earth, and star form the vertices of a right
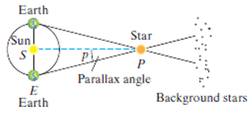
31.
a. Find the distance between the Earth and Proxima Centauri (the closest star to the Earth beyond the Sun) if the parallax angle is 0.772” (arcseconds). Round to the nearest hundred billion miles.
b. Write the distance in part (a) in light-years. Round to I decimal place. (Hint 1 light-year is the distance that light travels in 1 yr and is approximately 5.878 × 1012 mi.)
Want to see the full answer?
Check out a sample textbook solution
Chapter 7 Solutions
COLL ALG & TRIG ALEKS 360 ACCESS CARD
- Pls help.arrow_forwardSolve the system of equation for y using Cramer's rule. Hint: The determinant of the coefficient matrix is -23. - 5x + y − z = −7 2x-y-2z = 6 3x+2z-7arrow_forwarderic pez Xte in z= Therefore, we have (x, y, z)=(3.0000, 83.6.1 Exercise Gauss-Seidel iteration with Start with (x, y, z) = (0, 0, 0). Use the convergent Jacobi i Tol=10 to solve the following systems: 1. 5x-y+z = 10 2x-8y-z=11 -x+y+4z=3 iteration (x Assi 2 Assi 3. 4. x-5y-z=-8 4x-y- z=13 2x - y-6z=-2 4x y + z = 7 4x-8y + z = -21 -2x+ y +5z = 15 4x + y - z=13 2x - y-6z=-2 x-5y- z=-8 realme Shot on realme C30 2025.01.31 22:35 farrow_forward
- Use Pascal's triangle to expand the binomial (6m+2)^2arrow_forwardListen A falling object travels a distance given by the formula d = 6t + 9t2 where d is in feet and t is the time in seconds. How many seconds will it take for the object to travel 112 feet? Round answer to 2 decimal places. (Write the number, not the units). Your Answer:arrow_forwardSolve by the quadratic formula or completing the square to obtain exact solutions. 2 e 104 OA) -16±3√6 B) 8±√10 O c) -8±√10 OD) 8±3√√6 Uarrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,  Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning





