Intermediate Algebra for College Students (7th Edition)
Intermediate Algebra for College Students (7th Edition)
7th Edition
ISBN: 9780134178943
Author: Robert F. Blitzer
Publisher: PEARSON
bartleby

Concept explainers

Transformation of Graphs
The word ‘transformation’ means modification. Transformation of the graph of a function is a process by which we modify or change the original graph and make a new graph.
Exponential Functions
The exponential function is a type of mathematical function which is used in real-world contexts. It helps to find out the exponential decay model or exponential growth model, in mathematical models. In this topic, we will understand descriptive rules, c…
bartleby

Videos

Textbook Question
Book Icon
Chapter 7.1, Problem 28E

In Exercises 27–32, find the domain of each square root function. Then use the domain to match the radical function with its graph. [The graphs are labeled (a) through (f) and are shown in [ − 10 , 10 , 1 ] by [ − 10 , 10 , 1 ] viewing rectangles below and on the next page.]

f ( x ) = x + 2

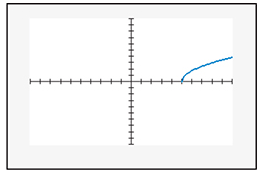
a. Chapter 7.1, Problem 28E, In Exercises 27–32, find the domain of each square root function. Then use the domain to match the , example 1

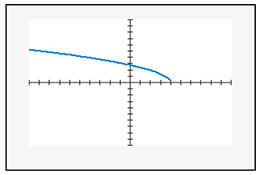
b. Chapter 7.1, Problem 28E, In Exercises 27–32, find the domain of each square root function. Then use the domain to match the , example 2

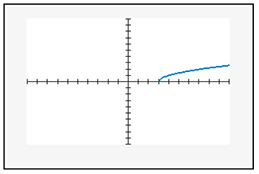
c. Chapter 7.1, Problem 28E, In Exercises 27–32, find the domain of each square root function. Then use the domain to match the , example 3

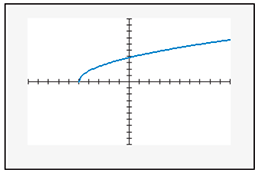
d. Chapter 7.1, Problem 28E, In Exercises 27–32, find the domain of each square root function. Then use the domain to match the , example 4

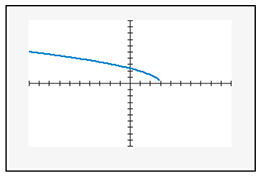
e. Chapter 7.1, Problem 28E, In Exercises 27–32, find the domain of each square root function. Then use the domain to match the , example 5

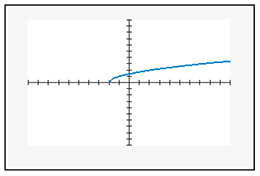
f. Chapter 7.1, Problem 28E, In Exercises 27–32, find the domain of each square root function. Then use the domain to match the , example 6

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 7.1, Problem 27E
Next
Chapter 7.1, Problem 29E
Students have asked these similar questions
Solve them
I want to learn this topic l dont know anything about it
Solve the linear system of equations attached using Gaussian elimination (not Gauss-Jordan) and back subsitution. Remember that: A matrix is in row echelon form if Any row that consists only of zeros is at the bottom of the matrix. The first non-zero entry in each other row is 1. This entry is called aleading 1. The leading 1 of each row, after the first row, lies to the right of the leading 1 of the previous row.

Chapter 7 Solutions

Intermediate Algebra for College Students (7th Edition)

Ch. 7.1 - Check Point 1 Evaluate: a. 64 b. 49 c. 1625 d....Ch. 7.1 - Prob. 2CPCh. 7.1 - Prob. 3CPCh. 7.1 - Check Point 4 If the trend from 1996 through 2011...Ch. 7.1 - Prob. 5CPCh. 7.1 - Check Point 6 For each function, find the...Ch. 7.1 - Prob. 7CPCh. 7.1 - Prob. 8CPCh. 7.1 - Prob. 9CPCh. 7.1 - Fill in each blank so that the resulting statement...
Ch. 7.1 - Prob. 2CVCCh. 7.1 - Prob. 3CVCCh. 7.1 - Prob. 4CVCCh. 7.1 - Prob. 5CVCCh. 7.1 - Prob. 6CVCCh. 7.1 - Prob. 7CVCCh. 7.1 - Prob. 8CVCCh. 7.1 - Prob. 9CVCCh. 7.1 - Prob. 10CVCCh. 7.1 - Prob. 11CVCCh. 7.1 - Prob. 12CVCCh. 7.1 - Prob. 13CVCCh. 7.1 - Prob. 14CVCCh. 7.1 - True or false: 14 is a real number.________Ch. 7.1 - Prob. 1ECh. 7.1 - Prob. 2ECh. 7.1 - In Exercises 1–20, evaluate each expression, or...Ch. 7.1 - Prob. 4ECh. 7.1 - Prob. 5ECh. 7.1 - Prob. 6ECh. 7.1 - Prob. 7ECh. 7.1 - Prob. 8ECh. 7.1 - In Exercises 1–20, evaluate each expression, or...Ch. 7.1 - Prob. 10ECh. 7.1 - Prob. 11ECh. 7.1 - In Exercises 120, evaluate each expression, or...Ch. 7.1 - Prob. 13ECh. 7.1 - Prob. 14ECh. 7.1 - In Exercises 120, evaluate each expression, or...Ch. 7.1 - Prob. 16ECh. 7.1 - Prob. 17ECh. 7.1 - In Exercises 120, evaluate each expression, or...Ch. 7.1 - Prob. 19ECh. 7.1 - Prob. 20ECh. 7.1 - In Exercises 21–26, find the indicated function...Ch. 7.1 - Prob. 22ECh. 7.1 - Prob. 23ECh. 7.1 - In Exercises 2126, find the indicated function...Ch. 7.1 - Prob. 25ECh. 7.1 - Prob. 26ECh. 7.1 - In Exercises 2732, find the domain of each square...Ch. 7.1 - In Exercises 27–32, find the domain of each square...Ch. 7.1 - In Exercises 27–32, find the domain of each square...Ch. 7.1 - In Exercises 2732, find the domain of each square...Ch. 7.1 - In Exercises 27–32, find the domain of each square...Ch. 7.1 - Prob. 32ECh. 7.1 - In Exercises 33–46, simplify each...Ch. 7.1 - Prob. 34ECh. 7.1 - Prob. 35ECh. 7.1 - In Exercises 33–46, simplify each...Ch. 7.1 - Prob. 37ECh. 7.1 - Prob. 38ECh. 7.1 - In Exercises 33–46, simplify each...Ch. 7.1 - Prob. 40ECh. 7.1 - Prob. 41ECh. 7.1 - In Exercises 3346, simplify each expression. 46x6Ch. 7.1 - Prob. 43ECh. 7.1 - Prob. 44ECh. 7.1 - In Exercises 33–46, simplify each...Ch. 7.1 - Prob. 46ECh. 7.1 - Prob. 47ECh. 7.1 - In Exercises 47–54, find each cube root. 48. Ch. 7.1 - Prob. 49ECh. 7.1 - Prob. 50ECh. 7.1 - In Exercises 47–54, find each cube root. 51. Ch. 7.1 - Prob. 52ECh. 7.1 - Prob. 53ECh. 7.1 - In Exercises 4754, find each cube root. 81253Ch. 7.1 - Prob. 55ECh. 7.1 - Prob. 56ECh. 7.1 - Prob. 57ECh. 7.1 - Prob. 58ECh. 7.1 - Prob. 59ECh. 7.1 - In Exercises 59–76, find the indicated root, or...Ch. 7.1 - In Exercises 59–76, find the indicated root, or...Ch. 7.1 - Prob. 62ECh. 7.1 - In Exercises 5976, find the indicated root, or...Ch. 7.1 - Prob. 64ECh. 7.1 - Prob. 65ECh. 7.1 - In Exercises 59–76, find the indicated root, or...Ch. 7.1 - Prob. 67ECh. 7.1 - Prob. 68ECh. 7.1 - In Exercises 59–76, find the indicated root, or...Ch. 7.1 - Prob. 70ECh. 7.1 - Prob. 71ECh. 7.1 - In Exercises 59–76, find the indicated root, or...Ch. 7.1 - Prob. 73ECh. 7.1 - Prob. 74ECh. 7.1 - In Exercises 59–76, find the indicated root, or...Ch. 7.1 - Prob. 76ECh. 7.1 - Prob. 77ECh. 7.1 - In Exercises 7790, simply each expression. Include...Ch. 7.1 - Prob. 79ECh. 7.1 - Prob. 80ECh. 7.1 - In Exercises 7790, simply each expression. Include...Ch. 7.1 - Prob. 82ECh. 7.1 - Prob. 83ECh. 7.1 - In Exercises 77–90, simply each expression....Ch. 7.1 - Prob. 85ECh. 7.1 - Prob. 86ECh. 7.1 - In Exercises 7790, simply each expression. Include...Ch. 7.1 - Prob. 88ECh. 7.1 - Prob. 89ECh. 7.1 - In Exercises 77–90, simply each expression....Ch. 7.1 - Prob. 91ECh. 7.1 - Prob. 92ECh. 7.1 - Prob. 93ECh. 7.1 - Prob. 94ECh. 7.1 - Prob. 95ECh. 7.1 - Prob. 96ECh. 7.1 - Prob. 97ECh. 7.1 - Prob. 98ECh. 7.1 - Prob. 99ECh. 7.1 - Prob. 100ECh. 7.1 - Prob. 101ECh. 7.1 - 102. The function models the median height, , in...Ch. 7.1 - Prob. 103ECh. 7.1 - Prob. 104ECh. 7.1 - Prob. 105ECh. 7.1 - Prob. 106ECh. 7.1 - Prob. 107ECh. 7.1 - Prob. 108ECh. 7.1 - Prob. 109ECh. 7.1 - Prob. 110ECh. 7.1 - Prob. 111ECh. 7.1 - Prob. 112ECh. 7.1 - Prob. 113ECh. 7.1 - Prob. 114ECh. 7.1 - Prob. 115ECh. 7.1 - Prob. 116ECh. 7.1 - Prob. 117ECh. 7.1 - Prob. 118ECh. 7.1 - Prob. 119ECh. 7.1 - Prob. 120ECh. 7.1 - Prob. 121ECh. 7.1 - Prob. 122ECh. 7.1 - Prob. 123ECh. 7.1 - Prob. 124ECh. 7.1 - Prob. 125ECh. 7.1 - Prob. 126ECh. 7.1 - Prob. 127ECh. 7.1 - Let f(x)=x3 and g(x)=x+1. Find the domain of f+g...Ch. 7.1 - Prob. 129ECh. 7.1 - Prob. 130ECh. 7.1 - Prob. 131ECh. 7.1 - Prob. 132ECh. 7.1 - Prob. 133ECh. 7.1 - Prob. 134ECh. 7.1 - Prob. 135ECh. 7.1 - Prob. 136ECh. 7.1 - Prob. 137ECh. 7.2 - Prob. 1CPCh. 7.2 - Prob. 2CPCh. 7.2 - Prob. 3CPCh. 7.2 - Prob. 4CPCh. 7.2 - Prob. 5CPCh. 7.2 - Prob. 6CPCh. 7.2 - Prob. 7CPCh. 7.2 - Prob. 1CVCCh. 7.2 - Prob. 2CVCCh. 7.2 - Prob. 3CVCCh. 7.2 - Prob. 4CVCCh. 7.2 - Prob. 5CVCCh. 7.2 - Prob. 6CVCCh. 7.2 - Prob. 7CVCCh. 7.2 - Prob. 1ECh. 7.2 - Prob. 2ECh. 7.2 - In Exercises 1–20, use radical notation to rewrite...Ch. 7.2 - Prob. 4ECh. 7.2 - Prob. 5ECh. 7.2 - In Exercises 1–20, use radical notation to rewrite...Ch. 7.2 - Prob. 7ECh. 7.2 - Prob. 8ECh. 7.2 - Prob. 9ECh. 7.2 - Prob. 10ECh. 7.2 - Prob. 11ECh. 7.2 - Prob. 12ECh. 7.2 - Prob. 13ECh. 7.2 - Prob. 14ECh. 7.2 - In Exercises 1–20, use radical notation to rewrite...Ch. 7.2 - Prob. 16ECh. 7.2 - In Exercises 1–20, use radical notation to rewrite...Ch. 7.2 - Prob. 18ECh. 7.2 - Prob. 19ECh. 7.2 - Prob. 20ECh. 7.2 - In Exercises 2138, rewrite each expression with...Ch. 7.2 - Prob. 22ECh. 7.2 - Prob. 23ECh. 7.2 - In Exercises 2138, rewrite each expression with...Ch. 7.2 - Prob. 25ECh. 7.2 - Prob. 26ECh. 7.2 - In Exercises 2138, rewrite each expression with...Ch. 7.2 - Prob. 28ECh. 7.2 - Prob. 29ECh. 7.2 - In Exercises 21–38, rewrite each expression with...Ch. 7.2 - Prob. 31ECh. 7.2 - Prob. 32ECh. 7.2 - In Exercises 21–38, rewrite each expression with...Ch. 7.2 - Prob. 34ECh. 7.2 - Prob. 35ECh. 7.2 - In Exercises 2138, rewrite each expression with...Ch. 7.2 - In Exercises 21–38, rewrite each expression with...Ch. 7.2 - Prob. 38ECh. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - Prob. 40ECh. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - Prob. 43ECh. 7.2 - Prob. 44ECh. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - Prob. 46ECh. 7.2 - Prob. 47ECh. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - In Exercises 3954, rewrite each expression with a...Ch. 7.2 - Prob. 50ECh. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - Prob. 52ECh. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - In Exercises 39–54, rewrite each expression with a...Ch. 7.2 - Prob. 55ECh. 7.2 - Prob. 56ECh. 7.2 - In Exercises 5578, use properties of rational...Ch. 7.2 - Prob. 58ECh. 7.2 - Prob. 59ECh. 7.2 - In Exercises 55–78, use properties of rational...Ch. 7.2 - In Exercises 55–78, use properties of rational...Ch. 7.2 - Prob. 62ECh. 7.2 - In Exercises 5578, use properties of rational...Ch. 7.2 - Prob. 64ECh. 7.2 - In Exercises 5578, use properties of rational...Ch. 7.2 - Prob. 66ECh. 7.2 - Prob. 67ECh. 7.2 - Prob. 68ECh. 7.2 - In Exercises 5578, use properties of rational...Ch. 7.2 - Prob. 70ECh. 7.2 - Prob. 71ECh. 7.2 - In Exercises 5578, use properties of rational...Ch. 7.2 - In Exercises 55–78, use properties of rational...Ch. 7.2 - Prob. 74ECh. 7.2 - In Exercises 5578, use properties of rational...Ch. 7.2 - Prob. 76ECh. 7.2 - In Exercises 55–78, use properties of rational...Ch. 7.2 - In Exercises 55–78, use properties of rational...Ch. 7.2 - Prob. 79ECh. 7.2 - Prob. 80ECh. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - Prob. 82ECh. 7.2 - Prob. 83ECh. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - Prob. 86ECh. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - Prob. 88ECh. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - Prob. 92ECh. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - Prob. 94ECh. 7.2 - Prob. 95ECh. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - Prob. 98ECh. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - Prob. 100ECh. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - Prob. 103ECh. 7.2 - Prob. 104ECh. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - Prob. 106ECh. 7.2 - Prob. 107ECh. 7.2 - In Exercises 79112, use rational exponents to...Ch. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - Prob. 110ECh. 7.2 - In Exercises 79–112, use rational exponents to...Ch. 7.2 - Prob. 112ECh. 7.2 - Prob. 113ECh. 7.2 - Prob. 114ECh. 7.2 - Prob. 115ECh. 7.2 - Prob. 116ECh. 7.2 - In Exercises 117–120, factor out the greatest...Ch. 7.2 - Prob. 118ECh. 7.2 - Prob. 119ECh. 7.2 - In Exercises 117–120, factor out the greatest...Ch. 7.2 - Prob. 121ECh. 7.2 - Prob. 122ECh. 7.2 - In Exercises 121124, simplify each expression....Ch. 7.2 - Prob. 124ECh. 7.2 - Prob. 125ECh. 7.2 - Prob. 126ECh. 7.2 - Prob. 127ECh. 7.2 - Prob. 128ECh. 7.2 - The way that we perceive the temperature on a cold...Ch. 7.2 - Prob. 130ECh. 7.2 - Prob. 131ECh. 7.2 - Prob. 132ECh. 7.2 - Prob. 133ECh. 7.2 - Prob. 134ECh. 7.2 - Prob. 135ECh. 7.2 - What is the meaning of amn ? Give an example.Ch. 7.2 - Prob. 137ECh. 7.2 - 138. Explain why is negative when is odd and is...Ch. 7.2 - Prob. 139ECh. 7.2 - Prob. 140ECh. 7.2 - Prob. 141ECh. 7.2 - Prob. 142ECh. 7.2 - Prob. 143ECh. 7.2 - Prob. 144ECh. 7.2 - Prob. 145ECh. 7.2 - Prob. 146ECh. 7.2 - Prob. 147ECh. 7.2 - Prob. 148ECh. 7.2 - Prob. 149ECh. 7.2 - Prob. 150ECh. 7.2 - Prob. 151ECh. 7.2 - Prob. 152ECh. 7.2 - Prob. 153ECh. 7.2 - Prob. 154ECh. 7.2 - Prob. 155ECh. 7.2 - Prob. 156ECh. 7.2 - Prob. 157ECh. 7.2 - Prob. 158ECh. 7.2 - Prob. 159ECh. 7.2 - Prob. 160ECh. 7.2 - Graph y32x+3.Ch. 7.2 - Prob. 162ECh. 7.2 - Prob. 163ECh. 7.2 - Prob. 164ECh. 7.2 - Simplify: a. x213 b. y246Ch. 7.3 - Check Point 1 Multiply: a. 511 b. x+4x4 c. 63103...Ch. 7.3 - Prob. 2CPCh. 7.3 - Prob. 3CPCh. 7.3 - Prob. 4CPCh. 7.3 - Prob. 5CPCh. 7.3 - Prob. 6CPCh. 7.3 - Prob. 7CPCh. 7.3 - Prob. 1CVCCh. 7.3 - Prob. 2CVCCh. 7.3 - Prob. 3CVCCh. 7.3 - Prob. 4CVCCh. 7.3 - Prob. 5CVCCh. 7.3 - Prob. 1ECh. 7.3 - Prob. 2ECh. 7.3 - In Exercises 120, use the product rule to...Ch. 7.3 - Prob. 4ECh. 7.3 - Prob. 5ECh. 7.3 - In Exercises 120, use the product rule to...Ch. 7.3 - Prob. 7ECh. 7.3 - Prob. 8ECh. 7.3 - In Exercises 1–20, use the product rule to...Ch. 7.3 - Prob. 10ECh. 7.3 - In Exercises 120, use the product rule to...Ch. 7.3 - In Exercises 120, use the product rule to...Ch. 7.3 - In Exercises 120, use the product rule to...Ch. 7.3 - Prob. 14ECh. 7.3 - In Exercises 1–20, use the product rule to...Ch. 7.3 - Prob. 16ECh. 7.3 - In Exercises 1–20, use the product rule to...Ch. 7.3 - In Exercises 1–20, use the product rule to...Ch. 7.3 - In Exercises 1–20, use the product rule to...Ch. 7.3 - Prob. 20ECh. 7.3 - In Exercises 21–32, simplify by factoring. 21. Ch. 7.3 - Prob. 22ECh. 7.3 - In Exercises 21–32, simplify by factoring. 23. Ch. 7.3 - In Exercises 2132, simplify by factoring. 28Ch. 7.3 - In Exercises 2132, simplify by factoring. 75xCh. 7.3 - Prob. 26ECh. 7.3 - In Exercises 21–32, simplify by factoring. 27. Ch. 7.3 - Prob. 28ECh. 7.3 - In Exercises 21–32, simplify by factoring. 29. Ch. 7.3 - In Exercises 2132, simplify by factoring. 250x33Ch. 7.3 - In Exercises 2132, simplify by factoring. 16x2y33Ch. 7.3 - Prob. 32ECh. 7.3 - In Exercises 33–38, express the function, , in...Ch. 7.3 - Prob. 34ECh. 7.3 - In Exercises 3338, express the function, f, in...Ch. 7.3 - In Exercises 33–38, express the function, , in...Ch. 7.3 - In Exercises 33–38, express the function, , in...Ch. 7.3 - Prob. 38ECh. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - Prob. 40ECh. 7.3 - In Exercises 3960, simplify by factoring. Assume...Ch. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - Prob. 44ECh. 7.3 - In Exercises 3960, simplify by factoring. Assume...Ch. 7.3 - Prob. 46ECh. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - Prob. 50ECh. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - Prob. 52ECh. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - In Exercises 3960, simplify by factoring. Assume...Ch. 7.3 - In Exercises 3960, simplify by factoring. Assume...Ch. 7.3 - Prob. 56ECh. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - Prob. 58ECh. 7.3 - In Exercises 39–60, simplify by factoring. Assume...Ch. 7.3 - In Exercises 3960, simplify by factoring. Assume...Ch. 7.3 - In Exercises 6182, multiply and simplify. Assume...Ch. 7.3 - Prob. 62ECh. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - Prob. 64ECh. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - In Exercises 6182, multiply and simplify. Assume...Ch. 7.3 - In Exercises 6182, multiply and simplify. Assume...Ch. 7.3 - Prob. 68ECh. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - Prob. 70ECh. 7.3 - In Exercises 6182, multiply and simplify. Assume...Ch. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - Prob. 74ECh. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - Prob. 76ECh. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - In Exercises 61–82, multiply and simplify. Assume...Ch. 7.3 - Prob. 80ECh. 7.3 - In Exercises 6182, multiply and simplify. Assume...Ch. 7.3 - Prob. 82ECh. 7.3 - In Exercises 83–90, simplify each expression....Ch. 7.3 - Prob. 84ECh. 7.3 - In Exercises 8390, simplify each expression....Ch. 7.3 - In Exercises 8390, simplify each expression....Ch. 7.3 - In Exercises 8390, simplify each expression....Ch. 7.3 - Prob. 88ECh. 7.3 - In Exercises 8390, simplify each expression....Ch. 7.3 - Prob. 90ECh. 7.3 - Prob. 91ECh. 7.3 - Prob. 92ECh. 7.3 - Prob. 93ECh. 7.3 - Prob. 94ECh. 7.3 - Your cardiac index is your heart’s output, in...Ch. 7.3 - Prob. 96ECh. 7.3 - Prob. 97ECh. 7.3 - Prob. 98ECh. 7.3 - Prob. 99ECh. 7.3 - Prob. 100ECh. 7.3 - Prob. 101ECh. 7.3 - Prob. 102ECh. 7.3 - Prob. 103ECh. 7.3 - Prob. 104ECh. 7.3 - Prob. 105ECh. 7.3 - Prob. 106ECh. 7.3 - Prob. 107ECh. 7.3 - Prob. 108ECh. 7.3 - Prob. 109ECh. 7.3 - Prob. 110ECh. 7.3 - Prob. 111ECh. 7.3 - Prob. 112ECh. 7.3 - Prob. 113ECh. 7.3 - Prob. 114ECh. 7.3 - Prob. 115ECh. 7.3 - Prob. 116ECh. 7.3 - Prob. 117ECh. 7.3 - Prob. 118ECh. 7.3 - Prob. 119ECh. 7.3 - Prob. 120ECh. 7.3 - Prob. 121ECh. 7.3 - Exercises 122124, will help you prepare for the...Ch. 7.3 - Prob. 123ECh. 7.3 - Exercises 122–124, will help you prepare for the...Ch. 7.4 - Check Point 1 Simplify by combining like radical...Ch. 7.4 - Prob. 2CPCh. 7.4 - Prob. 3CPCh. 7.4 - Prob. 4CPCh. 7.4 - Prob. 5CPCh. 7.4 - Prob. 1CVCCh. 7.4 - Prob. 2CVCCh. 7.4 - Prob. 3CVCCh. 7.4 - Fill in each blank so that the resulting statement...Ch. 7.4 - Fill in each blank so that the resulting statement...Ch. 7.4 - Prob. 6CVCCh. 7.4 - In Exercises 1–10, add or subtract as...Ch. 7.4 - Prob. 2ECh. 7.4 - In Exercises 110, add or subtract as indicated....Ch. 7.4 - Prob. 4ECh. 7.4 - In Exercises 1–10, add or subtract as...Ch. 7.4 - Prob. 6ECh. 7.4 - In Exercises 1–10, add or subtract as...Ch. 7.4 - Prob. 8ECh. 7.4 - In Exercises 1–10, add or subtract as...Ch. 7.4 - Prob. 10ECh. 7.4 - Prob. 11ECh. 7.4 - Prob. 12ECh. 7.4 - Prob. 13ECh. 7.4 - Prob. 14ECh. 7.4 - Prob. 15ECh. 7.4 - Prob. 16ECh. 7.4 - Prob. 17ECh. 7.4 - Prob. 18ECh. 7.4 - Prob. 19ECh. 7.4 - Prob. 20ECh. 7.4 - Prob. 21ECh. 7.4 - Prob. 22ECh. 7.4 - Prob. 23ECh. 7.4 - Prob. 24ECh. 7.4 - In Exercises 1128, add or subtract as indicated....Ch. 7.4 - Prob. 26ECh. 7.4 - Prob. 27ECh. 7.4 - Prob. 28ECh. 7.4 - In Exercises 2944, simplify using the quotient...Ch. 7.4 - Prob. 30ECh. 7.4 - In Exercises 29–44, simplify using the quotient...Ch. 7.4 - Prob. 32ECh. 7.4 - In Exercises 2944, simplify using the quotient...Ch. 7.4 - In Exercises 29–44, simplify using the quotient...Ch. 7.4 - In Exercises 2944, simplify using the quotient...Ch. 7.4 - Prob. 36ECh. 7.4 - In Exercises 2944, simplify using the quotient...Ch. 7.4 - Prob. 38ECh. 7.4 - In Exercises 2944, simplify using the quotient...Ch. 7.4 - Prob. 40ECh. 7.4 - In Exercises 29–44, simplify using the quotient...Ch. 7.4 - Prob. 42ECh. 7.4 - In Exercises 2944, simplify using the quotient...Ch. 7.4 - Prob. 44ECh. 7.4 - Prob. 45ECh. 7.4 - Prob. 46ECh. 7.4 - In Exercises 4566, divide and, if possible,...Ch. 7.4 - Prob. 48ECh. 7.4 - In Exercises 45–66, divide and, if possible,...Ch. 7.4 - Prob. 50ECh. 7.4 - In Exercises 45–66, divide and, if possible,...Ch. 7.4 - Prob. 52ECh. 7.4 - In Exercises 45–66, divide and, if possible,...Ch. 7.4 - Prob. 54ECh. 7.4 - In Exercises 4566, divide and, if possible,...Ch. 7.4 - Prob. 56ECh. 7.4 - In Exercises 4566, divide and, if possible,...Ch. 7.4 - Prob. 58ECh. 7.4 - In Exercises 45–66, divide and, if possible,...Ch. 7.4 - Prob. 60ECh. 7.4 - In Exercises 4566, divide and, if possible,...Ch. 7.4 - Prob. 62ECh. 7.4 - In Exercises 45–66, divide and, if possible,...Ch. 7.4 - Prob. 64ECh. 7.4 - In Exercises 45–66, divide and, if possible,...Ch. 7.4 - Prob. 66ECh. 7.4 - In Exercises 6776, perform the indicated...Ch. 7.4 - Prob. 68ECh. 7.4 - In Exercises 6776, perform the indicated...Ch. 7.4 - Prob. 70ECh. 7.4 - In Exercises 67–76, perform the indicated...Ch. 7.4 - Prob. 72ECh. 7.4 - In Exercises 67–76, perform the indicated...Ch. 7.4 - Prob. 74ECh. 7.4 - In Exercises 6776, perform the indicated...Ch. 7.4 - Prob. 76ECh. 7.4 - Prob. 77ECh. 7.4 - Prob. 78ECh. 7.4 - Prob. 79ECh. 7.4 - In Exercises 7780, find (fg)(x) and the domain of...Ch. 7.4 - Exercises 8184 involve the perimeter and area of...Ch. 7.4 - Exercises 81–84 involve the perimeter and area of...Ch. 7.4 - Exercises 8184 involve the perimeter and area of...Ch. 7.4 - Exercises 8184 involve the perimeter and area of...Ch. 7.4 - 85. America is getting older. The graph shows the...Ch. 7.4 - Prob. 86ECh. 7.4 - Prob. 87ECh. 7.4 - Prob. 88ECh. 7.4 - Prob. 89ECh. 7.4 - Prob. 90ECh. 7.4 - Prob. 91ECh. 7.4 - Prob. 92ECh. 7.4 - Prob. 93ECh. 7.4 - Prob. 94ECh. 7.4 - Prob. 95ECh. 7.4 - Prob. 96ECh. 7.4 - Prob. 97ECh. 7.4 - Prob. 98ECh. 7.4 - Prob. 99ECh. 7.4 - Prob. 100ECh. 7.4 - Prob. 101ECh. 7.4 - Prob. 102ECh. 7.4 - Prob. 103ECh. 7.4 - Prob. 104ECh. 7.4 - Prob. 105ECh. 7.4 - Prob. 106ECh. 7.4 - Prob. 107ECh. 7.4 - Prob. 108ECh. 7.4 - Prob. 109ECh. 7.4 - Prob. 110ECh. 7.4 - Prob. 111ECh. 7.4 - Prob. 112ECh. 7.4 - Prob. 113ECh. 7.4 - Prob. 1MCCPCh. 7.4 - Prob. 2MCCPCh. 7.4 - Prob. 3MCCPCh. 7.4 - Prob. 4MCCPCh. 7.4 - Prob. 5MCCPCh. 7.4 - Prob. 6MCCPCh. 7.4 - Prob. 7MCCPCh. 7.4 - Prob. 8MCCPCh. 7.4 - Prob. 9MCCPCh. 7.4 - Prob. 10MCCPCh. 7.4 - Prob. 11MCCPCh. 7.4 - Prob. 12MCCPCh. 7.4 - Prob. 13MCCPCh. 7.4 - In Exercises 123, simplify the given expression or...Ch. 7.4 - Prob. 15MCCPCh. 7.4 - Prob. 16MCCPCh. 7.4 - Prob. 17MCCPCh. 7.4 - Prob. 18MCCPCh. 7.4 - In Exercises 1–23, simplify the given expression...Ch. 7.4 - Prob. 20MCCPCh. 7.4 - Prob. 21MCCPCh. 7.4 - Prob. 22MCCPCh. 7.4 - Prob. 23MCCPCh. 7.4 - Prob. 24MCCPCh. 7.4 - In Exercises 2425, find the domain of each...Ch. 7.5 - Check Point 1 Multiply: a. b. c. Ch. 7.5 - Prob. 2CPCh. 7.5 - Prob. 3CPCh. 7.5 - Prob. 4CPCh. 7.5 - Prob. 5CPCh. 7.5 - Prob. 6CPCh. 7.5 - Prob. 7CPCh. 7.5 - Prob. 1CVCCh. 7.5 - Prob. 2CVCCh. 7.5 - Prob. 3CVCCh. 7.5 - Prob. 4CVCCh. 7.5 - Prob. 5CVCCh. 7.5 - Prob. 6CVCCh. 7.5 - Prob. 7CVCCh. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - Prob. 3ECh. 7.5 - Prob. 4ECh. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - Prob. 7ECh. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - Prob. 12ECh. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - Prob. 19ECh. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - Prob. 23ECh. 7.5 - Prob. 24ECh. 7.5 - Prob. 25ECh. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - Prob. 27ECh. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - In Exercises 1–38, multiply as indicated. If...Ch. 7.5 - Prob. 35ECh. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 138, multiply as indicated. If...Ch. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - In Exercises 3964, rationalize each denominator....Ch. 7.5 - Prob. 41ECh. 7.5 - Prob. 42ECh. 7.5 - In Exercises 3964, rationalize each denominator....Ch. 7.5 - In Exercises 3964, rationalize each denominator....Ch. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - Prob. 46ECh. 7.5 - In Exercises 3964, rationalize each denominator....Ch. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - In Exercises 3964, rationalize each denominator....Ch. 7.5 - Prob. 50ECh. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - Prob. 52ECh. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - Prob. 56ECh. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - Prob. 58ECh. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - Prob. 62ECh. 7.5 - In Exercises 39–64, rationalize each...Ch. 7.5 - Prob. 64ECh. 7.5 - In Exercises 65–74, simplify each radical...Ch. 7.5 - In Exercises 65–74, simplify each radical...Ch. 7.5 - In Exercises 65–74, simplify each radical...Ch. 7.5 - Prob. 68ECh. 7.5 - In Exercises 6574, simplify each radical...Ch. 7.5 - Prob. 70ECh. 7.5 - In Exercises 65–74, simplify each radical...Ch. 7.5 - Prob. 72ECh. 7.5 - Prob. 73ECh. 7.5 - Prob. 74ECh. 7.5 - Prob. 75ECh. 7.5 - Prob. 76ECh. 7.5 - In Exercises 75–92, rationalize each denominator....Ch. 7.5 - In Exercises 75–92, rationalize each denominator....Ch. 7.5 - In Exercises 7592, rationalize each denominator....Ch. 7.5 - Prob. 80ECh. 7.5 - In Exercises 7592, rationalize each denominator....Ch. 7.5 - Prob. 82ECh. 7.5 - Prob. 83ECh. 7.5 - In Exercises 7592, rationalize each denominator....Ch. 7.5 - In Exercises 75–92, rationalize each denominator....Ch. 7.5 - Prob. 86ECh. 7.5 - In Exercises 75–92, rationalize each denominator....Ch. 7.5 - Prob. 88ECh. 7.5 - In Exercises 7592, rationalize each denominator....Ch. 7.5 - In Exercises 75–92, rationalize each denominator....Ch. 7.5 - In Exercises 7592, rationalize each denominator....Ch. 7.5 - Prob. 92ECh. 7.5 - In Exercises 93–104, rationalize each numerator....Ch. 7.5 - Prob. 94ECh. 7.5 - In Exercises 93104, rationalize each numerator....Ch. 7.5 - Prob. 96ECh. 7.5 - In Exercises 93–104, rationalize each numerator....Ch. 7.5 - Prob. 98ECh. 7.5 - Prob. 99ECh. 7.5 - Prob. 100ECh. 7.5 - In Exercises 93–104, rationalize each numerator....Ch. 7.5 - Prob. 102ECh. 7.5 - In Exercises 93104, rationalize each numerator....Ch. 7.5 - In Exercises 93–104, rationalize each numerator....Ch. 7.5 - Prob. 105ECh. 7.5 - Prob. 106ECh. 7.5 - In Exercises 105112, add or subtract as indicated....Ch. 7.5 - In Exercises 105–112, add or subtract as...Ch. 7.5 - In Exercises 105–112, add or subtract as...Ch. 7.5 - In Exercises 105112, add or subtract as indicated....Ch. 7.5 - In Exercises 105112, add or subtract as indicated....Ch. 7.5 - Prob. 112ECh. 7.5 - Prob. 113ECh. 7.5 - Prob. 114ECh. 7.5 - Prob. 115ECh. 7.5 - Prob. 116ECh. 7.5 - Prob. 117ECh. 7.5 - Prob. 118ECh. 7.5 - In Exercises 119120, write expressions for the...Ch. 7.5 - Prob. 120ECh. 7.5 - Prob. 121ECh. 7.5 - The Pythagorean Theorem for right triangles tells...Ch. 7.5 - Prob. 123ECh. 7.5 - Prob. 124ECh. 7.5 - Prob. 125ECh. 7.5 - Prob. 126ECh. 7.5 - Prob. 127ECh. 7.5 - Prob. 128ECh. 7.5 - 129. When a radical expression has its denominator...Ch. 7.5 - Prob. 130ECh. 7.5 - Prob. 131ECh. 7.5 - Prob. 132ECh. 7.5 - Prob. 133ECh. 7.5 - Prob. 134ECh. 7.5 - Prob. 135ECh. 7.5 - Prob. 136ECh. 7.5 - Prob. 137ECh. 7.5 - Make Sense? In Exercises 135–138, determine...Ch. 7.5 - Prob. 139ECh. 7.5 - Prob. 140ECh. 7.5 - Prob. 141ECh. 7.5 - Prob. 142ECh. 7.5 - Prob. 143ECh. 7.5 - Prob. 144ECh. 7.5 - Prob. 145ECh. 7.5 - Prob. 146ECh. 7.5 - Prob. 147ECh. 7.5 - Prob. 148ECh. 7.5 - Prob. 149ECh. 7.5 - Prob. 150ECh. 7.5 - Exercises 149–151 will help you prepare for the...Ch. 7.6 - Prob. 1CPCh. 7.6 - Prob. 2CPCh. 7.6 - Prob. 3CPCh. 7.6 - Prob. 4CPCh. 7.6 - Prob. 5CPCh. 7.6 - Prob. 6CPCh. 7.6 - Prob. 1CVCCh. 7.6 - Prob. 2CVCCh. 7.6 - Prob. 3CVCCh. 7.6 - Prob. 4CVCCh. 7.6 - Prob. 5CVCCh. 7.6 - Prob. 6CVCCh. 7.6 - Prob. 7CVCCh. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - Prob. 6ECh. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - Prob. 10ECh. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - Prob. 14ECh. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - Prob. 16ECh. 7.6 - Prob. 17ECh. 7.6 - Prob. 18ECh. 7.6 - Prob. 19ECh. 7.6 - Prob. 20ECh. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - Prob. 22ECh. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - Prob. 24ECh. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - Prob. 26ECh. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - Prob. 28ECh. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - Prob. 30ECh. 7.6 - In Exercises 138, solve each radical equation....Ch. 7.6 - Prob. 32ECh. 7.6 - Prob. 33ECh. 7.6 - In Exercises 1–38, solve each radical...Ch. 7.6 - Prob. 35ECh. 7.6 - Prob. 36ECh. 7.6 - Prob. 37ECh. 7.6 - Prob. 38ECh. 7.6 - Prob. 39ECh. 7.6 - Prob. 40ECh. 7.6 - Prob. 41ECh. 7.6 - Prob. 42ECh. 7.6 - Prob. 43ECh. 7.6 - Prob. 44ECh. 7.6 - Prob. 45ECh. 7.6 - Prob. 46ECh. 7.6 - Prob. 47ECh. 7.6 - Prob. 48ECh. 7.6 - Prob. 49ECh. 7.6 - Prob. 50ECh. 7.6 - Prob. 51ECh. 7.6 - Prob. 52ECh. 7.6 - Prob. 53ECh. 7.6 - Prob. 54ECh. 7.6 - Prob. 55ECh. 7.6 - Prob. 56ECh. 7.6 - Prob. 57ECh. 7.6 - Prob. 58ECh. 7.6 - Prob. 59ECh. 7.6 - Prob. 60ECh. 7.6 - Prob. 61ECh. 7.6 - Prob. 62ECh. 7.6 - Prob. 63ECh. 7.6 - Prob. 64ECh. 7.6 - Prob. 65ECh. 7.6 - In Example 6 of the section, we used a square root...Ch. 7.6 - Prob. 67ECh. 7.6 - Prob. 68ECh. 7.6 - Prob. 69ECh. 7.6 - In Exercises 6872, use a graphing utility to solve...Ch. 7.6 - In Exercises 6872, use a graphing utility to solve...Ch. 7.6 - Prob. 72ECh. 7.6 - Prob. 73ECh. 7.6 - Prob. 74ECh. 7.6 - Prob. 75ECh. 7.6 - Prob. 76ECh. 7.6 - Prob. 77ECh. 7.6 - Prob. 78ECh. 7.6 - Prob. 79ECh. 7.6 - In Exercises 77–80, determine whether each...Ch. 7.6 - 81. Find the length of the three sides of the...Ch. 7.6 - In Exercises 82–84, solve each equation. 82. Ch. 7.6 - In Exercises 8284, solve each equation. x+x+9=3Ch. 7.6 - Prob. 84ECh. 7.6 - Prob. 85ECh. 7.6 - Prob. 86ECh. 7.6 - Prob. 87ECh. 7.6 - Exercises 8890 will help you prepare for the...Ch. 7.6 - Exercises 8890 will help you prepare for the...Ch. 7.6 - Exercises 88–90 will help you prepare for the...Ch. 7.7 - Prob. 1CPCh. 7.7 - Prob. 2CPCh. 7.7 - Prob. 3CPCh. 7.7 - Prob. 4CPCh. 7.7 - Check Point 5 Divide and simplify to the form Ch. 7.7 - Prob. 6CPCh. 7.7 - Prob. 7CPCh. 7.7 - Fill in each blank so that the resulting statement...Ch. 7.7 - Prob. 2CVCCh. 7.7 - Prob. 3CVCCh. 7.7 - Prob. 4CVCCh. 7.7 - Prob. 5CVCCh. 7.7 - Prob. 6CVCCh. 7.7 - Prob. 7CVCCh. 7.7 - Prob. 8CVCCh. 7.7 - Prob. 9CVCCh. 7.7 - Prob. 10CVCCh. 7.7 - Prob. 11CVCCh. 7.7 - Prob. 1ECh. 7.7 - Prob. 2ECh. 7.7 - Prob. 3ECh. 7.7 - Prob. 4ECh. 7.7 - Prob. 5ECh. 7.7 - Prob. 6ECh. 7.7 - Prob. 7ECh. 7.7 - Prob. 8ECh. 7.7 - Prob. 9ECh. 7.7 - Prob. 10ECh. 7.7 - Prob. 11ECh. 7.7 - Prob. 12ECh. 7.7 - Prob. 13ECh. 7.7 - Prob. 14ECh. 7.7 - Prob. 15ECh. 7.7 - Prob. 16ECh. 7.7 - In Exercises 1732, add or subtract as indicated....Ch. 7.7 - Prob. 18ECh. 7.7 - In Exercises 1732, add or subtract as indicated....Ch. 7.7 - Prob. 20ECh. 7.7 - In Exercises 17–32, add or subtract as indicated....Ch. 7.7 - Prob. 22ECh. 7.7 - In Exercises 17–32, add or subtract as indicated....Ch. 7.7 - Prob. 24ECh. 7.7 - In Exercises 1732, add or subtract as indicated....Ch. 7.7 - Prob. 26ECh. 7.7 - In Exercises 1732, add or subtract as indicated....Ch. 7.7 - Prob. 28ECh. 7.7 - In Exercises 17–32, add or subtract as indicated....Ch. 7.7 - Prob. 30ECh. 7.7 - In Exercises 1732, add or subtract as indicated....Ch. 7.7 - Prob. 32ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 34ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 36ECh. 7.7 - In Exercises 33–62, find each product. Write...Ch. 7.7 - Prob. 38ECh. 7.7 - In Exercises 33–62, find each product. Write...Ch. 7.7 - Prob. 40ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 42ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - In Exercises 33–62, find each product. Write...Ch. 7.7 - In Exercises 33–62, find each product. Write...Ch. 7.7 - Prob. 46ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 48ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 50ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 52ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 54ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 56ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 58ECh. 7.7 - In Exercises 33–62, find each product. Write...Ch. 7.7 - Prob. 60ECh. 7.7 - In Exercises 3362, find each product. Write...Ch. 7.7 - Prob. 62ECh. 7.7 - In Exercises 6384, divide and simplify to the form...Ch. 7.7 - Prob. 64ECh. 7.7 - In Exercises 63–84, divide and simplify to the...Ch. 7.7 - Prob. 66ECh. 7.7 - In Exercises 63–84, divide and simplify to the...Ch. 7.7 - Prob. 68ECh. 7.7 - In Exercises 6384, divide and simplify to the form...Ch. 7.7 - Prob. 70ECh. 7.7 - In Exercises 63–84, divide and simplify to the...Ch. 7.7 - Prob. 72ECh. 7.7 - In Exercises 63–84, divide and simplify to the...Ch. 7.7 - Prob. 74ECh. 7.7 - In Exercises 6384, divide and simplify to the form...Ch. 7.7 - Prob. 76ECh. 7.7 - In Exercises 6384, divide and simplify to the form...Ch. 7.7 - Prob. 78ECh. 7.7 - In Exercises 63–84, divide and simplify to the...Ch. 7.7 - Prob. 80ECh. 7.7 - In Exercises 6384, divide and simplify to the form...Ch. 7.7 - Prob. 82ECh. 7.7 - In Exercises 63–84, divide and simplify to the...Ch. 7.7 - Prob. 84ECh. 7.7 - In Exercises 85–100, simplify each...Ch. 7.7 - Prob. 86ECh. 7.7 - Prob. 87ECh. 7.7 - Prob. 88ECh. 7.7 - In Exercises 85100, simplify each expression. i22Ch. 7.7 - Prob. 90ECh. 7.7 - Prob. 91ECh. 7.7 - Prob. 92ECh. 7.7 - In Exercises 85–100, simplify each...Ch. 7.7 - Prob. 94ECh. 7.7 - Prob. 95ECh. 7.7 - Prob. 96ECh. 7.7 - In Exercises 85100, simplify each expression. (i)9Ch. 7.7 - Prob. 98ECh. 7.7 - Prob. 99ECh. 7.7 - Prob. 100ECh. 7.7 - In Exercises 101–108, perform the indicated...Ch. 7.7 - Prob. 102ECh. 7.7 - Prob. 103ECh. 7.7 - Prob. 104ECh. 7.7 - Prob. 105ECh. 7.7 - Prob. 106ECh. 7.7 - Prob. 107ECh. 7.7 - Prob. 108ECh. 7.7 - Prob. 109ECh. 7.7 - Prob. 110ECh. 7.7 - Prob. 111ECh. 7.7 - Prob. 112ECh. 7.7 - Prob. 113ECh. 7.7 - Prob. 114ECh. 7.7 - Prob. 115ECh. 7.7 - Prob. 116ECh. 7.7 - Prob. 117ECh. 7.7 - Prob. 118ECh. 7.7 - Prob. 119ECh. 7.7 - Prob. 120ECh. 7.7 - Prob. 121ECh. 7.7 - Prob. 122ECh. 7.7 - Prob. 123ECh. 7.7 - Prob. 124ECh. 7.7 - Prob. 125ECh. 7.7 - Prob. 126ECh. 7.7 - Prob. 127ECh. 7.7 - Prob. 128ECh. 7.7 - Prob. 129ECh. 7.7 - Prob. 131ECh. 7.7 - Prob. 132ECh. 7.7 - Prob. 133ECh. 7.7 - Prob. 134ECh. 7.7 - Prob. 135ECh. 7.7 - Make Sense? In Exercises 133136, determine whether...Ch. 7.7 - Prob. 137ECh. 7.7 - Prob. 138ECh. 7.7 - Prob. 139ECh. 7.7 - Prob. 140ECh. 7.7 - Prob. 141ECh. 7.7 - Prob. 142ECh. 7.7 - Prob. 143ECh. 7.7 - Prob. 144ECh. 7.7 - Prob. 145ECh. 7.7 - Prob. 146ECh. 7.7 - Prob. 147ECh. 7.7 - Prob. 148ECh. 7.7 - Prob. 149ECh. 7 - In Exercises 15, find the indicated root, or state...Ch. 7 - Prob. 2RECh. 7 - In Exercises 15, find the indicated root, or state...Ch. 7 - In Exercises 1–5, find the indicated root, or...Ch. 7 - Prob. 5RECh. 7 - In Exercises 6–7, find the indicated function...Ch. 7 - In Exercises 6–7, find the indicated function...Ch. 7 - Prob. 8RECh. 7 - In Exercises 89, find the domain of each square...Ch. 7 - In Exercises 1015, simplify each expression....Ch. 7 - Prob. 11RECh. 7 - In Exercises 10–15, simplify each expression....Ch. 7 - In Exercises 1015, simplify each expression....Ch. 7 - Prob. 14RECh. 7 - In Exercises 10–15, simplify each expression....Ch. 7 - Prob. 16RECh. 7 - Prob. 17RECh. 7 - Prob. 18RECh. 7 - In Exercises 1920, rewrite each expression with...Ch. 7 - In Exercises 1920, rewrite each expression with...Ch. 7 - In Exercises 21–22, rewrite each expression with a...Ch. 7 - In Exercises 21–22, rewrite each expression with a...Ch. 7 - Prob. 23RECh. 7 - In Exercises 2326, use properties of rational...Ch. 7 - Prob. 25RECh. 7 - Prob. 26RECh. 7 - In Exercises 27–31, use rational exponents to...Ch. 7 - In Exercises 2731, use rational exponents to...Ch. 7 - Prob. 29RECh. 7 - In Exercises 27–31, use rational exponents to...Ch. 7 - In Exercises 2731, use rational exponents to...Ch. 7 - Prob. 32RECh. 7 - In Exercises 33–35, use the product rule to...Ch. 7 - Prob. 34RECh. 7 - In Exercises 33–35, use the product rule to...Ch. 7 - If f(x)=7x214x+7, express the function, f, in...Ch. 7 - Prob. 37RECh. 7 - In Exercises 37–39, simplify by factoring. Assume...Ch. 7 - In Exercises 37–39, simplify by factoring. Assume...Ch. 7 - Prob. 40RECh. 7 - In Exercises 40–43, multiply and simplify, if...Ch. 7 - In Exercises 40–43, multiply and simplify, if...Ch. 7 - Prob. 43RECh. 7 - Assume that all variables represent positive real...Ch. 7 - Assume that all variables represent positive real...Ch. 7 - Prob. 46RECh. 7 - Assume that all variables represent positive real...Ch. 7 - In Exercises 4850, simplify using the quotient...Ch. 7 - Prob. 49RECh. 7 - In Exercises 4850, simplify using the quotient...Ch. 7 - In Exercises 5154, divide and, if possible,...Ch. 7 - Prob. 52RECh. 7 - In Exercises 5154, divide and, if possible,...Ch. 7 - In Exercises 51–54, simplify and, if possible,...Ch. 7 - Prob. 55RECh. 7 - Assume that all variables represent positive real...Ch. 7 - Assume that all variables represent positive real...Ch. 7 - Prob. 58RECh. 7 - Assume that all variables represent positive real...Ch. 7 - Assume that all variables represent positive real...Ch. 7 - Prob. 61RECh. 7 - Assume that all variables represent positive real...Ch. 7 - Prob. 63RECh. 7 - Prob. 64RECh. 7 - Prob. 65RECh. 7 - In Exercises 63–75, rationalize each denominator....Ch. 7 - Prob. 67RECh. 7 - In Exercises 63–75, rationalize each denominator....Ch. 7 - In Exercises 63–75, rationalize each denominator....Ch. 7 - Prob. 70RECh. 7 - Prob. 71RECh. 7 - In Exercises 6375, rationalize each denominator....Ch. 7 - Prob. 73RECh. 7 - In Exercises 6375, rationalize each denominator....Ch. 7 - In Exercises 6375, rationalize each denominator....Ch. 7 - Prob. 76RECh. 7 - In Exercises 76–79, rationalize each numerator....Ch. 7 - Prob. 78RECh. 7 - In Exercises 76–79, rationalize each numerator....Ch. 7 - In Exercises 80–84, solve each radical...Ch. 7 - In Exercises 80–84, solve each radical...Ch. 7 - In Exercises 80–84, solve each radical...Ch. 7 - In Exercises 8084, solve each radical equation....Ch. 7 - Prob. 84RECh. 7 - Prob. 85RECh. 7 - Prob. 86RECh. 7 - Prob. 87RECh. 7 - Prob. 88RECh. 7 - Prob. 89RECh. 7 - In Exercises 9099, perform the indicated...Ch. 7 - In Exercises 90–99, perform the indicated...Ch. 7 - Prob. 92RECh. 7 - In Exercises 9099, perform the indicated...Ch. 7 - Prob. 94RECh. 7 - Prob. 95RECh. 7 - Prob. 96RECh. 7 - In Exercises 90–99, perform the indicated...Ch. 7 - In Exercises 9099, perform the indicated...Ch. 7 - In Exercises 9099, perform the indicated...Ch. 7 - In Exercises 100101, simplify each expression. i16Ch. 7 - In Exercises 100–101, simplify each...Ch. 7 - Prob. 1TCh. 7 - Prob. 2TCh. 7 - Prob. 3TCh. 7 - Prob. 4TCh. 7 - Prob. 5TCh. 7 - Prob. 6TCh. 7 - Prob. 7TCh. 7 - Prob. 8TCh. 7 - Prob. 9TCh. 7 - Prob. 10TCh. 7 - Prob. 11TCh. 7 - Prob. 12TCh. 7 - Prob. 13TCh. 7 - Prob. 14TCh. 7 - Prob. 15TCh. 7 - Prob. 16TCh. 7 - Prob. 17TCh. 7 - Prob. 18TCh. 7 - Prob. 19TCh. 7 - Prob. 20TCh. 7 - Prob. 21TCh. 7 - Prob. 22TCh. 7 - Prob. 23TCh. 7 - Prob. 24TCh. 7 - Prob. 25TCh. 7 - Prob. 26TCh. 7 - Prob. 27TCh. 7 - Prob. 28TCh. 7 - Prob. 29TCh. 7 - Prob. 30TCh. 7 - Prob. 1CRECh. 7 - Prob. 2CRECh. 7 - Prob. 3CRECh. 7 - Prob. 4CRECh. 7 - Prob. 5CRECh. 7 - Prob. 6CRECh. 7 - Prob. 7CRECh. 7 - Prob. 8CRECh. 7 - Prob. 9CRECh. 7 - Prob. 10CRECh. 7 - Prob. 11CRECh. 7 - Prob. 12CRECh. 7 - Prob. 13CRECh. 7 - Prob. 14CRECh. 7 - Prob. 15CRECh. 7 - Prob. 16CRECh. 7 - Prob. 17CRECh. 7 - Prob. 18CRECh. 7 - Prob. 19CRECh. 7 - Prob. 20CRE
Knowledge Booster
Background pattern image
Algebra
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Text book image
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Text book image
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Text book image
College Algebra
Algebra
ISBN:9781337282291
Author:Ron Larson
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Inverse Functions; Author: Professor Dave Explains;https://www.youtube.com/watch?v=9fJsrnE1go0;License: Standard YouTube License, CC-BY