Chapter 7, Problem 7.1P

1. (a) Revisit Example 7-1. What is the error in assuming the concentration of species B is constant and what limits can you put on the calculated value of k? (I.e., k = 0.24 ±?)
2. (b) Revisit Example 7-3. Explain why the regression was carried out twice to find k′ and k.
3. (c) Revisit Example 7-4. Regress the data to lit the rate law

$r_{{\text{CH}}_4}=k{P}_{\text{CO}}^{\text{α}}{P}_{{\text{H}}_2}^{\text{β}}$

What is the difference in the correlation and sums of squares compared with those given in Example 7-4? Why was it necessary to regress the data twice, once to obtain Table E7-4.3 and once to obtain Table E7-4.4?

Interpretation Introduction

Interpretation:

The error in assuming the concentration of species B is constant and the limits that can be put over the calculated value of $k$ are to be stated.

Concept introduction:

The integral method is the quickest method to use to determine the rate law if the order turns out to zero, first, or second order. In the integral method, we guess the reaction order, α, in the combined batch reactor mole balance and rate law equation.

  $\frac{d{C}_{\text{A}}}{dt}=-k{C}_{\text{A}}^{\alpha }$

Integrate the differential equation to obtain the concentration as a function of time.  If the order we assume is correct, the appropriate plot of the concentration-time data should be linear. The integral method is used most often when the reaction order is known and it is desired to evaluate the specific reaction rate constant at different temperatures to determine the activation energy.

Answer to Problem 7.1P

The error in assuming the concentration of species B is constant and the limits that can be put over the calculated value of $k$ are $0.04{\left({\text{mol/dm}}^{\text{3}}\right)}^2\cdot {\min }^{-1}$ and $0$ to $X_{\text{A}}$; $0$ to $t$.

Explanation of Solution

The given liquid phase reaction which takes place in a batch reactor is as follows.

    $\text{Trityl(A)}+\text{methanol(B)}\to \text{Products(C)}$

The initial concentration of Trityl (A) in the feed is ${\text{C}}_{\text{A0}}=0.05{\text{mol/dm}}^{\text{3}}$.

The initial concentration of methanol (B) in the feed is ${\text{C}}_{\text{B0}}=0.5{\text{mol/dm}}^{\text{3}}$.

The temperature of the batch reactor is $\text{25°C}$.

The data for time and various concentration of A is given in the table below.

$t\left(\min \right)$	$0$	$50$	$100$	$150$	$200$	$250$	$300$
$C_{\text{A}}\left({\text{mol/dm}}^{\text{3}}\right)$	$0.05$	$0.038$	$0.0306$	$0.0256$	$0.0222$	$0.0195$	$0.0174$

The rate law for the above mentioned reaction is given below.

    $-r_{\text{A}}=k{C}_{\text{A}}^2{C}_{\text{B}}$        (1)

Where,

$k$ is the rate constant.

$C_{\text{A}}$ is the concentration of A.

$C_{\text{B}}$ is the concentration of B.

The value of $C_{\text{A}}=C_{\text{A0}}\left(1-X_{\text{A}}\right)$ and $C_{\text{B}}=C_{\text{A0}}\left({\Theta }_{\text{B}}-X\right)$.

Where,

$X_{\text{A}}$ is the conversion of A.

$C_{\text{A0}}$ is the initial concentration of A.

Substitute $C_{\text{A}}=C_{\text{A0}}\left(1-X_{\text{A}}\right)$ and $C_{\text{B}}=C_{\text{A0}}\left({\Theta }_{\text{B}}-X_{\text{A}}\right)$ in equation (1).

    $\begin{array}{c}-r_{\text{A}}=k\left(C_{{}_{\text{A0}}}^2{\left(1-X_{\text{A}}\right)}^2\right)\left(C_{\text{A0}}\left({\Theta }_{\text{B}}-X_{\text{A}}\right)\right)\\ =k{C}_{{}_{\text{A0}}}^3{\left(1-X_{\text{A}}\right)}^2\left({\Theta }_{\text{B}}-X_{\text{A}}\right)\end{array}$        (2)

The value of ${\Theta }_{\text{B}}=\frac{C_{\text{B0}}}{C_{\text{A0}}}$, substitute it in the above expression.

    $-r_{\text{A}}=k{C}_{{}_{\text{A0}}}^3{\left(1-X_{\text{A}}\right)}^2\left(\frac{C_{\text{B0}}}{C_{\text{A0}}}-X_{\text{A}}\right)$

Substitute ${\text{C}}_{\text{A0}}=0.05{\text{mol/dm}}^{\text{3}}$ and ${\text{C}}_{\text{B0}}=0.5{\text{mol/dm}}^{\text{3}}$ in the above expression.

    $\begin{array}{c}-r_{\text{A}}=k{C}_{{}_{\text{A0}}}^3{\left(1-X_{\text{A}}\right)}^2\left(\frac{0.5{\text{mol/dm}}^{\text{3}}}{0.05{\text{mol/dm}}^{\text{3}}}-X_{\text{A}}\right)\\ =k{C}_{{}_{\text{A0}}}^3{\left(1-X_{\text{A}}\right)}^2\left(10-X_{\text{A}}\right)\end{array}$

So, the differential rate equation for the equation is given below.

    $\begin{array}{c}-\frac{d{C}_{\text{A}}}{dt}=k{C}_{{}_{\text{A0}}}^3{\left(1-X_{\text{A}}\right)}^2\left(10-X_{\text{A}}\right)\\ C_{\text{A0}}\frac{d{X}_{\text{A}}}{dt}=k{C}_{{}_{\text{A0}}}^3{\left(1-X_{\text{A}}\right)}^2\left(10-X_{\text{A}}\right)\\ \frac{d{X}_{\text{A}}}{dt}=k{C}_{{}_{\text{A0}}}^2{\left(1-X_{\text{A}}\right)}^2\left(10-X_{\text{A}}\right)\end{array}$

The integration of the above equation with appropriate limits is given below and Substitute ${\text{C}}_{\text{A0}}=0.05{\text{mol/dm}}^{\text{3}}$ in the above equation.

    $\begin{array}{c}{\displaystyle \underset{0}{\overset{X_{\text{A}}}{\int }}\frac{d{X}_{\text{A}}}{{\left(1-X_{\text{A}}\right)}^2\left(10-X_{\text{A}}\right)}}=k{\left(0.05{\text{mol/dm}}^{\text{3}}\right)}^2{\displaystyle \underset{0}{\overset{t}{\int }}dt}\\ \frac{\ln \left(1-X_{\text{A}}\right)}{81}-\frac{\ln \left(10-X_{\text{A}}\right)}{81}+\frac{1}{9-9X_{\text{A}}}-0.08268=kt{\left(0.05{\text{mol/dm}}^{\text{3}}\right)}^2\end{array}$

If $\frac{\ln \left(1-X_{\text{A}}\right)}{81}-\frac{\ln \left(10-X_{\text{A}}\right)}{81}+\frac{1}{9-9X_{\text{A}}}-0.08268$ is assumed to be $y$ then the above equation becomes $y=k\left(\text{slope}\right)\times t$, equation of straight line.

Thus, the value of conversion, $X_{\text{A}}$ and intercept, $y$ is calculated in the table given below.

$t\left(\min \right)$	$0$	$50$	$100$	$150$	$200$	$250$	$300$
$C_{\text{A}}\left({\text{mol/dm}}^{\text{3}}\right)$	$0.05$	$0.038$	$0.0306$	$0.0256$	$0.0222$	$0.0195$	$0.0174$
$X_{\text{A}}=1-\frac{C_{\text{A}}}{C_{\text{A0}}}$	0	$0.24$	$0.388$	$0.488$	$0.556$	$0.61$	$0.652$
$\begin{array}{c}y=\frac{\ln \left(1-X_{\text{A}}\right)}{81}-\frac{\ln \left(10-X_{\text{A}}\right)}{81}\\ +\frac{1}{9-9X_{\text{A}}}-0.08268\end{array}$	$4.13\times {10}^{-6}$	$0.032004$	$0.064874$	$0.09826$	$0.12983$	$0.16295$	$0.19598$

Thus, the graph that can be plotted between $y$ and $t$ which gives the slope equals to $0.0025k$ is shown below.

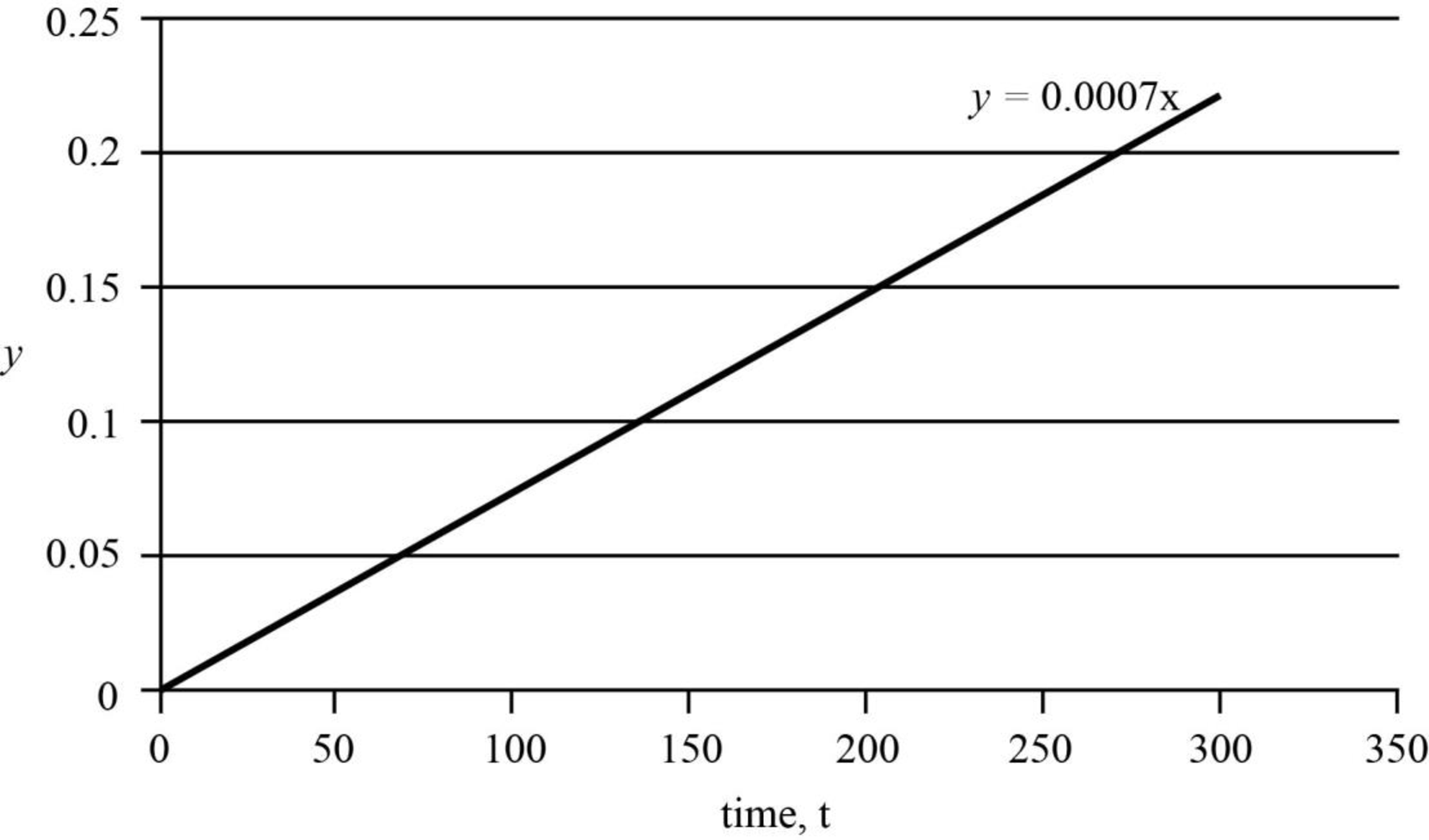
Figure 1

The intercept, $y=0.0007{\min }^{-1}$. So, the value of $k$ is calculated as follows.

    $\begin{array}{c}0.0007{\min }^{-1}=k\left(\text{slope}\right)\times {\left(0.05{\text{mol/dm}}^{\text{3}}\right)}^2\\ k\left(\text{slope}\right)=\frac{0.0007{\min }^{-1}}{{\left(0.05{\text{mol/dm}}^{\text{3}}\right)}^2}\\ =0.28{\left({\text{mol/dm}}^{\text{3}}\right)}^2\cdot {\min }^{-1}\end{array}$

The actual value of $k$ is $0.24{\left({\text{mol/dm}}^{\text{3}}\right)}^2\cdot {\min }^{-1}$. Thus, the total error in the value of $k$ is $0.28{\left({\text{mol/dm}}^{\text{3}}\right)}^2\cdot {\min }^{-1}-0.24{\left({\text{mol/dm}}^{\text{3}}\right)}^2\cdot {\min }^{-1}=0.04{\left({\text{mol/dm}}^{\text{3}}\right)}^2\cdot {\min }^{-1}$

Interpretation Introduction

Interpretation:

The reason as to why regression is carried out twice to find $k^{\text{'}}$ and $k$ is to be stated.

Concept Introduction:

In nonlinear regression analysis, we search for those parameter values that minimize the sum of the squares of the differences between the measured values and the calculated values for all the data points.

The initial estimates of the parameter values (e.g., reaction order, specific rate constant) in order to calculate the concentration for each data point, $C_{ic}$, obtained by solving an integrated form of the combined mole balance and rate law.

Explanation of Solution

After the first regression, the equation order is predicted $2.04$ and the corresponding rate constant is $2.04$. So, the order of the reaction at the end of the first regression is approximately $2$.

Thus, the value of rate constant can only be calculated at $\alpha =2$ instead of $2.04$. Therefore, the regression is carried out twice to find $k^{\text{'}}$ and $k$.

Interpretation Introduction

Interpretation:

The difference between correlation and sums of square compared with values in the given example is to be stated. The reason as to why regression is carried out twice is to be stated.

Concept Introduction:

In nonlinear regression analysis, we search for those parameter values that minimize the sum of the squares of the differences between the measured values and the calculated values for all the data points.

The initial estimates of the parameter values (e.g., reaction order, specific rate constant) in order to calculate the concentration for each data point, $C_{ic}$, obtained by solving an integrated form of the combined mole balance and rate law.

Explanation of Solution

The given rate law is as follows.

    $k_{{\text{CH}}_{\text{4}}}=k{P}_{\text{CO}}^{\text{α}}{P}_{{\text{H}}_{\text{2}}}^{\text{β}}$

During the first regression, the equation order is predicted as an integer and the corresponding rate constant is also integer. So, the regression is proceeded at the order of $0.5$ for the partial pressure of hydrogen. After taking the second regression, the second value has been attained.