Mathematics For Machine Technology
8th Edition
ISBN: 9781337798310
Author: Peterson, John.
Publisher: Cengage Learning,
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 68, Problem 27A
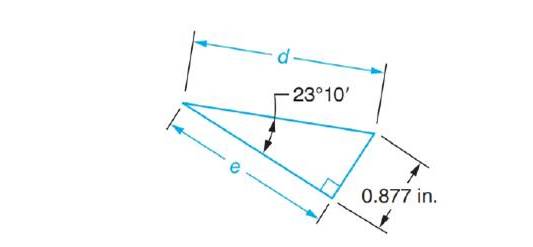
Solve the following exercises. Compute the sides to 3 decimal places in triangles dimensioned in customary units. Compute the sides to 2 decimal places in triangles dimensioned in metric units.
a. Determine side d.
b. Determine side e.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Prove for any graph G, δ(G) ≤ d(G) ≤ ∆(G) using the definition of average degree, make a formal proof
Restart
box
ixl.com/math/grade-6/area-of-compound-figures-with-triangles
ass
BModules
Dashboard | Khan...
Grades 6-8 Life S... t Typing Lessons
BDashboard f
IXL
My IXL
Learning
Assessm
Sixth grade >GG.12 Area of compound figures with triangles 5V2
What is the area of this figure?
4 km
2 km
5 km
4 km
2 km
Learn with an example
13 km
Write your answer using decimals, if necessary.
square kilometers
Submit
Area of compound figures
Area of triangles (74)
Work it out
Not feeling ready yet? Thes
Not use ai please
Chapter 68 Solutions
Mathematics For Machine Technology
Ch. 68 - If cos 3518', write the cofunction of the...Ch. 68 - If sinA=0.3617 , determine the value of angle A in...Ch. 68 - Find the volume to the nearest tenth cubic...Ch. 68 - Prob. 4ACh. 68 - Prob. 5ACh. 68 - Prob. 6ACh. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...
Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute angles to...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. Compute the sides...Ch. 68 - Solve the following exercises. For triangles...Ch. 68 - Solve the following exercises. For triangles...Ch. 68 - Solve the following exercises. For triangles...Ch. 68 - Solve the following exercises. For triangles...Ch. 68 - Solve the following exercises. For triangles...Ch. 68 - Solve the following exercises. For triangles...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- Determine the volume and the surface area of the shape obtained by rotating the area of the figure about the x-axis and the y-axis.arrow_forwardI'm getting only chatgpt answer that are wrong Plz don't use chatgpt answer will upvote .arrow_forwardFind xyz cordinates of center of gravity given z = 3.47 inarrow_forward
- pls helparrow_forwardIn Problems 1-16 the indicated function y₁(x) is a solution of the given differential equation. Use reduction of order or formula (5), as instructed, to find a second solution y2(x). 1. y" - 4y' + 4y = 0; yı = e2xarrow_forward- Problem 3: For a short time, the 300-kg roller-coaster car with passengers is traveling along the spiral track at a constant speed of v = 8 m/s with r = 15 m. If the track descends d = 6 m for every full revolution, 0 = 2π rad, determine the magnitudes of the components of force which the track exerts on the car in the r, 0, and z directions. Neglect the size of the car. Bonus: Develop a MATLAB program to solve for this problem.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning 
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Matrix Operations Full Length; Author: ProfRobBob;https://www.youtube.com/watch?v=K5BLNZw7UeU;License: Standard YouTube License, CC-BY
Intro to Matrices; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=yRwQ7A6jVLk;License: Standard YouTube License, CC-BY