Mathematics For Machine Technology
8th Edition
ISBN: 9781337798310
Author: Peterson, John.
Publisher: Cengage Learning,
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 67, Problem 6A
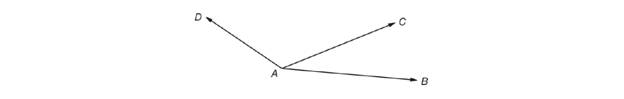
Identify each of the following angles as acute. obtuse, right, straight, or reflex. (a)
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Burger Dome sells hamburgers, cheeseburgers, french fries, soft drinks, and milk shakes, as well as a limited number of specialty items and dessert selections. Although Burger Dome would like to serve each customer immediately, at times more customers arrive than
can be handled by the Burger Dome food service staff. Thus, customers wait in line to place and receive their orders.
Burger Dome analyzed data on customer arrivals and concluded that the arrival rate is 30 customers per hour. Burger Dome also studied the order-filling process and found that a single employee can process an average of 44 customer orders per hour. Burger Dome is
concerned that the methods currently used to serve customers are resulting in excessive waiting times and a possible loss of sales. Management wants to conduct a waiting line study to help determine the best approach to reduce waiting times and improve service.
Suppose Burger Dome establishes two servers but arranges the restaurant layout so that an…
Note: A waiting line model solver computer package is needed to answer these questions.
The Kolkmeyer Manufacturing Company uses a group of six identical machines, each of which operates an average of 18 hours between breakdowns. With randomly occurring breakdowns, the Poisson probability distribution is used to describe the machine breakdown
arrival process. One person from the maintenance department provides the single-server repair service for the six machines.
Management is now considering adding two machines to its manufacturing operation. This addition will bring the number of machines to eight. The president of Kolkmeyer asked for a study of the need to add a second employee to the repair operation. The service rate
for each individual assigned to the repair operation is 0.50 machines per hour.
(a) Compute the operating characteristics if the company retains the single-employee repair operation. (Round your answers to four decimal places. Report time in hours.)
La
=
L =
Wa
=
W =…
Use the Euclidean algorithm to find two sets of integers (a, b, c) such
that
55a65b+143c:
Solution
= 1.
By the Euclidean algorithm, we have:
143 = 2.65 + 13 and 65 = 5.13, so 13 = 143 – 2.65.
-
Also, 55 = 4.13+3, 13 = 4.3 + 1 and 3 = 3.1,
so 1 = 13 — 4.3 = 13 — 4(55 – 4.13) = 17.13 – 4.55.
Combining these, we have:
1 = 17(143 – 2.65) - 4.55 = −4.55 - 34.65 + 17.143,
so we can take a = − −4, b = −34, c = 17. By carrying out the division
algorithm in other ways, we obtain different solutions, such as
19.55 23.65 +7.143, so a = = 9, b -23, c = 7.
=
=
how
?
come
[Note that 13.55 + 11.65 - 10.143 0, so we can obtain new solutions by
adding multiples of this equation, or similar equations.]
Chapter 67 Solutions
Mathematics For Machine Technology
Ch. 67 - If tan A=4.13792 , determine the value of angle A...Ch. 67 - Find the number of cubic inches of material...Ch. 67 - Find the number of cubic inches of material...Ch. 67 - The sector of a circle has an area of 231.3 sq in....Ch. 67 - Determine the arc length ABC if r=5.75in. and...Ch. 67 - Identify each of the following angles as acute....Ch. 67 - Refer to the following figure in answering...Ch. 67 - Refer to the following figure in answering...Ch. 67 - Refer to the following figure in answering...Ch. 67 - Refer to the following figure in answering...
Ch. 67 - Refer to the following figure in answering...Ch. 67 - Prob. 12ACh. 67 - Refer to the following figure in answering...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - For each exercise, functions of two angles are...Ch. 67 - Prob. 25ACh. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - Prob. 41ACh. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - For each function of an angle, write the...Ch. 67 - Prob. 45ACh. 67 - For each exercise, functions and cofunctions of...Ch. 67 - Prob. 47ACh. 67 - For each exercise, functions and cofunctions of...Ch. 67 - Prob. 49ACh. 67 - For each exercise, functions and cofunctions of...Ch. 67 - Prob. 51ACh. 67 - For each exercise, functions and cofunctions of...Ch. 67 - Prob. 53ACh. 67 - For each exercise, functions and cofunctions of...Ch. 67 - Prob. 55ACh. 67 - For each exercise, functions and cofunctions of...Ch. 67 - Prob. 57A
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- - Let n = 7, let p = 23 and let S be the set of least positive residues mod p of the first (p − 1)/2 multiple of n, i.e. n mod p, 2n mod p, ..., p-1 2 -n mod p. Let T be the subset of S consisting of those residues which exceed p/2. Find the set T, and hence compute the Legendre symbol (7|23). 23 32 how come? The first 11 multiples of 7 reduced mod 23 are 7, 14, 21, 5, 12, 19, 3, 10, 17, 1, 8. The set T is the subset of these residues exceeding So T = {12, 14, 17, 19, 21}. By Gauss' lemma (Apostol Theorem 9.6), (7|23) = (−1)|T| = (−1)5 = −1.arrow_forwardLet n = 7, let p = 23 and let S be the set of least positive residues mod p of the first (p-1)/2 multiple of n, i.e. n mod p, 2n mod p, ..., 2 p-1 -n mod p. Let T be the subset of S consisting of those residues which exceed p/2. Find the set T, and hence compute the Legendre symbol (7|23). The first 11 multiples of 7 reduced mod 23 are 7, 14, 21, 5, 12, 19, 3, 10, 17, 1, 8. 23 The set T is the subset of these residues exceeding 2° So T = {12, 14, 17, 19, 21}. By Gauss' lemma (Apostol Theorem 9.6), (7|23) = (−1)|T| = (−1)5 = −1. how come?arrow_forwardShading a Venn diagram with 3 sets: Unions, intersections, and... The Venn diagram shows sets A, B, C, and the universal set U. Shade (CUA)' n B on the Venn diagram. U Explanation Check A- B Q Search 田arrow_forward
- Find all solutions of the polynomial congruence x²+4x+1 = 0 (mod 143). (The solutions of the congruence x² + 4x+1=0 (mod 11) are x = 3,4 (mod 11) and the solutions of the congruence x² +4x+1 = 0 (mod 13) are x = 2,7 (mod 13).)arrow_forwardDetermine whether each function is an injection and determine whether each is a surjection.The notation Z_(n) refers to the set {0,1,2,...,n-1}. For example, Z_(4)={0,1,2,3}. f: Z_(6) -> Z_(6) defined by f(x)=x^(2)+4(mod6). g: Z_(5) -> Z_(5) defined by g(x)=x^(2)-11(mod5). h: Z*Z -> Z defined by h(x,y)=x+2y. j: R-{3} -> R defined by j(x)=(4x)/(x-3).arrow_forwardDetermine whether each function is an injection and determine whether each is a surjection.arrow_forward
- Let A = {a, b, c, d}, B = {a,b,c}, and C = {s, t, u,v}. Draw an arrow diagram of a function for each of the following descriptions. If no such function exists, briefly explain why. (a) A function f : AC whose range is the set C. (b) A function g: BC whose range is the set C. (c) A function g: BC that is injective. (d) A function j : A → C that is not bijective.arrow_forwardLet f:R->R be defined by f(x)=x^(3)+5.(a) Determine if f is injective. why?(b) Determine if f is surjective. why?(c) Based upon (a) and (b), is f bijective? why?arrow_forwardLet f:R->R be defined by f(x)=x^(3)+5.(a) Determine if f is injective.(b) Determine if f is surjective. (c) Based upon (a) and (b), is f bijective?arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:CengageMathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781305652224
Author:Charles P. McKeague, Mark D. Turner
Publisher:Cengage Learning
01 - Angles and Angle Measure in Degrees - Part 1 - Types of Angles & What is an Angle?; Author: Math and Science;https://www.youtube.com/watch?v=hy95VyPet-M;License: Standard YouTube License, CC-BY