Chapter 6.6, Problem 122E

a.

To determine

To calculate:

The roots of complex number.

Answer to Problem 122E

The roots of the complex numbers are,

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi }{20}+i\sin \frac{7\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{3\pi }{4}+i\sin \frac{3\pi }{4}\right)\\ \sqrt{2}\left(\cos \frac{23\pi }{20}+i\sin \frac{23\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{31\pi }{20}+i\sin \frac{31\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{39\pi }{20}+i\sin \frac{39\pi }{20}\right)\end{array}$

Explanation of Solution

Given information:

Fifth roots of $4\left(1-i\right)$ .

Formula used:

  $\sqrt[n]{r}\left(\cos \frac{\theta +2\pi k}{n}+i\sin \frac{\theta +2\pi k}{n}\right)$

Where,

  $n=$ positive integer,

complex number $z=r\left(\cos \pi +i\sin \theta \right)$ has exactly $n$ distinct $n^{th}$ roots, $k=0,1,\mathrm{2.....},n-1$

Calculation:

Consider the $4\left(1-i\right)$

The complex number $\left(1-i\right)$ lies in the fourth quadrant,

Its modulus is given by

  $\begin{array}{l}r=\sqrt{1^2+{\left(-1\right)}^2}\\ r=\sqrt{2}\end{array}$

Its argument is

  $\begin{array}{l}\theta =\arctan \frac{-1}{1}\\ \theta =\arctan -\frac{\pi }{4}\end{array}$

As the complex number lies in the fourth quadrant, therefore

  $\begin{array}{l}\theta =2\pi -\frac{\pi }{4}\\ \theta =\frac{7\pi }{4}\end{array}$

Hence, its trigonometric form comes out to be

  $z=\sqrt{2}\left(\cos \frac{7\pi }{4}+i\sin \frac{7\pi }{4}\right)$

Therefore, the given complex number is

  $\begin{array}{l}z=4\left[\sqrt{2}\left(\cos \frac{7\pi }{4}+i\sin \frac{7\pi }{4}\right)\right]\\ z=4\sqrt{2}\left(\cos \frac{7\pi }{4}+i\sin \frac{7\pi }{4}\right)\end{array}$

By using the formula for $n^{th}$ roots of a complex number that states,

For a positive integer $n$ , the complex number $z=r\left(\cos \pi +i\sin \theta \right)$ has exactly $n$ distinct $n^{th}$ roots as, $k=0,1,\mathrm{2.....},n-1$

  $\sqrt[n]{r}\left(\cos \frac{\theta +2\pi k}{n}+i\sin \frac{\theta +2\pi k}{n}\right)$

So, by using the formula the result is as follows:

  $\begin{array}{l}z=4\sqrt{2}\left(\cos \frac{7\pi }{4}+i\sin \frac{7\pi }{4}\right)\\ =\sqrt[5]{4\sqrt{2}}\left(\cos \frac{\frac{7\pi }{4}+2\pi k}{5}+i\sin \frac{\frac{7\pi }{4}+2\pi k}{5}\right)\\ =\sqrt[5]{{\left(\sqrt{2}\right)}^5}\left(\cos \frac{7\pi +8\pi k}{20}+i\sin \frac{7\pi +8\pi k}{20}\right)\\ =\sqrt{2}\left(\cos \frac{7\pi +8\pi k}{20}+i\sin \frac{7\pi +8\pi k}{20}\right)\end{array}$

So, for $k=0,1,2,3,4$ the square roots are as follows:

For $0:$

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi +8\pi k}{20}+i\sin \frac{7\pi +8\pi k}{20}\right)\\ =\sqrt{2}\left(\cos \frac{7\pi }{20}+i\sin \frac{7\pi }{20}\right)\end{array}$

For $1:$

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi +8\pi \times 1}{20}+i\sin \frac{7\pi +8\pi \times 1}{20}\right)\\ =\sqrt{2}\left(\cos \frac{15\pi }{20}+i\sin \frac{15\pi }{20}\right)\\ =\sqrt{2}\left(\cos \frac{3\pi }{4}+i\sin \frac{3\pi }{4}\right)\end{array}$

For $2:$

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi +8\pi \times 2}{20}+i\sin \frac{7\pi +8\pi \times 2}{20}\right)\\ =\sqrt{2}\left(\cos \frac{7\pi +16\pi }{20}+i\sin \frac{7\pi +16\pi }{20}\right)\\ =\sqrt{2}\left(\cos \frac{23\pi }{20}+i\sin \frac{23\pi }{20}\right)\end{array}$

For $3:$

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi +8\pi \times 3}{20}+i\sin \frac{7\pi +8\pi \times 3}{20}\right)\\ =\sqrt{2}\left(\cos \frac{7\pi +24\pi }{20}+i\sin \frac{7\pi +24\pi }{20}\right)\\ =\sqrt{2}\left(\cos \frac{31\pi }{20}+i\sin \frac{31\pi }{20}\right)\end{array}$

For $4:$

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi +8\pi \times 4}{20}+i\sin \frac{7\pi +8\pi \times 4}{20}\right)\\ =\sqrt{2}\left(\cos \frac{7\pi +32\pi }{20}+i\sin \frac{7\pi +32\pi }{20}\right)\\ =\sqrt{2}\left(\cos \frac{39\pi }{20}+i\sin \frac{39\pi }{20}\right)\end{array}$

b.

To determine

To calculate:

Given roots in standard form.

Answer to Problem 122E

The complex roots are in the standard form as,

  $\begin{array}{l}0.6420+1.260i\\ -1+i\\ -1.2601+0.6420i\\ 0.2212-1.3968i\\ 1.3968-0.2212i\end{array}$

Explanation of Solution

Given information:

Fifth roots of $4\left(1-i\right)$ .

The roots are,

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi }{20}+i\sin \frac{7\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{3\pi }{4}+i\sin \frac{3\pi }{4}\right)\\ \sqrt{2}\left(\cos \frac{23\pi }{20}+i\sin \frac{23\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{31\pi }{20}+i\sin \frac{31\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{39\pi }{20}+i\sin \frac{39\pi }{20}\right)\end{array}$

Calculation:

Since,

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi }{20}+i\sin \frac{7\pi }{20}\right)\\ =\sqrt{2}\left(\cos 63+i\sin 63\right)\\ =0.6420+1.260i\end{array}$

For,

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{3\pi }{4}+i\sin \frac{3\pi }{4}\right)\\ =\sqrt{2}\left(\cos \left(\pi -\frac{\pi }{4}\right)+i\sin \left(\pi -\frac{\pi }{4}\right)\right)\end{array}$

As know that sine is positive and cosine is negative in the second quadrant, so

  $\begin{array}{l}=\sqrt{2}\left(-\cos \frac{\pi }{4}+i\sin \frac{\pi }{4}\right)\\ =\sqrt{2}\left(-\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}\right)\\ =-1+i\end{array}$

For,

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{23\pi }{20}+i\sin \frac{23\pi }{20}\right)\\ =\sqrt{2}\left(\cos \left(\pi +\frac{3\pi }{20}\right)+i\sin \left(\pi +\frac{3\pi }{20}\right)\right)\end{array}$

As know that sine and cosine are negative in the third quadrant, so

  $\begin{array}{l}=\sqrt{2}\left(\cos \frac{3\pi }{4}+i\sin \frac{3\pi }{4}\right)\\ =\sqrt{2}\left(-\cos 27-i\sin 27\right)\\ =-1.2601+0.6420i\end{array}$

For,

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{31\pi }{20}+i\sin \frac{31\pi }{20}\right)\\ =\sqrt{2}\left(\cos \left(2\pi -\frac{9\pi }{20}\right)+i\sin \left(2\pi -\frac{9\pi }{20}\right)\right)\end{array}$

As know that cosine is positive and sine is negative in the fourth quadrant, so

  $\begin{array}{l}=\sqrt{2}\left(\cos \frac{9\pi }{20}+i\sin \frac{9\pi }{20}\right)\\ =0.2212-1.3968i\end{array}$

For,

  $\begin{array}{l}=\sqrt{2}\left(\cos \frac{39\pi }{20}+i\sin \frac{39\pi }{20}\right)\\ =\sqrt{2}\left(\cos \left(2\pi -\frac{\pi }{20}\right)+i\sin \left(2\pi -\frac{\pi }{20}\right)\right)\end{array}$

As know that cosine is positive and sine is negative in the fourth quadrant, so

  $\begin{array}{l}=\sqrt{2}\left(\cos \frac{\pi }{20}+i\sin \frac{\pi }{20}\right)\\ =1.3968-0.2212i\end{array}$

c.

To determine

To graph:

The given roots graphically.

Explanation of Solution

Given information:

The roots are,

  $\begin{array}{l}\sqrt{2}\left(\cos \frac{7\pi }{20}+i\sin \frac{7\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{3\pi }{4}+i\sin \frac{3\pi }{4}\right)\\ \sqrt{2}\left(\cos \frac{23\pi }{20}+i\sin \frac{23\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{31\pi }{20}+i\sin \frac{31\pi }{20}\right)\\ \sqrt{2}\left(\cos \frac{39\pi }{20}+i\sin \frac{39\pi }{20}\right)\end{array}$

Graph:

  

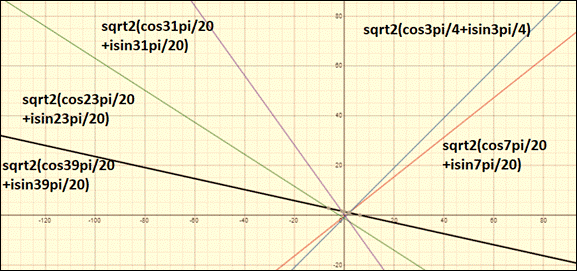
Interpretation:

In above graph, complex roots are described.