CALCULUS,EARLY TRANSCENTALS
9th Edition
ISBN: 9780357114049
Author: Stewart
Publisher: CENGAGE L
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 6.4, Problem 25E
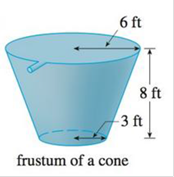
A tank is full of water. Find the work required to pump the water out of the spout. In Exercises 25 and 26 use the fact that water weighs 62.5 lb/ ft3.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
The cup on the 9th hole of a golf course is located dead center in the middle of a circular green which is 40 feet in radius. Your ball is located as in the picture below. The ball follows a straight line path and exits the green at the right-most edge. Assume the ball travels 8 ft/sec.
Introduce coordinates so that the cup is the origin of an xy-coordinate system and start by writing down the equations of the circle and the linear path of the ball. Provide numerical answers below with two decimal places of accuracy.
50 feet
green
ball
40 feet
9
cup
ball path
rough
(a) The x-coordinate of the position where the ball enters the green will be
(b) The ball will exit the green exactly
seconds after it is hit.
(c) Suppose that L is a line tangent to the boundary of the golf green and parallel to the path of the ball. Let Q be the point where the line is tangent to the circle. Notice that there are two possible positions for Q. Find the possible x-coordinates of Q:
smallest x-coordinate =…
Draw the unit circle and plot the point P=(8,2). Observe there are TWO lines tangent to the circle passing through the point P. Answer the questions below with 3 decimal places of accuracy.
P
L1
L
(a) The line L₁ is tangent to the unit circle at the point
(b) The tangent line L₁ has equation:
X +
(c) The line L₂ is tangent to the unit circle at the point (
(d) The tangent line 42 has equation:
y=
x +
).
What is a solution to a differential equation? We said that a differential equation is an equation that
describes the derivative, or derivatives, of a function that is unknown to us. By a solution to a differential
equation, we mean simply a function that satisfies this description.
2. Here is a differential equation which describes an unknown position function s(t):
ds
dt
318
4t+1,
ds
(a) To check that s(t) = 2t2 + t is a solution to this differential equation, calculate
you really do get 4t +1.
and check that
dt'
(b) Is s(t) = 2t2 +++ 4 also a solution to this differential equation?
(c) Is s(t)=2t2 + 3t also a solution to this differential equation?
ds
1
dt
(d) To find all possible solutions, start with the differential equation = 4t + 1, then move dt to the
right side of the equation by multiplying, and then integrate both sides. What do you get?
(e) Does this differential equation have a unique solution, or an infinite family of solutions?
Chapter 6 Solutions
CALCULUS,EARLY TRANSCENTALS
Ch. 6.1 - (a) Set up an integral for the area of the shaded...Ch. 6.1 - (a) Set up an integral for the area of the shaded...Ch. 6.1 - Find the area of the shaded region.Ch. 6.1 - Find the area of the shaded region.Ch. 6.1 - Find the area of the shaded region. 5.Ch. 6.1 - Find the area of the shaded region. 6.Ch. 6.1 - Set up, but do not evaluate, an integral...Ch. 6.1 - Prob. 8ECh. 6.1 - Set up, but do not evaluate, an integral...Ch. 6.1 - Set up, but do not evaluate, an integral...
Ch. 6.1 - Sketch the region enclosed by the given curves....Ch. 6.1 - Prob. 12ECh. 6.1 - Sketch the region enclosed by the given curves....Ch. 6.1 - Sketch the region enclosed by the given curves....Ch. 6.1 - Sketch the region enclosed by the given curves....Ch. 6.1 - Sketch the region enclosed by the given curves....Ch. 6.1 - Sketch the region enclosed by the given curves....Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Prob. 32ECh. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Prob. 37ECh. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Sketch the region enclosed by the given curves and...Ch. 6.1 - Use calculus to find the area of the triangle with...Ch. 6.1 - Use calculus to find the area of the triangle with...Ch. 6.1 - Evaluate the integral and interpret it as the area...Ch. 6.1 - Evaluate the integral and interpret it as the area...Ch. 6.1 - Use a graph to find approximate x-coordinates of...Ch. 6.1 - Prob. 46ECh. 6.1 - Prob. 47ECh. 6.1 - Prob. 48ECh. 6.1 - Graph the region between the curves and use your...Ch. 6.1 - Prob. 50ECh. 6.1 - Prob. 51ECh. 6.1 - Prob. 52ECh. 6.1 - Sketch the region in the xy-plane defined by the...Ch. 6.1 - Racing cars driven by Chris and Kelly are side by...Ch. 6.1 - The widths (in meters) of a kidney-shaped swimming...Ch. 6.1 - A cross-section of an airplane wing is shown....Ch. 6.1 - If the birth rate of a population is b(t) =...Ch. 6.1 - Prob. 60ECh. 6.1 - Two cars, A and B, start side by side and...Ch. 6.1 - The figure shows graphs of the marginal revenue...Ch. 6.1 - The curve with equation y2 = x2(x + 3) is called...Ch. 6.1 - Find the area of the region bounded by the...Ch. 6.1 - Find the number b such that the line y = b divides...Ch. 6.1 - (a) Find the number a such that the line x = a...Ch. 6.1 - Find the values of c such that the area of the...Ch. 6.1 - Suppose that 0 c /2. For what value of c is the...Ch. 6.1 - For what values of m do the line y = mx and the...Ch. 6.2 - Prob. 7ECh. 6.2 - Set up, but do not evaluate, an integral for the...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Find the volume of the solid obtained by rotating...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Refer to the figure and find the volume generated...Ch. 6.2 - Set up an integral for the volume of the solid...Ch. 6.2 - Set up an integral for the volume of the solid...Ch. 6.2 - Set up an integral for the volume of the solid...Ch. 6.2 - Set up an integral for the volume of the solid...Ch. 6.2 - Use a graph to find approximate x-coordinates of...Ch. 6.2 - Use a graph to find approximate x-coordinates of...Ch. 6.2 - Prob. 49ECh. 6.2 - Prob. 50ECh. 6.2 - Prob. 51ECh. 6.2 - Each integral represents the volume of a solid....Ch. 6.2 - Prob. 53ECh. 6.2 - Each integral represents the volume of a solid....Ch. 6.2 - A log 10 m long is cut at 1-meter intervals and...Ch. 6.2 - (a) If the region shown in the figure is rotated...Ch. 6.2 - Find the volume of the described solid S. A right...Ch. 6.2 - Find the volume of the described solid S. A...Ch. 6.2 - Find the volume of the described solid S. A cap of...Ch. 6.2 - Find the volume of the described solid S. A...Ch. 6.2 - Find the volume of the described solid S. A...Ch. 6.2 - Find the volume of the described solid S. A...Ch. 6.2 - Find the volume of the described solid S. A...Ch. 6.2 - Find the volume of the described solid S. The base...Ch. 6.2 - Find the volume of the described solid S. The base...Ch. 6.2 - Find the volume of the described solid S. The base...Ch. 6.2 - Find the volume of the described solid S. The base...Ch. 6.2 - Find the volume of the described solid S. The base...Ch. 6.2 - Find the volume of the described solid S. The base...Ch. 6.2 - Find the volume of the described solid S. The base...Ch. 6.2 - Find the volume of the described solid S. The...Ch. 6.2 - (a) Set up an integral for the volume of a solid...Ch. 6.2 - Prob. 77ECh. 6.2 - Find the volume common to two circular cylinders,...Ch. 6.2 - Prob. 81ECh. 6.2 - A bowl is shaped like a hemisphere with diameter...Ch. 6.2 - A hole of radius r is bored through the middle of...Ch. 6.2 - A hole of radius r is bored through the center of...Ch. 6.2 - Some of the pioneers of calculus, such as Kepler...Ch. 6.2 - Suppose that a region has area A and lies above...Ch. 6.3 - Let S be the solid obtained by rotating the region...Ch. 6.3 - Let S be the solid obtained by rotating the region...Ch. 6.3 - Set up, but do not evaluate, an integral for the...Ch. 6.3 - Prob. 6ECh. 6.3 - Prob. 7ECh. 6.3 - Prob. 9ECh. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - Use the method of cylindrical shells to find the...Ch. 6.3 - (a) Set up an integral for the volume of the solid...Ch. 6.3 - (a) Set up an integral for the volume of the solid...Ch. 6.3 - (a) Set up an integral for the volume of the solid...Ch. 6.3 - (a) Set up an integral for the volume of the solid...Ch. 6.3 - (a) Set up an integral for the volume of the solid...Ch. 6.3 - (a) Set up an integral for the volume of the solid...Ch. 6.3 - Use the Midpoint Rule with n = 5 to estimate the...Ch. 6.3 - If the region shown in the figure is rotated about...Ch. 6.3 - Prob. 39ECh. 6.3 - Prob. 40ECh. 6.3 - Prob. 41ECh. 6.3 - Prob. 42ECh. 6.3 - Use a graph to estimate the x-coordinates of the...Ch. 6.3 - Prob. 44ECh. 6.3 - The region bounded by the given curves is rotated...Ch. 6.3 - The region bounded by the given curves is rotated...Ch. 6.3 - The region bounded by the given curves is rotated...Ch. 6.3 - The region bounded by the given curves is rotated...Ch. 6.3 - The region bounded by the given curves is rotated...Ch. 6.3 - The region bounded by the given curves is rotated...Ch. 6.3 - The region bounded by the given curves is rotated...Ch. 6.3 - Let T be the triangular region with vertices (0,...Ch. 6.3 - Prob. 61ECh. 6.3 - Use cylindrical shells to find the volume of the...Ch. 6.3 - Use cylindrical shells to find the volume of the...Ch. 6.4 - How much work is done when a weight lifter lifts...Ch. 6.4 - Compute the work done in hoisting an 1100-lb grand...Ch. 6.4 - Prob. 3ECh. 6.4 - A variable force of 4x newtons moves a particle...Ch. 6.4 - Shown is the graph of a force function (in...Ch. 6.4 - Prob. 6ECh. 6.4 - A force of 10 lb is required to hold a spring...Ch. 6.4 - A spring has a natural length of 40 cm. If a 60-N...Ch. 6.4 - Suppose that 2 J of work is needed to stretch a...Ch. 6.4 - If the work required to stretch a spring 1 ft...Ch. 6.4 - A spring has natural length 20 cm. Compare the...Ch. 6.4 - If 6 J of work is needed to stretch a spring from...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - A 0.4-kg model rocket is loaded with 0.75kg of...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - Show how to approximate the required work by a...Ch. 6.4 - A tank is full of water. Find the work required to...Ch. 6.4 - A tank is full of water. Find the work required to...Ch. 6.4 - A tank is full of water. Find the work required to...Ch. 6.4 - A tank is full of water. Find the work required to...Ch. 6.4 - Suppose that for the tank in Exercise 23 the pump...Ch. 6.4 - Solve Exercise 24 if the tank is half full of oil...Ch. 6.4 - When gas expands in a cylinder with radius r, the...Ch. 6.4 - In a steam engine the pressure P and volume V of...Ch. 6.4 - Prob. 31ECh. 6.4 - Prob. 32ECh. 6.4 - Work-Energy Theorem The kinetic energy KE of an...Ch. 6.4 - The Great Pyramid of King Khufu was built of...Ch. 6.5 - Find the average value of the function on the...Ch. 6.5 - Find the average value of the function on the...Ch. 6.5 - Find the average value of the function on the...Ch. 6.5 - Find the average value of the function on the...Ch. 6.5 - Prob. 5ECh. 6.5 - Find the average value of the function on the...Ch. 6.5 - Find the average value of the function on the...Ch. 6.5 - Find the average value of the function on the...Ch. 6.5 - If f is continuous and 13f(x)dx=8, show that f...Ch. 6.5 - Find the numbers b such that the average value of...Ch. 6.5 - Find the average value of f on [0, 8].Ch. 6.5 - The velocity graph of an accelerating car is...Ch. 6.5 - In a certain city the temperature (in F) t hours...Ch. 6.5 - The linear density in a rod 8 m long is...Ch. 6.5 - The velocity v of blood that flows in a blood...Ch. 6.5 - In Example 3.8.1 we modeled the world population...Ch. 6.5 - Prob. 23ECh. 6.5 - Use the diagram to show that if f is concave...Ch. 6.5 - Prob. 25ECh. 6.5 - Prob. 26ECh. 6.5 - Prob. 27ECh. 6.5 - Prob. 28ECh. 6 - (a) Draw two typical curves y = f(x) and y = g(x),...Ch. 6 - Suppose that Sue runs faster than Kathy throughout...Ch. 6 - Prob. 3CCCh. 6 - Prob. 4CCCh. 6 - Suppose that you push a book across a 6-meter-long...Ch. 6 - Prob. 6CCCh. 6 - Determine whether the statement is true or false....Ch. 6 - Prob. 2TFQCh. 6 - Prob. 3TFQCh. 6 - Prob. 4TFQCh. 6 - Prob. 5TFQCh. 6 - Prob. 6TFQCh. 6 - Prob. 7TFQCh. 6 - Prob. 8TFQCh. 6 - Prob. 9TFQCh. 6 - A cable hangs vertically from a winch located at...Ch. 6 - Find the area of the region bounded by the given...Ch. 6 - Find the area of the region bounded by the given...Ch. 6 - Prob. 3ECh. 6 - Find the area of the region bounded by the given...Ch. 6 - Find the area of the region bounded by the given...Ch. 6 - Find the area of the region bounded by the given...Ch. 6 - Prob. 7ECh. 6 - Find the volume of the solid obtained by rotating...Ch. 6 - Find the volume of the solid obtained by rotating...Ch. 6 - Find the volume of the solid obtained by rotating...Ch. 6 - Prob. 11ECh. 6 - Set up, but do not evaluate, an integral for the...Ch. 6 - Prob. 13ECh. 6 - Set up, but do not evaluate, an integral for the...Ch. 6 - Find the volumes of the solids obtained by...Ch. 6 - Let be the region in the first quadrant bounded...Ch. 6 - Prob. 19ECh. 6 - Let be the region bounded by the curves y = 1 x2...Ch. 6 - Prob. 21ECh. 6 - Each integral represents the volume of a solid....Ch. 6 - Each integral represents the volume of a solid....Ch. 6 - Each integral represents the volume of a solid....Ch. 6 - The base of a solid is a circular disk with radius...Ch. 6 - The base of a solid is the region bounded by the...Ch. 6 - Prob. 27ECh. 6 - Prob. 28ECh. 6 - Prob. 29ECh. 6 - A 1600-lb elevator is suspended by a 200-ft cable...Ch. 6 - A tank full of water has the shape of a paraboloid...Ch. 6 - A steel tank has the shape of a circular cylinder...Ch. 6 - Prob. 33ECh. 6 - Prob. 34ECh. 6 - Prob. 35ECh. 6 - There is a line through the origin that divides...Ch. 6 - The figure shows a horizontal line y = c...Ch. 6 - A cylindrical glass of radius r and height L is...Ch. 6 - Archimedes Principle states that the buoyant force...Ch. 6 - Prob. 7PPCh. 6 - A paper drinking cup filled with water has the...Ch. 6 - A clepsydra, or water clock, is a glass container...Ch. 6 - A cylindrical container of radius r and height L...Ch. 6 - Prob. 11PPCh. 6 - If the tangent at a point P on the curve y = x3...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Ministry of Higher Education & Scientific Research Babylon University College of Engineering - Al musayab Automobile Department Subject :Engineering Analysis Time: 2 hour Date:27-11-2022 کورس اول تحليلات تعمیر ) 1st month exam / 1st semester (2022-2023)/11/27 Note: Answer all questions,all questions have same degree. Q1/: Find the following for three only. 1- 4s C-1 (+2-3)2 (219) 3.0 (6+1)) (+3+5) (82+28-3),2- ,3- 2-1 4- Q2/:Determine the Laplace transform of the function t sint. Q3/: Find the Laplace transform of 1, 0≤t<2, -2t+1, 2≤t<3, f(t) = 3t, t-1, 3≤t 5, t≥ 5 Q4: Find the Fourier series corresponding to the function 0 -5arrow_forwardMinistry of Higher Education & Scientific Research Babylon University College of Engineering - Al musayab Subject :Engineering Analysis Time: 80 min Date:11-12-2022 Automobile Department 2nd month exam / 1" semester (2022-2023) Note: Answer all questions,all questions have same degree. کورس اول شعر 3 Q1/: Use a Power series to solve the differential equation: y" - xy = 0 Q2/:Evaluate using Cauchy's residue theorem, sinnz²+cosz² dz, where C is z = 3 (z-1)(z-2) Q3/:Evaluate dz (z²+4)2 Where C is the circle /z-i/-2,using Cauchy's residue theorem. Examiner: Dr. Wisam N. Hassanarrow_forwardMinistry of Higher Education & Scientific Research Babylon University College of Engineering - Al musayab Subject :Engineering Analysis Time: 80 min Date:11-12-2022 Automobile Department 2nd month exam / 1" semester (2022-2023) Note: Answer all questions,all questions have same degree. کورس اول شعر 3 Q1/: Use a Power series to solve the differential equation: y" - xy = 0 Q2/:Evaluate using Cauchy's residue theorem, sinnz²+cosz² dz, where C is z = 3 (z-1)(z-2) Q3/:Evaluate dz (z²+4)2 Where C is the circle /z-i/-2,using Cauchy's residue theorem. Examiner: Dr. Wisam N. Hassanarrow_forwardWhich degenerate conic is formed when a double cone is sliced through the apex by a plane parallel to the slant edge of the cone?arrow_forward1/ Solve the following: 1 x + X + cos(3X) -75 -1 2 2 (5+1) e 5² + 5 + 1 3 L -1 1 5² (5²+1) 1 5(5-5)arrow_forwardI need expert handwritten solution.to this integralarrow_forwardExample: If ƒ (x + 2π) = ƒ (x), find the Fourier expansion f(x) = eax in the interval [−π,π]arrow_forwardExample: If ƒ (x + 2π) = ƒ (x), find the Fourier expansion f(x) = eax in the interval [−π,π]arrow_forwardPlease can you give detailed steps on how the solutions change from complex form to real form. Thanks.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
Recommended textbooks for you
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Chain Rule dy:dx = dy:du*du:dx; Author: Robert Cappetta;https://www.youtube.com/watch?v=IUYniALwbHs;License: Standard YouTube License, CC-BY
CHAIN RULE Part 1; Author: Btech Maths Hub;https://www.youtube.com/watch?v=TIAw6AJ_5Po;License: Standard YouTube License, CC-BY