THINK.MATH.LOOSELEAF W/18 WEEK MATHLAB
THINK.MATH.LOOSELEAF W/18 WEEK MATHLAB
7th Edition
ISBN: 9780136209171
Author: Blitzer
Publisher: PEARSON
bartleby

Videos

Textbook Question
Book Icon
Chapter 6.2, Problem 131E

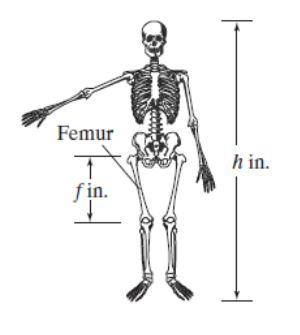
A woman’s height, h, is related to the length of the femur, f (the bone from the knee to the hip socket), by the formula f = 0.432 h − 10.44 . Both h and f are measured in inches. A partial skeleton is found of a woman in which the femur is 16 inches long. Police find the skeleton in an area where a woman slightly over 5 feet tall has been missing for over a year. Can the partial skeleton be that of the missing woman? Explain.

Chapter 6.2, Problem 131E, 131. A woman’s height, h, is related to the length of the femur, f (the bone from the knee to the

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 6.2, Problem 130E
Next
Chapter 6.3, Problem 1CP
Students have asked these similar questions
3. Construct a triangle in the Poincare plane with all sides equal to ln(2).   (Hint: Use the fact that, the circle with center (0,a) and radius ln(r), r>1 in    the Poincaré plane is equal to the point set       { (x,y) : x^2+(y-1/2(r+1/r)a)^2=1/4(r-1/r)^2a^2 }
n. g. = neutral geometry  <ABC  = angle ABC \leq  = less or equal than sqrt{x} = square root of x cLr = the line in the Poincaré plane defined by the equation (x-c)^2+y^2=r^2 1. Find the bisector of the angle <ABC in the Poincaré plane, where    A=(0,5), B=(0,3) and C=(2,\sqrt{21})
Task: 2 Multivariable Calculus: Divergence Theorem Refer to Question 42 in the provided document. Link: https://drive.google.com/file/d/1wKSrun-GlxirS31Z9qoHazb9tC440 AZF/view?usp=sharing

Chapter 6 Solutions

THINK.MATH.LOOSELEAF W/18 WEEK MATHLAB

Ch. 6.1 - CHECK POINT 1 Evaluate . Ch. 6.1 - CHECK POINT 2 Evaluate x 2 + 4 x − 7   for   x = −...Ch. 6.1 - CHECK POINT 3 Evaluate − 3 x 2 + 4 x y − y 3   for...Ch. 6.1 - CHECK POINT 4 The mathematic model M = − 120 x 2 +...Ch. 6.1 - Prob. 5CPCh. 6.1 - CHECK POINT 6 Simplify: 7 ( 4 x 2 + 3 x ) + 2 ( 5...Ch. 6.1 - Prob. 7CPCh. 6.1 - Fill in each blank so that the resulting statement...Ch. 6.1 - Fill in each blank so that the resulting statement...Ch. 6.1 - Fill in each blank so that the resulting statement...
Ch. 6.1 - Prob. 4CVCCh. 6.1 - Prob. 5CVCCh. 6.1 - Prob. 6CVCCh. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - Prob. 3ECh. 6.1 - Prob. 4ECh. 6.1 - Prob. 5ECh. 6.1 - Prob. 6ECh. 6.1 - Prob. 7ECh. 6.1 - Prob. 8ECh. 6.1 - Prob. 9ECh. 6.1 - Prob. 10ECh. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - Prob. 12ECh. 6.1 - Prob. 13ECh. 6.1 - Prob. 14ECh. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - Prob. 16ECh. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - In Exercises 1-34, evaluate the algebraic...Ch. 6.1 - Prob. 24ECh. 6.1 - Prob. 25ECh. 6.1 - Prob. 26ECh. 6.1 - Prob. 27ECh. 6.1 - Prob. 28ECh. 6.1 - Prob. 29ECh. 6.1 - Prob. 30ECh. 6.1 - Prob. 31ECh. 6.1 - Prob. 32ECh. 6.1 - Prob. 33ECh. 6.1 - Prob. 34ECh. 6.1 - The formula C = 5 9 ( F − 32 ) expresses the...Ch. 6.1 - Prob. 36ECh. 6.1 - Prob. 37ECh. 6.1 - Prob. 38ECh. 6.1 - In Exercises 39-40, name the property used to go...Ch. 6.1 - Prob. 40ECh. 6.1 - In Exercises 41-62, simplify each algebraic...Ch. 6.1 - Prob. 42ECh. 6.1 - Prob. 43ECh. 6.1 - Prob. 44ECh. 6.1 - In Exercises 41-62, simplify each algebraic...Ch. 6.1 - In Exercises 41-62, simplify each algebraic...Ch. 6.1 - Prob. 47ECh. 6.1 - Prob. 48ECh. 6.1 - Prob. 49ECh. 6.1 - In Exercises 41-62, simplify each algebraic...Ch. 6.1 - Prob. 51ECh. 6.1 - Prob. 52ECh. 6.1 - Prob. 53ECh. 6.1 - In Exercises 41-62, simplify each algebraic...Ch. 6.1 - In Exercises 41-62, simplify each algebraic...Ch. 6.1 - Prob. 56ECh. 6.1 - Prob. 57ECh. 6.1 - In Exercises 41-62, simplify each algebraic...Ch. 6.1 - Prob. 59ECh. 6.1 - Prob. 60ECh. 6.1 - Prob. 61ECh. 6.1 - Prob. 62ECh. 6.1 - Prob. 63ECh. 6.1 - Prob. 64ECh. 6.1 - Prob. 65ECh. 6.1 - Prob. 66ECh. 6.1 - 67. If you exercise goal is to improve...Ch. 6.1 - Prob. 68ECh. 6.1 - The bar graph shows the estimated number of...Ch. 6.1 - Prob. 70ECh. 6.1 - Prob. 71ECh. 6.1 - Source: MonsterCollege TM The mathematical model p...Ch. 6.1 - Read the Blitzer Bonus on page 346. Use the...Ch. 6.1 - Prob. 74ECh. 6.1 - Prob. 75ECh. 6.1 - Prob. 76ECh. 6.1 - Prob. 77ECh. 6.1 - Prob. 78ECh. 6.1 - Prob. 79ECh. 6.1 - Prob. 80ECh. 6.1 - Prob. 81ECh. 6.1 - Prob. 82ECh. 6.1 - Prob. 83ECh. 6.1 - Prob. 84ECh. 6.1 - Prob. 85ECh. 6.1 - Prob. 86ECh. 6.1 - Prob. 87ECh. 6.1 - Prob. 88ECh. 6.1 - Prob. 89ECh. 6.1 - Prob. 90ECh. 6.1 - Prob. 91ECh. 6.1 - Prob. 92ECh. 6.1 - A business that manufactures small alarm clocks...Ch. 6.2 - CHECK POINT 1 Solve and check: Ch. 6.2 - CHECK POINT 2 Solve and check: 6 ( x − 3 ) − 10 x...Ch. 6.2 - CHECK POINT 3 Solve the equation: 2 x + 9 = 8 x −...Ch. 6.2 - CHECK POINT 4 Solve and check: 4 ( 2 x + 1 ) = 29...Ch. 6.2 - CHECK POINT 5 Solve and check: 2 x 3 = 7 − x 2 .Ch. 6.2 - CHECK POINT 6 Use the model the low-humor group...Ch. 6.2 - $$ CHECK POINT 7 Solve each proportion and check:...Ch. 6.2 - CHECK POINT 8 The property tax on a house with an...Ch. 6.2 - CHECK POINT 9 Wildlife biologists catch, tag and...Ch. 6.2 - CHECK POINT 10 Solve: 3 x + 7 = 3 ( x + 1 ) .Ch. 6.2 - CHECK POINT 11 Solve: . Ch. 6.2 - 1. An equation in the form such as is called...Ch. 6.2 - 2. Two or more equations that have the same...Ch. 6.2 - 3. The addition property of equality states that...Ch. 6.2 - 4. The multiplication property of equality states...Ch. 6.2 - 5. The first step in solving is to ____. Ch. 6.2 - 6. The algebraic expression can be ____, whereas...Ch. 6.2 - The equation x 4 = 2 + x 3 can be cleared of...Ch. 6.2 - A statement that two ratios are equal is called...Ch. 6.2 - The cross-products principle states that if a b =...Ch. 6.2 - In solving an equation, if you eliminate the...Ch. 6.2 - 11. In solving an equation with variable x, if you...Ch. 6.2 - In Exercises 12-15, determine whether each...Ch. 6.2 - In Exercises 12-15, determine whether each...Ch. 6.2 - In Exercises 12-15, determine whether each...Ch. 6.2 - In Exercises 12-15, determine whether each...Ch. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 2ECh. 6.2 - Prob. 3ECh. 6.2 - Prob. 4ECh. 6.2 - Prob. 5ECh. 6.2 - Prob. 6ECh. 6.2 - Prob. 7ECh. 6.2 - Prob. 8ECh. 6.2 - Prob. 9ECh. 6.2 - Prob. 10ECh. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 12ECh. 6.2 - Prob. 13ECh. 6.2 - Prob. 14ECh. 6.2 - Prob. 15ECh. 6.2 - Prob. 16ECh. 6.2 - Prob. 17ECh. 6.2 - Prob. 18ECh. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 20ECh. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - Prob. 22ECh. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 24ECh. 6.2 - Prob. 25ECh. 6.2 - Prob. 26ECh. 6.2 - Prob. 27ECh. 6.2 - Prob. 28ECh. 6.2 - Prob. 29ECh. 6.2 - Prob. 30ECh. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - Prob. 32ECh. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 34ECh. 6.2 - Prob. 35ECh. 6.2 - Prob. 36ECh. 6.2 - Prob. 37ECh. 6.2 - Prob. 38ECh. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 40ECh. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 42ECh. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - Prob. 46ECh. 6.2 - In Exercises 1-58, solve and check each...Ch. 6.2 - Prob. 48ECh. 6.2 - Prob. 49ECh. 6.2 - Prob. 50ECh. 6.2 - Prob. 51ECh. 6.2 - Prob. 52ECh. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - Prob. 56ECh. 6.2 - Prob. 57ECh. 6.2 - In Exercises 1-58, solve and check each equation....Ch. 6.2 - In Exercises 59-72, solve each proportion and...Ch. 6.2 - Prob. 60ECh. 6.2 - In Exercises 59-72, solve each proportion and...Ch. 6.2 - Prob. 62ECh. 6.2 - In Exercises 59-72, solve each proportion and...Ch. 6.2 - Prob. 64ECh. 6.2 - Prob. 65ECh. 6.2 - Prob. 66ECh. 6.2 - In Exercises 59-72, solve each proportion and...Ch. 6.2 - Prob. 68ECh. 6.2 - Prob. 69ECh. 6.2 - Prob. 70ECh. 6.2 - In Exercises 59-72, solve each proportion and...Ch. 6.2 - In Exercises 59-72, solve each proportion and...Ch. 6.2 - Prob. 73ECh. 6.2 - Prob. 74ECh. 6.2 - Prob. 75ECh. 6.2 - Prob. 76ECh. 6.2 - Prob. 77ECh. 6.2 - Prob. 78ECh. 6.2 - Prob. 79ECh. 6.2 - Prob. 80ECh. 6.2 - Prob. 81ECh. 6.2 - Prob. 82ECh. 6.2 - Prob. 83ECh. 6.2 - Prob. 84ECh. 6.2 - In Exercises 73-92, solve each equation. Use set...Ch. 6.2 - In Exercises 73-92, solve each equation. Use set...Ch. 6.2 - In Exercises 73-92, solve each equation. Use set...Ch. 6.2 - Prob. 88ECh. 6.2 - In Exercises 73-92, solve each equation. Use set...Ch. 6.2 - Prob. 90ECh. 6.2 - Prob. 91ECh. 6.2 - In Exercises 73-92, solve each equation. Use set...Ch. 6.2 - Prob. 93ECh. 6.2 - Prob. 94ECh. 6.2 - Prob. 95ECh. 6.2 - 96. Evaluate for x satisfying and y satisfying...Ch. 6.2 - Prob. 97ECh. 6.2 - In Exercises 97- 104, solve each equation. 98. Ch. 6.2 - Prob. 99ECh. 6.2 - Prob. 100ECh. 6.2 - Prob. 101ECh. 6.2 - Prob. 102ECh. 6.2 - Prob. 103ECh. 6.2 - Prob. 104ECh. 6.2 - The mathematical model describe a weight, W in...Ch. 6.2 - The mathematical model W 2 − 3 H = 53 describe a...Ch. 6.2 - Grade Inflation. The bar graph shows the...Ch. 6.2 - Grade Inflation. The bar graph shows the...Ch. 6.2 - 109. The volume of blood in a person’s body is...Ch. 6.2 - Prob. 110ECh. 6.2 - 111. An alligator’s tail length is proportional to...Ch. 6.2 - 112. An object’s weight on the moon is...Ch. 6.2 - Prob. 113ECh. 6.2 - 114. To estimate the number of base in a lake,...Ch. 6.2 - Prob. 115ECh. 6.2 - State the addition property of equality and give...Ch. 6.2 - Prob. 117ECh. 6.2 - Prob. 118ECh. 6.2 - Prob. 119ECh. 6.2 - Prob. 120ECh. 6.2 - Prob. 121ECh. 6.2 - Prob. 122ECh. 6.2 - Prob. 123ECh. 6.2 - Prob. 124ECh. 6.2 - Prob. 125ECh. 6.2 - Prob. 126ECh. 6.2 - Prob. 127ECh. 6.2 - Prob. 128ECh. 6.2 - 129. Write three equations whose solution set is...Ch. 6.2 - 130. If x represent a number, write an English...Ch. 6.2 - 131. A woman’s height, h, is related to the length...Ch. 6.3 - CHECK POINT 1 The average yearly salary of a woman...Ch. 6.3 - Prob. 2CPCh. 6.3 - CHECK POINT 3 The toll to a bridge costs $5....Ch. 6.3 - CHECK POINT 4 After a 30% price reducing, you...Ch. 6.3 - Prob. 5CPCh. 6.3 - Prob. 6CPCh. 6.3 - 1. According to the U.S. Office of Management and...Ch. 6.3 - In 2000, 31% of U.S. adults viewed a college...Ch. 6.3 - Prob. 3CVCCh. 6.3 - Prob. 4CVCCh. 6.3 - Prob. 5CVCCh. 6.3 - Prob. 6CVCCh. 6.3 - Use the five-step strategy for solving word...Ch. 6.3 - Use the five- step strategy for solving word...Ch. 6.3 - Prob. 3ECh. 6.3 - Prob. 4ECh. 6.3 - Use the five- step strategy for solving word...Ch. 6.3 - Use the five- step strategy for solving word...Ch. 6.3 - Use the five- step strategy for solving word...Ch. 6.3 - Prob. 8ECh. 6.3 - Prob. 9ECh. 6.3 - Prob. 10ECh. 6.3 - In Exercises 11-18, write each English phrase as...Ch. 6.3 - Prob. 12ECh. 6.3 - In Exercises 11-18, write each English phrase as...Ch. 6.3 - In Exercises 11-18, write each English phrase as...Ch. 6.3 - Prob. 15ECh. 6.3 - Prob. 16ECh. 6.3 - Prob. 17ECh. 6.3 - In Exercises 11-18, write each English phrase as...Ch. 6.3 - How will you spend your average life expectancy of...Ch. 6.3 - How will you spend your average life expectancy of...Ch. 6.3 - The bar graph shows average yearly earnings in the...Ch. 6.3 - The bar graph shows average yearly earnings in the...Ch. 6.3 - Prob. 23ECh. 6.3 - Prob. 24ECh. 6.3 - On average, every minute of every day, 158 babies...Ch. 6.3 - On average, every minute of every day, 158 babies...Ch. 6.3 - Prob. 27ECh. 6.3 - Prob. 28ECh. 6.3 - Prob. 29ECh. 6.3 - You need to rent a rug cleaner. Company A will...Ch. 6.3 - Prob. 31ECh. 6.3 - Prob. 32ECh. 6.3 - Prob. 33ECh. 6.3 - Prob. 34ECh. 6.3 - Prob. 35ECh. 6.3 - Prob. 36ECh. 6.3 - Prob. 37ECh. 6.3 - Prob. 38ECh. 6.3 - Prob. 39ECh. 6.3 - Prob. 40ECh. 6.3 - Prob. 41ECh. 6.3 - Prob. 42ECh. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - Prob. 44ECh. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - In Exercises 43-60, solve each formula for the...Ch. 6.3 - Prob. 58ECh. 6.3 - Prob. 59ECh. 6.3 - Prob. 60ECh. 6.3 - Prob. 61ECh. 6.3 - Prob. 62ECh. 6.3 - Prob. 63ECh. 6.3 - Prob. 64ECh. 6.3 - Make Sense? In Exercises 65-68, determine whether...Ch. 6.3 - Make Sense? In Exercises 65-68, determine whether...Ch. 6.3 - Prob. 67ECh. 6.3 - Prob. 68ECh. 6.3 - Prob. 69ECh. 6.3 - Prob. 70ECh. 6.3 - Prob. 71ECh. 6.3 - Prob. 72ECh. 6.3 - A thief steals a number of rare plants from a...Ch. 6.3 - Prob. 74ECh. 6.3 - Prob. 75ECh. 6.3 - One of the best ways to learn how to solve a word...Ch. 6.4 - $$ CHECK POINT I Graph each set: a. { x | x < 4 }...Ch. 6.4 - Prob. 2CPCh. 6.4 - Prob. 3CPCh. 6.4 - Prob. 4CPCh. 6.4 - Prob. 5CPCh. 6.4 - Prob. 6CPCh. 6.4 - Prob. 7CPCh. 6.4 - 1. On a number line, an open dot indicates that a...Ch. 6.4 - 2. If an inequality’s solution set consists of...Ch. 6.4 - 3. If an inequality’s solution set consists of all...Ch. 6.4 - Prob. 4CVCCh. 6.4 - Prob. 5CVCCh. 6.4 - Prob. 6CVCCh. 6.4 - Prob. 7CVCCh. 6.4 - In Exercises 5-8, determine whether each statement...Ch. 6.4 - In Exercises 1-12, graph each set of real numbers...Ch. 6.4 - Prob. 2ECh. 6.4 - Prob. 3ECh. 6.4 - In Exercises 1-12, graph each set of real numbers...Ch. 6.4 - Prob. 5ECh. 6.4 - Prob. 6ECh. 6.4 - Prob. 7ECh. 6.4 - Prob. 8ECh. 6.4 - Prob. 9ECh. 6.4 - Prob. 10ECh. 6.4 - Prob. 11ECh. 6.4 - Prob. 12ECh. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - Prob. 30ECh. 6.4 - Prob. 31ECh. 6.4 - Prob. 32ECh. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - Prob. 34ECh. 6.4 - Prob. 35ECh. 6.4 - Prob. 36ECh. 6.4 - Prob. 37ECh. 6.4 - Prob. 38ECh. 6.4 - Prob. 39ECh. 6.4 - Prob. 40ECh. 6.4 - Prob. 41ECh. 6.4 - Prob. 42ECh. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - Prob. 52ECh. 6.4 - Prob. 53ECh. 6.4 - Prob. 54ECh. 6.4 - Prob. 55ECh. 6.4 - Prob. 56ECh. 6.4 - Prob. 57ECh. 6.4 - Prob. 58ECh. 6.4 - Prob. 59ECh. 6.4 - Prob. 60ECh. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - In Exercises 13-66, solve each inequality and...Ch. 6.4 - Prob. 64ECh. 6.4 - Prob. 65ECh. 6.4 - Prob. 66ECh. 6.4 - Prob. 67ECh. 6.4 - Prob. 68ECh. 6.4 - Prob. 69ECh. 6.4 - Prob. 70ECh. 6.4 - In Exercises 71-76, use set-builder notation to...Ch. 6.4 - Prob. 72ECh. 6.4 - Prob. 73ECh. 6.4 - Prob. 74ECh. 6.4 - Prob. 75ECh. 6.4 - Prob. 76ECh. 6.4 - Prob. 77ECh. 6.4 - Prob. 78ECh. 6.4 - The graphs show that the three components of love,...Ch. 6.4 - Prob. 80ECh. 6.4 - Prob. 81ECh. 6.4 - The graphs show that the three components of love,...Ch. 6.4 - Prob. 83ECh. 6.4 - Prob. 84ECh. 6.4 - models the percentage of U.S. households in which...Ch. 6.4 - Prob. 86ECh. 6.4 - Prob. 87ECh. 6.4 - Prob. 88ECh. 6.4 - Prob. 89ECh. 6.4 - Prob. 90ECh. 6.4 - Prob. 91ECh. 6.4 - Prob. 92ECh. 6.4 - Prob. 93ECh. 6.4 - Prob. 94ECh. 6.4 - Prob. 95ECh. 6.4 - Prob. 96ECh. 6.4 - Prob. 97ECh. 6.4 - Prob. 98ECh. 6.4 - Prob. 99ECh. 6.4 - Prob. 100ECh. 6.4 - Prob. 101ECh. 6.4 - Prob. 102ECh. 6.4 - Prob. 103ECh. 6.4 - Prob. 104ECh. 6.5 - CHECK POINT 1 Multiple: Ch. 6.5 - CHECK POINT 2 Multiple: ( 7 x + 5 ) ( 4 x − 3 )Ch. 6.5 - Prob. 3CPCh. 6.5 - Prob. 4CPCh. 6.5 - CHECK POINT 5 Factor: 5 x 2 − 14 x + 8Ch. 6.5 - Prob. 6CPCh. 6.5 - Prob. 7CPCh. 6.5 - Prob. 8CPCh. 6.5 - Prob. 9CPCh. 6.5 - Prob. 10CPCh. 6.5 - Prob. 11CPCh. 6.5 - Prob. 12CPCh. 6.5 - Prob. 1CVCCh. 6.5 - Prob. 2CVCCh. 6.5 - Prob. 3CVCCh. 6.5 - Prob. 4CVCCh. 6.5 - Prob. 5CVCCh. 6.5 - Prob. 6CVCCh. 6.5 - Prob. 7CVCCh. 6.5 - Prob. 8CVCCh. 6.5 - Prob. 9CVCCh. 6.5 - Prob. 10CVCCh. 6.5 - The zero-product principle states that if A B = 0...Ch. 6.5 - Prob. 12CVCCh. 6.5 - Prob. 13CVCCh. 6.5 - Prob. 14CVCCh. 6.5 - Prob. 15CVCCh. 6.5 - Prob. 16CVCCh. 6.5 - Prob. 17CVCCh. 6.5 - Prob. 18CVCCh. 6.5 - Prob. 1ECh. 6.5 - Prob. 2ECh. 6.5 - Prob. 3ECh. 6.5 - Prob. 4ECh. 6.5 - Prob. 5ECh. 6.5 - Prob. 6ECh. 6.5 - Prob. 7ECh. 6.5 - Prob. 8ECh. 6.5 - Prob. 9ECh. 6.5 - Prob. 10ECh. 6.5 - Factor the trinomials in Exercises 9-32, or state...Ch. 6.5 - Prob. 12ECh. 6.5 - Factor the trinomials in Exercises 9-32, or state...Ch. 6.5 - Prob. 14ECh. 6.5 - Prob. 15ECh. 6.5 - Prob. 16ECh. 6.5 - Prob. 17ECh. 6.5 - Prob. 18ECh. 6.5 - Prob. 19ECh. 6.5 - Prob. 20ECh. 6.5 - Factor the trinomials in Exercises 9-32, or state...Ch. 6.5 - Prob. 22ECh. 6.5 - Factor the trinomials in Exercises 9-32, or state...Ch. 6.5 - Prob. 24ECh. 6.5 - Prob. 25ECh. 6.5 - Prob. 26ECh. 6.5 - Prob. 27ECh. 6.5 - Prob. 28ECh. 6.5 - Factor the trinomials in Exercises 9-32, or state...Ch. 6.5 - Prob. 30ECh. 6.5 - Prob. 31ECh. 6.5 - Prob. 32ECh. 6.5 - In Exercises 33-36, solve each equation using the...Ch. 6.5 - Prob. 34ECh. 6.5 - In Exercises 33-36, solve each equation using the...Ch. 6.5 - Prob. 36ECh. 6.5 - Solve the quadratic equations in Exercises 37-52,...Ch. 6.5 - Prob. 38ECh. 6.5 - Prob. 39ECh. 6.5 - Prob. 40ECh. 6.5 - Prob. 41ECh. 6.5 - Prob. 42ECh. 6.5 - Prob. 43ECh. 6.5 - Prob. 44ECh. 6.5 - Prob. 45ECh. 6.5 - Prob. 46ECh. 6.5 - Prob. 47ECh. 6.5 - Prob. 48ECh. 6.5 - Prob. 49ECh. 6.5 - Prob. 50ECh. 6.5 - Prob. 51ECh. 6.5 - Prob. 52ECh. 6.5 - Prob. 53ECh. 6.5 - Prob. 54ECh. 6.5 - Solve the equations in Exercises 53-72 using the...Ch. 6.5 - Prob. 56ECh. 6.5 - Prob. 57ECh. 6.5 - Prob. 58ECh. 6.5 - Prob. 59ECh. 6.5 - Prob. 60ECh. 6.5 - Prob. 61ECh. 6.5 - Prob. 62ECh. 6.5 - Prob. 63ECh. 6.5 - Prob. 64ECh. 6.5 - Prob. 65ECh. 6.5 - Prob. 66ECh. 6.5 - Prob. 67ECh. 6.5 - Prob. 68ECh. 6.5 - Prob. 69ECh. 6.5 - Prob. 70ECh. 6.5 - Prob. 71ECh. 6.5 - Prob. 72ECh. 6.5 - Prob. 73ECh. 6.5 - Prob. 74ECh. 6.5 - Prob. 75ECh. 6.5 - Prob. 76ECh. 6.5 - Prob. 77ECh. 6.5 - Prob. 78ECh. 6.5 - Prob. 79ECh. 6.5 - Prob. 80ECh. 6.5 - Prob. 81ECh. 6.5 - Prob. 82ECh. 6.5 - Prob. 83ECh. 6.5 - Prob. 84ECh. 6.5 - Prob. 85ECh. 6.5 - Prob. 86ECh. 6.5 - Prob. 87ECh. 6.5 - Prob. 88ECh. 6.5 - 89. Explain how to factor . Ch. 6.5 - Prob. 90ECh. 6.5 - Prob. 91ECh. 6.5 - Prob. 92ECh. 6.5 - Prob. 93ECh. 6.5 - Prob. 94ECh. 6.5 - Prob. 95ECh. 6.5 - Prob. 96ECh. 6.5 - The radicand of the quadratic formula, b 2 − 4 a c...Ch. 6.5 - Prob. 98ECh. 6.5 - In Exercises 98-99, find all positive integers b...Ch. 6.5 - Prob. 100ECh. 6.5 - Prob. 101ECh. 6 - In Exercises 1-3, evaluate the algebraic...Ch. 6 - In Exercises 1-3, evaluate the algebraic...Ch. 6 - Prob. 3RECh. 6 - Prob. 4RECh. 6 - In Exercises 5-7, simplify each algebraic...Ch. 6 - Prob. 6RECh. 6 - Prob. 7RECh. 6 - Prob. 8RECh. 6 - Prob. 9RECh. 6 - Prob. 10RECh. 6 - Prob. 11RECh. 6 - Prob. 12RECh. 6 - Prob. 13RECh. 6 - Prob. 14RECh. 6 - Prob. 15RECh. 6 - In Exercises 15-18, solve each proportion. 16. Ch. 6 - Prob. 17RECh. 6 - Prob. 18RECh. 6 - Prob. 19RECh. 6 - 20. To determine the number of trout in a lake, a...Ch. 6 - 21. The line graph showy he cost of inflation....Ch. 6 - Prob. 22RECh. 6 - Prob. 23RECh. 6 - 24. You are choosing between two cellphone plans....Ch. 6 - Prob. 25RECh. 6 - Prob. 26RECh. 6 - Prob. 27RECh. 6 - Prob. 28RECh. 6 - Prob. 29RECh. 6 - Prob. 30RECh. 6 - Prob. 31RECh. 6 - Prob. 32RECh. 6 - Prob. 33RECh. 6 - Prob. 34RECh. 6 - Prob. 35RECh. 6 - Prob. 36RECh. 6 - In Exercises 31-37, solve each inequality and...Ch. 6 - Prob. 38RECh. 6 - Prob. 39RECh. 6 - Prob. 40RECh. 6 - Prob. 41RECh. 6 - Prob. 42RECh. 6 - Prob. 43RECh. 6 - Prob. 44RECh. 6 - Prob. 45RECh. 6 - Prob. 46RECh. 6 - Prob. 47RECh. 6 - Prob. 48RECh. 6 - Prob. 49RECh. 6 - Prob. 50RECh. 6 - Prob. 51RECh. 6 - Prob. 52RECh. 6 - Prob. 53RECh. 6 - Prob. 54RECh. 6 - Prob. 55RECh. 6 - 1. Evaluate when . Ch. 6 - Prob. 2TCh. 6 - Prob. 3TCh. 6 - Prob. 4TCh. 6 - Prob. 5TCh. 6 - Prob. 6TCh. 6 - Prob. 7TCh. 6 - Prob. 8TCh. 6 - Prob. 9TCh. 6 - Prob. 10TCh. 6 - Prob. 11TCh. 6 - Prob. 12TCh. 6 - Prob. 13TCh. 6 - Prob. 14TCh. 6 - Prob. 15TCh. 6 - Prob. 16TCh. 6 - Prob. 17TCh. 6 - Prob. 18TCh. 6 - Prob. 19TCh. 6 - Prob. 20TCh. 6 - Prob. 21TCh. 6 - Prob. 22TCh. 6 - Prob. 23TCh. 6 - Prob. 24TCh. 6 - Prob. 25TCh. 6 - Prob. 26T

Additional Math Textbook Solutions

Find more solutions based on key concepts
1. How much money is Joe earning when he’s 30?

Pathways To Math Literacy (looseleaf)

Is there a relationship between wine consumption and deaths from heart disease? The table gives data from 19 de...

College Algebra Essentials (5th Edition)

(a) Make a stem-and-leaf plot for these 24 observations on the number of customers who used a down-town CitiBan...

APPLIED STAT.IN BUS.+ECONOMICS

Evaluate the integrals in Exercises 1–46. 1.

University Calculus: Early Transcendentals (4th Edition)

Empirical versus Theoretical A Monopoly player claims that the probability of getting a 4 when rolling a six-si...

Introductory Statistics

For Problems 23-28, write in simpler form, as in Example 4. logbFG

Finite Mathematics for Business, Economics, Life Sciences and Social Sciences

Knowledge Booster
Background pattern image
Math
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Text book image
Intermediate Algebra
Algebra
ISBN:9781285195728
Author:Jerome E. Kaufmann, Karen L. Schwitters
Publisher:Cengage Learning
Text book image
Algebra for College Students
Algebra
ISBN:9781285195780
Author:Jerome E. Kaufmann, Karen L. Schwitters
Publisher:Cengage Learning
Text book image
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Text book image
PREALGEBRA
Algebra
ISBN:9781938168994
Author:OpenStax
Publisher:OpenStax
Text book image
Elementary Algebra
Algebra
ISBN:9780998625713
Author:Lynn Marecek, MaryAnne Anthony-Smith
Publisher:OpenStax - Rice University
SEE MORE TEXTBOOKS
Interpreting Graphs of Quadratic Equations (GMAT/GRE/CAT/Bank PO/SSC CGL) | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=BHgewRcuoRM;License: Standard YouTube License, CC-BY
Solve a Trig Equation in Quadratic Form Using the Quadratic Formula (Cosine, 4 Solutions); Author: Mathispower4u;https://www.youtube.com/watch?v=N6jw_i74AVQ;License: Standard YouTube License, CC-BY