
Concept explainers
Break into small groups and discuss the following topics. Organize a brief outline in which you summarize the main points of your group discussion.
Iris setosa is a beautiful wildflower that is found in such diverse places as Alaska, the Gulf of St. Lawrence, much of North America, and even in English meadows and parks. R. A. Fisher, with his colleague Dr. Edgar Anderson, studied these flowers extensively. Dr. Anderson described how he collected information on irises:
I have studied such irises as I could get to see, in as great detail as possible, measuring iris standard after iris standard and iris fall after iris fall, sitting squat-legged with record book and ruler in mountain meadows, in cypress swamps, on lake beaches, and in English parks. [E. Anderson, “The Irises of the Gaspé Peninsula,” Bulletin, American Iris Society, Vol. 59 pp. 2–5, 1935.]
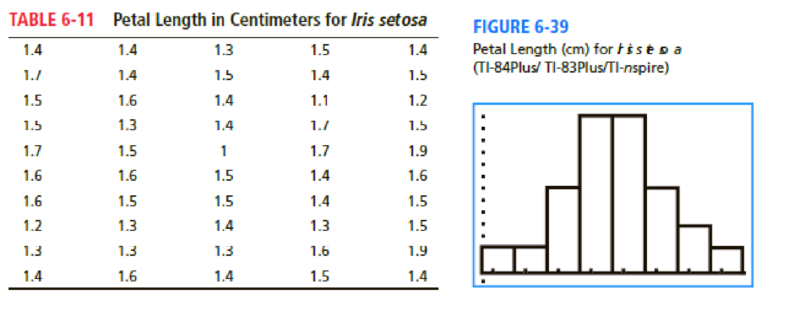
The data in Table 6-11 were collected by Dr. Anderson and were published by his friend and colleague R. A. Fisher in a paper titled “The Use of Multiple Measurements in Taxonomic Problems” (Annals of Eugenics, part II, pp. 179–188, 1936). To find these data, visit the Carnegie Mellon University Data and Story Library (DASL) web site. From the DASL site, look under Biology and Wild iris select Fisher's Irises Story.
Let x be a random variable representing petal length. Using a TI-84Plus/ TI-83Plus/TI-nspire calculator, it was found that the sample
- (a) Examine the histogram for petal lengths. Would you say that the distribution is approximately mound-shaped and symmetric? Our sample has only 50 irises; if many thousands of irises had been used, do you think the distribution would look even more like a normal curve? Let x be the petal length of Iris setosa. Research has shown that x has an approximately
normal distribution , with mean μ = 1.5 cm and standard deviation σ = 0.2 cm. - (b) Use the
empirical rule with μ = 1.5 and σ = 0.2 to get an interval into which approximately 68% of the petal lengths will fall. Repeat this for 95% and 99.7%. Examine the raw data and compute the percentage of the raw data that actually fall into each of these intervals (the 68% interval, the 95% interval, and the 99.7% interval). Compare your computed percentages with those given by the empirical rule. - (c) Compute the
probability that a petal length is between 1.3 and 1.6 cm. Compute the probability that a petal length is greater than 1.6 cm. - (d) Suppose that a random sample of 30 irises is obtained. Compute the probability that the average petal length for this sample is between 1.3 and 1.6 cm. Compute the probability that the average petal length is greater than 1.6 cm.
- (e) Compare your answers to parts (c) and (d). Do you notice any differences? Why would these differences occur?
(a)
Explain whether the distribution is approximately mound-shaped and symmetrical.
Answer to Problem 1DH
Yes, the distribution is approximately mound-shaped and symmetrical.
Explanation of Solution
From the histogram for petal lengths, the distribution is approximately bell-shaped or mound-shaped and symmetrical because approximately the left half of the graph is the mirror image of the right half of the graph.
Our sample has only 50 irises; if many thousands of irises had been used, the distribution would look more similar to a normal curve because the sample is very large and the distribution of the sample will be approximately normally distributed.
(b)
Obtain the 68%, 95%, and 99% interval and compare the computed percentages with those given by the empirical rule.
Answer to Problem 1DH
The 68%, 95%, and 99% intervals are (1.3, 1.7), (1.1, 1.9), and (0.9, 2.1), respectively.
Explanation of Solution
Let x be the petal length of Iris Setosa and x has an approximately normal distribution, with mean
It is known that 68% of the observations will fall within one standard deviation of mean.
The 68% interval is as follows:
The 95% of the observations will fall within two standard deviations of mean.
The 95% interval is as follows:
The 99.7% of the observations will fall within two standard deviations of mean.
The 99.7% interval is as follows:
The 33 observations fall within the intervals 1.3 and 1.7;thus, the percentage of data within the intervals 1.3 and 1.7 is
The 46 observations fall within the intervals 1.1 and 1.9;thus, the percentage of data within the intervals 1.3 and 1.7 is
All data values fall within the intervals 0.9 and 2.1;thus, the percentage of data within the intervals 1.3 and 1.7 is
(c)
Obtain the probability that a petal length is between 1.3 and 1.6 cm and the probability that a petal length is greater than 1.6 cm.
Answer to Problem 1DH
The probability that a petal length is between 1.3 and 1.6 cm is 0.5328.
The probability that a petal length is greater than 1.6 cm is 0.3085.
Explanation of Solution
Let x be the petal length of Iris Setosa and x has an approximately normal distribution, with mean
The interval
The z-score for
The z-score for
The probabilitythat a petal length is between 1.3 and 1.6 cm is obtained as shown below:
In Appendix II, Table 5: Areas of a Standard Normal Distribution.
The values of
The probability corresponding to 0.5 is 0.6915 and the probability corresponding to -1 is 0.1587.
Hence, the probability that a petal length is between 1.3 and 1.6 cm is 0.5328.
The z-score for
The probability that a petal length is greater than 1.6 cm is obtained as given below:
Using Table 5 from the Appendix, the probability corresponding to 0.5 is 0.6915.
Hence, the probability that a petal length is greater than 1.6 cm is 0.3085.
(d)
Obtain the probability that the average petal length is between 1.3 and 1.6 cm and the probability that the average petal length is greater than 1.6 cm.
Answer to Problem 1DH
The probability that the average petal length is between 1.3 and 1.6 cm is 0.9972.
The probability that the averagepetal length is greater than 1.6 cm is 0.0027.
Explanation of Solution
Let x be the petal length of Iris Setosa and x has an approximately normal distribution, with mean
With sample size as n = 30, the sampling distribution for
The interval
The z-score for
The z-score for
The probability that the average petal length is between 1.3 and 1.6 cm is obtained as shown below:
In Appendix II, Table 5: Areas of a Standard Normal Distribution.
The values of
The probability corresponding to 2.78 is 0.9973 and the probability corresponding to -5.55does exist; Thus, it is considered as 0.0001.
Hence, the probability that the average petal length is between 1.3 and 1.6 cm is 0.9972.
The z-score for
The probability that the average petal length is greater than 1.6 cm is obtained as given below:
Using Table 5 from the Appendix, the probability corresponding to 2.78 is 0.9973.
Hence, the probability that the average petal length is greater than 1.6 cm is 0.0027.
(e)
Compare the results of Part (c) and Part (d); also delineate the differences.
Answer to Problem 1DH
The standard deviation of the sample mean is much smaller than the population standard deviation.
Explanation of Solution
In Part (c), x has a distribution that is approximately normal with
In Part (d),
The central limit theorem tells us that the standard deviation of the sample mean is much smaller than the population standard deviation.
Want to see more full solutions like this?
Chapter 6 Solutions
Bundle: Understandable Statistics: Concepts And Methods, 12th + Webassign, Single-term Printed Access Card
- Analyze the residuals of a linear regression model and select the best response. yes, the residual plot does not show a curve no, the residual plot shows a curve yes, the residual plot shows a curve no, the residual plot does not show a curve I answered, "No, the residual plot shows a curve." (and this was incorrect). I am not sure why I keep getting these wrong when the answer seems obvious. Please help me understand what the yes and no references in the answer.arrow_forwarda. Find the value of A.b. Find pX(x) and py(y).c. Find pX|y(x|y) and py|X(y|x)d. Are x and y independent? Why or why not?arrow_forwardAnalyze the residuals of a linear regression model and select the best response.Criteria is simple evaluation of possible indications of an exponential model vs. linear model) no, the residual plot does not show a curve yes, the residual plot does not show a curve yes, the residual plot shows a curve no, the residual plot shows a curve I selected: yes, the residual plot shows a curve and it is INCORRECT. Can u help me understand why?arrow_forward
- You have been hired as an intern to run analyses on the data and report the results back to Sarah; the five questions that Sarah needs you to address are given below. please do it step by step on excel Does there appear to be a positive or negative relationship between price and screen size? Use a scatter plot to examine the relationship. Determine and interpret the correlation coefficient between the two variables. In your interpretation, discuss the direction of the relationship (positive, negative, or zero relationship). Also discuss the strength of the relationship. Estimate the relationship between screen size and price using a simple linear regression model and interpret the estimated coefficients. (In your interpretation, tell the dollar amount by which price will change for each unit of increase in screen size). Include the manufacturer dummy variable (Samsung=1, 0 otherwise) and estimate the relationship between screen size, price and manufacturer dummy as a multiple…arrow_forwardHere is data with as the response variable. x y54.4 19.124.9 99.334.5 9.476.6 0.359.4 4.554.4 0.139.2 56.354 15.773.8 9-156.1 319.2Make a scatter plot of this data. Which point is an outlier? Enter as an ordered pair, e.g., (x,y). (x,y)= Find the regression equation for the data set without the outlier. Enter the equation of the form mx+b rounded to three decimal places. y_wo= Find the regression equation for the data set with the outlier. Enter the equation of the form mx+b rounded to three decimal places. y_w=arrow_forwardYou have been hired as an intern to run analyses on the data and report the results back to Sarah; the five questions that Sarah needs you to address are given below. please do it step by step Does there appear to be a positive or negative relationship between price and screen size? Use a scatter plot to examine the relationship. Determine and interpret the correlation coefficient between the two variables. In your interpretation, discuss the direction of the relationship (positive, negative, or zero relationship). Also discuss the strength of the relationship. Estimate the relationship between screen size and price using a simple linear regression model and interpret the estimated coefficients. (In your interpretation, tell the dollar amount by which price will change for each unit of increase in screen size). Include the manufacturer dummy variable (Samsung=1, 0 otherwise) and estimate the relationship between screen size, price and manufacturer dummy as a multiple linear…arrow_forward
- Exercises: Find all the whole number solutions of the congruence equation. 1. 3x 8 mod 11 2. 2x+3= 8 mod 12 3. 3x+12= 7 mod 10 4. 4x+6= 5 mod 8 5. 5x+3= 8 mod 12arrow_forwardScenario Sales of products by color follow a peculiar, but predictable, pattern that determines how many units will sell in any given year. This pattern is shown below Product Color 1995 1996 1997 Red 28 42 21 1998 23 1999 29 2000 2001 2002 Unit Sales 2003 2004 15 8 4 2 1 2005 2006 discontinued Green 26 39 20 22 28 14 7 4 2 White 43 65 33 36 45 23 12 Brown 58 87 44 48 60 Yellow 37 56 28 31 Black 28 42 21 Orange 19 29 Purple Total 28 42 21 49 68 78 95 123 176 181 164 127 24 179 Questions A) Which color will sell the most units in 2007? B) Which color will sell the most units combined in the 2007 to 2009 period? Please show all your analysis, leave formulas in cells, and specify any assumptions you make.arrow_forwardOne hundred students were surveyed about their preference between dogs and cats. The following two-way table displays data for the sample of students who responded to the survey. Preference Male Female TOTAL Prefers dogs \[36\] \[20\] \[56\] Prefers cats \[10\] \[26\] \[36\] No preference \[2\] \[6\] \[8\] TOTAL \[48\] \[52\] \[100\] problem 1 Find the probability that a randomly selected student prefers dogs.Enter your answer as a fraction or decimal. \[P\left(\text{prefers dogs}\right)=\] Incorrect Check Hide explanation Preference Male Female TOTAL Prefers dogs \[\blueD{36}\] \[\blueD{20}\] \[\blueE{56}\] Prefers cats \[10\] \[26\] \[36\] No preference \[2\] \[6\] \[8\] TOTAL \[48\] \[52\] \[100\] There were \[\blueE{56}\] students in the sample who preferred dogs out of \[100\] total students.arrow_forward
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman





