Chapter 6, Problem 16CR

To determine

To complete: the given statement using $<,=,\text{ or}>$ .

Answer to Problem 16CR

  $m\angle 3\underset{\_}{\text{ < }}m\angle 4.$

Explanation of Solution

Given information:

The given figure is as follows:

  

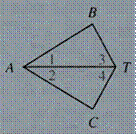
The given statement is “ $\overline{TB}\cong \overline{TC}$ and $AB<AC$ , then $m\angle 3\underset{\_}{}m\angle 4.$

Concept used:

The angle opposite to largest side is greater than the angle opposite to smallest side of the

triangle.

The angle opposite to $AB$ is $m\angle 3$

The angle opposite to $AC$ is $m\angle 4$ .

Consider $AB<AC$

  $\Rightarrow m\angle 3<m\angle 4.$

Hence, $m\angle 3\underset{\_}{\text{ < }}m\angle 4.$