
Concept explainers
a)
To draw: A decision tree for the given problem.
Introduction:
Decision tree is one of the methods used in decision-making process. It would graphically represent the available alternatives and states of nature. It would also mention the payoffs and probabilities of the alternatives. It helps to choose the best alternative that would give the best result among the alternatives.
a)
Explanation of Solution
Given information:
Three decision alternatives:
- 1st construct small stamping plant
- 2nd construct medium stamping plant
- 3rd construct stamping plant
Each decision has demand:
- Low demand which have 20% probability
- Low demand which have 80% probability
- If firm build small facility and demand turn out to be low than
NPV is $42,000,000 - If firm build small facility and demand turn out to be high than either subcontract or expand greatly, if sub contract than NPV is $42,000,000 and if expand greatly than $48,000,000.
- If firm build medium facility and demand turn out to be low than NPV is $22,000,000.
- If firm build medium facility and demand turn out to be high than either it do nothing or expand, if sub contract than NPV is $46,000,000 and if expand greatly than $50,000,000.
- If firm build large facility and demand turn out to be low than NPV is -$20,000,000.
- If firm build large facility and demand turn out to be high than NPV is $72,000,000.
As per given information, construct the decision tree:
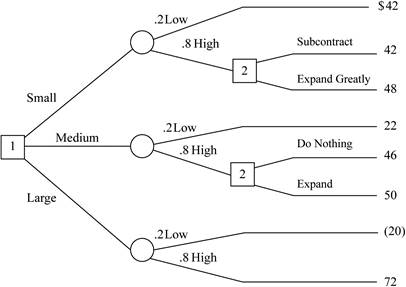
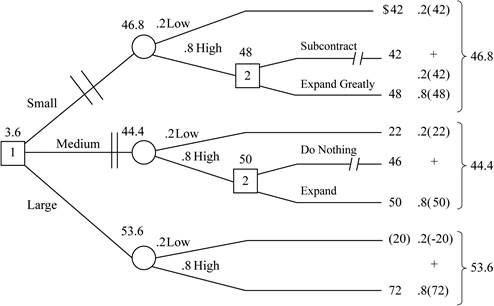
Now, calculate the value of payoff and monetary value in decision tree:
Analysis the decision from right to left:
- In the decision tree, there are two decisions making; first decision is between small, medium and large and second decision is between high demand of small and medium build facilities.
- If small facilities build with high demand then select expand greatly because the payoff of expand greatly is higher than subcontract. The payoff of expand greatly is $48,000,000 and place double slash (reject) on subcontract.
- If large facility build with high demand then select expand, because the payoff of expand greatly is higher than do nothing. The payoff of expand is $50,000,000 place double slash (reject) on do nothing.
Calculate the product of the chance probabilities and their respective payoffs for the remaining branches:
- If small facility build:
- If medium facility IS build:
- If large facility is build:
Calculation of the expected value in each alternative:
- If small facility build
- If medium facility build
- If large facility build
Here, it is obtained that large facility build have higher expected value, hence it should be selected. And small and medium facilities have to be rejected.
b)
To determine: Maximin alternatives
Introduction:
Maximin is the decision making method which is used to make decision under uncertainty. This method will find an alternative that maximizes the minimum outcome of every alternative or we can say that calculating the minimum outcome within the each alternative.
b)
Answer to Problem 9P
Explanation of Solution
Determine the worst possible alternatives from the given demand:
As definition stated above, Maximim is the selection from the best of the worst possible payoff for each alternatives.
| Alternative | Next Year’s Demand | Worst Payoff | Decision | |
| Low | High | |||
| Small | $42,000,000.00 | $48,000,000.00 | $42,000,000.00 | Best of the Worst |
| Medium | $22,000,000.00 | $50,000,000.00 | $22,000,000.00 | |
| Large | -$20,000,000.00 | $72,000,000.00 | -$20,000,000.00 | |
Hence, the best decision according to Maximin decision is to select a small medium facility.
c)
To determine: Expected value of perfect information (EVPI) and interpret it.
Expected value of perfect information: It is the rate that a person is willing to pay to gain access to perfect information. A common area which uses expected value of perfect information is the healthcare economy. This value tries to evaluate the expected cost of the uncertainty, which can be interpreted as the expected value of perfect information
The expected value of perfect information can be calculated by using below given formula:
c)
Answer to Problem 9P
Explanation of Solution
Calculate the expected value with perfection information or Expected payoff under certainty:
Calculate the expected value with perfect information:
Therefore, EVPI is $12,400,000.
d)
To determine: The sensitive analysis on P (high).
Introduction
Decision table is formats or visual representations were data is expressed arranged, determined and calculated to make a effective decision making. A decision table is a tabular representation that is used to analyze decision alternatives and states of nature.
d)
Explanation of Solution
Explanation
Here we draw each alternative to P (high), low demand should be on the left hand side and high demand value on the right hand side:
| Next Year’s Demand | ||
| Alternative | Low | High |
| Small | $42,000,000.00 | $48,000,000.00 |
| Medium | $22,000,000.00 | $50,000,000.00 |
| Large | -$20,000,000.00 | $72,000,000.00 |
Graph plot:
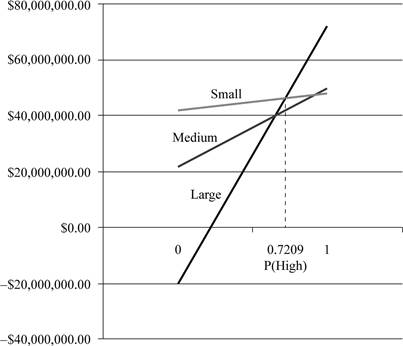
From the above graph, we can determine the value of P (high) where each alternative are optimal.
From the graph, we can obtain that small build facility is best option, because of higher expected value. For low value of P(high) while for higher and intermediate value of P(high) large is the best option.
Calculate the range needed to determine upper part of line intersects:
For each line, b is the slope of the line and x = P(High). The slope of each line
Determination of equation:
Small build facility = 42+6P (because high demand $48 million which is subtracted with low demand value $42 million)
Large build facility =-20+92P (because high demand $72million which is subtracted with low demand value -$20million)
Determination of intersection between small and large
Hence, the intersection between small and large is 0.7209.
Therefore, optimal range can be derived as:
Small: P (High) = 0 to < .7209
Large: P (High) > .7209 to 1.00
Want to see more full solutions like this?
Chapter 5 Solutions
Operations Management (Comp. Instructor's Edition)
- A local fast-food restaurant processes several customer orders at once. Service clerks’ cross paths, sometimes nearly colliding, while they trace different paths to fill customer orders. If customers order a special combination of toppings on their burgers, they must wait quite some time while the special order is cooked. How would you modify the restaurant’s operations to achieve competitive advantage? Because demand surges at lunchtime, volume flexibility is a competitive priority in the fast-food business. How would you achieve volume flexibility? What are the potential downsides of relying too heavily on automation and standardization in this industry? What data would you want to gather, if any, before making recommendations?arrow_forwardWhat are 4 key points that are interesting about this video https://youtu.be/LAoMuvYZ_QM?si=Mognj_KBU9EIOLSParrow_forwarda) Select all of the correct impacts the maturing of a product might have on OM strategy below. (Check all that apply.) A. Cost cutting is instituted. B. Inventory needs to be revised. C. Labor skills decrease. D. Product design needs to be revised. E. Design compromises are instituted. F. New human resources skills.arrow_forward
- Please help! Multifactor!arrow_forwardKlassen Toy Company, Inc., assembles two parts (parts 1 and 2): Part 1 is first processed at workstation A for 10 minutes per unit and then processed at workstation B for 20 minutes per unit. Part 2 is simultaneously processed at workstation C for 12 minutes per unit. Work stations B and C feed the parts to an assembler at workstation D, where the two parts are assembled. The time at workstation D is 15 minutes. a) The bottleneck of this process is at minutes per unit (enter your response as a whole number).arrow_forwardJust HELParrow_forward
- I need help with C. I'm strugglingarrow_forwardBy signaling their willingness to share information about their interests, but not their BATNA, a negotiator can capitalize on the powerful principle of reciprocity. Which of the following situations best illustrates the reciprocity principle? Group of answer choices A. A car salesman shares information about the town where he grew up, and his custo.mer shares that he also grew up near that town B. A cab driver takes a customer to her hotel and picks up a new customer at the hotel. C. A woman compliments a friend about her purse and the friend says thank you. D. An employee shares information about a project's progress with a coworker who is uncertain.arrow_forwardWhat benefits can negotiators get from using the strategy of multiple equivalent simultaneous offers (MESOs)? Give a real life example.arrow_forward
- What benefits can negotiators get from using the strategy of multiple equivalent simultaneous offers (MESOs)?arrow_forwardWhat is the Perspective on Research? How is the Research from a Biblical perspective conducting Business? What is the connection between Biblical principles and the concepts chosen for conducting Business Research? What is the Perspective on Research? How is the Research from a Biblical perspective conducting Business? What is the connection between Biblical principles and the concepts chosen for conducting Business Research? What is the Perspective on Research? How is the Research from a Biblical perspective conducting Business? What is the connection between Biblical principles and the concepts chosen for conducting Business Research?arrow_forwardwhat is the arithmetic average annual return per year?arrow_forward
 Practical Management ScienceOperations ManagementISBN:9781337406659Author:WINSTON, Wayne L.Publisher:Cengage,
Practical Management ScienceOperations ManagementISBN:9781337406659Author:WINSTON, Wayne L.Publisher:Cengage, Purchasing and Supply Chain ManagementOperations ManagementISBN:9781285869681Author:Robert M. Monczka, Robert B. Handfield, Larry C. Giunipero, James L. PattersonPublisher:Cengage LearningMarketingMarketingISBN:9780357033791Author:Pride, William MPublisher:South Western Educational Publishing
Purchasing and Supply Chain ManagementOperations ManagementISBN:9781285869681Author:Robert M. Monczka, Robert B. Handfield, Larry C. Giunipero, James L. PattersonPublisher:Cengage LearningMarketingMarketingISBN:9780357033791Author:Pride, William MPublisher:South Western Educational Publishing Management, Loose-Leaf VersionManagementISBN:9781305969308Author:Richard L. DaftPublisher:South-Western College Pub
Management, Loose-Leaf VersionManagementISBN:9781305969308Author:Richard L. DaftPublisher:South-Western College Pub




