Chapter 50, Problem 16A

Given: Hole centrelines $AB\Vert CD$ and $EF\Vert GH$ . Determine the values of $\angle 1$ through $\angle 22$ for these given values of $\angle 23,\angle 24$ , and $\angle 25.$
a. $\angle 23=97°,\angle 24=34°,\text{and }\angle 25=102°$
b. $\angle 23=112°23\text{'},\angle 24=27°53\text{'},\text{and }\angle 25=95°18\text{'}$

To determine

(a)

The values of angles $\angle 1\text{ through }\angle 22$.

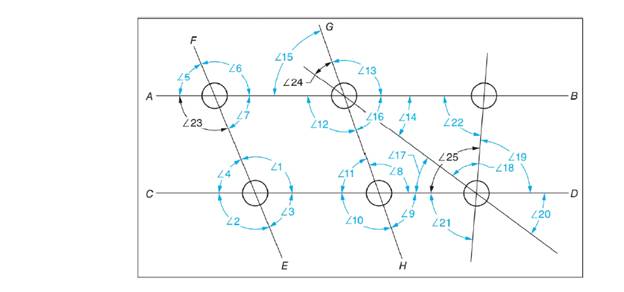
Answer to Problem 16A

The values of angles are

  $\begin{array}{l}\angle 1=97°\\ \angle 2=97°\\ \angle 3=83°\\ \angle 4=83°\\ \angle 5=83°\\ \angle 6=97°\\ \angle 7=83°\\ \angle 8=97°\\ \angle 9=83°\\ \angle 10=97°\end{array}$.

  $\begin{array}{l}\angle 11=83°\\ \angle 12=97°\\ \angle 13=97°\\ \angle 14=49°\\ \angle 15=83°\\ \angle 16=83°\\ \angle 17=49°\\ \angle 18=53°\\ \angle 19=78°\\ \angle 20=49°\\ \angle 21=78°\\ \angle 22=78°\end{array}$

Explanation of Solution

Given:

The given value of angles is

  $\begin{array}{l}\angle 23=97°\\ \angle 24=34°\\ \angle 25=102°\end{array}$.

The following figure is given

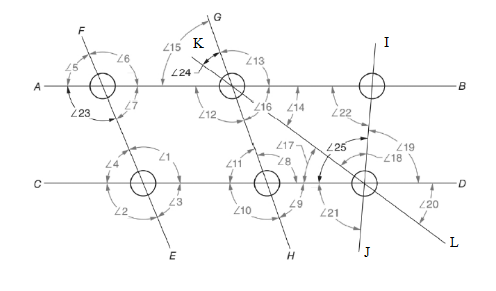
The angles $\angle 23\text{and }\angle 6$ are opposite angles. The opposite angles are equal, so, the angle $\angle 6\text{=97}°$

Now, angle 6 and angle 5 together make a straight line. The angle of straight line is 180^o. Thus, the value of angle 5 can be calculated as

  $\begin{array}{l}\angle 6+\angle 5=180°\\ 97°+\angle 5=180°\\ \angle 5=180°-97°\\ \angle 5=83°\end{array}$

The angles $\angle 7\text{and }\angle 5$ are opposite angles. The opposite angles are equal, so, the angle $\angle 7\text{=83}°$

The two lines AB and CD are parallel; hence the corresponding angles will be equal. Hence,

  $\begin{array}{l}\angle 5=\angle 4=83°\\ \angle 6=\angle 1=97°\\ \angle 23=\angle 2=97°\\ \angle 7=\angle 3=83°\end{array}$

The two lines EF and GH are also parallel; hence the corresponding angles will be equal. Hence,

  $\begin{array}{l}\angle 8=\angle 1=97°\\ \angle 9=\angle 3=83°\\ \angle 10=\angle 2=97°\\ \angle 11=\angle 4=83°\\ \end{array}$

The two lines AB and CD are parallel; hence the corresponding angles will be equal. Hence,

  $\begin{array}{l}\angle 8=\angle 13=97°\\ \angle 9=\angle 16=83°\\ \angle 10=\angle 12=97°\\ \angle 11=\angle 15=83°\end{array}$

Now, angle 25 and angle 21 together make a straight line. The angle of straight line is 180^o. Thus, the value of angle 21 can be calculated as

  $\begin{array}{l}\angle 21+\angle 25=180°\\ \angle 21+102°=180°\\ \angle 21=180°-102°\\ \angle 21=78°\end{array}$

The angle 21 and 22 are corresponding angles, thus, the value of angle 22 is

  $\angle 22=\angle 21=78°$

The angles 22 and 19 are interior alternate angles for the parallel lines AB and CD. The alternate angles are equal.

  $\angle 19=\angle 22=78°$

The value of angle 14 can be calculated by subtracting the value of angle 24 from the angle 15.

  $\begin{array}{l}\angle 14=\angle 15-\angle 24\\ \angle 14=83°-34°\\ \angle 14=49°\end{array}$

The lines AB, KH and IJ make a triangle. The sum of internal angles of a triangle is 180^o.

  $\begin{array}{l}\angle 14+\angle 22+\angle 18=180°\\ 49°+78°+\angle 18=180°\\ \angle 18=53°\end{array}$

Now,

  $\begin{array}{l}\angle 21+53°+\angle 20=180°\\ 78°+53°+\angle 20=180°\\ \angle 20=49°\end{array}$

Angle 20 and angle 17 are opposite angles.

  $\angle 17=\angle 20=49°$

To determine

(b)

The values of angles $\angle 1\text{ through }\angle 22$.

Answer to Problem 16A

The values of angles are

  $\begin{array}{l}\angle 1=112°23\text{'}\\ \angle 2=112°23\text{'}\\ \angle 3=67°37\text{'}\\ \angle 4=67°37\text{'}\\ \angle 5=67°37\text{'}\\ \angle 6=112°23\text{'}\\ \angle 7=67°37\text{'}\\ \angle 8=112°23\text{'}\\ \angle 9=67°37\text{'}\\ \angle 10=112°23\text{'}\end{array}$.

  $\begin{array}{l}\angle 11=67°37\text{'}\\ \angle 12=112°23\text{'}\\ \angle 13=112°23\text{'}\\ \angle 14=39°44\text{'}\\ \angle 15=67°37\text{'}\\ \angle 16=67°37\text{'}\\ \angle 17=39°44\text{'}\\ \angle 18=55°34\text{'}\\ \angle 19=84°42\text{'}\\ \angle 20=39°44\text{'}\\ \angle 21=84°42\text{'}\\ \angle 22=84°42\text{'}\end{array}$

Explanation of Solution

Given:

The given value of angles is

  $\begin{array}{l}\angle 23=112°23\text{'}\\ \angle 24=27°53\text{'}\\ \angle 25=95°18\text{'}\end{array}$.

The following figure is given

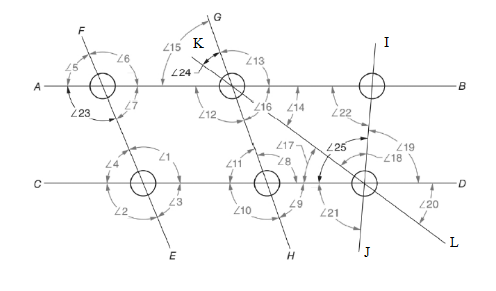
The angles $\angle 23\text{and }\angle 6$ are opposite angles. The opposite angles are equal, so, the angle $\angle 6\text{=}112°23\text{'}$

Now, angle 6 and angle 5 together make a straight line. The angle of straight line is 180^o. Thus, the value of angle 5 can be calculated as

  $\begin{array}{l}\angle 6+\angle 5=180°\\ 112°23\text{'}+\angle 5=180°\\ \angle 5=180°-112°23\text{'}\\ \angle 5=67°37\text{'}\end{array}$

The angles $\angle 7\text{and }\angle 5$ are opposite angles. The opposite angles are equal, so, the angle $\angle 7\text{=}67°37\text{'}$

The two lines AB and CD are parallel; hence the corresponding angles will be equal. Hence,

  $\begin{array}{l}\angle 5=\angle 4=67°37\text{'}\\ \angle 6=\angle 1=112°23\text{'}\\ \angle 23=\angle 2=112°23\text{'}\\ \angle 7=\angle 3=67°37\text{'}\end{array}$

The two lines EF and GH are also parallel; hence the corresponding angles will be equal. Hence,

  $\begin{array}{l}\angle 8=\angle 1=112°23\text{'}\\ \angle 9=\angle 3=67°37\text{'}\\ \angle 10=\angle 2=112°23\text{'}\\ \angle 11=\angle 4=67°37\text{'}\\ \end{array}$

The two lines AB and CD are parallel; hence the corresponding angles will be equal. Hence,

  $\begin{array}{l}\angle 8=\angle 13=112°23\text{'}\\ \angle 9=\angle 16=67°37\text{'}\\ \angle 10=\angle 12=112°23\text{'}\\ \angle 11=\angle 15=67°37\text{'}\end{array}$

Now, angle 25 and angle 21 together make a straight line. The angle of straight line is 180^o. Thus, the value of angle 21 can be calculated as

  $\begin{array}{l}\angle 21+\angle 25=180°\\ \angle 21+95°18\text{'}=180°\\ \angle 21=180°-95°18\text{'}\\ \angle 21=84°42\text{'}\end{array}$

The angle 21 and 22 are corresponding angles, thus, the value of angle 22 is

  $\angle 22=\angle 21=84°42\text{'}$

The angles 22 and 19 are interior alternate angles for the parallel lines AB and CD. The alternate angles are equal.

  $\angle 19=\angle 22=84°42\text{'}$

The value of angle 14 can be calculated by subtracting the value of angle 24 from the angle 15.

  $\begin{array}{l}\angle 14=\angle 15-\angle 24\\ \angle 14=67°37\text{'}-27°53\text{'}\\ \angle 14=39°44\text{'}\end{array}$

The lines AB, KH and IJ make a triangle. The sum of internal angles of a triangle is 180^o.

  $\begin{array}{l}\angle 14+\angle 22+\angle 18=180°\\ 39°44\text{'}+84°42\text{'}+\angle 18=180°\\ \angle 18=55°34\text{'}\end{array}$

Now,

  $\begin{array}{l}\angle 21+55°34\text{'}+\angle 20=180°\\ 84°42\text{'}+55°34\text{'}+\angle 20=180°\\ \angle 20=39°44\text{'}\end{array}$

Angle 20 and angle 17 are opposite angles.

  $\angle 17=\angle 20=39°44\text{'}$