
Concept explainers
Consider the three connected objects shown in Figure P5.88. Assume first that the inclined plane is friction-less and that the system is in equilibrium. In terms of m, g, and θ, find (a) the mass M and (b) the tensions T, and T2. Now assume that the value of Af is double the value found in part (a). Find (c) the acceleration of each object and (d) the tensions T1 and T2. Next, assume that the coefficient of static friction between m and 2m and the inclined plane is m, and that the system is in equilibrium. Find (e) the maximum value of M and (0 the minimum value of M. (g) Compare the values of T2 when M has its minimum and maximum values.
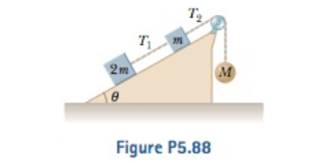
(a)
The expression for the mass of
Answer to Problem 5.88AP
The expression for the mass
Explanation of Solution
The free body diagram of the three connected objects is shown in Figure below,
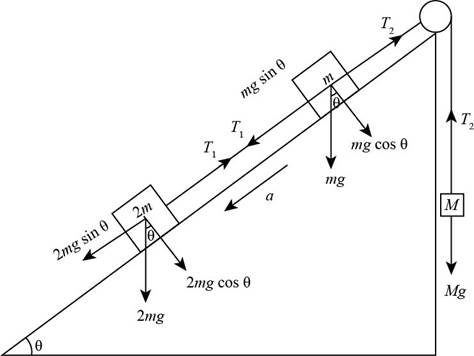
Figure (1)
From Figure (1), the equilibrium forces acts on the object of mass
Here,
From the Newton’s second law of motion, the net force on the object of mass
Here,
Substitute
From Figure (1), the equilibrium forces act on the object of mass
Here,
From the Newton’s second law of motion, the net force on the object of mass
Substitute
Add the equation (1) with equation (2).
From Figure (1), the equilibrium forces act on the object of mass
Here,
Substitute
From the Newton’s second law of motion, the net force on the object of mass
Substitute
The system is in equilibrium so value of the acceleration is zero.
Substitute
Conclusion:
Therefore, the expression for the mass
(b)
The expressions for tensions
Answer to Problem 5.88AP
The expression for the tension
Explanation of Solution
From part (a), the expression for the mass of
From part (a), the equilibrium forces act on the object is,
The system is in equilibrium, the value of acceleration is zero so the net force acts on the system is also zero.
Substitute
Substitute
Thus, the expression for the tension
From part (a), the equation (2) is,
Substitute
Substitute
Thus, the expression for the tension
Conclusion:
Therefore, the expression for the tension
(c)
The acceleration of each object.
Answer to Problem 5.88AP
The acceleration of each object is
Explanation of Solution
Given info: The value of mass
From part (a), the expression for the mass when it is double represents as,
From part (a), the equation (1) is,
Rearrange the above equation.
From part (a), the equation (2) is,
Rearrange the above equation.
Substitute
From Figure (1), the equilibrium forces act on the object of mass
Substitute
Subtract the equation (3) from equation (4).
Conclusion:
Therefore, the acceleration of each object is
(d)
The expressions for tensions
Answer to Problem 5.88AP
The expression for the tension
Explanation of Solution
From part (c), the expression for the acceleration is,
From part (c), the equation for tension
Substitute
Thus, the expression for tension
From part (c), the equation (3) is,
Substitute
Thus, the expression for tension
Conclusion:
Therefore, the expression for the tension
(e)
The maximum value of
Answer to Problem 5.88AP
The maximum value of
Explanation of Solution
Given info: The coefficient of static friction between mass
The static friction forces on the masses
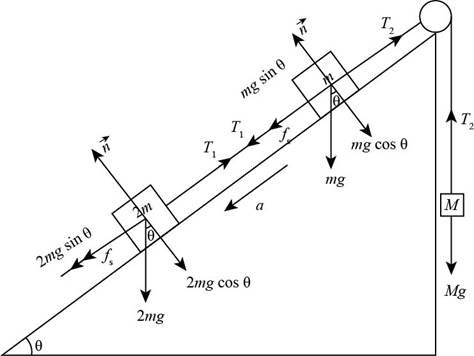
Figure (2)
From the Figure (2), the normal force on the object of mass
The expression for the static friction force on the object of mass
Here,
Substitute
From the Figure (1), the equilibrium forces acts on the object of mass
Substitute
Rearrange the above equation for
Substitute
From the Figure (2), the normal force on the object of mass
Substitute
From the Figure (1), the equilibrium forces acts on the object of mass
Substitute
Add equation (4) with the above equation.
Substitute
The equilibrium forces on the block of mass
Substitute
Conclusion:
Therefore, the maximum value of
(f)
The minimum value of
Answer to Problem 5.88AP
The minimum value of
Explanation of Solution
From part (e), the expression of tension
The equilibrium forces acts on block for minimum mass of
Substitute
Conclusion:
Therefore, the minimum value of
(g)
The difference between the tension
Answer to Problem 5.88AP
The difference between the tension for maximum and minimum mass is
Explanation of Solution
From part (e), the expression for maximum mass is,
From part (f), the expression for the minimum mass is,
From part (e), the expression for the tension for maximum mass is,
From part (f), the expression for the tension for maximum mass is,
Compare both the above equation.
Substitute
Conclusion:
Therefore, the difference between the tension for maximum and minimum mass is
Want to see more full solutions like this?
Chapter 5 Solutions
PHYSICS 1250 PACKAGE >CI<
- The 10-lb weight is supported by the cord AC and roller and by the spring that has a stiffness of k = 10 lb/in. and an unstretched length of 12 in. as shown in. Part A Determine the distance d to maintain equilibrium. Express your answer in inches to three significant figures. 節 ΕΠΙ ΑΣΦ d = *k J vec 5 t 0 ? d C A in. 12 in. Barrow_forwardThe members of a truss are connected to the gusset plate as shown in . The forces are concurrent at point O. Take = 90° and T₁ = 7.5 kN. Part A Determine the magnitude of F for equilibrium. Express your answer to three significant figures and include the appropriate units. F = Value Submit Request Answer Part B 0 ? Units Determine the magnitude of T2 for equilibrium. Express your answer to three significant figures and include the appropriate units. ? T₂ = Value Units T₁ Carrow_forwardpls help on botharrow_forward
- pls helparrow_forwardpls helparrow_forward6. 6. There are 1000 turns on the primary side of a transformer and 200 turns on thesecondary side. If 440 V are supplied to the primary winding, what is the voltageinduced in the secondary winding? Is this a step-up or step-down transformer? 7. 80 V are supplied to the primary winding of a transformer that has 50 turns. If thesecondary side has 50,000 turns, what is the voltage induced on the secondary side?Is this a step-up or step-down transformer? 8. There are 50 turns on the primary side of a transformer and 500 turns on thesecondary side. The current through the primary winding is 6 A. What is the turnsratio of this transformer? What is the current, in milliamps, through the secondarywinding?9. The current through the primary winding on a transformer is 5 A. There are 1000turns on the primary winding and 20 turns on the secondary winding. What is theturns ratio of this transformer? What is the current, in amps, through the secondarywinding?arrow_forward
- No chatgpt plsarrow_forwardWhat is the current, in amps, across a conductor that has a resistance of10 Ω and a voltage of 20 V? 2. A conductor draws a current of 100 A and a resistance of 5 Ω. What is thevoltageacross the conductor? 3. What is the resistance, in ohm’s, of a conductor that has a voltage of 80 kVand acurrent of 200 mA? 4. An x-ray imaging system that draws a current of 90 A is supplied with 220V. What is the power consumed? 5. An x-ray is produced using 800 mA and 100 kV. What is the powerconsumed in kilowatts?arrow_forwardՍՈՈՒ XVirginia Western Community Coll x P Course Home X + astering.pearson.com/?courseld=13289599#/ Figure y (mm) x=0x = 0.0900 m All ✓ Correct For either the time for one full cycle is 0.040 s; this is the period. Part C - ON You are told that the two points x = 0 and x = 0.0900 m are within one wavelength of each other. If the wave is moving in the +x-direction, determine the wavelength. Express your answer to two significant figures and include the appropriate units. 0 t(s) λ = Value m 0.01 0.03 0.05 0.07 Copyright © 2025 Pearson Education Inc. All rights reserved. 日 F3 F4 F5 1775 % F6 F7 B F8 Submit Previous Answers Request Answer ? × Incorrect; Try Again; 3 attempts remaining | Terms of Use | Privacy Policy | Permissions | Contact Us | Cookie Settings 28°F Clear 4 9:23 PM 1/20/2025 F9 prt sc F10 home F11 end F12 insert delete 6 7 29 & * ( 8 9 0 t = back Οarrow_forward
- Part C Find the height yi from which the rock was launched. Express your answer in meters to three significant figures. Learning Goal: To practice Problem-Solving Strategy 4.1 for projectile motion problems. A rock thrown with speed 12.0 m/s and launch angle 30.0 ∘ (above the horizontal) travels a horizontal distance of d = 19.0 m before hitting the ground. From what height was the rock thrown? Use the value g = 9.800 m/s2 for the free-fall acceleration. PROBLEM-SOLVING STRATEGY 4.1 Projectile motion problems MODEL: Is it reasonable to ignore air resistance? If so, use the projectile motion model. VISUALIZE: Establish a coordinate system with the x-axis horizontal and the y-axis vertical. Define symbols and identify what the problem is trying to find. For a launch at angle θ, the initial velocity components are vix=v0cosθ and viy=v0sinθ. SOLVE: The acceleration is known: ax=0 and ay=−g. Thus, the problem becomes one of…arrow_forwardPhys 25arrow_forwardPhys 22arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning
Classical Dynamics of Particles and SystemsPhysicsISBN:9780534408961Author:Stephen T. Thornton, Jerry B. MarionPublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





