Chapter 5, Problem 5.79AP

Two blocks of masses m₁ and m₂, are placed on a table in contact with each other as discussed in Example 5.7 and shown in Figure 5.13a. The coefficient of kinetic friction between the block of mass m₁ and the table is μ₁, and that between the block of mass m₂ and the table is μ₂. A horizontal force of magnitude F is applied to the block of mass m₁. We wish to find P, the magnitude of the contact force between the blocks. (a) Draw diagrams showing the forces for each block. (b) What is the net force on the system of two blocks? (c) What is the net force acting on m₁? (d) What is the net force acting on m₂? (e) Write Newton’s second law in the x direction for each block. (f) Solve the two equations in two unknowns for the acceleration a of the blocks in terms of the masses, the applied force F, the coefficients of friction, and g. (g) Find the magnitude P of the contact force between the blocks in terms of the same quantities.

To determine

The free body diagram of each block with forces.

The free body diagram of an object represents the direction and magnitude of forces acting on the body.

Explanation of Solution

The mass of block $1$ is $m_1$, the mass of block $2$ is $m_2$, the coefficient of kinetic friction between $m_1$ and the table is ${\mu }_1$, the coefficient of kinetic friction between $m_2$ and the table is ${\mu }_2$ and the magnitude of horizontal force is $F$.

The free body diagram of the book is given below.

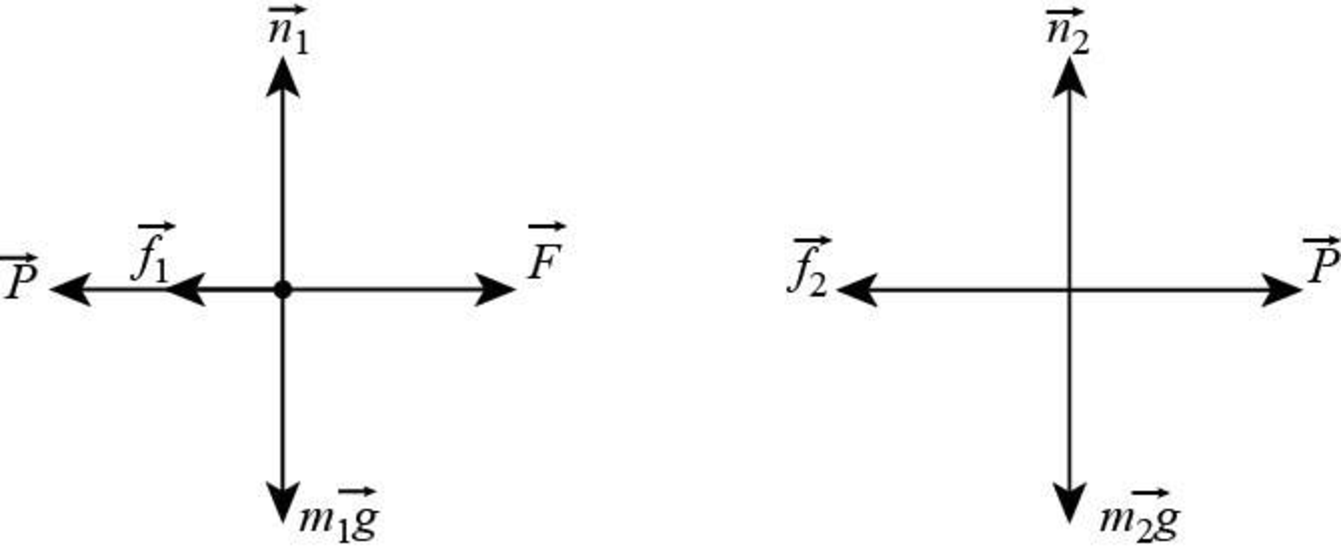
Figure (1)

The sum of all vertical forces is zero because the block moves on a horizontal surface. So the vertical acceleration, $a_{\text{y}}=0$.

Write the net force in the y-direction for mass $m_1$ using Newton’s law

    $\begin{array}{c}{\displaystyle \sum F_{\text{1y}}}=m_1{a}_{\text{y}}\\ -m_{1}g+n_1=0\\ n_1=m_{1}g\end{array}$

Here, $F_{\text{1y}}$ is the vertical force of block $1$, $a_{\text{y}}$ is the acceleration in the y-direction, $n_1$ is the normal force of block $1$ and $g$ is the acceleration due to gravity.

Write the net force in the y-direction for mass $m_2$ using Newton’s law

    $\begin{array}{c}{\displaystyle \sum F_{\text{2y}}}=m_2{a}_{\text{y}}\\ -m_{2}g+n_2=0\\ n_2=m_{2}g\end{array}$

Here, $F_{\text{2y}}$ is the vertical force of block $2$ and $n_2$ is the normal force of block $2$.

Write the equation for kinetic friction for block $1$

    $\begin{array}{c}{f}_1={\mu }_1{n}_1\\ ={\mu }_1{m}_{1}g\end{array}$

Here, $f_1$ is the frictional force due to mass $m_1$.

Write the equation for kinetic friction for block $2$

    $\begin{array}{c}{f}_2={\mu }_2{n}_2\\ ={\mu }_2{m}_{2}g\end{array}$

Here, $f_2$ is the frictional force due to mass $m_2$.

In the figure, $\overrightarrow{P}$ is the contact force that arises due to the contact between $m_1$ and $m_2$.

Conclusion:

Therefore, the free body diagram of each block to show the forces is given in figure I.

To determine

The net force on the system of two blocks.

Answer to Problem 5.79AP

The net force on the system of two blocks is the external force applied minus the frictional force.

Explanation of Solution

Write the expression for the net force in x-direction for the system of two blocks from the figure I,

  $\begin{array}{c}{\displaystyle \sum F}=F-f_1-f_2+P-P\\ =F-f_1-f_2\end{array}$

Here, ${\displaystyle \sum F}$ is the net force on the system of two blocks.

Conclusion:

Therefore, the net force on the system of two blocks is the external force applied minus the frictional force.

To determine

The net force acting on $m_1$.

Answer to Problem 5.79AP

The net force acting on $m_1$ is $F-f_1-P$.

Explanation of Solution

Write the expression for the net force in x-direction for the system of two blocks from the figure I,

  ${\displaystyle \sum F_{\text{1x}}}=F-f_1-P$

Here, ${\displaystyle \sum F_{\text{1x}}}$ is the net force on the block of mass $m_1$ .

Conclusion:

Therefore, the net force acting on $m_1$ is $F-f_1-P$.

To determine

The net force acting on $m_2$.

Answer to Problem 5.79AP

The net force acting on $m_2$ is $P-f_2$.

Explanation of Solution

Write the expression for the net force in x-direction for the system of two blocks from the figure I,

  $\begin{array}{c}{\displaystyle \sum F_{\text{2x}}}=-f_2+P\\ =P-f_2\end{array}$

Here, ${\displaystyle \sum F_{\text{2x}}}$ is the net force on the block of mass $m_2$ .

Conclusion:

Therefore, the net force acting on $m_2$ is $P-f_2$.

To determine

The Newton’s second law in the $x$ direction for each block.

Answer to Problem 5.79AP

The Newton’s second law in the $x$ direction, for $m_1$ is $F-P=m_{1}a$ and for $m_2$ is $P=m_{2}a$.

Explanation of Solution

The block has on a horizontal acceleration $a_{\text{x}}=a$.

Write the Newton’s second law for block $1$

  ${\displaystyle \sum F_{\text{1x}}}=m_1{a}_{\text{x}}$

Substitute $F-f_1-P$ for ${\displaystyle \sum F_{1\text{x}}}$ in the above equation

  $F-f_1-P=m_{1}a$

Substitute ${\mu }_1{m}_{1}g$ for $f_1$ in the above equation

    $F-{\mu }_1{m}_{1}g-P=m_{1}a$

Write the Newton’s second law for block $2$

  ${\displaystyle \sum F_{\text{2x}}}=m_2{a}_{\text{x}}$

Substitute $P-f_2$ for ${\displaystyle \sum F_{2\text{x}}}$ in the above equation

  $P-f_2=m_{2}a$

Substitute ${\mu }_2{m}_{2}g$ for $f_2$ in the above equation

    $P-{\mu }_2{m}_{2}g=m_{2}a$

Conclusion:

Therefore, the Newton’s second law in the $x$ direction, for $m_1$ is $F-{\mu }_1{m}_{1}g-P=m_{1}a$ and for $m_2$ is $P-{\mu }_2{m}_{2}g=m_{2}a$.

To determine

The acceleration of the blocks.

Answer to Problem 5.79AP

The acceleration of the blocks is $\left(\frac{F-{\mu }_1{m}_{1}g-{\mu }_2{m}_{2}g}{m_1+m_2}\right)$.

Explanation of Solution

Write the Newton’s second law is for block $1$

    $F-P-{\mu }_1{m}_{1}g=m_{1}a$                                                              (I)

Write the Newton’s second law is for block $2$

    $P-{\mu }_2{m}_{2}g=m_{2}a$                                                                 (II)

Conclusion:

Add the equation (I) and equation (II) and solve for $a$.

    $\begin{array}{c}F-P-{\mu }_1{m}_{1}g+P-{\mu }_2{m}_{2}g=m_{1}a+m_{2}a\\ a=\left(\frac{F-{\mu }_1{m}_{1}g-{\mu }_2{m}_{2}g}{m_1+m_2}\right)\end{array}$

Therefore, the acceleration of the blocks is $\left(\frac{F-{\mu }_1{m}_{1}g-{\mu }_2{m}_{2}g}{m_1+m_2}\right)$.

To determine

The magnitude of the contact force between the blocks in terms of acceleration, mass, applied force and the friction coefficient.

Answer to Problem 5.79AP

The magnitude $P$ of the contact force between the blocks is $\left(\frac{m_2}{m_1+m_2}\right)\left(F+\left({\mu }_2-{\mu }_1\right)m_{1}g\right)$.

Explanation of Solution

Recall the equation (II).

    $\begin{array}{c}P-{\mu }_2{m}_{2}g=m_{2}a\\ P={\mu }_2{m}_{2}g+m_{2}a\end{array}$

Substitute $\left(\frac{F-{\mu }_1{m}_{1}g-{\mu }_2{m}_{2}g}{m_1+m_2}\right)$ for $a$ in above expression.

    $\begin{array}{c}P={\mu }_2{m}_{2}g+m_2\left(\frac{F-{\mu }_1{m}_{1}g-{\mu }_2{m}_{2}g}{m_1+m_2}\right)\\ =\left(\frac{m_2}{m_1+m_2}\right)\left(F+\left({\mu }_2-{\mu }_1\right)m_{1}g\right)\end{array}$

Conclusion:

Therefore, the magnitude $P$ of the contact force between the blocks is $\left(\frac{m_2}{m_1+m_2}\right)\left(F+\left({\mu }_2-{\mu }_1\right)m_{1}g\right)$.