Chapter 5, Problem 5.24P

To determine

(a)

The price of y is $Py=1$ and price of x is P, then Optimal baskets of consumers to be determined.

Answer to Problem 5.24P

TheJ's optimal basket is 50 units of y and $\frac{50}{P}$ units of x.

The D's optimal basket is 50 units of y and $\frac{100}{P}$ units of x.

Explanation of Solution

Budget constraint/line shows all possible combinations of two goods that can be purchased, given the level of income of the consumer and the market prices of both goods.

Indifference curve (IC) shows all possible combination of two goods that gives the same level of satisfaction to the consumer.

At the point of tangency of the budget line and the indifference curve, the consumption bundle is optimal. The slope of the IC and the budget line are equal at this point.

There are two consumers J and D and the market for goods x and y.

The utility function of J is $U=xy$

The marginal utility $MUx=yandMUy=x$ and J has an income of 100.

The utility function of D is $U=x^{2}y$

The marginal utilities $MUx=2xy\text{ and }MUy=x^2$

D has an income of 150.

The price of good y is $Py=1$.

Find Optimal basket of J when $Py=1$, and the price of x is $Px=P$ by adding the solution to the equation,

  $\frac{MUx}{MUy}=\frac{Px}{Py}andx.P_x+y.P_y=100$

  $\begin{array}{l}\frac{MUx}{MUy}=\frac{Px}{Py}\\ \frac{y}{x}=\frac{p}{1}\\ y=xp\end{array}$

Find y by substituting $y=Px$ into $x.P_x+y.P_y=100$

  $\begin{array}{l}x.P_x+y.P_y=100\\ x{P}_x+y\left(1\right)=100\\ xP+xP=100\\ 2xP=100\\ x=\frac{50}{P}\end{array}$

Also,

  $\begin{array}{l}y=xP\\ y=\left\{\frac{50}{p}\right\}p\\ y=50\end{array}$

Hence J's optimal basket is 50 units of y and $\frac{50}{P}$ units of x.

Find the optimal basket od D when the price of y is $Py=1$, and the price of x is $Px=P$ by adding the solution to the equation,

  $\frac{MUx}{MUy}=\frac{Px}{Py}andx.P_x+y.P_y=150$

  $\begin{array}{l}\frac{MUx}{MUy}=\frac{Px}{Py}\\ \frac{2xy}{x^2}=\frac{p}{1}\\ \frac{2y}{x}=p\\ y=\frac{xp}{2}\end{array}$

Find y by substituting $y=Px$ into $x.P_x+y.P_y=150$

  $\begin{array}{l}x.P_x+y.P_y=150\\ x{P}_x+y\left(1\right)=150\\ xP+\frac{xP}{2}=150\\ 3xP=300\\ x=\frac{100}{P}\end{array}$

Also,

  $\begin{array}{l}y=\frac{xP}{2}\\ y=\frac{\left\{\frac{100}{p}\right\}p}{2}\\ y=50\end{array}$

Hence D's optimal basket is 50 units of y and $\frac{100}{P}$ units of x.

To determine

(b)

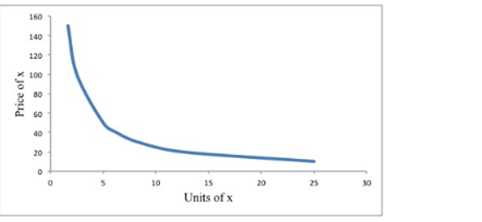
For all values of P, Separate graph for consumers demand schedule x is to be plotted.

Explanation of Solution

This the J's demand for x for all values of P:

This is the D's demand for x for all values of P:

To determine

(c)

The given consumers are only consumers then aggregate demand is to be computedand plotted.

Answer to Problem 5.24P

Graph for the aggregate demand curve for x, for $D=\frac{150}{P}$

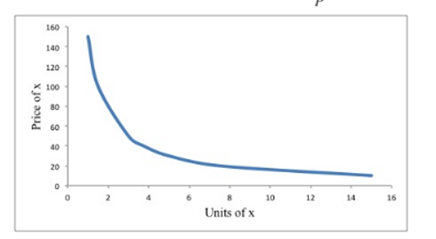
Explanation of Solution

To compute the demand D When J and D are the only consumers by adding consumer's demand for x.

  $\begin{array}{l}Q=\frac{50}{P}+\frac{100}{P}\\ =\frac{150}{P}\end{array}$

Graph for the aggregate demand curve for x, Where $D=\frac{150}{P}$

To determine

(d)

There is one more consumer that has the same utility function and income as consumer 2 then aggregate demand is to be plotted.

Explanation of Solution

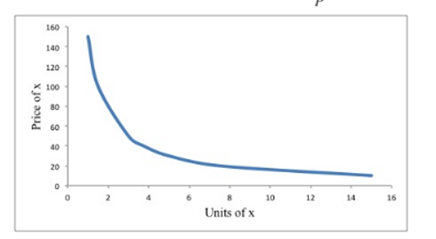
Compute aggregate demand when J, D and another consumer with the same utility function and income as D are the only consumers.

Calculate aggregate demand by adding each consumer's demand x.

  $\begin{array}{l}Q=\frac{50}{P}+2\left(\frac{100}{P}\right)\\ =\frac{250}{P}\end{array}$

Graph the aggregate demand curve for x where $D=\frac{250}{P}$