Chapter 5, Problem 5.18P

To determine

Show that when the mass moves to a position $\left(x,y\right)$ the potential energy is in the form of $U=\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)$ for an anisotropic oscillator. And also show that if $a<l_0$ the equilibrium at the origin is unstable.

Answer to Problem 5.18P

Therefore, it is proved that when the mass moves to a position $\left(x,y\right)$ the potential energy is in the form of $U=\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)$ for an anisotropic oscillator. . And it is true that  if $a<l_0$, the equilibrium at the origin is unstable.

Explanation of Solution

Write the expression for the potential energy of the spring

    $U=\frac{1}{2}k{x}^2$        (I)

Here, $U$ is the potential energy, $k$ is the spring constant and $x$ is the stretched or compressed length of the spring.

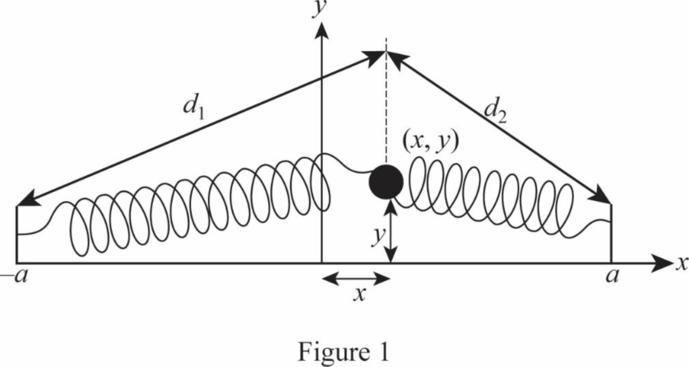
The above figure represents the given situation.

From figure, the spring on the left hand side is stretched and the spring on the right hand side is compressed.

Spring on the left hand side [stretched]:

The total length of the spring,

$d_1=\sqrt{{\left(a+x\right)}^2+y^2}$

The stretched length of the spring from its equilibrium length $l_0$ is

$\begin{array}{c}\Delta d_1=d_1-l_0\\ =\sqrt{{\left(a+x\right)}^2+y^2}-l_0\end{array}$

Spring on the right hand side [compressed]:

The total length of the spring,

$d_2=\sqrt{{\left(a-x\right)}^2+y^2}$

The compressed length of the spring from its equilibrium length $l_0$ is

$\begin{array}{c}\Delta d_2=d_2-l_0\\ =\sqrt{{\left(a-x\right)}^2+y^2}-l_0\end{array}$

The potential energy stored in the stretched spring on left is

  $U_1=\frac{1}{2}k{\left(\Delta d_1\right)}^2$

Substitute $\sqrt{{\left(a+x\right)}^2+y^2}-l_0$ for $\Delta d_1$ in the above equation to solve for $U_1$

$U_1=\frac{1}{2}k{\left(\sqrt{{\left(a+x\right)}^2+y^2}-l_0\right)}^2$        (II)

The potential energy stored in the compressed spring on right is

  $U_2=\frac{1}{2}k{\left(\Delta d_2\right)}^2$

Substitute $\sqrt{{\left(a-x\right)}^2+y^2}-l_0$ for $\Delta d_2$ in the above equation to solve for $U_2$

$U_2=\frac{1}{2}k{\left(\sqrt{{\left(a-x\right)}^2+y^2}-l_0\right)}^2$        (III)

The total potential energy of the system,

  $U\left(x,y\right)=U_1+U_2$

Substitute equation (II) and (III) in the above equation to solve for $U$

$\begin{array}{c}U\left(x,y\right)=\frac{1}{2}k{\left(\sqrt{{\left(a+x\right)}^2+y^2}-l_0\right)}^2+\frac{1}{2}k{\left(\sqrt{{\left(a-x\right)}^2+y^2}-l_0\right)}^2\\ =\frac{1}{2}k{\left(\sqrt{{\left[a\left(1+\frac{x}{a}\right)\right]}^2+y^2}-l_0\right)}^2+\frac{1}{2}k{\left(\sqrt{{\left[a\left(1-\frac{x}{a}\right)\right]}^2+y^2}-l_0\right)}^2\\ =\frac{1}{2}k{\left(\sqrt{a^2{\left(1+\frac{x}{a}\right)}^2+y^2}-l_0\right)}^2+\frac{1}{2}k{\left(\sqrt{a^2{\left(1-\frac{x}{a}\right)}^2+y^2}-l_0\right)}^2\\ =\frac{1}{2}k{a}^2{\left(\sqrt{{\left(1+\frac{x}{a}\right)}^2+\frac{y^2}{a^2}}-\frac{l_0}{a^2}\right)}^2+\frac{1}{2}k{a}^2{\left(\sqrt{{\left(1-\frac{x}{a}\right)}^2+\frac{y^2}{a^2}}-\frac{l_0}{a^2}\right)}^2\end{array}$

$\begin{array}{c}=\frac{1}{2}k{a}^2{\left(\sqrt{1+\frac{x^2}{a^2}+\frac{2x}{a}+\frac{y^2}{a^2}}-\frac{l_0}{a}\right)}^2+\frac{1}{2}k{a}^2{\left(\sqrt{1+\frac{x^2}{a^2}-\frac{2x}{a}+\frac{y^2}{a^2}}-\frac{l_0}{a}\right)}^2\\ U\left(x,y\right)=\frac{1}{2}k{a}^2{\left({\left(1+\left(\frac{x^2}{a^2}+\frac{2x}{a}+\frac{y^2}{a^2}\right)\right)}^{\frac{1}{2}}-\frac{l_0}{a}\right)}^2+\frac{1}{2}k{a}^2{\left({\left(1+\left(-\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)\right)}^{\frac{1}{2}}-\frac{l_0}{a}\right)}^2\end{array}$        (IV)

Write the Taylor expansion for ${\left(1+\delta \right)}^2$

    ${\left(1+\delta \right)}^2=1+\frac{1}{2}\delta -\frac{1}{8}{\delta }^2+\mathrm{.....},\left|\delta \right|<1$

Applying Taylor expansion for the terms $1+\left(\frac{x^2}{a^2}+\frac{2x}{a}+\frac{y^2}{a^2}\right)$ and $1+\left(-\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)$ in equation (IV)

$\begin{array}{l}U\left(x,y\right)=\frac{1}{2}k{a}^2{\left(\left(1+\frac{1}{2}\left(\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)-\frac{1}{8}{\left(\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)}^2\right)-\frac{l_0}{a}\right)}^2\\ +\frac{1}{2}k{a}^2{\left(\left(1+\frac{1}{2}\left(-\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)-\frac{1}{8}{\left(-\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)}^2\right)-\frac{l_0}{a}\right)}^2\end{array}$

Given that $x$ and $y$ are small. Thus, neglecting the higher order powers for $x$ and $y$ in the above equation

$\begin{array}{c}U\left(x,y\right)=\frac{1}{2}k{a}^2{\left(\left(1+\frac{1}{2}\left(\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)-\frac{1}{8}{\left(\frac{2x}{a}\right)}^2\right)-\frac{l_0}{a}\right)}^2\\ +\frac{1}{2}k{a}^2{\left(\left(1+\frac{1}{2}\left(-\frac{2x}{a}+\frac{x^2}{a^2}+\frac{y^2}{a^2}\right)-\frac{1}{8}{\left(-\frac{2x}{a}\right)}^2\right)-\frac{l_0}{a}\right)}^2\\ =\frac{1}{2}k{a}^2{\left(\left(1+\frac{1}{2}\left(\frac{2x}{a}+\frac{x^2}{a^2}\right)-\frac{1}{8}{\left(\frac{2x}{a}\right)}^2+\frac{1}{2}\frac{y^2}{a^2}\right)-\frac{l_0}{a}\right)}^2\\ +\frac{1}{2}k{a}^2{\left(\left(1+\frac{1}{2}\left(-\frac{2x}{a}+\frac{x^2}{a^2}\right)-\frac{1}{8}{\left(-\frac{2x}{a}\right)}^2+\frac{1}{2}\frac{y^2}{a^2}\right)-\frac{l_0}{a}\right)}^2\\ =\frac{1}{2}k{a}^2{\left(\left(1+\frac{x}{a}+\frac{x^2}{2a^2}-\frac{x^2}{2a^2}+\frac{1}{2}\frac{y^2}{a^2}\right)-\frac{l_0}{a}\right)}^2\\ +\frac{1}{2}k{a}^2{\left(\left(1-\frac{x}{a}+\frac{x^2}{2a^2}-\frac{x^2}{2a^2}+\frac{1}{2}\frac{y^2}{a^2}\right)-\frac{l_0}{a}\right)}^2\\ =\frac{1}{2}k{a}^2{\left(\left(1+\frac{x}{a}+\frac{1}{2}\frac{y^2}{a^2}\right)-\frac{l_0}{a}\right)}^2+\frac{1}{2}k{a}^2{\left(\left(1-\frac{x}{a}+\frac{1}{2}\frac{y^2}{a^2}\right)-\frac{l_0}{a}\right)}^2\end{array}$

Solving further,

$\begin{array}{c}U\left(x,y\right)=\frac{1}{2}k{a}^2{\left(\left(1-\frac{l_0}{a}+\frac{x}{a}\right)+\frac{y^2}{2a^2}\right)}^2+\frac{1}{2}k{a}^2{\left(\left(1-\frac{l_0}{a}-\frac{x}{a}\right)+\frac{y^2}{2a^2}\right)}^2\\ =\frac{1}{2}k{a}^2\left({\left(1-\frac{l_0}{a}+\frac{x}{a}\right)}^2+{\left(\frac{y^2}{2a^2}\right)}^2+2\left(1-\frac{l_0}{a}+\frac{x}{a}\right)\frac{y^2}{2a^2}\right)\\ +\frac{1}{2}k{a}^2\left({\left(1-\frac{l_0}{a}-\frac{x}{a}\right)}^2+{\left(\frac{y^2}{2a^2}\right)}^2+2\left(1-\frac{l_0}{a}-\frac{x}{a}\right)\frac{y^2}{2a^2}\right)\\ =\frac{1}{2}k{a}^2\left(\left({\left(1-\frac{l_0}{a}\right)}^2+\frac{x^2}{a^2}+2\left(1-\frac{l_0}{a}\right)\frac{x}{a}\right)+{\left(\frac{y^2}{2a^2}\right)}^2+2\left(1-\frac{l_0}{a}+\frac{x}{a}\right)\frac{y^2}{2a^2}\right)\\ +\frac{1}{2}k{a}^2\left(\left({\left(1-\frac{l_0}{a}\right)}^2+\frac{x^2}{a^2}-2\left(1-\frac{l_0}{a}\right)\frac{x}{a}\right)+{\left(\frac{y^2}{2a^2}\right)}^2+2\left(1-\frac{l_0}{a}-\frac{x}{a}\right)\frac{y^2}{2a^2}\right)\\ =\frac{1}{2}k{a}^2\left(2{\left(1-\frac{l_0}{a}\right)}^2+2\frac{x^2}{a^2}+2{\left(\frac{y^2}{2a^2}\right)}^2+4\left(1-\frac{l_0}{a}+\frac{x}{a}\right)\frac{y^2}{2a^2}\right)\end{array}$

Neglecting the higher power terms and solving

$\begin{array}{c}U\left(x,y\right)=\frac{1}{2}k{a}^2\left(2{\left(1-\frac{l_0}{a}\right)}^2+2\frac{x^2}{a^2}+4\left(1-\frac{l_0}{a}\right)\frac{y^2}{2a^2}\right)\\ =k{a}^2{\left(1-\frac{l_0}{a}\right)}^2+k{x}^2+k\left(1-\frac{l_0}{a}\right)y^2\\ =k{\left(a-l_0\right)}^2+k{x}^2+k\left(\frac{a-l_0}{a}\right)y^2\end{array}$        (V)

Here, the potential energy is shifted by the constant $k{\left(a-l_0\right)}^2$ which is the position of zero potential energy.

The potential energy of the system is

$\begin{array}{c}U\left(x,y\right)=k{x}^2+k\left(\frac{a-l_0}{a}\right)y^2\\ =\frac{1}{2}\left(2k\right)x^2+\frac{1}{2}\left[2k\left(\frac{a-l_0}{a}\right)\right]y^2\\ =\frac{1}{2}{k}_x{x}^2+\frac{1}{2}{k}_y{y}^2\end{array}$

In the above equation, $k_x=2k$ and $k_y=2k\left(\frac{a-l_0}{a}\right)$

Therefore, it is proved that when the mass moves to a position $\left(x,y\right)$ the potential energy is in the form of $U=\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)$ for an anisotropic oscillator.

When the mass is at the origin, $a=l_0$

Substitute $a$ for $l_0$ in $0$ for $x$ and $y$ in equation (V)

$\begin{array}{c}U\left(x,y\right)=k{a}^2{\left(1-\frac{l_0}{a}\right)}^2+k{x}^2+k\left(1-\frac{l_0}{a}\right)y^2\\ =k{a}^2{\left(1-\frac{a}{a}\right)}^2+k{\left(0\right)}^2+k\left(1-\frac{a}{a}\right)y^2\\ =k{a}^2{\left(0\right)}^2+0+k\left(0\right)y^2\\ =0\end{array}$

The potential energy of the two-spring-mass is zero at the origin. Thus, the system is in unstable equilibrium state at origin.

Write the Hessian $2\times 2$ matrix

    $H=\left|\begin{array}{cc}\frac{{\partial }^{2}U}{\partial x\partial x}& \frac{{\partial }^{2}U}{\partial x\partial y}\\ \frac{{\partial }^{2}U}{\partial y\partial x}& \frac{{\partial }^{2}U}{\partial y\partial y}\end{array}\right|$        (VI)

Solve for $\frac{{\partial }^{2}U}{\partial x\partial x}$

$\begin{array}{c}\frac{{\partial }^{2}U}{\partial x\partial x}=\frac{\partial }{\partial x}\left[\frac{\partial }{\partial x}\left(\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)\right)\right]\\ =\frac{\partial }{\partial x}\left[\frac{1}{2}\left(k_x\left(2x\right)+0\right)\right]\\ =\frac{\partial }{\partial x}\left[k_{x}x\right]\\ =k_x\end{array}$

Solve for $\frac{{\partial }^{2}U}{\partial y\partial y}$

$\begin{array}{c}\frac{{\partial }^{2}U}{\partial y\partial y}=\frac{\partial }{\partial y}\left[\frac{\partial }{\partial y}\left(\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)\right)\right]\\ =\frac{\partial }{\partial y}\left[\frac{1}{2}\left(0+k_y\left(2y\right)\right)\right]\\ =\frac{\partial }{\partial y}\left[k_{y}y\right]\\ =k_y\end{array}$

Solve for $\frac{{\partial }^{2}U}{\partial y\partial x}$

$\begin{array}{c}\frac{{\partial }^{2}U}{\partial y\partial x}=\frac{\partial }{\partial y}\left[\frac{\partial }{\partial x}\left(\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)\right)\right]\\ =\frac{\partial }{\partial y}\left[\frac{1}{2}\left(k_x\left(2x\right)+0\right)\right]\\ =\frac{\partial }{\partial y}\left[k_{x}x\right]\\ =0\end{array}$

Solve for $\frac{{\partial }^{2}U}{\partial x\partial y}$

$\begin{array}{c}\frac{{\partial }^{2}U}{\partial x\partial y}=\frac{\partial }{\partial x}\left[\frac{\partial }{\partial y}\left(\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)\right)\right]\\ =\frac{\partial }{\partial x}\left[\frac{1}{2}\left(0+k_y\left(2y\right)\right)\right]\\ =\frac{\partial }{\partial x}\left[k_{y}y\right]\\ =0\end{array}$

Substitute $k_x$ for $\frac{{\partial }^{2}U}{\partial x\partial x}$, $k_y$ for $\frac{{\partial }^{2}U}{\partial y\partial y}$ and $0$  for $\frac{{\partial }^{2}U}{\partial x\partial y}$ and $\frac{{\partial }^{2}U}{\partial y\partial x}$ in equation (VI) to solve for $H\left(0,0\right)$

$H\left(0,0\right)=\left|\begin{array}{cc}{k}_x& 0\\ 0& k_y\end{array}\right|$

Substitute $2k$ for $k_x$ and $2k\left(\frac{a-l_0}{a}\right)$ for $k_y$ in the above matrix

$H\left(0,0\right)=\left|\begin{array}{cc}2k& 0\\ 0& 2k\left(\frac{a-l_0}{a}\right)\end{array}\right|$

In the above matrix, when $a>l_0$ both the Eigen values will have a positive value. Therefore, the system will be in stable equilibrium at $\left(0,0\right)$. And when $a<l_0$ one of the Eigen value will have a negative sign. In this care both the springs compresses and a small pluck along $y$ direction makes an infinite push to the mass along this direction. Therefore, for $a<l_0$ the equilibrium at the origin is unstable.

Conclusion:

Therefore, it is proved that when the mass moves to a position $\left(x,y\right)$ the potential energy is in the form of $U=\frac{1}{2}\left(k_x{x}^2+k_y{y}^2\right)$ for an anisotropic oscillator. . And it is true that  if $a<l_0$, the equilibrium at the origin is unstable.