Chapter 5, Problem 36SP

An object is subjected to the forces shown in Fig. 5-25. What single force F applied at a point on the x-axis will balance these forces leaving the object motionless? (First find its components, and then find the force.) Where on the x-axis should the force be applied? Notice that before F is applied there is an unbalanced force with components to the left and upward.

Fig. 5-25

To determine

The magnitude of the applied force and direction of that force on the $x$-axisinFigure $5.25$ that will balance all other forces on the object leaving it motionless.

Answer to Problem 36SP

Solution:

$F_x=232\text{ N}$, $F_y=-338\text{ N}$, $F=400\text{ N}$ at $-55.5°$, $x=2.14\text{ m}$

Explanation of Solution

Given data:

Refer to theFigure $5.25$.

Formula used:

From the parallelogram law of vector addition, if the expression of resultant force is written as

$\overrightarrow{F}=\left(F_x\widehat{i}+F_y\widehat{j}\right)$

The magnitude of $\overrightarrow{F}$ is

$\overrightarrow{F}=\sqrt{{\left(F_x\right)}^2+{\left(F_y\right)}^2+2F_x{F}_y\cos \varphi }$

Here, $\varphi$ is the angle between both the vectors.

The direction of $\overrightarrow{F}$ is

$\theta ={\tan }^{-1}\left(\frac{F_y}{F_x}\right)$

Write the expression for the first condition of force equilibrium:

$\begin{array}{l}{\displaystyle \sum F_x}=0\\ {\displaystyle \sum F_y}=0\end{array}$

Here, ${\displaystyle \sum F_x}$ is the sum of the forces in the x- direction or the horizontal direction and ${\displaystyle \sum F_y}$ is the sum of the forces in the y-direction or the vertical direction.

Write the expression for torque:

$\tau =rF\sin \theta$

Here, $r$ is the distance of the applied force, $F$, from the axis of rotation and $\theta$ is the angle.

Explanation:

Draw the free body diagram of the system:

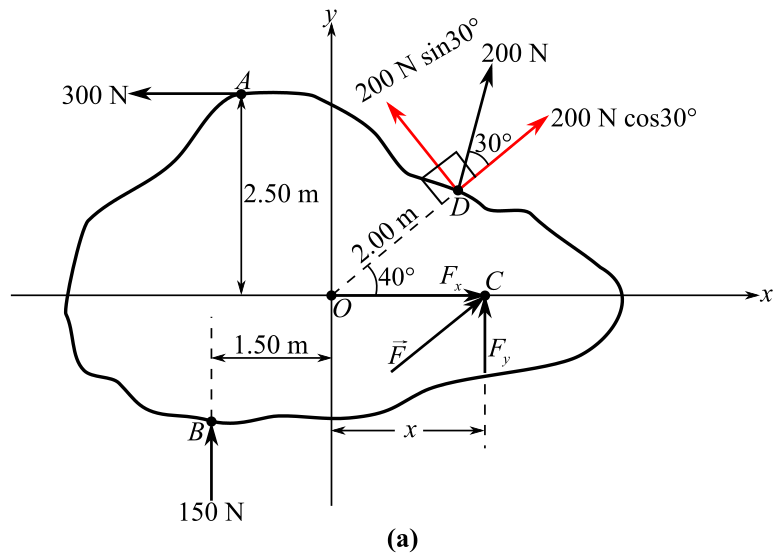
In the above diagram, $O$ is origin of the figure, $\overrightarrow{F}$ is applied on the x-axis at point $C$, and $\overrightarrow{F}$ has two components—$F_y$ is the vertical force atpoint $C$ and $F_x$ is the horizontal force at point $C$. At point $D$, $200\sin 30°$ and $200\cos 30°$ are the components of the $200\text{ N}$ force.

Refer the above figure, recall the expression of torque:

$\tau =rF\sin \theta$

Apply the equation for torque at point $O$ and considering the direction of counter-clockwise torque to be positive and that of clockwise torqueto be negative:

$\begin{array}{c}{\displaystyle \sum {\tau }_O}=0\\ \left(300\text{ N}\right)\left(2.5\text{ m}\right)\left(\sin 90°\right)+\left[\begin{array}{c}\left(\text{200sin30}°\right)\left(2\text{ m}\right)\left(\sin 90°\right)\\ -\left(\text{150 N}\right)\left(1.5\right)\left(\sin 90°\right)\end{array}\right]+F_y\left(x\right)=0\\ 750\text{ N}\cdot \text{m}+200\text{ N}\cdot \text{m}-225\text{ N}\cdot \text{m}+F_y\left(x\right)=0\end{array}$

Further solving the equation for $F_y\left(x\right)$, we get

$F_y\left(x\right)=-725\text{ N}\cdot \text{m}$ …… $\left(1\right)$

Draw the free body diagram of the system when resolving the force of $200\text{ N}$:

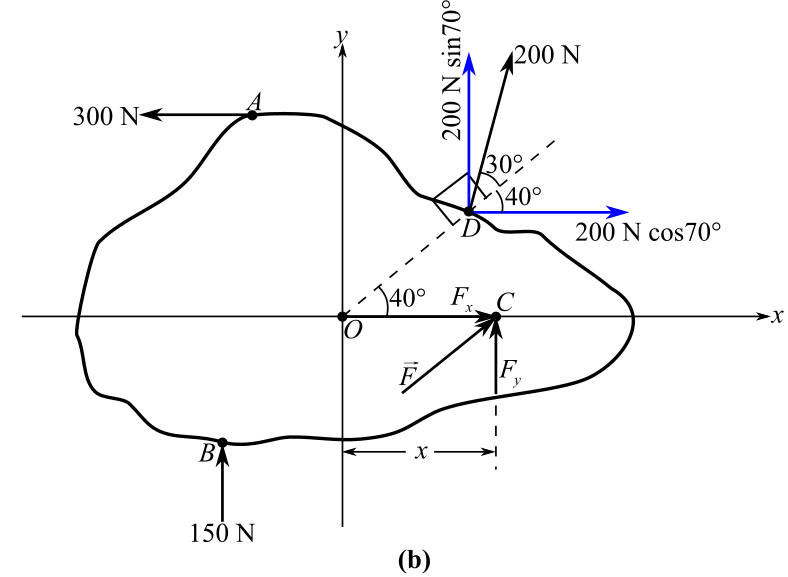
In the above diagram, $O$ is origin of the figure, $\overrightarrow{F}$ is applied on the x-axis at point $C$, and $\overrightarrow{F}$ has two components—$F_x$ is the horizontal force at point $C$ and $F_y$ is the vertical force atpoint $C$;$200\text{ N}$ acting at point $D$ has two force components—$200\text{ N}\sin 30°$ is the vertical force at point $D$ and $200\text{ Ncos}30°$ is the horizontal force at point $D$.

Consider theFigure (b)and recall the expression for the first condition of the force’s equilibriumin the x-direction:

${{\displaystyle \sum F}}_x=0$

Consider the direction of the rightward forces to be positive and the direction of the leftward forces to be negative. Therefore,

$\begin{array}{c}{F}_x+200\cos 70°-300\text{ N=0}\\ F_x=231.5\text{ N}\\ \simeq \text{232 N}\end{array}$

Rewrite the expression for the first condition of force equilibrium in the y-direction.

${{\displaystyle \sum F}}_y=0$

Consider the direction of the upward forces to be positive and the direction of the downward forces to be negative. Therefore,

$\begin{array}{c}{F}_y+150\text{ N}+200\sin 70°=0\\ F_y+338\text{ N}=\text{0}\\ F_y=-338\text{ N}\end{array}$

The negative sign shows the direction of the force is downward.

Recall the expression for the magnitude of the resultant force:

$\overrightarrow{F}=\sqrt{{\left(F_x\right)}^2+{\left(F_y\right)}^2+2F_x{F}_y\cos \varphi }$

Substitute $\text{232 N}$ for $F_x$, $-338\text{ N }$ for $F_y$, and $90°$ for $\varphi$

$\begin{array}{c}\overrightarrow{F}=\sqrt{{\left(232\text{ N}\right)}^2+{\left(-338\text{ N}\right)}^2+2\left(232\text{ N}\right)\left(-338\text{ N}\right)\cos 90°}\\ =\sqrt{{\left(232\text{ N}\right)}^2+{\left(-338\text{ N}\right)}^2}\\ =\sqrt{168,068\text{ N}}\\ =410\text{ N}\end{array}$

Recall the expression for the direction of the resultant force:

$\theta ={\tan }^{-1}\left(\frac{F_y}{F_x}\right)$

Substitute $\text{232 N}$ for $F_x$ and $-338\text{ N }$ for $F_y$

$\begin{array}{c}\theta ={\tan }^{-1}\left(\frac{-338\text{ N }}{\text{232 N}}\right)\\ ={\tan }^{-1}\left(-1.456\right)\\ =-55.51°\end{array}$

Rewrite equation(1)

$F_y\left(x\right)=725\text{ N}\cdot \text{m}$

Substitute $-338\text{ N}$ for $F_y$

$\begin{array}{c}\left(-338\text{ N}\right)\left(x\right)=725\text{ N}\cdot \text{m}\\ x=\frac{725\text{ m}}{-338}\\ =2.14\text{ m}\end{array}$

Conclusion:

The value of the horizontal force $F_x$ is $\text{0}\text{.64 kN}$, the vertical force $F_y$ is $-338\text{ N}$, the magnitude of the force $F$ is $400\text{ N}$, the direction of the force is $-55.5°$, and the distance of the force at the origin is $2.14\text{ m}$.