Single Variable Essential Calculus: Early Transcendentals
2nd Edition
ISBN: 9781133112785
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 4.6, Problem 2E
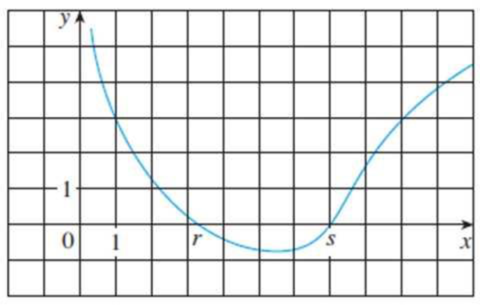
Follow the instructions for Exercise 1 (a) but use x1 = 9 as the starting approximation for finding the root s.
- 1. The figure shows the graph of a function f. Suppose that Newton’s method is used to approximate the root r of the equation f(x) = 0 with initial approximation x1 = 1.
- a. Draw the tangent lines that are used to find x2 and x3, and estimate the numerical values of x2 and x3.
- b. Would x1 = 5 be a better first approximation? Explain.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Find a plane containing the point (3, -3, 1) and the line of intersection of the planes 2x + 3y - 3z = 14
and -3x - y + z = −21.
The equation of the plane is:
Determine whether the lines
L₁ : F(t) = (−2, 3, −1)t + (0,2,-3) and
L2 : ƒ(s) = (2, −3, 1)s + (−10, 17, -8)
intersect. If they do, find the point of intersection.
● They intersect at the point
They are skew lines
They are parallel or equal
Answer questions 2
Chapter 4 Solutions
Single Variable Essential Calculus: Early Transcendentals
Ch. 4.1 - Explain the difference between an absolute minimum...Ch. 4.1 - Suppose f is a continuous function defined on a...Ch. 4.1 - For each of the numbers a, b, c, d, r, and s,...Ch. 4.1 - For each of the numbers a, b, c, d, r, and s,...Ch. 4.1 - Use the graph to state the absolute and local...Ch. 4.1 - Use the graph to state the absolute and local...Ch. 4.1 - Sketch the graph of a function f that is...Ch. 4.1 - 710 Sketch the graph of a function f that is...Ch. 4.1 - 710 Sketch the graph of a function f that is...Ch. 4.1 - 710 Sketch the graph of a function f that is...
Ch. 4.1 - (a) Sketch the graph of a function that has a...Ch. 4.1 - (a) Sketch the graph of a function on [1, 2] that...Ch. 4.1 - (a) Sketch the graph of a function on [1, 2] that...Ch. 4.1 - (a) Sketch the graph of a function that has two...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Sketch the graph of f by hand and use your sketch...Ch. 4.1 - Find the critical numbers of the function....Ch. 4.1 - Find the critical numbers of the function. f(x) =...Ch. 4.1 - Find the critical numbers of the function. f(x) =...Ch. 4.1 - Find the critical numbers of the function. f(x) =...Ch. 4.1 - Find the critical numbers of the function. g(t) =...Ch. 4.1 - Find the critical numbers of the function. g(t) =...Ch. 4.1 - Find the critical numbers of the function....Ch. 4.1 - Find the critical numbers of the function....Ch. 4.1 - Find the critical numbers of the function. F(x) =...Ch. 4.1 - Find the critical numbers of the function. g() = 4...Ch. 4.1 - Find the critical numbers of the function. f() = 2...Ch. 4.1 - Find the critical numbers of the function. g(x) =...Ch. 4.1 - Find the critical numbers of the function. f(x) =...Ch. 4.1 - Find the critical numbers of the function. f(x) =...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - Find the absolute maximum and absolute minimum...Ch. 4.1 - If a and b are positive numbers, find the maximum...Ch. 4.1 - Use a graph to estimate the critical numbers of...Ch. 4.1 - (a) Use a graph to estimate the absolute maximum...Ch. 4.1 - (a) Use a graph to estimate the absolute maximum...Ch. 4.1 - (a) Use a graph to estimate the absolute maximum...Ch. 4.1 - (a) Use a graph to estimate the absolute maximum...Ch. 4.1 - Between 0C and 30C, the volume V (in cubic...Ch. 4.1 - An object with weight W is dragged along a...Ch. 4.1 - A model for the U S average price of a pound of...Ch. 4.1 - The Hubble Space Telescope was deployed April 24,...Ch. 4.1 - When a foreign object lodged in the trachea...Ch. 4.1 - Show that 5 is a critical number of the function...Ch. 4.1 - Prove that the function f(x)=x101+x51+x+1 has...Ch. 4.1 - If f has a local minimum value at c, show that the...Ch. 4.1 - Prove Fermats Theorem for the case in which f has...Ch. 4.1 - A cubic function is a polynomial of degree 3; that...Ch. 4.2 - Verify that the function satisfies the three...Ch. 4.2 - Verify that the function satisfies the three...Ch. 4.2 - Verify that the function satisfies the three...Ch. 4.2 - Verify that the function satisfies the three...Ch. 4.2 - Let f(x) = 1 x2/3. Show that f(l) = f(1) but...Ch. 4.2 - Let f(x) = tan x. Show that f(0) = f() but there...Ch. 4.2 - Use the graph of f to estimate the values of c...Ch. 4.2 - Use the graph of f given in Exercise 7 to estimate...Ch. 4.2 - Verify that the function satisfies the hypotheses...Ch. 4.2 - Verify that the function satisfies the hypotheses...Ch. 4.2 - Verify that the function satisfies the hypotheses...Ch. 4.2 - Verify that the function satisfies the hypotheses...Ch. 4.2 - Find the number c that satisfies the conclusion of...Ch. 4.2 - Find the number c that satisfies the conclusion of...Ch. 4.2 - Let f(x) = (x 3)2. Show that there is no value of...Ch. 4.2 - Let f(x) = 2 |2x 1|. Show that there is no value...Ch. 4.2 - Show that the equation has exactly one real root....Ch. 4.2 - Show that the equation has exactly one real root....Ch. 4.2 - Show that the equation x3 15x + c = 0 has at most...Ch. 4.2 - Show that the equation x4 + 4x + c = 0 has at most...Ch. 4.2 - (a) Show that a polynomial of degree 3 has at most...Ch. 4.2 - (a) Suppose that f is differentiable on and has...Ch. 4.2 - If f(1) = 10 and f(x) 2 for 1 x 4, how small...Ch. 4.2 - Suppose that 3 f(x) 5 for all values of x. Show...Ch. 4.2 - Does there exist a function f such that f(0) = 1,...Ch. 4.2 - Suppose that f and g are continuous on [a, b] and...Ch. 4.2 - Show that 1+x1+12xifx0.Ch. 4.2 - Suppose f is an odd function and is differentiable...Ch. 4.2 - Use the Mean Value Theorem to prove the inequality...Ch. 4.2 - If f(x) = c (c a constant) for all x, use...Ch. 4.2 - Let f(x) = l/x and g(x)={1xifx01+1xifx0 Show that...Ch. 4.2 - Use Theorem 5 to prove the identity...Ch. 4.2 - Prove the identity arcsinx1x+1=2arctanx2Ch. 4.2 - At 2:00 PM a cars speedometer reads 30 mi/h. At...Ch. 4.2 - Two runners start a race at the same time and...Ch. 4.2 - A number a is called a fixed point of a function f...Ch. 4.3 - In each part state the x-coordinates of the...Ch. 4.3 - The graph of the first derivative f of a function...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - (a) Find the intervals on which f is increasing or...Ch. 4.3 - Find the local maximum and minimum values of f...Ch. 4.3 - Find the local maximum and minimum values of f...Ch. 4.3 - (a) Find the critical numbers of f(x) = x4(x 1)3....Ch. 4.3 - Suppose f is continuous on (, ). (a) If f(2) = 0...Ch. 4.3 - 1720 Sketch the graph of a function that satisfies...Ch. 4.3 - Sketch the graph of a function that satisfies all...Ch. 4.3 - Sketch the graph of a function that satisfies all...Ch. 4.3 - Sketch the graph of a function that satisfies all...Ch. 4.3 - Sketch the graph of a function that satisfes all...Ch. 4.3 - Sketch the graph of a function that satisfes all...Ch. 4.3 - The graph of the derivative f of a continuous...Ch. 4.3 - The graph of the derivative f of a continuous...Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the intervals of increase or decrease....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - (a) Find the vertical and horizontal asymptotes....Ch. 4.3 - Suppose the derivative of a function f is f(x) =...Ch. 4.3 - Use the methods of this section to sketch the...Ch. 4.3 - (a) Use a graph of f to estimate the maximum and...Ch. 4.3 - (a) Use a graph of f to estimate the maximum and...Ch. 4.3 - A drug response curve describes the level of...Ch. 4.3 - Prob. 50ECh. 4.3 - Find a cubic function f(x) = ax3 + bx2 + cx + d...Ch. 4.3 - For what values of the numbers a and b does the...Ch. 4.3 - (a) If the function f(x) = x3 + ax2 + bx has the...Ch. 4.3 - Show that the curve y = (1 + x)/(1 + x2) has three...Ch. 4.3 - Show that the curves y = ex and y = ex touch the...Ch. 4.3 - Show that the inflection points of the curve y = x...Ch. 4.3 - Show that tan x x for 0 x /2. [Hint: Show that...Ch. 4.3 - (a) Show that ex 1 + x for x 0. (b) Deduce that...Ch. 4.3 - Show that a cubic function (a third-degree...Ch. 4.3 - For what values of c does the polynomial P(x) = x4...Ch. 4.3 - Prove that if (c, f(c)) is a point of inflection...Ch. 4.3 - Show that if f(x) = x4, then f(0) = 0, but (0, 0)...Ch. 4.3 - Show that the function g(x) = x | x | has an...Ch. 4.3 - Suppose that f is continuous and f(c) = f(c) = 0,...Ch. 4.3 - Suppose f is differentiable on an interval I and...Ch. 4.3 - For what values of c is the function f(x)=cx+1x2+3...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - 144 Use the guidelines of this section to sketch...Ch. 4.4 - 144 Use the guidelines of this section to sketch...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - 144 Use the guidelines of this section to sketch...Ch. 4.4 - 144 Use the guidelines of this section to sketch...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - The table gives the population of the world P(t),...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Prob. 27ECh. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - 144 Use the guidelines of this section to sketch...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - 144 Use the guidelines of this section to sketch...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - In the theory of relativity, the mass of a...Ch. 4.4 - In the theory of relativity, the energy of a...Ch. 4.4 - The figure shows a beam of length L embedded in...Ch. 4.4 - Coulombs Law states that the force of attraction...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Use the guidelines of this section to sketch the...Ch. 4.4 - Show that the curve y = x tan1 x has two slant...Ch. 4.4 - Show that the curve y=x2+4x has two slant...Ch. 4.4 - Produce graphs of f that reveal all the important...Ch. 4.4 - Produce graphs of f that reveal all the important...Ch. 4.4 - Produce graphs of f that reveal all the important...Ch. 4.4 - Produce graphs of f that reveal all the important...Ch. 4.4 - Produce graphs of f that reveal all the important...Ch. 4.4 - Produce graphs of f that reveal all the important...Ch. 4.4 - Describe how the graph of f varies as c varies....Ch. 4.4 - Describe how the graph of f varies as c varics....Ch. 4.4 - Describe how the graph of f varies as c varics....Ch. 4.4 - Describe how the graph of f varies as c varics....Ch. 4.4 - Describe how the graph of f varies as c varies....Ch. 4.4 - Investigate the family of curves given by the...Ch. 4.5 - Consider the following problem: Find two numbers...Ch. 4.5 - Find two numbers whose difference is 100 and whose...Ch. 4.5 - Prob. 3ECh. 4.5 - Prob. 4ECh. 4.5 - Prob. 5ECh. 4.5 - Prob. 6ECh. 4.5 - Prob. 7ECh. 4.5 - Prob. 8ECh. 4.5 - Prob. 9ECh. 4.5 - Consider the following problem: A box with an open...Ch. 4.5 - Prob. 12ECh. 4.5 - Prob. 11ECh. 4.5 - A rectangular storage container with an open top...Ch. 4.5 - Prob. 13ECh. 4.5 - Prob. 15ECh. 4.5 - Prob. 16ECh. 4.5 - Prob. 17ECh. 4.5 - Prob. 18ECh. 4.5 - Prob. 24ECh. 4.5 - Prob. 19ECh. 4.5 - Prob. 20ECh. 4.5 - Prob. 21ECh. 4.5 - Prob. 22ECh. 4.5 - Prob. 23ECh. 4.5 - Prob. 26ECh. 4.5 - Prob. 25ECh. 4.5 - Prob. 27ECh. 4.5 - Prob. 28ECh. 4.5 - Prob. 29ECh. 4.5 - Prob. 30ECh. 4.5 - Prob. 31ECh. 4.5 - Prob. 33ECh. 4.5 - Prob. 34ECh. 4.5 - Prob. 35ECh. 4.5 - Prob. 36ECh. 4.5 - Prob. 38ECh. 4.5 - Prob. 37ECh. 4.5 - Prob. 39ECh. 4.5 - Prob. 40ECh. 4.5 - Prob. 41ECh. 4.5 - Prob. 42ECh. 4.5 - Prob. 43ECh. 4.5 - Prob. 44ECh. 4.5 - Prob. 45ECh. 4.5 - Prob. 46ECh. 4.5 - Prob. 47ECh. 4.5 - Prob. 48ECh. 4.5 - Prob. 49ECh. 4.5 - Prob. 50ECh. 4.5 - Prob. 32ECh. 4.5 - Prob. 51ECh. 4.5 - Prob. 52ECh. 4.5 - Prob. 53ECh. 4.5 - Prob. 54ECh. 4.5 - Prob. 56ECh. 4.5 - Prob. 57ECh. 4.5 - Prob. 58ECh. 4.5 - Prob. 55ECh. 4.6 - The figure shows the graph of a function f....Ch. 4.6 - Follow the instructions for Exercise 1 (a) but use...Ch. 4.6 - Prob. 3ECh. 4.6 - For each initial approximation, determine...Ch. 4.6 - Prob. 5ECh. 4.6 - Use Newtons method with the specified initial...Ch. 4.6 - Prob. 7ECh. 4.6 - Prob. 8ECh. 4.6 - Prob. 9ECh. 4.6 - Prob. 10ECh. 4.6 - Use Newtons method to approximate the given number...Ch. 4.6 - Prob. 12ECh. 4.6 - Prob. 13ECh. 4.6 - Prob. 14ECh. 4.6 - Prob. 15ECh. 4.6 - Prob. 16ECh. 4.6 - Prob. 17ECh. 4.6 - Prob. 18ECh. 4.6 - Prob. 21ECh. 4.6 - Prob. 19ECh. 4.6 - Prob. 20ECh. 4.6 - Prob. 22ECh. 4.6 - Prob. 23ECh. 4.6 - Prob. 24ECh. 4.6 - Prob. 25ECh. 4.6 - Prob. 26ECh. 4.6 - Prob. 27ECh. 4.6 - Prob. 28ECh. 4.6 - Prob. 29ECh. 4.6 - Prob. 30ECh. 4.6 - Prob. 31ECh. 4.6 - Prob. 32ECh. 4.7 - Find the most general antiderivative of the...Ch. 4.7 - Find the most general antiderivative of the...Ch. 4.7 - Prob. 3ECh. 4.7 - Prob. 5ECh. 4.7 - Prob. 4ECh. 4.7 - Prob. 6ECh. 4.7 - Prob. 7ECh. 4.7 - Find the most general antiderivative of the...Ch. 4.7 - Prob. 9ECh. 4.7 - Prob. 10ECh. 4.7 - Prob. 11ECh. 4.7 - Prob. 12ECh. 4.7 - Prob. 13ECh. 4.7 - Prob. 14ECh. 4.7 - Prob. 15ECh. 4.7 - Prob. 16ECh. 4.7 - Prob. 17ECh. 4.7 - Find f. f(x) = x6 4x4 + x + 1Ch. 4.7 - Prob. 19ECh. 4.7 - Prob. 20ECh. 4.7 - Prob. 21ECh. 4.7 - Prob. 22ECh. 4.7 - Prob. 23ECh. 4.7 - Prob. 24ECh. 4.7 - Prob. 25ECh. 4.7 - Prob. 26ECh. 4.7 - Prob. 27ECh. 4.7 - Prob. 28ECh. 4.7 - Prob. 29ECh. 4.7 - Prob. 30ECh. 4.7 - Find f. f() = sin + cos , f(0) = 3, f(0) = 4Ch. 4.7 - Prob. 32ECh. 4.7 - Prob. 33ECh. 4.7 - Prob. 34ECh. 4.7 - Prob. 35ECh. 4.7 - Prob. 36ECh. 4.7 - Prob. 37ECh. 4.7 - Prob. 38ECh. 4.7 - Prob. 39ECh. 4.7 - Prob. 40ECh. 4.7 - Prob. 41ECh. 4.7 - A particle is moving with the given data. Find the...Ch. 4.7 - Prob. 43ECh. 4.7 - Prob. 44ECh. 4.7 - Prob. 45ECh. 4.7 - Prob. 46ECh. 4.7 - Prob. 47ECh. 4.7 - Prob. 48ECh. 4.7 - Prob. 49ECh. 4.7 - Prob. 50ECh. 4.7 - Prob. 51ECh. 4.7 - Prob. 52ECh. 4.7 - Prob. 53ECh. 4.7 - Prob. 54ECh. 4.7 - Prob. 55ECh. 4 - Prob. 44RECh. 4 - Prob. 1RCCCh. 4 - Prob. 2RCCCh. 4 - Prob. 3RCCCh. 4 - Prob. 4RCCCh. 4 - Prob. 5RCCCh. 4 - Prob. 6RCCCh. 4 - Prob. 7RCCCh. 4 - Prob. 8RCCCh. 4 - Prob. 9RCCCh. 4 - Prob. 1RQCh. 4 - Prob. 2RQCh. 4 - Prob. 3RQCh. 4 - Prob. 4RQCh. 4 - Prob. 5RQCh. 4 - Prob. 6RQCh. 4 - Prob. 7RQCh. 4 - Prob. 8RQCh. 4 - Prob. 9RQCh. 4 - Prob. 10RQCh. 4 - Prob. 11RQCh. 4 - Prob. 12RQCh. 4 - Prob. 13RQCh. 4 - Prob. 14RQCh. 4 - Prob. 15RQCh. 4 - Prob. 16RQCh. 4 - Prob. 17RQCh. 4 - Prob. 18RQCh. 4 - Prob. 19RQCh. 4 - Prob. 1RECh. 4 - Prob. 2RECh. 4 - Prob. 3RECh. 4 - Prob. 4RECh. 4 - Prob. 5RECh. 4 - Prob. 6RECh. 4 - Prob. 7RECh. 4 - The figure shows the graph of the derivative f of...Ch. 4 - Prob. 9RECh. 4 - Prob. 10RECh. 4 - Prob. 11RECh. 4 - Prob. 12RECh. 4 - Prob. 13RECh. 4 - Prob. 14RECh. 4 - 1524 Use the guidelines of Section 4.4 to sketch...Ch. 4 - Prob. 16RECh. 4 - Prob. 18RECh. 4 - Prob. 17RECh. 4 - Prob. 20RECh. 4 - Prob. 19RECh. 4 - Prob. 22RECh. 4 - Prob. 21RECh. 4 - Prob. 23RECh. 4 - Prob. 24RECh. 4 - Prob. 25RECh. 4 - Prob. 26RECh. 4 - Prob. 27RECh. 4 - Prob. 28RECh. 4 - Prob. 29RECh. 4 - Prob. 33RECh. 4 - Prob. 34RECh. 4 - Prob. 35RECh. 4 - Prob. 36RECh. 4 - Prob. 37RECh. 4 - Prob. 38RECh. 4 - Prob. 39RECh. 4 - Prob. 40RECh. 4 - Prob. 41RECh. 4 - Prob. 42RECh. 4 - Prob. 43RECh. 4 - Prob. 45RECh. 4 - A metal storage tank with volume V is to be...Ch. 4 - Prob. 47RECh. 4 - Prob. 48RECh. 4 - Prob. 49RECh. 4 - Prob. 50RECh. 4 - Prob. 51RECh. 4 - Prob. 52RECh. 4 - Prob. 53RECh. 4 - Prob. 54RECh. 4 - Prob. 55RECh. 4 - Prob. 56RECh. 4 - Prob. 57RECh. 4 - Prob. 58RECh. 4 - Prob. 60RECh. 4 - Prob. 59RECh. 4 - Prob. 61RE
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- How does a fourier transform works?arrow_forwardDetermine the radius of convergence of a power series:12.6.5, 12.6.6, 12.6.7, 12.6.8Hint: Use Theorem12.5.1 and root test, ratio test, integral testarrow_forwardCan you answer this question and give step by step and why and how to get it. Can you write it (numerical method)arrow_forward
- Can you answer this question and give step by step and why and how to get it. Can you write it (numerical method)arrow_forwardThere are three options for investing $1150. The first earns 10% compounded annually, the second earns 10% compounded quarterly, and the third earns 10% compounded continuously. Find equations that model each investment growth and use a graphing utility to graph each model in the same viewing window over a 20-year period. Use the graph to determine which investment yields the highest return after 20 years. What are the differences in earnings among the three investment? STEP 1: The formula for compound interest is A = nt = P(1 + − − ) n², where n is the number of compoundings per year, t is the number of years, r is the interest rate, P is the principal, and A is the amount (balance) after t years. For continuous compounding, the formula reduces to A = Pert Find r and n for each model, and use these values to write A in terms of t for each case. Annual Model r=0.10 A = Y(t) = 1150 (1.10)* n = 1 Quarterly Model r = 0.10 n = 4 A = Q(t) = 1150(1.025) 4t Continuous Model r=0.10 A = C(t) =…arrow_forwardUse a graphing utility to find the point of intersection, if any, of the graphs of the functions. Round your result to three decimal places. (Enter NONE in any unused answer blanks.) y = 100e0.01x (x, y) = y = 11,250 ×arrow_forward
- 5. For the function y-x³-3x²-1, use derivatives to: (a) determine the intervals of increase and decrease. (b) determine the local (relative) maxima and minima. (e) determine the intervals of concavity. (d) determine the points of inflection. (e) sketch the graph with the above information indicated on the graph.arrow_forwardCan you solve this 2 question numerical methodarrow_forward1. Estimate the area under the graph of f(x)-25-x from x=0 to x=5 using 5 approximating rectangles Using: (A) right endpoints. (B) left endpoints.arrow_forward
- 9. Use fundamental theorem of calculus to find the derivative d a) *dt sin(x) b)(x)√1-2 dtarrow_forward3. Evaluate the definite integral: a) √66x²+8dx b) x dx c) f*(2e* - 2)dx d) √√9-x² e) (2-5x)dx f) cos(x)dx 8)²₁₂√4-x2 h) f7dx i) f² 6xdx j) ²₂(4x+3)dxarrow_forward2. Consider the integral √(2x+1)dx (a) Find the Riemann sum for this integral using right endpoints and n-4. (b) Find the Riemann sum for this same integral, using left endpoints and n=4arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Chain Rule dy:dx = dy:du*du:dx; Author: Robert Cappetta;https://www.youtube.com/watch?v=IUYniALwbHs;License: Standard YouTube License, CC-BY
CHAIN RULE Part 1; Author: Btech Maths Hub;https://www.youtube.com/watch?v=TIAw6AJ_5Po;License: Standard YouTube License, CC-BY