MyLab Math with Pearson eText -- 24-Month Standalone Access Card -- for College Algebra in Context
5th Edition
ISBN: 9780135909621
Author: HARSHBARGER, Ronald, YOCCO, Lisa
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 4.2, Problem 42E
Population of Children The following table gives the estimated population (in millions) of U.S. boys age 5 and under and U.S. girls age 5 and under in selected years.
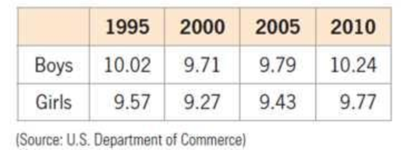
A function that models the population (in millions) of U.S. boys age 5 and under t years after 1990 is B(t) = 0.0076t2 – 0.1752t + 10.705, and a function that models the population (in millions) of U.S. girls age 5 and under t years after 1990 is G(t) = 0.0064t2 – 0.1448t + 10.12.
a. Find the equation of a function that models the estimated U.S. population (in millions) of children age 5 and under t years after 1990.
b. Use the result of part (a) to estimate the U.S. population of children age 5 and under in 2003.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Melissa claims the triangles are similar. Is her claim correct? If her claim is correct, state the transformations that map
△MNO
△MNO
onto
△PRQ.
△PRQ.
If her claim is not correct, explain why.
2 (VaR and ES) Suppose X1
are independent. Prove that
~
Unif[-0.5, 0.5] and X2
VaRa (X1X2) < VaRa(X1) + VaRa (X2).
~
Unif[-0.5, 0.5]
8 (Correlation and Diversification)
Assume we have two stocks, A and B, show that a particular combination
of the two stocks produce a risk-free portfolio when the correlation between
the return of A and B is -1.
Chapter 4 Solutions
MyLab Math with Pearson eText -- 24-Month Standalone Access Card -- for College Algebra in Context
Ch. 4.1 - In Exercises 116, (a) sketch the graph of each...Ch. 4.1 - In Exercises 116, (a) sketch the graph of each...Ch. 4.1 - Prob. 3ECh. 4.1 - Prob. 4ECh. 4.1 - Prob. 5ECh. 4.1 - In Exercises 116, (a) sketch the graph of each...Ch. 4.1 - Prob. 7ECh. 4.1 - Prob. 8ECh. 4.1 - Prob. 9ECh. 4.1 - Prob. 10E
Ch. 4.1 - Prob. 11ECh. 4.1 - In Exercises 116, (a) sketch the graph of each...Ch. 4.1 - Prob. 13ECh. 4.1 - Prob. 14ECh. 4.1 - Prob. 15ECh. 4.1 - Prob. 16ECh. 4.1 - Prob. 17ECh. 4.1 - Prob. 18ECh. 4.1 - Prob. 19ECh. 4.1 - Prob. 20ECh. 4.1 - Prob. 21ECh. 4.1 - Prob. 22ECh. 4.1 - Prob. 23ECh. 4.1 - Prob. 24ECh. 4.1 - Prob. 25ECh. 4.1 - Prob. 26ECh. 4.1 - Prob. 27ECh. 4.1 - Prob. 28ECh. 4.1 - Prob. 29ECh. 4.1 - In Exercises 2932, write the equation of the...Ch. 4.1 - Prob. 31ECh. 4.1 - Prob. 32ECh. 4.1 - Prob. 33ECh. 4.1 - Prob. 34ECh. 4.1 - Prob. 35ECh. 4.1 - Prob. 36ECh. 4.1 - Prob. 37ECh. 4.1 - Prob. 38ECh. 4.1 - Prob. 39ECh. 4.1 - Prob. 40ECh. 4.1 - Prob. 41ECh. 4.1 - Prob. 42ECh. 4.1 - Prob. 43ECh. 4.1 - Prob. 44ECh. 4.1 - Prob. 45ECh. 4.1 - Prob. 46ECh. 4.1 - Prob. 47ECh. 4.1 - Prob. 48ECh. 4.1 - Prob. 49ECh. 4.1 - Prob. 50ECh. 4.1 - Prob. 51ECh. 4.1 - Prob. 52ECh. 4.1 - Determine whether each of the complete graphs in...Ch. 4.1 - Prob. 54ECh. 4.1 - Prob. 55ECh. 4.1 - Prob. 56ECh. 4.1 - Marijuana Use The number of millions of people age...Ch. 4.1 - Prob. 58ECh. 4.1 - Prob. 59ECh. 4.1 - Prob. 60ECh. 4.1 - Prob. 61ECh. 4.1 - Pollution The daily cost C (in dollars) of...Ch. 4.1 - Population Growth Suppose the population of a...Ch. 4.1 - Mortgages The balance owed y on a 50,000 mortgage...Ch. 4.1 - Personal Expenditures Personal consumption...Ch. 4.1 - Prob. 66ECh. 4.1 - Prob. 67ECh. 4.1 - Prob. 68ECh. 4.1 - Prob. 69ECh. 4.1 - Prob. 70ECh. 4.1 - Prob. 71ECh. 4.1 - Prob. 72ECh. 4.2 - In Exercises 18, find the following: (a) (f + g)...Ch. 4.2 - In Exercises 18, find the following: (a) (f + g)...Ch. 4.2 - Prob. 3ECh. 4.2 - Prob. 4ECh. 4.2 - Prob. 5ECh. 4.2 - Prob. 6ECh. 4.2 - Prob. 7ECh. 4.2 - Prob. 8ECh. 4.2 - Prob. 9ECh. 4.2 - Prob. 10ECh. 4.2 - Prob. 11ECh. 4.2 - Prob. 12ECh. 4.2 - Prob. 13ECh. 4.2 - Prob. 14ECh. 4.2 - Prob. 15ECh. 4.2 - Prob. 16ECh. 4.2 - Prob. 17ECh. 4.2 - Prob. 18ECh. 4.2 - Prob. 19ECh. 4.2 - Prob. 20ECh. 4.2 - Prob. 21ECh. 4.2 - Prob. 22ECh. 4.2 - Prob. 23ECh. 4.2 - Prob. 24ECh. 4.2 - Profit Suppose that the total weekly cost for the...Ch. 4.2 - Prob. 26ECh. 4.2 - Revenue and Cost The total revenue function for...Ch. 4.2 - Prob. 28ECh. 4.2 - Prob. 29ECh. 4.2 - Revenue and Cost The total monthly revenue...Ch. 4.2 - Prob. 31ECh. 4.2 - Cost-Benefit Suppose that for a certain city the...Ch. 4.2 - Prob. 33ECh. 4.2 - Electronic Components The monthly cost of...Ch. 4.2 - Football Tickets At a certain school, the number...Ch. 4.2 - Prob. 36ECh. 4.2 - Prob. 37ECh. 4.2 - Prob. 38ECh. 4.2 - Prob. 39ECh. 4.2 - Profit A manufacturer of computers has monthly...Ch. 4.2 - Prob. 41ECh. 4.2 - Population of Children The following table gives...Ch. 4.2 - Prob. 43ECh. 4.2 - Prob. 44ECh. 4.2 - Shoe Sizes A womans shoe that is size x in Japan...Ch. 4.2 - Shoe Sizes A mans shoe that is size x in Britain...Ch. 4.2 - Exchange Rates On a certain date, each Japanese...Ch. 4.2 - Prob. 48ECh. 4.2 - Prob. 49ECh. 4.2 - Prob. 50ECh. 4.2 - Prob. 51ECh. 4.2 - Prob. 52ECh. 4.2 - Prob. 53ECh. 4.2 - Prob. 54ECh. 4.3 - In Exercises 1 and 2, determine if the function f...Ch. 4.3 - Prob. 2ECh. 4.3 - Prob. 3ECh. 4.3 - Prob. 4ECh. 4.3 - Prob. 5ECh. 4.3 - Prob. 6ECh. 4.3 - Prob. 7ECh. 4.3 - Prob. 8ECh. 4.3 - Prob. 9ECh. 4.3 - Prob. 10ECh. 4.3 - Prob. 11ECh. 4.3 - Prob. 12ECh. 4.3 - Prob. 13ECh. 4.3 - Prob. 14ECh. 4.3 - Prob. 15ECh. 4.3 - In Exercises 15 and 16, determine whether each...Ch. 4.3 - Prob. 17ECh. 4.3 - Prob. 18ECh. 4.3 - a. Write the inverse of f(x) = 3x 4. b. Do the...Ch. 4.3 - a.Write the inverse of g(x)=2x31. b.Do the values...Ch. 4.3 - Prob. 21ECh. 4.3 - Prob. 22ECh. 4.3 - Prob. 23ECh. 4.3 - Prob. 24ECh. 4.3 - Prob. 25ECh. 4.3 - Prob. 26ECh. 4.3 - Prob. 27ECh. 4.3 - Prob. 28ECh. 4.3 - Prob. 29ECh. 4.3 - Prob. 30ECh. 4.3 - Prob. 31ECh. 4.3 - Prob. 32ECh. 4.3 - Shoe Sizes If x is the size of a mans shoe in...Ch. 4.3 - Investments If x dollars are invested at 10% for 6...Ch. 4.3 - Currency Conversion Suppose the function that...Ch. 4.3 - Apparent Temperature If the outside temperature is...Ch. 4.3 - Prob. 37ECh. 4.3 - Body-Heat Loss The model for body-heat loss...Ch. 4.3 - Prob. 39ECh. 4.3 - Decoding Messages If we assign numbers to the...Ch. 4.3 - Prob. 41ECh. 4.3 - Social Security Numbers and Income Taxes Consider...Ch. 4.3 - Prob. 43ECh. 4.3 - Volume of a Cube The volume of a cube is f(x) = x3...Ch. 4.3 - Prob. 45ECh. 4.3 - Surface Area The surface area of a cube is...Ch. 4.3 - Internet Access Using data from 2009 through 2015,...Ch. 4.3 - Prob. 48ECh. 4.3 - Prob. 49ECh. 4.3 - First-Class Postage The postage charged for...Ch. 4.3 - Prob. 51ECh. 4.3 - Path of a Ball If a ball is thrown into the air at...Ch. 4.4 - In Exercises 120, solve the equations...Ch. 4.4 - Prob. 2ECh. 4.4 - Prob. 3ECh. 4.4 - Prob. 4ECh. 4.4 - Prob. 5ECh. 4.4 - In Exercises 120, solve the equations...Ch. 4.4 - Prob. 7ECh. 4.4 - In Exercises 120, solve the equations...Ch. 4.4 - Prob. 9ECh. 4.4 - Prob. 10ECh. 4.4 - Prob. 11ECh. 4.4 - Prob. 12ECh. 4.4 - Prob. 13ECh. 4.4 - Prob. 14ECh. 4.4 - Prob. 15ECh. 4.4 - Prob. 16ECh. 4.4 - Prob. 17ECh. 4.4 - Prob. 18ECh. 4.4 - Prob. 19ECh. 4.4 - Prob. 20ECh. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - Prob. 22ECh. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - Prob. 24ECh. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - In Exercises 2130, use algebraic methods to solve...Ch. 4.4 - In Exercises 3134, use graphical methods to solve...Ch. 4.4 - In Exercises 3134, use graphical methods to solve...Ch. 4.4 - Prob. 33ECh. 4.4 - Prob. 34ECh. 4.4 - In Exercises 3542, solve the inequalities by using...Ch. 4.4 - Prob. 36ECh. 4.4 - Prob. 37ECh. 4.4 - Prob. 38ECh. 4.4 - In Exercises 3542, solve the inequalities by using...Ch. 4.4 - Prob. 40ECh. 4.4 - Prob. 41ECh. 4.4 - Prob. 42ECh. 4.4 - Prob. 43ECh. 4.4 - Prob. 44ECh. 4.4 - Prob. 45ECh. 4.4 - Prob. 46ECh. 4.4 - Prob. 47ECh. 4.4 - Prob. 48ECh. 4.4 - Prob. 49ECh. 4.4 - Prob. 50ECh. 4.4 - Prob. 51ECh. 4.4 - Prob. 52ECh. 4.4 - Use algebraic and/or graphical methods to solve...Ch. 4.4 - Prob. 54ECh. 4.4 - Prob. 55ECh. 4.4 - Prob. 56ECh. 4.4 - Prob. 57ECh. 4.4 - Prob. 58ECh. 4.4 - Prob. 59ECh. 4.4 - Prob. 60ECh. 4.4 - Prob. 61ECh. 4.4 - Prob. 62ECh. 4.4 - Prob. 63ECh. 4.4 - Use graphical and/or numerical methods to solve...Ch. 4.4 - Retail Sales November and December retail sales,...Ch. 4.4 - Voltage Required voltage for an electric oven is...Ch. 4.4 - Purchasing Power Inflation causes a decrease in...Ch. 4 - The domain of the reciprocal function is _____,...Ch. 4 - The domain of the constant function g(x) = k is...Ch. 4 - The reciprocal function decreases on _____.Ch. 4 - The absolute value function increase on the...Ch. 4 - The range of the squaring function is _____.Ch. 4 - Prob. 6TECh. 4 - Prob. 7TECh. 4 - Prob. 8TECh. 4 - Prob. 9TECh. 4 - In Exercises 712, determine whether the function...Ch. 4 - In Exercises 712, determine whether the function...Ch. 4 - Prob. 12TECh. 4 - Prob. 13TECh. 4 - Prob. 14TECh. 4 - Prob. 15TECh. 4 - Prob. 16TECh. 4 - Prob. 17TECh. 4 - Prob. 18TECh. 4 - Prob. 19TECh. 4 - Prob. 20TECh. 4 - Prob. 21TECh. 4 - Prob. 22TECh. 4 - How is the graph of g(x) = (x 8)2 + 7 transformed...Ch. 4 - How is the graph of g(x) = 2(x + 1)3 transformed...Ch. 4 - Prob. 3RECh. 4 - Prob. 4RECh. 4 - Prob. 5RECh. 4 - Prob. 6RECh. 4 - Prob. 7RECh. 4 - Prob. 8RECh. 4 - Prob. 9RECh. 4 - In Exercises 10 and 11, determine algebraically...Ch. 4 - In Exercises 10 and 11, determine algebraically...Ch. 4 - Prob. 12RECh. 4 - Prob. 13RECh. 4 - Prob. 14RECh. 4 - Prob. 15RECh. 4 - Prob. 16RECh. 4 - Prob. 17RECh. 4 - For Exercise 1320, use the functions f(x)=3x25x,...Ch. 4 - Prob. 19RECh. 4 - Prob. 20RECh. 4 - Prob. 21RECh. 4 - Prob. 22RECh. 4 - Prob. 23RECh. 4 - Prob. 24RECh. 4 - Is the function f(x)=xx1 a one-to-one function?Ch. 4 - Prob. 26RECh. 4 - Prob. 27RECh. 4 - Prob. 28RECh. 4 - Solve 4x5x1/2+1=0.Ch. 4 - Prob. 30RECh. 4 - Prob. 31RECh. 4 - Prob. 32RECh. 4 - Prob. 33RECh. 4 - Prob. 34RECh. 4 - Prob. 35RECh. 4 - Prob. 36RECh. 4 - Prob. 37RECh. 4 - Prob. 38RECh. 4 - Prob. 39RECh. 4 - Prob. 40RECh. 4 - Prob. 41RECh. 4 - Prob. 42RECh. 4 - Prob. 43RECh. 4 - Prob. 44RECh. 4 - Prob. 45RECh. 4 - Prob. 46RECh. 4 - Prob. 47RECh. 4 - Prison Sentences The mean time in prison y for...Ch. 4 - Prob. 49RECh. 4 - Prob. 50RECh. 4 - Prob. 51RECh. 4 - The Cloud North American cloud computing revenues...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- 9 (Portfolio allocation) Suppose R₁ and R2 are returns of 2 assets and with expected return and variance respectively r₁ and 72 and variance-covariance σ2, 0%½ and σ12. Find −∞ ≤ w ≤ ∞ such that the portfolio wR₁ + (1 - w) R₂ has the smallest risk.arrow_forward7 (Multivariate random variable) Suppose X, €1, €2, €3 are IID N(0, 1) and Y2 Y₁ = 0.2 0.8X + €1, Y₂ = 0.3 +0.7X+ €2, Y3 = 0.2 + 0.9X + €3. = (In models like this, X is called the common factors of Y₁, Y₂, Y3.) Y = (Y1, Y2, Y3). (a) Find E(Y) and cov(Y). (b) What can you observe from cov(Y). Writearrow_forward1 (VaR and ES) Suppose X ~ f(x) with 1+x, if 0> x > −1 f(x) = 1−x if 1 x > 0 Find VaRo.05 (X) and ES0.05 (X).arrow_forward
- (^) k Recall that for numbers 0 ≤ k ≤ n the binomial coefficient (^) is defined as n! k! (n−k)! Question 1. (1) Prove the following identity: (22) + (1121) = (n+1). (2) Use the identity above to prove the binomial theorem by induction. That is, prove that for any a, b = R, n (a + b)" = Σ (^) an- n-kyk. k=0 n Recall that Σ0 x is short hand notation for the expression x0+x1+ +xn- (3) Fix x = R, x > 0. Prove Bernoulli's inequality: (1+x)" ≥1+nx, by using the binomial theorem. - Question 2. Prove that ||x| - |y|| ≤ |x − y| for any real numbers x, y. Question 3. Assume (In) nEN is a sequence which is unbounded above. That is, the set {xn|nЄN} is unbounded above. Prove that there are natural numbers N] k for all k Є N. be natural numbers (nk Є N). Prove thatarrow_forwardQuestion content area top Part 1 Find the measure of ABC for the congruent triangles ABC and Upper A prime Upper B prime Upper C primeA′B′C′. 79 degrees79° 1533 2930 Part 1 m ABCequals=enter your response heredegreesarrow_forwardJoy is making Christmas gifts. She has 6 1/12 feet of yarn and will need 4 1/4 to complete our project. How much yarn will she have left over compute this solution in two different ways arrow_forward
- Solve for X. Explain each step. 2^2x • 2^-4=8arrow_forwardFind the range and all the answers. Remark that the range isn’t between -(pi/2) and (pi/2)arrow_forwardOne hundred people were surveyed, and one question pertained to their educational background. The results of this question and their genders are given in the following table. Female (F) Male (F′) Total College degree (D) 30 20 50 No college degree (D′) 30 20 50 Total 60 40 100 If a person is selected at random from those surveyed, find the probability of each of the following events.1. The person is female or has a college degree. Answer: equation editor Equation Editor 2. The person is male or does not have a college degree. Answer: equation editor Equation Editor 3. The person is female or does not have a college degree.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
01 - What Is A Differential Equation in Calculus? Learn to Solve Ordinary Differential Equations.; Author: Math and Science;https://www.youtube.com/watch?v=K80YEHQpx9g;License: Standard YouTube License, CC-BY
Higher Order Differential Equation with constant coefficient (GATE) (Part 1) l GATE 2018; Author: GATE Lectures by Dishank;https://www.youtube.com/watch?v=ODxP7BbqAjA;License: Standard YouTube License, CC-BY
Solution of Differential Equations and Initial Value Problems; Author: Jefril Amboy;https://www.youtube.com/watch?v=Q68sk7XS-dc;License: Standard YouTube License, CC-BY