
A Thomson-type experiment with relativistic electrons. One of the earliest experiments to show that p = γmv (rather than p = mv) was that of Neumann. [G. Neumann, Ann. Physik 45:529 (1914)]. The apparatus shown in Figure P4.5 is identical to Thomson’s except that the source of high-speed electrons is a radioactive radium source and the magnetic field B is arranged to act on the electron over its entire trajectory from source to detector. The combined electric and magnetic fields act as a velocity selector, only passing electrons with speed v, where v = V/Bd (Equation 4.6), while in the region where there is only a magnetic field the electron moves in a circle of radius r, with r given by p = Bre. This latter region (E = 0, B = constant) acts as a momentum selector because electrons with larger momenta have paths with larger radii. (a) Show that the radius of the circle described by the electron is given by r = (l2 + y2)/2y. (b) Typical values for the Neumann experiment were d = 2.51 × 10−4 m, B = 0.0177 T, and l = 0.0247 m. For V = 1060 V, y, the most critical value, was measured to be 0.0024 ± 0.0005 m. Show that these values disagree with the y value calculated from p = mv but agree with the y value calculated from p = γmv within experimental error. (Hint: Find v from Equation 4.6, use mv = Bre or γmv = Bre to find r, and use r to find y.)
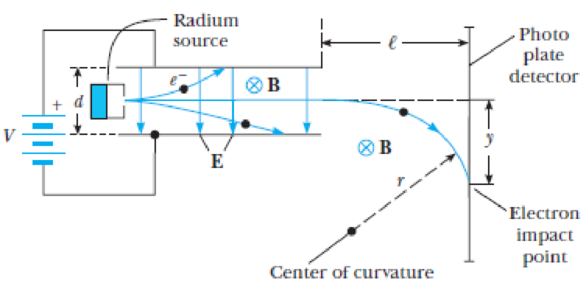
Figure P4.5 The Neumann apparatus.
(a)
To show that the radius of the circle described by th electron is given by
Answer to Problem 5P
It is showed that the radius of the circle described by th electron is given by
Explanation of Solution
The curved path of the electron is shown in figure 1.
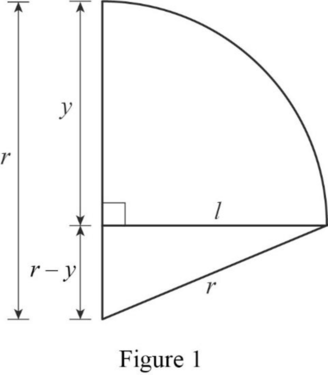
Write the equation for the curved path of the electron.
Here,
Rewrite the above equation.
Rewrite the above equation for
Conclusion:
Therefore, it is showed that the radius of the circle described by th electron is given by
(b)
To show that the value of
Answer to Problem 5P
It is showed that the calculated that the value of
Explanation of Solution
Write the equation for the velocity of the electron.
Here,
Write the equation for the momentum of the electron.
Here,
Write the classical expression for the momentum of a particle.
Here,
Equate equations (III) and (IV) and rewrite it for
Write the relativistic equation for the momentum of the particle.
Here,
Equate equations (III) and (VI) and rewrite it for
Write the equation for the Lorentz factor.
Here,
Put the above equation in equation (VII).
Write the equation for the root of a quadratic equation
Conclusion:
The mass of the electron is
Substitute
Substitute
Substitute
Comparison of the above equation with the quadratic equation
Substitute
The value
The value
Substitute
Substitute
Comparison of the above equation with the quadratic equation
Substitute
The value
The value
Therefore, it is showed that the calculated that the value of
Want to see more full solutions like this?
Chapter 4 Solutions
Modern Physics, 3rd Edition
- air is pushed steadily though a forced air pipe at a steady speed of 4.0 m/s. the pipe measures 56 cm by 22 cm. how fast will air move though a narrower portion of the pipe that is also rectangular and measures 32 cm by 22 cmarrow_forwardNo chatgpt pls will upvotearrow_forward13.87 ... Interplanetary Navigation. The most efficient way to send a spacecraft from the earth to another planet is by using a Hohmann transfer orbit (Fig. P13.87). If the orbits of the departure and destination planets are circular, the Hohmann transfer orbit is an elliptical orbit whose perihelion and aphelion are tangent to the orbits of the two planets. The rockets are fired briefly at the depar- ture planet to put the spacecraft into the transfer orbit; the spacecraft then coasts until it reaches the destination planet. The rockets are then fired again to put the spacecraft into the same orbit about the sun as the destination planet. (a) For a flight from earth to Mars, in what direction must the rockets be fired at the earth and at Mars: in the direction of motion, or opposite the direction of motion? What about for a flight from Mars to the earth? (b) How long does a one- way trip from the the earth to Mars take, between the firings of the rockets? (c) To reach Mars from the…arrow_forward
- No chatgpt pls will upvotearrow_forwarda cubic foot of argon at 20 degrees celsius is isentropically compressed from 1 atm to 425 KPa. What is the new temperature and density?arrow_forwardCalculate the variance of the calculated accelerations. The free fall height was 1753 mm. The measured release and catch times were: 222.22 800.00 61.11 641.67 0.00 588.89 11.11 588.89 8.33 588.89 11.11 588.89 5.56 586.11 2.78 583.33 Give in the answer window the calculated repeated experiment variance in m/s2.arrow_forward
- How can i solve this if n1 (refractive index of gas) and n2 (refractive index of plastic) is not known. And the brewsters angle isn't knownarrow_forward2. Consider the situation described in problem 1 where light emerges horizontally from ground level. Take k = 0.0020 m' and no = 1.0001 and find at which horizontal distance, x, the ray reaches a height of y = 1.5 m.arrow_forward2-3. Consider the situation of the reflection of a pulse at the interface of two string described in the previous problem. In addition to the net disturbances being equal at the junction, the slope of the net disturbances must also be equal at the junction at all times. Given that p1 = 4.0 g/m, H2 = 9.0 g/m and Aj = 0.50 cm find 2. A, (Answer: -0.10 cm) and 3. Ay. (Answer: 0.40 cm)please I need to show all work step by step problems 2 and 3arrow_forward
