
Concept explainers
Mark each statement True or False. Justify each answer. (If true, cite appropriate facts or theorems. If false, explain why or give a counterexample that shows why the statement is not true in every case.) In parts (a)-(f), v1, ..., vp are
- a. The set of all linear combinations of v1, ..., vp is a vector space.
- b. If {v1, ..., vp−1} spans V, then S spans V.
- c. If {v1, ..., vp−1} is linearly independent, then so is S.
- d. If S is linearly independent, then S is a basis for V.
- e. If Span S = V, then some subset of S is a basis for V.
- f. If dim V = p and Span, S = V, then S cannot be linearly dependent.
- g. A plane in ℝ3 is a two-dimensional subspace.
- h. The nonpivot columns of a matrix are always linearly dependent.
- i. Row operations on a matrix A can change the linear dependence relations among the rows of A.
- j. Row operations on a matrix can change the null space.
- k. The rank of a matrix equals the number of nonzero rows.
- l. If an m × n matrix A is row equivalent to an echelon matrix U and if U has k nonzero rows, then the dimension of the solution space of Ax = 0 is m − k.
- m. If B is obtained from a matrix A by several elementary row operations, then rank B = rank A.
- n. The nonzero rows of a matrix A form a basis for Row A.
- ○. If matrices A and B have the same reduced echelon form, then Row A = Row B.
- p. If H is a subspace of ℝ3, then there is a 3 × 3 matrix A such that H = Col A.
- q. If A is m × n and rank A = m, then the linear transformation x ↦ Ax is one-to-one.
- r. If A is m × n and the linear transformation x ↦ Ax is onto, then rank A = m.
- s. A change-of-coordinates matrix is always invertible.
- t. If B = {b1, ..., bn} and C = {c1, ..., cn} are bases for a vector space V, then the jth column of the change-of-coordinates matrix
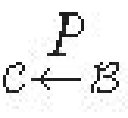 is the coordinate vector [cj]B.
is the coordinate vector [cj]B.
a.
To find: Whether the statement “The set of all linear combinations of
Answer to Problem 1SE
The statement is true.
Explanation of Solution
Here, the given vectors are
The span {
Thus, the linear combinations of
Hence, the statement is true.
b.
To find: Whether the statement “If
Answer to Problem 1SE
The statement is true.
Explanation of Solution
The set S is
It is given that the set
That is, every element in the vector space
Here, the set
The smaller set
If the vector space V is spanned by
Hence, the statement is true.
c.
To find: Whether the statement “If
Answer to Problem 1SE
The statement is false.
Explanation of Solution
If
Therefore, it does not imply that S is linearly independent.
Hence, the statement is false.
d.
To find: Whether the statement “If S is linearly independent then S is a basis for V” is true or false.
Answer to Problem 1SE
The statement is false.
Explanation of Solution
A set of vectors
1. The set of vectors
2. The set
It can be seen that the second condition is not satisfied.
Hence, the statement is false.
e.
To find: Whether the statement “If
Answer to Problem 1SE
The statement is true.
Explanation of Solution
It is given that the vector space V is spanned by S, which is nonzero set.
Suppose the set S is linearly independent then, the set S form a basis for V.
Suppose the set S is linearly dependent then, some subset of S linearly independent and which spans V.
That is, some subset of S form a basis for V.
Hence, the statement is true.
f.
To find: Whether the statement “If
Answer to Problem 1SE
The statement is true.
Explanation of Solution
It is given that
Here,
Which implies the
Hence, the statement is false.
g.
To find: Whether the statement “A plane in
Answer to Problem 1SE
The statement is false.
Explanation of Solution
Every plane in
Sometimes plane in
Hence, the statement is false.
h.
To find: Whether the statement “The non-pivot columns of a matrix are always linearly dependent” is true or false.
Answer to Problem 1SE
The statement is false.
Explanation of Solution
Consider the matrix
Here non-pivot columns are linearly independent.
Hence, the statement is false.
i.
To find: Whether the statement “Row operations on a matrix A can change the linear dependence relations among the rows of A” is true or false.
Answer to Problem 1SE
The statement is true.
Explanation of Solution
Row operations on matrix
Hence, the statement is true.
j.
To find: Whether the statement “Row operations on a matrix can change the null space” is true or false.
Answer to Problem 1SE
The statement is false.
Explanation of Solution
Row operations do not change the solution set of the system
Therefore, row operations do not change the null space.
Hence, the statement is false.
k.
To find: Whether the statement “The rank of a matrix equals the number of nonzero rows” is true or false.
Answer to Problem 1SE
The statement is false.
Explanation of Solution
The rank of a matrix A is the dimension of the column space of A.
The dimension of column space of A is the number of pivot columns in A.
Therefore, the rank of matrix equals the number of pivot columns.
Consider the matrix
The above matrix has 2 rows but rank of the matrix is 1.
Hence, the statement is false.
l.
To find: Whether the statement “If an
Answer to Problem 1SE
The statement is false.
Explanation of Solution
If U has k nonzero rows then,
According to the Rank Theorem, the rank of an
Hence, the statement is false.
m.
To find: Whether the statement “If B is obtained from a matrix A by several elementary row operations, then
Answer to Problem 1SE
The statement is true.
Explanation of Solution
Elementary row operations does not change the number of pivot columns and hence, does not change the rank of a matrix.
Therefore, the rank of matrix B will be same as the rank of matrix A.
Hence, the statement is true.
n.
To find: Whether the statement “The nonzero rows of a matrix A form a basis for Row A” is true or false.
Answer to Problem 1SE
The statement is false.
Explanation of Solution
To form a basis for A, the rows have to span A and should also be linearly independent.
The nonzero rows of a matrix A span Row A but that does not guarantee that they are linearly independent.
Hence, the statement is false.
o.
To find: Whether the statement “If matrices A and B have the same reduced echelon form, then
Answer to Problem 1SE
The statement is true.
Explanation of Solution
The nonzero rows of the echelon form of a matrix, form a basis for the row space of that matrix.
If the echelon form for two matrices is same, then the basis for the row spaces is also same.
Since, row spaces are vector spaces and if two vector spaces have same basis, then the vector spaces are same.
Hence, the statement is true.
p.
To find: Whether the statement “If H is a subspace of
Answer to Problem 1SE
The statement is true.
Explanation of Solution
If H is a zero, 1, 2, or 3 dimensional subspace of
The basis of H will then be in the column space of A.
Therefore,
Hence, the statement is true.
q.
To find: Whether the statement “If A is
Answer to Problem 1SE
The statement is false.
Explanation of Solution
Here the matrix A is linear transformation from
The transformation
Here, rank of the matrix is A thus, by the rank nullity theorem null space of
Hence, the statement is false.
r.
To find: Whether the statement “If A is
Answer to Problem 1SE
The statement is true.
Explanation of Solution
Here the matrix A is linear transformation from
If the transformation is onto then,
The rank of a matrix A is the dimension of the column space of A.
Therefore, the rank of A is m.
Hence, the statement is true.
s.
To find: Whether the statement “A change-of-coordinate matrix is always invertible” is true or false.
Answer to Problem 1SE
The statement is true.
Explanation of Solution
The columns of
The matrix
Hence, the statement is true.
t.
To find: Whether the statement “If
Answer to Problem 1SE
The statement is false.
Explanation of Solution
The jth column of the change-of-coordinates matrix
Hence, the statement is false.
Want to see more full solutions like this?
Chapter 4 Solutions
Linear Algebra and Its Applications (5th Edition)
Additional Math Textbook Solutions
Thinking Mathematically (6th Edition)
Pre-Algebra Student Edition
Elementary Statistics
University Calculus: Early Transcendentals (4th Edition)
Calculus: Early Transcendentals (2nd Edition)
- Problem #1 In the 2010 census, it was reported that the United States had a population of 310 million people. Of those, 74 million were under the age of 18. If you chose a person from the U.S. population at random, what is the probability they are under the age of 18? Problem #2 Given a set S={1, 2, 3, 4, 5, 6, 7, 8, 9, 10}, if you were choosing a number at random what is the probability that you would choose an even number or a number larger than 7?arrow_forwardComplete the table below. For solutions, round to the nearest whole number.arrow_forwardLet the universal set be whole numbers 1 through 20 inclusive. That is, U = {1, 2, 3, 4, . . ., 19, 20}. Let A, B, and C be subsets of U. Let A be the set of all prime numbers: A = {2, 3, 5, 7, 11, 13, 17, 19} Let B be the set of all odd numbers: B = {1,3,5,7, . . ., 17, 19} Let C be the set of all square numbers: C = {1,4,9,16}arrow_forward
- A research team consists of 4 senior researchers and 10 research assistants. The team needs to select 2 senior researchers and 2 research assistants to attend a conference. How many different ways can the group being sent to the conference be formed?arrow_forwardThere are 25 different varieties of flowering plants found in a natural habitat you are studying. You are asked to randomly select 5 of these flowering plant varieties to bring back to your laboratory for further study. How many different combinations of are possible? That is, how many possible 5 plant subgroups can be formed out of the 25 total plants found?arrow_forwardA person is tossing a fair, two-sided coin three times and recording the results (either a Heads, H, or a Tails, T). Let E be the event that exactly two heads are tossed. Which of the following sets represent the event E? Group of answer choices {HHT, HTH, THH} {HHT, THH} {HHH, HHT, HTH, THH, TTT, TTH, THT, HTT} {HH}arrow_forward
- Take Quiz 54m Exit Let the universal set be whole numbers 1 through 20 inclusive. That is, U = {1, 2, 3, 4, . . ., 19, 20}. Let A, B, and C be subsets of U. Let A be the set of all prime numbers: A = {2, 3, 5, 7, 11, 13, 17, 19} Let B be the set of all odd numbers: B = {1,3,5,7, • • , 17, 19} Let C be the set of all square numbers: C = {1,4,9,16} ☐ Question 2 3 pts Which of the following statement(s) is true? Select all that apply. (1) АСВ (2) A and C are disjoint (mutually exclusive) sets. (3) |B| = n(B) = 10 (4) All of the elements in AC are even numbers. ☐ Statement 1 is true. Statement 2 is true. Statement 3 is true. Statement 4 is true.arrow_forward☐ Question 1 2 pts Let G be the set that represents all whole numbers between 5 and 12 exclusive. Which of the following is set G in standard set notation. (Roster Method)? O G = [5, 12] G = {5, 6, 7, 8, 9, 10, 11, 12} O G = (5, 12) OG = {6, 7, 8, 9, 10, 11}arrow_forwardSolve thisarrow_forward
- int/PlayerHomework.aspx?homeworkId=689099898&questionId=1&flushed=false&cid=8120746¢erw BP Physical Geograph... HW Score: 0%, 0 of 13 points ○ Points: 0 of 1 Determine if the values of the variables listed are solutions of the system of equations. 2x - y = 4 3x+5y= - 6 x=1, y = 2; (1,-2) Is (1, 2) a solution of the system of equations? L No Yes iew an example Get more help - Aarrow_forward12:01 PM Tue May 13 < AA ✓ Educatic S s3.amazona... A Assess Your... 目 accelerate-iu15-bssd.vschool.com S s3.amazona... Trigonometric Identities Module Exam Dashboard ... Dashboard ... Algebra 2 Pa... Algebra 2 Part 4 [Honors] (Acc. Ed.) (Zimmerman) 24-25 / Module 11: Trigonometric Identities i + 38% ✰ Start Page Alexis Forsythe All changes saved 10. A sound wave's amplitude can be modeled by the function y = −7 sin ((x-1) + 4). Within the interval 0 < x < 12, when does the function have an amplitude of 4? (Select all that apply.) 9.522 seconds 4.199 seconds 0.522 seconds 1.199 seconds Previous 10 of 20 Nextarrow_forwardJamal wants to save $48,000 for a down payment on a home. How much will he need to invest in an account with 11.8% APR, compounding daily, in order to reach his goal in 10 years? Round to the nearest dollar.arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning  Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning



