
Interpretation:
We are asked to plot the potentials of the non-linear differential equations of three different kinds of bifurcations.
a) Saddle node bifurcation
b) Transcritical bifurcation
c) Subcritical pitchfork bifurcation.
Concept Introduction:
Bifurcations and Bifurcation points - The qualitative structure of the flow can change as parameters are varied. In particular, fixed points can be created or destroyed, or their stability canchange. These qualitative changes in the dynamics are calledbifurcations, and the parameter values at which they occur are called bifurcation points.
Saddle node bifurcation is a mechanism by which the fixed points move toward each other and eventually annihilate.
Pitchfork bifurcation is the bifurcation mainly seen in symmetrical physical problems. Stable or unstable fixed points appear and disappear in pairs in such cases.
Problems in which pair of stable symmetric fixed points emerge are called subcritical pitchfork bifurcation.
Problems in which pair of unstable symmetric fixed points emerge are called supercritical pitchfork bifurcation.
In some physical systems, there exists a stable point irrespective of parameter values. (Either r < 0, r = 0 or r > 0) such cases are called transcritical bifurcation.
Answer to Problem 16E
Solution:
Plots for potentials of equations a, b and c with their different r values are plotted below.
Explanation of Solution
(a) We have,
To find the potential
By integrating the above equation,
Let’s take,
To find the critical points, substitute
These are the fixed point of the potential function.
If r > 0, there are two fixed points, one stable and another unstable as the value of r decreases further, both points approach towards each other. At r = 0, both fixed points collide and annihilate after r <0 thus r = 0 issaddle-node bifurcationpoint.
Potential V(x) for different values of r is plotted below.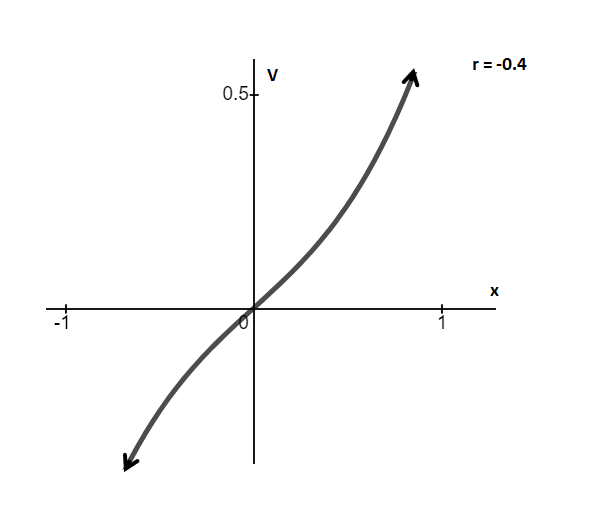
V vs. x for r = 0
V vs. x for r > 0
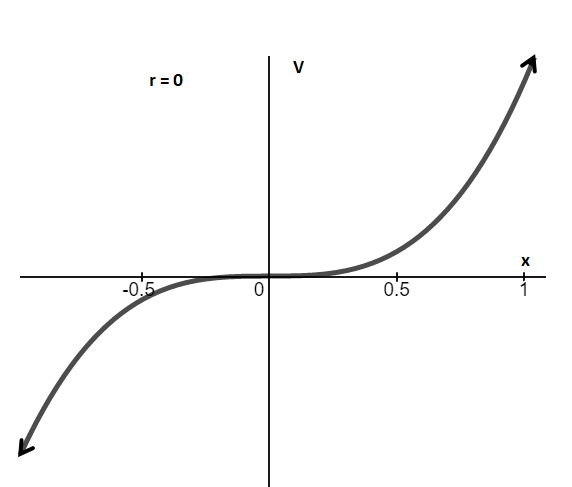
(b) The first-order system equation is
To calculate the potential V(x) for the system let us use,
Integrating,
To find the critical points, substitute 0 for
As we can see, out of two fixed points, one point always lies on the origin and as parameter changes its value, fixed points change their stability.
Therefore, at r = 0, transcritical bifurcation occurs.
Potentials for different r valued are plotted below.
Graph between V and x for r < 0 (r = -1)
Graph between V and x for r = 0
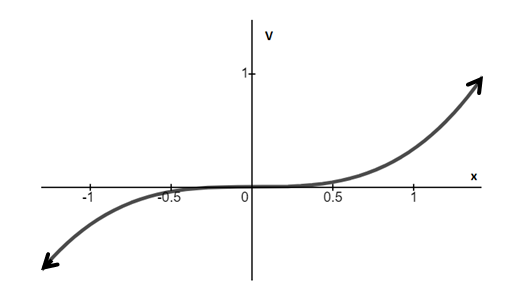
The graph between V and x for r>0
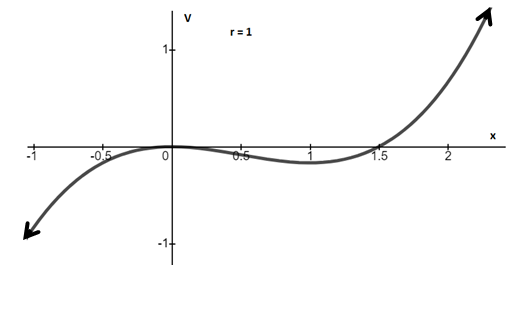
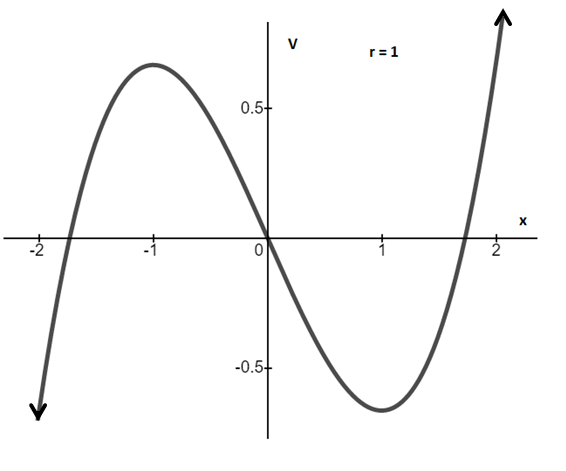
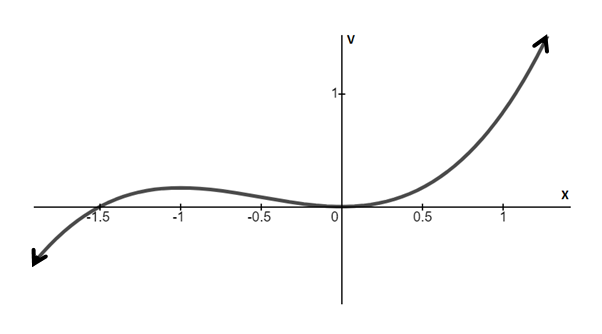
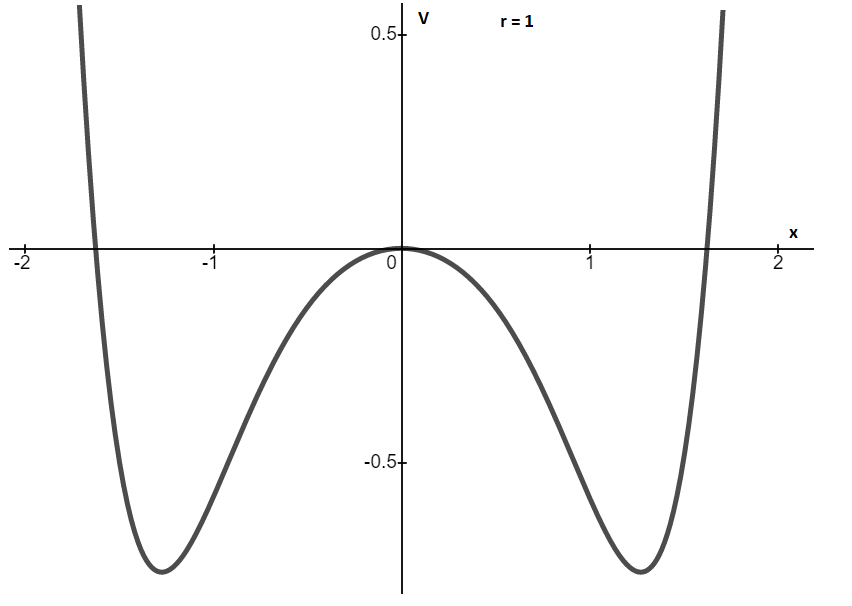
(c)
We have the equation of the system,
To calculate the potential
Integrating,
To find the critical points, substitute
And
Others fixed points are:
Obtain the second differentiation of the potential function,
Local minima occur when,
Check out at differential points for the local minima as,
Similarly,
Therefore, three critical points wells occur at
For simplicity, take
Substitute
Further, solve for r,
Here,
Hence,
Graphs of potential vs. position x are plotted below.
Graph between V and x for r<0
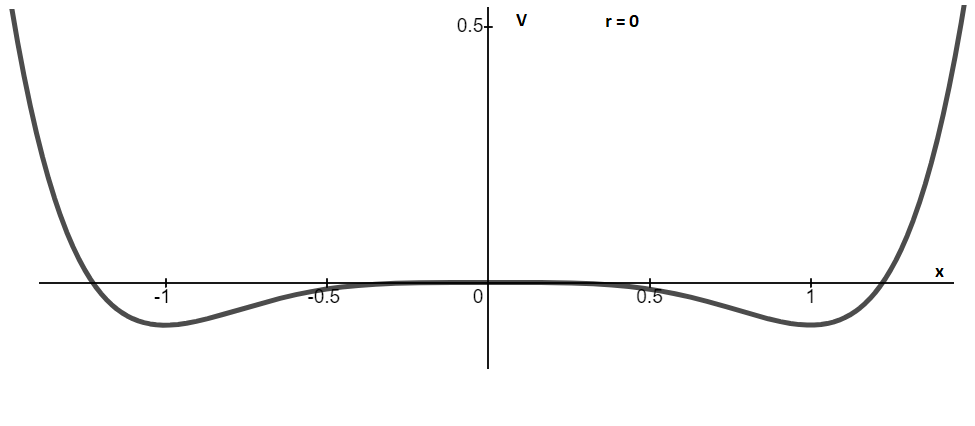
Graph between V and x for r=0
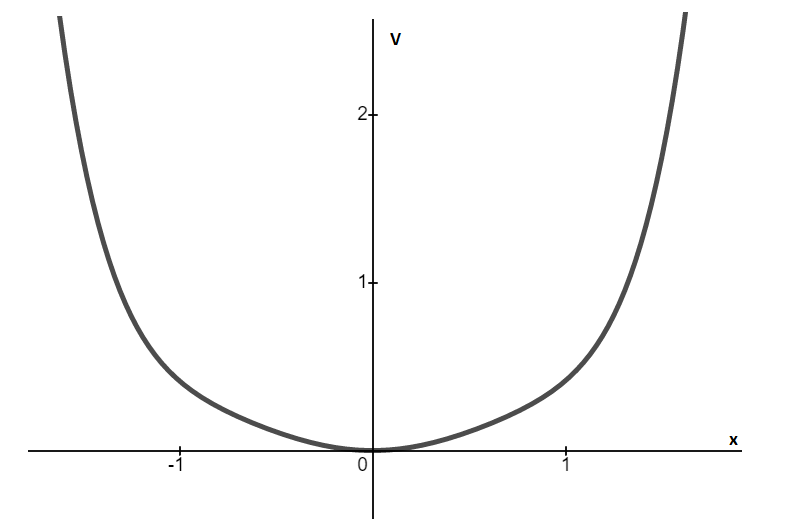
Graph between V and x for r>0
Want to see more full solutions like this?
Chapter 3 Solutions
EBK NONLINEAR DYNAMICS AND CHAOS WITH S
- Q/ Find the Laurent series of (2-3) cos around z = 1 2-1arrow_forward31.5. Let be the circle |+1| = 2 traversed twice in the clockwise direction. Evaluate dz (22 + 2)²arrow_forwardUsing FDF, BDF, and CDF, find the first derivative; 1. The distance x of a runner from a fixed point is measured (in meters) at an interval of half a second. The data obtained is: t 0 x 0 0.5 3.65 1.0 1.5 2.0 6.80 9.90 12.15 Use CDF to approximate the runner's velocity at times t = 0.5s and t = 1.5s 2. Using FDF, BDF, and CDF, find the first derivative of f(x)=x Inx for an input of 2 assuming a step size of 1. Calculate using Analytical Solution and Absolute Relative Error: = True Value - Approximate Value| x100 True Value 3. Given the data below where f(x) sin (3x), estimate f(1.5) using Langrage Interpolation. x 1 1.3 1.6 1.9 2.2 f(x) 0.14 -0.69 -0.99 -0.55 0.31 4. The vertical distance covered by a rocket from t=8 to t=30 seconds is given by: 30 x = Loo (2000ln 140000 140000 - 2100 9.8t) dt Using the Trapezoidal Rule, n=2, find the distance covered. 5. Use Simpson's 1/3 and 3/8 Rule to approximate for sin x dx. Compare the results for n=4 and n=8arrow_forward
- 1. A Blue Whale's resting heart rate has period that happens to be approximately equal to 2π. A typical ECG of a whale's heartbeat over one period may be approximated by the function, f(x) = 0.005x4 2 0.005x³-0.364x² + 1.27x on the interval [0, 27]. Find an nth-order Fourier approximation to the Blue Whale's heartbeat, where n ≥ 3 is different from that used in any other posts on this topic, to generate a periodic function that can be used to model its heartbeat, and graph your result. Be sure to include your chosen value of n in your Subject Heading.arrow_forward7. The demand for a product, in dollars, is p = D(x) = 1000 -0.5 -0.0002x² 1 Find the consumer surplus when the sales level is 200. [Hints: Let pm be the market price when xm units of product are sold. Then the consumer surplus can be calculated by foam (D(x) — pm) dx]arrow_forward4. Find the general solution and the definite solution for the following differential equations: (a) +10y=15, y(0) = 0; (b) 2 + 4y = 6, y(0) =arrow_forward
- 5. Find the solution to each of the following by using an appropriate formula developed in the lecture slides: (a) + 3y = 2, y(0) = 4; (b) dy - 7y = 7, y(0) = 7; (c) 3d+6y= 5, y(0) = 0arrow_forward1. Evaluate the following improper integrals: (a) fe-rt dt; (b) fert dt; (c) fi da dxarrow_forward8. Given the rate of net investment I(t) = 9t¹/2, find the level of capital formation in (i) 16 years and (ii) between the 4th and the 8th years.arrow_forward
- 9. If the marginal revenue function of a firm in the production of output is MR = 40 - 10q² where q is the level of output, and total revenue is 120 at 3 units of output, find the total revenue function. [Hints: TR = √ MRdq]arrow_forward6. Solve the following first-order linear differential equations; if an initial condition is given, definitize the arbitrary constant: (a) 2 + 12y + 2et = 0, y(0) = /; (b) dy+y=tarrow_forward4. Let A = {a, b, c, d, e, f}, B = {e, f, g, h} and C = {a, e, h,i}. Let U = {a, b, c, d, e, f, g, h, i, j, k}. • Draw a Venn Diagram to describe the relationships between these sets Find (AB) NC • Find (AC) UB Find AUBUC • Find (BC) N (A - C)arrow_forward
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,






