Chapter 31, Problem 25SP

Two long fixed parallel wires, A and B, are 10 cm apart in air and carry 40 A and 20 A, respectively, in opposite directions. Determine the resultant field (a) on a line midway between the wires and parallel to them and (b) on a line 8.0 cm from wire A and 18 cm from wire B. (c) What is the force per meter on a third long wire, midway between A and B and in their plane, when it carries a current of 5.0 A in the same direction as the current in A?

To determine

The magnetic field at the line midway between the parallel wires $A$ and $B$, which are $10\text{ cm}$ apart in the air and carry40 A and 20 A in opposite directions.

Answer to Problem 25SP

Solution:

$2.4\times {10}^{-4}\text{ T}$

Explanation of Solution

Given data:

The distance between the wires $A$ and $B$ is $10\text{ cm}$.

The current flowing in wire $A$ is $40\text{ A}$.

The current flowing in wire $B$ is $20\text{ A}$.

The direction of current in the wires is opposite to each other.

Formula used:

The expression for the magnetic field due to the long straight wire is written as

$B=\frac{{\mu }_0}{2\pi }\left(\frac{I}{r}\right)$

Here, $I$ is the current flowing in a wire, $r$ is the distance of the point at which field is to calculate from the wire, and ${\mu }_0$ is the magnetic permeability of vacuum.

The right-hand thumb rule states that if the thumb shows the direction of the current, then the curled fingers show the direction of the magnetic field. Pictorially, this is expressed as

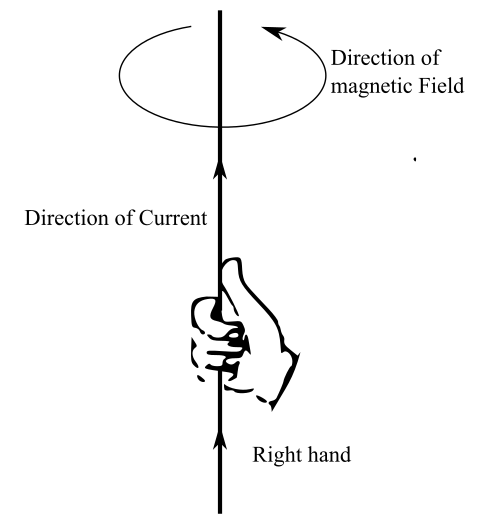
Explanation:

Draw the diagram according to the problem:

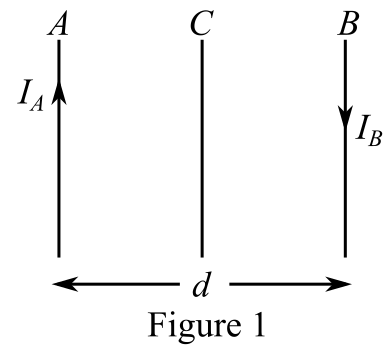
Here, $I_A$ is the current flowing in wire $A$, $I_B$ is the current flowing in wire $B$, $d$ is the distance between wires $A$ and $B$, and wire $C$ is midway between the wires $A$ and $B$. Assume the direction of current in wire $A$ is in the upward direction and that in wire $B$ is in the downward direction.

Now, write the expression for magnetic field around wire $C$ due to wire $A$:

$B_{CA}=\frac{{\mu }_0}{2\pi }\left(\frac{I_A}{r}\right)$

Here, $r$ is the distance between wires $A$ and $C$.

Substitute $d/2$ for r

$B_{CA}=\frac{{\mu }_0}{2\pi }\left(\frac{I_A}{d/2}\right)$

Understand that wire $C$ is midway between wires $A$ and $B$. So, the distance of wire $C$ from wire $A$ will be half of the distance between $A$ and $B$.

Substitute $40.0\text{ A}$ for $I_A$, $4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}$ for ${\mu }_0$, and $10\text{ cm}$ for $d$

$\begin{array}{c}{B}_{CA}=\frac{\left(4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}\right)}{2\pi }\left(\frac{40.0\text{ A}}{10\text{ cm}/2}\right)\\ =\left(2\times {10}^{-7}\text{ T}\cdot \text{m/A}\right)\left[\frac{40.0\text{ A}}{\left(5\text{ cm}\right)\left(\frac{{10}^{-2}\text{ m}}{1\text{ cm}}\right)}\right]\\ =1.6\times {10}^{-4}\text{ T}\end{array}$

The direction of the magnetic field around wire $C$ due to wire $A$ will be into the page according to the right-hand thumb rule.

Write the expression for magnetic field around wire $C$ due to wire $B$:

$B_{CB}=\frac{{\mu }_0}{2\pi }\left(\frac{I_B}{r}\right)$

Here, $r$ is the distance between wires $B$ and $C$.

Substitute $d/2$ for r

$B_{CB}=\frac{{\mu }_0}{2\pi }\left(\frac{I_B}{d/2}\right)$

Understand that wire $C$ is midway between wires $A$ and $B$. So, the distance of wire $C$ from $B$ will be half of the distance between $A$ and $B$.

Substitute $20.0\text{ A}$ for $I_B$, $4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}$ for ${\mu }_0$, and $10\text{ cm}$ for $d$

$\begin{array}{c}{B}_{CB}=\frac{\left(4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}\right)}{2\pi }\left(\frac{20.0\text{ A}}{10\text{ cm}/2}\right)\\ =\left(2\times {10}^{-7}\text{ T}\cdot \text{m/A}\right)\left[\frac{20.0\text{ A}}{\left(5\text{ cm}\right)\left(\frac{{10}^{-2}\text{ m}}{1\text{ cm}}\right)}\right]\\ =0.8\times {10}^{-4}\text{ T}\end{array}$

The direction of the magnetic field around wire $C$ due to wire $B$ will be into the page according to the right-hand thumb rule.

Write the expression for the total magnetic field aroundwire $C$:

$B=B_{CA}+B_{CB}$

Substitute $1.6\times {10}^{-4}\text{ T}$ for $B_{CA}$ and $0.8\times {10}^{-4}\text{ T}$ for $B_{CB}$

$\begin{array}{c}B=\left(1.6\times {10}^{-4}\text{ T}\right)+\left(0.8\times {10}^{-4}\text{ T}\right)\\ =2.4\times {10}^{-4}\text{ T}\end{array}$

Understand that the direction of the magnetic field at wire $C$ due to wires $A$ and $B$ is in same direction. So, the expression for total magnetic field will be $B=B_{CA}+B_{CB}$.

Conclusion:

Hence, the magnetic field around the line midway between the parallel wires $A$ and $B$ is $2.4\times {10}^{-4}\text{ T}$

To determine

The magnetic field ona line $8.0\text{ cm}$ away from wire $A$ and $18\text{ cm}$ away from wire $B$ if the wires $A$ and $B$ are $10\text{ cm}$ apart in the air and carry40 A and 20 A in opposite directions.

Answer to Problem 25SP

Solution:

$7.8\times {10}^{-5}\text{ T}$

Explanation of Solution

Given data:

The current flowing in wire $A$ is $40\text{ A}$.

The current flowing in wire $B$ is $20\text{ A}$.

The line is $8.0\text{ cm}$ away from wire $A$.

The line is $\text{18 cm}$ away from wire $B$.

The direction of current in the wires is opposite to each other.

Formula used:

The expression for the magnetic field due to the long straight wire is written as

$B=\frac{{\mu }_0}{2\pi }\left(\frac{I}{r}\right)$

Here, $I$ is the current flowing in a wire, $r$ is the distance of the point at which field is to calculate from the wire, and ${\mu }_0$ is the magnetic permeability of vacuum.

The right-hand thumb rule states that if the thumb shows the direction of the current, then the curled fingers show the direction of the magnetic field. Pictorially, this is expressed as

Explanation:

Draw the diagram according to the problem:

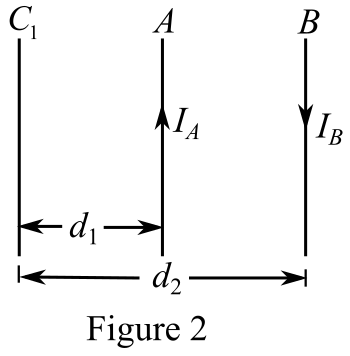
Here, wire $C_1$ is at distance $d_1$ from wire $A$ and distance $d_2$ from wire $B$. Assume the direction of current in wire $A$ in the upward direction and that in wire $B$ in the downward direction.

Now, write the expression for the magnetic field at wire $C_1$ due to wire $A$:

$B_{C_{1}A}=\frac{{\mu }_0}{2\pi }\left(\frac{I_A}{d_1}\right)$

Substitute $40.0\text{ A}$ for $I_A$, $4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}$ for ${\mu }_0$, and $\text{8}\text{.0 cm}$ for $d_1$

$\begin{array}{c}{B}_{C_{1}A}=\frac{\left(4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}\right)}{2\pi }\left(\frac{40.0\text{ A}}{\text{8}\text{.0 cm}}\right)\\ =\left(2\times {10}^{-7}\text{ T}\cdot \text{m/A}\right)\left[\frac{40.0\text{ A}}{\left(\text{8}\text{.0 cm}\right)\left(\frac{{10}^{-2}\text{ m}}{1\text{ cm}}\right)}\right]\\ =10\times {10}^{-5}\text{ T}\end{array}$

The direction of the magnetic field around wire $C_1$ due to wire $A$ will be out of the page according to the right-hand thumb rule.

Write the expression for the magnetic field around wire $C_1$ due to wire $B$:

$B_{C_{1}B}=\frac{{\mu }_0}{2\pi }\left(\frac{I_B}{d_2}\right)$

Substitute $20.0\text{ A}$ for $I_B$, $4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}$ for ${\mu }_0$, and $18\text{ cm}$ for $d_2$

$\begin{array}{c}{B}_{C_{1}B}=\frac{\left(4\pi \times {10}^{-7}\text{ T}\cdot \text{m/A}\right)}{2\pi }\left(\frac{20.0\text{ A}}{18\text{ cm}}\right)\\ =\left(2\times {10}^{-7}\text{ T}\cdot \text{m/A}\right)\left[\frac{20.0\text{ A}}{\left(\text{18 cm}\right)\left(\frac{{10}^{-2}\text{ m}}{1\text{ cm}}\right)}\right]\\ =2.2\times {10}^{-5}\text{ T}\end{array}$

The direction of the magnetic field around wire $C_1$ due to wire $B$ will be into the page according to the right-hand thumb rule.

Write the expression for the total magnetic field aroundwire $C_1$:

$B=B_{C_{1}A}-B_{C_{1}B}$

Substitute $10\times {10}^{-5}\text{ T}$ for $B_{C_{1}A}$ and $2.2\times {10}^{-5}\text{ T}$ for $B_{C_{1}B}$

$\begin{array}{c}B=\left(10\times {10}^{-5}\text{ T}\right)-\left(2.2\times {10}^{-5}\text{ T}\right)\\ =7.8\times {10}^{-5}\text{ T}\end{array}$

Understand that the direction of the magnetic field around wire $C_1$ due to wire $A$ and $B$ is in the opposite direction. So, the expression for total magnetic field will be $B=B_{C_{1}A}-B_{C_{1}B}$.

Conclusion:

Hence, the magnetic field around a line between the wires $A$ and $B$, which is $8.0\text{ cm}$ away from wire $A$ and $18\text{ cm}$ away from wire $B$, is $7.8\times {10}^{-5}\text{ T}$.

To determine

The force per meter on the third wire midway between wires $A$ and $B$ when it carries a $5.0\text{ A}$ current.

Answer to Problem 25SP

Solution:

$1.2\times {10}^{-3}\text{ N/m}$ , toward wire $A$.

Explanation of Solution

Given data:

The current flowing in wire $A$ is $40\text{ A}$.

The current flowing in wire $B$ is $20\text{ A}$.

The direction of current in the wires is opposite to each other.

The current in third wire is $5.0\text{ A}$.

Formula used:

The expression for the force acting on a wire due to magnetic field is written as

$F=BIL\sin \theta$

Here, $B$ is the magnetic field, $I$ is the current flowing in the wire, $L$ is the length of the wire, and $\theta$ is the angle between magnetic field and the direction of current.

Explanation:

Draw the diagram according to the problem:

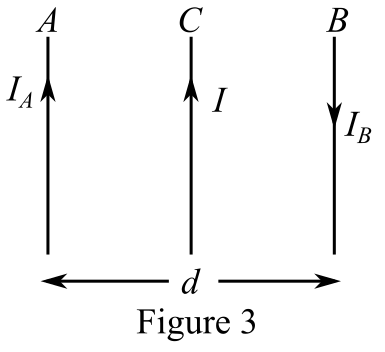
Recall the expression for the force acting on a wire due to magnetic field:

$F=BIL\sin \theta$

Rearrange the expression for $\frac{F}{L}$

$\frac{F}{L}=BI\sin \theta$

Recall the value of the calculated magnetic field from subpart (a) and substitute $90°$ for $\theta$, $5.0\text{ A}$ for $I$, and $2.4\times {10}^{-4}\text{ T}$ for $B$

$\begin{array}{c}\frac{F}{L}=\left(2.4\times {10}^{-4}\text{ T}\right)\left(5.0\text{ A}\right)\sin 90°\\ =1.2\times {10}^{-3}\text{ N/m}\end{array}$

Using the right-hand rule, wire $C$ tells that the force on it will be toward wire $A$.

Conclusion:

Hence, the force per meter on the third wire midway between wire $A$ and $B$ when it carries a $5.0\text{ A}$ current is $1.2\times {10}^{-3}\text{ N/m}$ toward wire $A$.