Principles Of Taxation For Business And Investment Planning 2020 Edition
23rd Edition
ISBN: 9781259969546
Author: Sally Jones, Shelley C. Rhoades-Catanach, Sandra R Callaghan
Publisher: McGraw-Hill Education
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 3, Problem 17AP
Investor W has the opportunity to invest $500,000 in a new venture. The projected
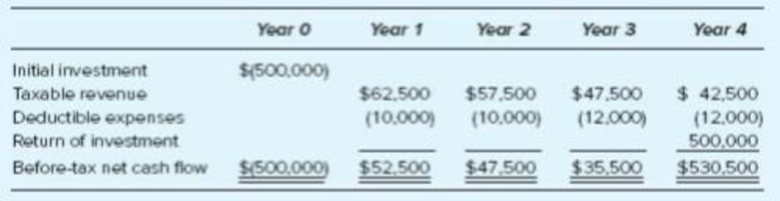
Investor W uses a 7 percent discount rate to compute
- a. Her marginal tax rate over the life of the investment is 15 percent.
- b. Her marginal tax rate over the life of the investment is 20 percent.
- c. Her marginal tax rate in years 1 and 2 is 10 percent and in years 3 and 4 is 25 percent.
a.
Expert Solution
To determine
Calculate net present value (NPV) and identify whether Investor W should make the investment or not.
Explanation of Solution
Calculate net present value (NPV) and identify whether Investor W should make the investment or not.
Step 1: Calculate present value.
| Year 0 | Year 1 | Year 2 | Year 3 | Year 4 | |
| Before-tax cash flow | $(500,000) | $52,500 | $47,500 | $35,500 | $530,500 |
| Less: Tax cost | - | $(7,875) | $(7,125) | $(5,325) | $(4,575) |
| After-tax cash flow | - | $44,625 | $40,375 | $30,175 | $525,925 |
| Multiply: Discount factor at 7% | - | ||||
| Present value | $(500,000) | $41,724 | $35,247 | $24,623 | $401,281 |
Working note:
Calculate tax cost.
| Year 1 | Year 2 | Year 3 | Year 4 | |
| Taxable income | $52,500 | $47,500 | $35,500 | $30,500 |
| Multiply: Marginal tax rate | ||||
| Tax cost | $7,875 | $7,125 | $5,325 | $4,575 |
Step 2: Calculate NPV.
The value of net present value (NPV) is positive. Hence, Investor W should make the investment.
b.
Expert Solution
To determine
Calculate net present value (NPV) and identify whether Investor W should make the investment or not.
Explanation of Solution
Calculate net present value (NPV) and identify whether Investor W should make the investment or not.
Step 1: Calculate present value.
| Year 0 | Year 1 | Year 2 | Year 3 | Year 4 | |
| Before-tax cash flow | $(500,000) | $52,500 | $47,500 | $35,500 | $530,500 |
| Less: Tax cost | - | $(10,500) | $(9,500) | $(7,100) | $(6,100) |
| After-tax cash flow | - | $42,000 | $38,000 | $28,400 | $524,400 |
| Multiply: Discount factor at 7% | - | ||||
| Present value | $(500,000) | $39,270 | $33,174 | $23,174 | $400,117 |
Working note:
Calculate tax cost.
| Year 1 | Year 2 | Year 3 | Year 4 | |
| Taxable income | $52,500 | $47,500 | $35,500 | $30,500 |
| Multiply: Marginal tax rate | ||||
| Tax cost | $10,500 | $9,500 | $7,100 | $6,100 |
Step 2: Calculate NPV.
The value of net present value (NPV) is negative. Hence, Investor W should not make the investment.
c.
Expert Solution
To determine
Calculate net present value (NPV) and identify whether Investor W should make the investment or not.
Explanation of Solution
Calculate net present value (NPV) and identify whether Investor W should make the investment or not.
Step 1: Calculate present value.
| Year 0 | Year 1 | Year 2 | Year 3 | Year 4 | |
| Before-tax cash flow | $(500,000) | $52,500 | $47,500 | $35,500 | $530,500 |
| Less: Tax cost | - | $(5,250) | $(4,750) | $(8,875) | $(7,625) |
| After-tax cash flow | - | $47,250 | $42,750 | $26,625 | $522,875 |
| Multiply: Discount factor at 7% | - | ||||
| Present value | $(500,000) | $44,179 | $37,321 | $21,726 | $398,954 |
Working note:
Calculate tax cost.
| Year 1 | Year 2 | Year 3 | Year 4 | |
| Taxable income | $52,500 | $47,500 | $35,500 | $30,500 |
| Multiply: Marginal tax rate | ||||
| Tax cost | $5,250 | $4,750 | $8,875 | $7,625 |
Step 2: Calculate NPV.
The value of net present value (NPV) is positive. Hence, Investor W should make the investment.
Want to see more full solutions like this?
Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!
Students have asked these similar questions
Zachary has purchased an investment that he expects to produce income of $3,000 at the end of the first year and $4,000 at the end of the second year. If he pays
$5,800 for this investment, what is the internal rate of return?
Komiko Tanaka invests $14,500 in LymaBean, Incorporated. LymaBean does not pay any dividends. Komiko projects that her investment will generate a 10 percent before-tax rate of return. She plans to invest for the long term.
C. How much cash will Komiko retain, after taxes, if she holds the investment for five years and then sells when the long-term capital gains rate is 25 percent?
D. What is Komiko's after-tax rate of return on her investment in part (c)?
Please step by step answer.
Chapter 3 Solutions
Principles Of Taxation For Business And Investment Planning 2020 Edition
Ch. 3 - Does the NPV of future cash flows increase or...Ch. 3 - Explain the relationship between the degree of...Ch. 3 - Does the after-tax cost of a deductible expense...Ch. 3 - Prob. 4QPDCh. 3 - Prob. 5QPDCh. 3 - Prob. 6QPDCh. 3 - Prob. 7QPDCh. 3 - Which type of tax law provision should be more...Ch. 3 - In the U.S. system of criminal justice, a person...Ch. 3 - Identify two reasons why a firms actual marginal...
Ch. 3 - Prob. 11QPDCh. 3 - Prob. 12QPDCh. 3 - Prob. 1APCh. 3 - Prob. 2APCh. 3 - Prob. 3APCh. 3 - Use a 5 percent discount rate to compute the NPV...Ch. 3 - Consider the following opportunities: Opportunity...Ch. 3 - Prob. 6APCh. 3 - Refer to the income tax rate structure in the...Ch. 3 - Prob. 8APCh. 3 - Company N will receive 100,000 of taxable revenue...Ch. 3 - Prob. 10APCh. 3 - Investor B has 100,000 in an investment paying 9...Ch. 3 - Firm E must choose between two alternative...Ch. 3 - Company J must choose between two alternate...Ch. 3 - Firm Q is about to engage in a transaction with...Ch. 3 - Corporation ABC invested in a project that will...Ch. 3 - Prob. 16APCh. 3 - Investor W has the opportunity to invest 500,000...Ch. 3 - Prob. 18APCh. 3 - Prob. 19APCh. 3 - Prob. 20APCh. 3 - Prob. 21APCh. 3 - Prob. 1IRPCh. 3 - Firm V must choose between two alternative...Ch. 3 - Prob. 3IRPCh. 3 - Refer to the facts in problem 3. Company WB is...Ch. 3 - Prob. 5IRPCh. 3 - Prob. 6IRPCh. 3 - Prob. 7IRPCh. 3 - Prob. 8IRPCh. 3 - Prob. 9IRPCh. 3 - Prob. 1TPCCh. 3 - Firm D is considering investing 400,000 cash in a...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, accounting and related others by exploring similar questions and additional content below.Similar questions
- A taxpayer can invest $1,000 in a money market fund that yields an annual pretax rate of return of 8% or buy an acre of land for $1,000 that appreciates at a 7% annual rate. The taxpayer plans to sell the land after 20 years and faces a 25% tax rate each year. (For purposes of this problem, ignore any risk differences between investments.) What is the after-tax accumulation at the end of 20 years from each investment? What is the annualized after-tax rate of return from each investment?arrow_forwardMr. Hayes plans to pay $100,000 for one of three investment alternatives that have the same risk. The income from investment 1 would be taxed at Mr. Hayes' 24% regular tax rate, the income from investment 2 would be taxed at a 15% preferential rate, and the income from investment 3 is tax-exempt. The investments offer the following before-tax yields. • Investment 1: 8.25% • Investment 2: 7.50% • Investment 3: 6.375% Which investment should Mr. Hayes select? Group of answer choices Mr. Hayes is neutral between investment 2 and investment 3. Investment 1 Investment 2 Investment 3arrow_forwardAnswer each of the following independent questions. Ignore personal income taxes. Use Appendix A for your reference. (Use appropriate factor(s) from the tables provided.) Required: 1. Suppose you invest $4,100 in an account bearing interest at the rate of 10 percent per year. What will be the future value of your investment in five years? 2. Your best friend won the state lottery and has offered to give you $11,600 in four years, after he has made his first million dollars. You figure that if you had the money today, you could invest it at 8 percent annual interest. What is the present value of your friend’s future gift? 3. In four years, you would like to buy a small cabin in the mountains. You estimate that the property will cost you $68,500 when you are ready to buy. How much money would you need to invest each year in an account bearing interest at the rate of 4 percent per year in order to accumulate the $68,500 purchase price? 4. You have estimated that your educational expenses…arrow_forward
- Stan Marsh, an investor, is considering two financing plans for purchasing a parcel of real estate costing $152,264. Alternative 1 involves paying cash; alternative 2 involves obtaining 82% financing at 9.4% interest. If the parcel of real estate appreciates in value by $17,153 in 1 year, calculate (a) Stan's net return and (b) his return on equity for each alternative. If the value dropped by $17,153, what effect would this have on your answers to parts a and b?arrow_forwardDanny creates up an investment arrangement for friend that will return $5000 the first year,$6125 the second year, $7250 the third year, and so on, for 30 years.State appropriate formulaeand determine, how much will the investment yield altogether?arrow_forwardKomiko Tanaka invests $13,000 in LymaBean, Inc. LymaBean does not pay any dividends. Komiko projects that her investment will generate a 10 percent before-tax rate of return. She plans to invest for the long term. a. How much cash will Komiko retain, after-taxes, if she holds the investment for five years and then she sells it when the long-term capital gains rate is 15 percent?arrow_forward
- Each of the following scenarios is independent. Assume that all cash flows are after-tax cash flows. a. Campbell Manufacturing is considering the purchase of a new welding system. The cash benefits will be $480,000 per year. The system costs $2,150,000 and will last 10 years. b. Evee Cardenas is interested in investing in a women's specialty shop. The cost of the investment is $280,000. She estimates that the return from owning her own shop will be $40,000 per year. She estimates that the shop will have a useful life of 6 years. c. Barker Company calculated the NPV of a project and found it to be $63,900. The project's life was estimated to be 8 years. The required rate of return used for the NPV calculation was 10%. The project was expected to produce annual after-tax cash flows of $135,000. Required: 1. Compute the NPV for Campbell Manufacturing, assuming a discount rate of 12%. If required, round all present value calculations to the nearest dollar. Use the minus sign to indicate a…arrow_forwardLaura wants to buy a delivery truck. The truck costs $76,000 , and will allow her to increase her after tax profits by $14,000 per year for the next 10 years. She will borrow 82% of the cost of the truck for 10 years, at an interest rate of 6%. Laura’s unlevered cost of capital is 17% and her tax rate is 21%. The loan includes a $2,000 application fee. What is the NPV of buying the truck on these terms? Please use the APV method.arrow_forwardJohn has an investment opportunity that promises to pay him $16,000 in four years. Suppose the opportunity requires John to invest $13,200 today. (FV of $1, PV of $1, FVA of $1, PVA of $1, FVAD of $1 and PVAD of $1) (Use appropriate factor(s) from the tables provided.) What is the interest rate John would earn on this investment? (Round your interest rate to the nearest whole percentage.) Solve for i Present Value: n = i = Future Value:arrow_forward
- Marian Plunket owns her own business and is considering an investment. If she undertakes the investment, it will pay $4,080 at the end of each of the next three years. The opportunity requires an initial investment of $1,020 plus an additional investment at the end of the second year of $5,100. What is the NPV of this opportunity if the cost of capital is 2.3% per year? Should Marian take it? What is the NPV of this opportunity if the cost of capital is 2.3% per year? The NPV of this opportunity is $. (Round to the nearest cent.)arrow_forwardMarian Plunket owns her own business and is considering an investment. If she undertakes the investment, it will pay $4,440 at the end of each of the next 3 years. The opportunity requires an initial investment of $1,110 plus an additional investment at the end of the second year of $5,550. What is the NPV of this opportunity if the interest rate is 1.5% per year? What is the NPV of this opportunity if the interest rate is 1.5% per year? The NPV of this opportunity is $_______ (Round to the nearest cent)arrow_forwardOlga owns two investments, A and B, that have a combined total value of $28,500.00. Investment A is expected to pay $26,560.00 in 3 years from today and has an expected return of 12.32 percent per year. Investment B is expected to pay $17,158.82 in 7 years from today and has an expected return of R per year. What is R, the expected annual return for investment B? O9.87% (plus or minus 10bps) 8.40% (plus or minus 10bps) 6.30% (plus or minus 10bps) 7.31% (plus or minus 10bps) none of the answers are within 10bps of the correct answerarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Capital Budgeting Introduction & Calculations Step-by-Step -PV, FV, NPV, IRR, Payback, Simple R of R; Author: Accounting Step by Step;https://www.youtube.com/watch?v=hyBw-NnAkHY;License: Standard Youtube License