
(a)
The current in each branch of the circuit.
(a)
Answer to Problem 23P
The current in right hand branch is
Explanation of Solution
Write the expression based on junction rule.
Here,
Write the expression to obtain the loop rule.
Here,
The flow of current in the circuit is as shown in the figure below.
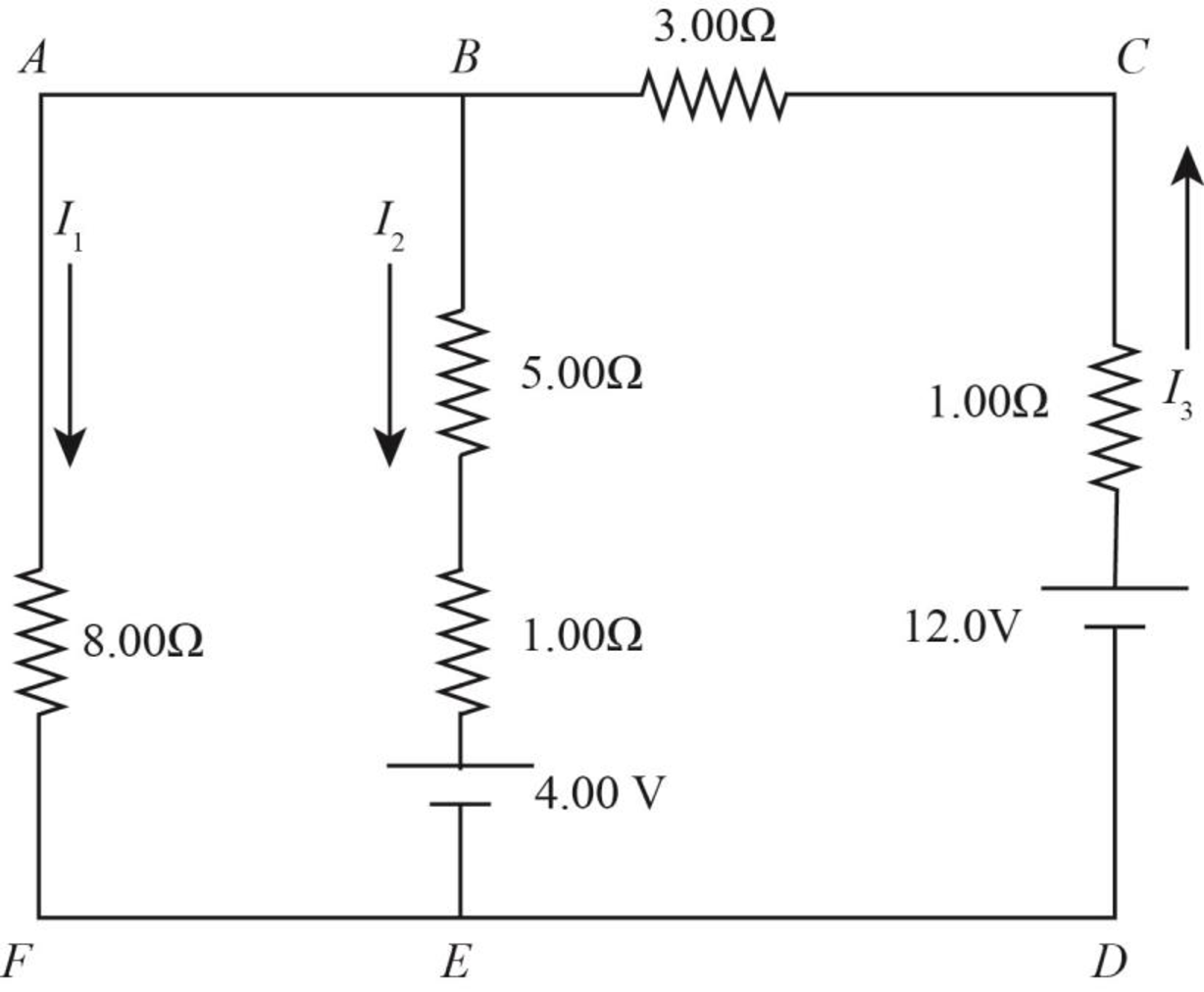
Figure-(1)
Here,
Writer the equation of Kirchhoff’s voltage rule in loop
Writer the equation of Kirchhoff’s voltage rule in loop
Write the expression based on junction rule at node
Conclusion:
Substitute
Substitute
Solve equation (IV) and (V).
Substitute
Substitute
Therefore, the current in right hand branch is
(b)
The energy delivered by each battery.
(b)
Answer to Problem 23P
The energy delivered by
Explanation of Solution
Write the expression to obtain power.
Here,
Write the expression to obtain energy.
Here,
Substitute
Conclusion:
Substitute
Here,
Substitute
Here,
Therefore, the energy delivered by
(c)
The energy delivered to each resistor.
(c)
Answer to Problem 23P
The energy delivered to the
Explanation of Solution
Write the expression of power in terms of current and resistance.
Here,
Substitute
Conclusion:
Substitute
Here,
Substitute
Here,
Substitute
Here,
Substitute
Here,
Substitute
Here,
Therefore, the energy delivered to the
(d)
The type of energy storage transformation that produced in the operation of circuit.
(d)
Explanation of Solution
The chemical energy of the
(e)
The total amount of energy transformed into internal energy in the resistor.
(e)
Answer to Problem 23P
The total amount of energy transformed into internal energy in the resistor is
Explanation of Solution
Write the expression to obtain the total amount of energy transformed into internal energy in the resistor.
Here,
Conclusion:
Substitute
Therefore, the total amount of energy transformed into internal energy in the resistor is
Want to see more full solutions like this?
Chapter 28 Solutions
Physics For Scientists And Engineers With Modern Physics, 9th Edition, The Ohio State University
- (a) For a spherical capacitor with inner radius a and outer radius b, we have the following for the capacitance. ab C = k₂(b- a) 0.0695 m 0.145 m (8.99 × 10º N · m²/c²)( [0.145 m- 0.0695 m × 10-11 F = PF IIarrow_forwardA pendulum bob A (0.5 kg) is given an initialspeed of vA = 4 m/s when the chord ishorizontal. It then hits a stationary block B (1kg) which then slides to a maximum distanced before it stops. Determine the value of d.The coefficient of static friction between theblock and the plane is μk = 0.2. The coefficientof restitution between A and B is e = 0.8.Ans: d=1.0034 marrow_forwardFigure 29-43 Problem 12. ••13 In Fig. 29-44, point P₁ is at distance R = 13.1 cm on the perpendicular bisector of a straight wire of length L = 18.0 cm carrying current i = 58.2 mA. (Note that the wire is not long.) What is the magnitude of the magnetic field at P₁ due to i? P2° R R Larrow_forward
- Checkpoint 1 The figure shows the current i in a single-loop circuit with a battery B and a resistance R (and wires of neg- ligible resistance). (a) Should the emf arrow at B be drawn pointing leftward or rightward? At points a, B C R b, and c, rank (b) the magnitude of the current, (c) the electric potential, and (d) the electric potential energy of the charge carriers, greatest first.arrow_forwardPls help ASAParrow_forwardPls help asaparrow_forward
- Pls help asaparrow_forward3. If the force of gravity stopped acting on the planets in our solar system, what would happen? a) They would spiral slowly towards the sun. b) They would continue in straight lines tangent to their orbits. c) They would continue to orbit the sun. d) They would fly straight away from the sun. e) They would spiral slowly away from the sun. 4. 1 The free-body diagram of a wagon being pulled along a horizontal surface is best represented by A F N B C 0 Ꭰ FN E a) A b) B c) C app app The app 10 app d) e) ס ח D E 10 apparrow_forwardPls help ASAParrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning





