
This problem is a continuation of Problem 45. You are part of a team working in a machine parts mechanic’s shop. An important customer has asked your company to provide springs with a very precise force constant k. You devise the electrical circuit shown in Figure P25.45 to measure the spring constant of each of the springs to be provided to the customer.
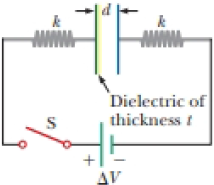
The circuit consists of two identical, parallel metal plates connected to identical metal springs, a switch, and a battery with emf ΔV. With the switch open, the plates are uncharged, are separated by a distance d, and have a capacitance C.
To provide a comparison value for the spring constant that you found in Problem 45, you slide a slab of material with dielectric constant κ and thickness t between the plates, so that it is in contact with one of the plates as shown in Figure P25.50. When the switch is closed, the plates become charged and attract each other. The distance between the plates changes by a factor f, after which the plates are in equilibrium between the spring forces and the attractive electric force between the plates. To keep the plates from going into oscillations, you hold each plate with insulating gloves as the switch is closed and apply a force on the plates that allows them to move together at a slow constant speed until they are at the equilibrium separation, at which point you can release the plates. Find an expression for the spring constant in terms of C, d, ΔV, k, t, and f.
Figure P25.50
Trending nowThis is a popular solution!

Chapter 25 Solutions
Physics for Scientists and Engineers
- 3aarrow_forward44 please help with the this.arrow_forward4a Which of the following values COULD NOT be a magnitude? Choose all that apply. 626 0 -0.806 8.63 -48.5 72 131 156 4b Px = -1248 & Py = 261. Determine P.P = Qx = -1540 & Qy = 375. Determine Q.Q = 4c. T = 1105 & Ty = 425. Determine the two possible values for Tx. 4d. Uy = -38. Which of the following COULD NOT be the value of U? Choose all that apply. 10 70 72 31 47 0 75 38 4e. R has a magnitude of 165. Which of the following COULD be Rx? Choose all that apply. 165 -171 155 0 -156 -165 172 -130arrow_forward

 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





