Chapter 25, Problem 25.43E

a.

To determine

To construct: The 99% confidence interval for the proportion of Americans who are more than 18 years of age and using social networking sites on their phones.

Answer to Problem 25.43E

The 99% confidence interval for the proportion of Americans who are more than 18 years of age and using social networking sites on their phones is 0.3039 to 0.3593 or 30.39% to 35.93%.

Explanation of Solution

Given info:

The data shows the number of cell phone users who tend to use social media on their phones and the data is separated according to their age group.

Calculation:

Let $\widehat{p}$ denote the proportion of Americans who are more than 18 years of age and using social networking sites on their phones.

$\begin{array}{c}\widehat{p}=\frac{\text{Number of Americans who are using social network on their phone}}{\text{Total number of Americans}}\\ =\frac{636}{1,918}\\ =0.3316\end{array}$

Thus, the proportion of Americans who are more than 18 years of age and using social networking sites on their phones is 0.3316.

Software procedure:

Step-by-step procedure for constructing 99% confidence interval for the given proportion is shown below:

• Click on Stat, Basic statistics and 1-proportion.
• Choose Summarized data, under Number of events enter636, under Number of trials enter 1,918.
• Click on options, choose 99% confidence interval.
• Click ok.

Output using MINITAB is given below:

Thus, the 99% confidence interval for the proportion of Americans who are more than 18 years of age and using social networking sites on their phones is 0.3039 to 0.3593.

b.

To determine

To compare: The two conditional distributions and also the graph of conditional distributions.

To find: The conditional distribution of age for the Americans who use social networking sites and who don’t use social networking sites on their phones.

To construct: A suitable graph for the conditional distribution of age for the Americans who use social networking sites and who don’t use social networking sites on their phones.

Answer to Problem 25.43E

The Americans who are aged below 50 have ahigher percentage for using social networking sites on their phones, whereas Americans who are aged greater than 50 are using comparatively less percentage of social networking sites on their phones.

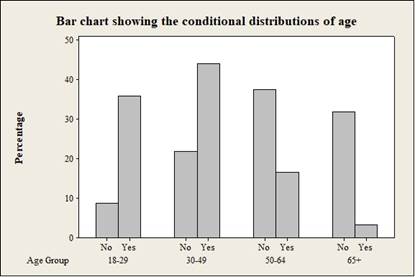
The conditional distribution of age for the Americans who use social networking sites and who don’t use social networking sites on their phones is given below:

Age Group	Conditional distribution age for the Americans who use social networking sites and who don’t use social networking sites on their phones
	Yes	No
18-29	35.85	8.74
30-49	44.18	21.92
50-64	16.67	37.52
65+	3.30	31.83

The bar graph showing the conditional distribution of age for the Americans who use social networking sites and who don’t use social networking sites on their phones is given below:

Output obtained from MINITAB is given below:

Explanation of Solution

Calculation:

The conditional distribution of age for the Americans who use social networking sites on their phones is calculated as follows:

$\left(\begin{array}{l}\text{Conditional distribution of age}\\ \text{for Americans who use social network}\\ \text{on their phones}\end{array}\right)=\frac{\left(\begin{array}{l}\text{Number of Americans who have said yes }\\ \text{under each category of age }\end{array}\right)}{\text{Total number of Americans who have said yes}}$

The conditional distribution of age for the Americans who don’t use social networking sites on their phones is calculated as follows:

$\left(\begin{array}{l}\text{Conditional distribution of age}\\ \text{for Americans who don't use}\\ \text{social network on their phones}\end{array}\right)=\frac{\left(\begin{array}{l}\text{Number of Americans who have said no }\\ \text{under each category of age }\end{array}\right)}{\text{Total number of Americans who have said no}}$

The conditional distribution age for the Americans who use social networking sites and who don’t use social networking sites on their phones is given below:

	Conditional distribution age for the Americans who use social networking sites and who don’t use social networking sites on their phones
AgeGroup	Yes	No
18-29	$\begin{array}{c}\frac{228}{636}\times 100=0.3585\times 100\\ =35.85\end{array}$	$\begin{array}{c}\frac{112}{1,282}\times 100=0.0874\times 100\\ =8.74\end{array}$
30-49	$\begin{array}{c}\frac{281}{636}\times 100=0.4418\times 100\\ =44.18\end{array}$	$\begin{array}{c}\frac{281}{1,282}\times 100=0.2192\times 100\\ =21.92\end{array}$
50-64	$\begin{array}{c}\frac{106}{636}\times 100=0.1667\times 100\\ =16.67\end{array}$	$\begin{array}{c}\frac{481}{1,282}\times 100=0.3752\times 100\\ =37.52\end{array}$
65+	$\begin{array}{c}\frac{21}{636}\times 100=0.330\times 100\\ =33.0\end{array}$	$\begin{array}{c}\frac{408}{1,282}\times 100=0.3183\times 100\\ =31.83\end{array}$

Software Procedure:

Step-by-step procedure to construct the Bar Chart using the MINITAB software:

• Choose Graph > Bar Chart.
• From Bars represent, choose Values from a table.
• Under TwoColumns of values, choose Cluster. Click OK.
• In Graph variables, enter the columns of Yes and No.
• In Row labels, enter “Age Group”.
• In Table arrangement, click “Rows are outermost categories and columns are innermost”.
• Click OK.

Interpretation:

The bar chart for comparing two conditional distributions is constructed with bars to the extreme left side of the graph, which represents the age group 18-29. Similarly, the other age groups are shown.

Justification:

The bar graph and the conditional distributions show that Americans who are aged below 50 are using the social media network much more when compared to the age group 50-65 and 65+

c.

To determine

To test: Whether there is a significant difference in the distribution of age group on social network media usage.

To find: The mean value of the test statistic given that the null hypothesis is true.

To give: The P-value.

Answer to Problem 25.43E

There is a significant difference in the distribution of age group on social network media usage.

The mean value of the chi-square test statistic given that the null hypothesis is true is given is to be 3.

The P-value is 0.000.

Explanation of Solution

Calculation:

The claim is to test whether there is any significant difference in the distribution of age group on social network media usage.

Cell frequency for using Chi-square test:

• When at most 20% of the cell frequencies are less than 5
• If all the individual frequencies are 1 or more than 1.
• All the expected frequencies must be 5 or greater than 5

The hypotheses used for testing are given below:

$H_0:$ There is no relationship between age and social network media usage.

$H_a:$ There is a relationship between age and social network media usage.

Software procedure:

Step-by-step procedure for calculating the chi-square test statistic is given below:

• Click on Stat, select Tables and then click on Chi-square Test (Two-way table in a worksheet).
• Under Columns containing the table: enter the columns of Yes and No.
• Click ok.

Output obtained from MINITAB is given below:

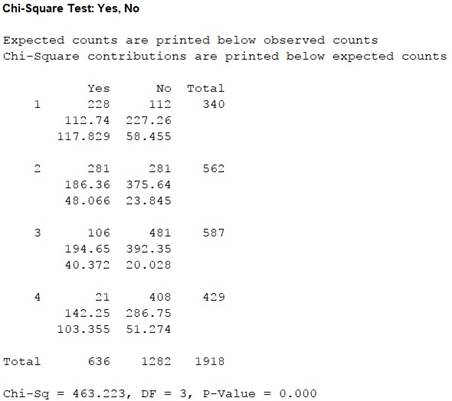
Thus, the test statistic is 463.223, the degree of freedom is 3, and the P-value is 0.000.

Since all the expected frequencies are greater than 5, the usage of chi-square test is appropriate.

Conclusion:

The P-value is 0.000 and the level of significance is 0.05.

Here, the P-value is less than the level of significance.

Therefore, the null hypothesis is rejected.

Thus, there is sufficient evidence to support the claim that there is a significant difference in the distribution of age groups on social network media usage.

Justification:

Fact:

If the null hypothesis is true, then the mean of any chi-square distribution is equal to its degrees of freedom.

From the MINITAB output, it can be observed that the degree of freedom is calculated as 3. So, the mean value of the chi-square statistic is 3.

Thus, the mean value of the chi-square statistic is 3.

Also, the observed chi-square value is ${\chi }^2=463.223$ and the mean value is 3. Thus, the observed value is larger than the mean value.

d.

To determine

To give: A comparison for the difference in the distribution of age across social media network usage.

Answer to Problem 25.43E

The age group 18-29 who have said “Yes” and the 65+ age group who have said “Yes” are contributing more to the chi-square test statistic.

The comparison shows that the age group 18 and below 50 are using social network media on their phones in a higher percentage when compared with the other age group or other people.

Explanation of Solution

From the MINITAB output, it can be seen that the observed frequencies for age group 18-29, 30-49 are higher than the expected frequency, given that the null hypothesis is true.

The age group 65+ that observed frequency for “Yes” is less than the expected frequency, given that the null hypothesis is true.