
Precalculus Plus MyLab Math with eText -- Access Card Package (10th Edition) (Sullivan & Sullivan Precalculus Titles)
10th Edition
ISBN: 9780321978981
Author: Michael Sullivan
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 2.5, Problem 16AYU
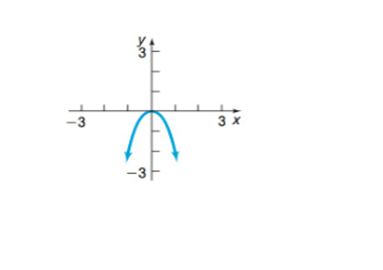
In problems 7-18, match each graph to one of the following functions:
A. B. C. D.
E. F. G. H.
I. J. K. L.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Total marks 15
3.
(i)
Let FRN Rm be a mapping and x = RN is a given
point. Which of the following statements are true? Construct counterex-
amples for any that are false.
(a)
If F is continuous at x then F is differentiable at x.
(b)
If F is differentiable at x then F is continuous at x.
If F is differentiable at x then F has all 1st order partial
(c)
derivatives at x.
(d) If all 1st order partial derivatives of F exist and are con-
tinuous on RN then F is differentiable at x.
[5 Marks]
(ii) Let mappings
F= (F1, F2) R³ → R² and
G=(G1, G2) R² → R²
:
be defined by
F₁ (x1, x2, x3) = x1 + x²,
G1(1, 2) = 31,
F2(x1, x2, x3) = x² + x3,
G2(1, 2)=sin(1+ y2).
By using the chain rule, calculate the Jacobian matrix of the mapping
GoF R3 R²,
i.e., JGoF(x1, x2, x3). What is JGOF(0, 0, 0)?
(iii)
[7 Marks]
Give reasons why the mapping Go F is differentiable at
(0, 0, 0) R³ and determine the derivative matrix D(GF)(0, 0, 0).
[3 Marks]
5.
(i)
Let f R2 R be defined by
f(x1, x2) = x² - 4x1x2 + 2x3.
Find all local minima of f on R².
(ii)
[10 Marks]
Give an example of a function f: R2 R which is not bounded
above and has exactly one critical point, which is a minimum. Justify briefly
Total marks 15
your answer.
[5 Marks]
Total marks 15
4.
:
Let f R2 R be defined by
f(x1, x2) = 2x²- 8x1x2+4x+2.
Find all local minima of f on R².
[10 Marks]
(ii) Give an example of a function f R2 R which is neither
bounded below nor bounded above, and has no critical point. Justify
briefly your answer.
[5 Marks]
Chapter 2 Solutions
Precalculus Plus MyLab Math with eText -- Access Card Package (10th Edition) (Sullivan & Sullivan Precalculus Titles)
Ch. 2.1 - The inequality 1x3 can be written in interval...Ch. 2.1 - If x=2 , the value of the expression 3 x 2 5x+ 1 x...Ch. 2.1 - The domain of the variable in the expression x3...Ch. 2.1 - Solve the inequality: 32x5 . Graph the solution...Ch. 2.1 - To rationalize the denominator of 3 5 2 , multiply...Ch. 2.1 - A quotient is considered rationalized if its...Ch. 2.1 - If f is a function defined by the equation y=f( x...Ch. 2.1 - If the domain of is all real numbers in the...Ch. 2.1 - Prob. 9AYUCh. 2.1 - Prob. 10AYU
Ch. 2.1 - The set of all images of the elements in the...Ch. 2.1 - The independent variable is sometimes referred to...Ch. 2.1 - True or False Every relation is a function.Ch. 2.1 - Prob. 12AYUCh. 2.1 - True or False If no domain is specified for a...Ch. 2.1 - Prob. 14AYUCh. 2.1 - The expression f(x+h)f(x)h is called the...Ch. 2.1 - When written as y=f(x), a function is said to be...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - Prob. 27AYUCh. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - Prob. 29AYUCh. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 19-30, state the domain and range for...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In Problems , determine whether the equation...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - Prob. 41AYUCh. 2.1 - In Problems 31-42, determine whether the equation...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In problems 43-50, find the following for each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - Prob. 53AYUCh. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - Prob. 61AYUCh. 2.1 - Prob. 62AYUCh. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In Problems 51-66, find the domain of each...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - In problems 67-76, for the given functions f and g...Ch. 2.1 - Given f( x )=3x+1 and ( f+g )( x )=6- 1 2 x , find...Ch. 2.1 - Given f( x )= 1 x and ( f g )( x )= x+1 x 2 -x ,...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - Prob. 85AYUCh. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - In Problems 79-90, find the difference quotient of...Ch. 2.1 - Given f( x )= x 2 -2x+3 , find the value(s) for x...Ch. 2.1 - Given f( x )= 5 6 x- 3 4 , find the value(s) for x...Ch. 2.1 - If f( x )=2 x 3 +A x 2 +4x-5 and f( 2 )=5 , what...Ch. 2.1 - If f( x )=3 x 2 -Bx+4 and f( 1 )=12 , what is the...Ch. 2.1 - If f( x )= 3x+8 2x-A and f( 0 )=2 , what is the...Ch. 2.1 - If f( x )= 2x-B 3x+4 and f( 2 )= 1 2 , what is the...Ch. 2.1 - Geometry Express the area A of a rectangle as a...Ch. 2.1 - Geometry Express the area A of an isosceles right...Ch. 2.1 - Prob. 99AYUCh. 2.1 - Prob. 100AYUCh. 2.1 - Prob. 101AYUCh. 2.1 - Prob. 102AYUCh. 2.1 - Effect of Gravity on Earth If a rock falls from a...Ch. 2.1 - Effect of Gravity on Jupiter If a rock falls from...Ch. 2.1 - Cost of Transatlantic Travel A Boeing 747 crosses...Ch. 2.1 - Cross-sectional Area The cross-sectional area of a...Ch. 2.1 - Economics The participation rate is the number of...Ch. 2.1 - Crimes Suppose that V( x ) represents the number...Ch. 2.1 - Health Care Suppose that P( x ) represents the...Ch. 2.1 - Income Tax Suppose that I( x ) represents the...Ch. 2.1 - Profit Function Suppose that the revenue R , in...Ch. 2.1 - Prob. 112AYUCh. 2.1 - Stopping Distance When the driver of a vehicle...Ch. 2.1 - Some functions f have the property that f( a+b...Ch. 2.1 - Prob. 115AYUCh. 2.1 - Investigate when, historically, the use of the...Ch. 2.1 - Prob. 116AYUCh. 2.1 - Problems 118-121 are based on material learned...Ch. 2.1 - Problems 118-121 are based on material learned...Ch. 2.1 - Prob. 120AYUCh. 2.1 - Prob. 121AYUCh. 2.2 - The intercepts of the equation x 2 +4 y 2 =16 are...Ch. 2.2 - True or False The point ( 2,6 ) is on the graph of...Ch. 2.2 - 3. A set of points in the xy -plane is the graph...Ch. 2.2 - 4. If the point ( 5,3 ) is a point on the graph of...Ch. 2.2 - 5. Find a so that the point ( 1,2 ) is on the...Ch. 2.2 - True or False Every graph represents a function.Ch. 2.2 - True or False The graph of a function y=f( x )...Ch. 2.2 - True or False The y-intercept of the graph of the...Ch. 2.2 - If a function is defined by an equation in x and y...Ch. 2.2 - The graph of a function y=f(x) can have more than...Ch. 2.2 - Use the given graph the function f to answer parts...Ch. 2.2 - Use the given graph the function f to answer parts...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - In Problems 13-24, determine whether the graph is...Ch. 2.2 - Prob. 25AYUCh. 2.2 - In Problems 25-30, answer the questions about the...Ch. 2.2 - In Problems 25-30, answer the questions about the...Ch. 2.2 - In Problems 25-30, answer the questions about the...Ch. 2.2 - Prob. 29AYUCh. 2.2 - In Problems 25-30, answer the questions about the...Ch. 2.2 - The graphs of two functions, f and g , arc...Ch. 2.2 - Granny Shots The last player in the NBA to use an...Ch. 2.2 - Free-throw Shots According to physicist Peter...Ch. 2.2 - Cross-sectional Area The cross-sectional area of a...Ch. 2.2 - Motion οf a Golf Ball A golf ball is hit with an...Ch. 2.2 - Effect of Elevation on Weight If an object weighs...Ch. 2.2 - Cost of Transatlantic Travel A Boeing 747 crosses...Ch. 2.2 - Reading and Interpreting Graphs Let C be the...Ch. 2.2 - Reading and Interpreting Graphs Let C be the...Ch. 2.2 - Describe how you would find the domain and range...Ch. 2.2 - How many x-intercepts can the graph of a function...Ch. 2.2 - Is a graph that consists of a single point the...Ch. 2.2 - Match each of the following functions with the...Ch. 2.2 - Match each of the following functions with the...Ch. 2.2 - Consider the following scenario: Barbara decides...Ch. 2.2 - Consider the following scenario: Jayne enjoys...Ch. 2.2 - The following sketch represents the distance d(in...Ch. 2.2 - The following sketch represents the speed v (in...Ch. 2.2 - Draw the graph of a function whose domain is { x|...Ch. 2.2 - Prob. 50AYUCh. 2.2 - Explain why the vertical-line test works.Ch. 2.2 - Prob. 52AYUCh. 2.2 - Problems 52-55 are based on material learned...Ch. 2.2 - Prob. 54AYUCh. 2.2 - Prob. 55AYUCh. 2.3 - ‘Are You Prepared?' Answers are given at the end...Ch. 2.3 - ‘Are You Prepared?' Answers are given at the end...Ch. 2.3 - ‘Are You Prepared?' Answers are given at the end...Ch. 2.3 - ‘Are You Prepared?' Answers are given at the end...Ch. 2.3 - ‘Are You Prepared?' Answers are given at the end...Ch. 2.3 - 6. A function f is _____ on an interval I if, for...Ch. 2.3 - A(n)_____function is one for which for every in...Ch. 2.3 - 8. True or False A function f is decreasing on an...Ch. 2.3 - Prob. 9AYUCh. 2.3 - 10. True or False Even functions have graphs that...Ch. 2.3 - 11. An odd function is symmetric with respect to...Ch. 2.3 - 12. Which of the following intervals is required...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 13-24, use the graph of the function f...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 25-32, the graph of a function is...Ch. 2.3 - In Problems 33-36, the graph of a function f is...Ch. 2.3 - In Problems 33-36, the graph of a function f is...Ch. 2.3 - In Problems 33-36, the graph of a function f is...Ch. 2.3 - In Problems 33-36, the graph of a function f is...Ch. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - Prob. 39AYUCh. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - In Problems 3748, determine algebraically whether...Ch. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - Prob. 45AYUCh. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - In Problems 37-48, determine algebraically whether...Ch. 2.3 - In Problem 49-56, for each graph of a function...Ch. 2.3 - In Problem 49-56, for each graph of a function...Ch. 2.3 - In Problem 49-56, for each graph of a function...Ch. 2.3 - In Problem 49-56, for each graph of a function...Ch. 2.3 - In Problem 49-56, for each graph of a function...Ch. 2.3 - In Problems 49-56, for each graph of a function...Ch. 2.3 - In Problems 49-56, for each graph of a function...Ch. 2.3 - In Problems 49-56, for each graph of a function...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - In Problems 57-64, use a graphing utility to graph...Ch. 2.3 - Find the average rate of change of f(x)=2x2+4:...Ch. 2.3 - Find the average rate of change of
From to ...Ch. 2.3 - Prob. 67AYUCh. 2.3 - 68. Find the average rate of change of h( x )= x 2...Ch. 2.3 - 69. f( x )=5x2 (a) Find the average rate of change...Ch. 2.3 - 70. f( x )=4x+1 (a) Find the average rate of...Ch. 2.3 - g(x)=x22 Find the average rate of change from 2 to...Ch. 2.3 - g(x)=x2+1 Find the average rate of change from -1...Ch. 2.3 - h(x)=x22x Find the average rate of change from 2...Ch. 2.3 - h(x)=2x2+x Find the average rate of change from 0...Ch. 2.3 - 75. g( x )= x 3 27x (a) Determine whether g is...Ch. 2.3 - 76. f( x )= x 3 +12x (a) Determine whether f is...Ch. 2.3 - Prob. 77AYUCh. 2.3 - 78. G( x )= x 4 +32 x 2 +144 (a) Determine whether...Ch. 2.3 - 80. Medicine Concentration The concentration C of...Ch. 2.3 - Data Plan Cost The monthly cost C, in dollars, for...Ch. 2.3 -
National Debt The size of the total debt owned...Ch. 2.3 - Prob. 83AYUCh. 2.3 - Prob. 84AYUCh. 2.3 - 85. For the function f( x )= x 2 , compute the...Ch. 2.3 - 86. For the function f( x )= x 2 , compute the...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - Problems 87-94 require the following discussion of...Ch. 2.3 - 95. Draw the graph of a function that has the...Ch. 2.3 - 96. Redo Problem 95 with the following additional...Ch. 2.3 - 97. How many x-intercept can a function defined on...Ch. 2.3 - 98. Suppose that a friend of yours does not...Ch. 2.3 - 99. Can a function be both even and odd? Explain.Ch. 2.3 - 100. Using a graphing utility, graph y=5 on the...Ch. 2.3 - 101. A function f has a positive average rate of...Ch. 2.3 - 102. Show that a constant function f( x )=b has an...Ch. 2.3 - Prob. 103AYUCh. 2.3 - In Problems are based on material learned earlier...Ch. 2.3 - Prob. 105AYUCh. 2.3 - Prob. 106AYUCh. 2.4 - Sketch the graph of y= x . (p. 22)Ch. 2.4 - Sketch the graph of y= 1 x . (pp. 22-23)Ch. 2.4 - List the intercepts of the equation y= x 3 8 ....Ch. 2.4 - The function f( x )= x 2 is decreasing on the...Ch. 2.4 - When functions are defined by more than one...Ch. 2.4 - True or False The cube function is odd and is...Ch. 2.4 - True or False The cube root function is odd and is...Ch. 2.4 - True or False The domain and the range of the...Ch. 2.4 - Which of the following functions has a graph that...Ch. 2.4 - Consider the following function. f( x )= 3x2ifx2 x...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 11-18, match each graph to its...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - In Problems 19-26, sketch the graph of each...Ch. 2.4 - Prob. 27AYUCh. 2.4 - Prob. 28AYUCh. 2.4 - Prob. 29AYUCh. 2.4 - If f( x )={ x 3 if2x1 3x+2if1x4 find: (a) f( 1 )...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 - In Problems 31-42: (a) Find the domain of each...Ch. 2.4 -
In problems
Find the domain of each...Ch. 2.4 - In Problems 3142 Find the domain of each function....Ch. 2.4 - In Problems 43-46, the graph of a...Ch. 2.4 - In Problems 43-46, the graph of a...Ch. 2.4 - In Problems 43-46, the graph of a...Ch. 2.4 - In Problems 43-46, the graph of a...Ch. 2.4 - Prob. 47AYUCh. 2.4 - If f( x )=int( x 2 ) , find (a) f( 1.2 ) (b) f(...Ch. 2.4 - Tablet Service A monthly tablet plan costs 34.99 ....Ch. 2.4 - Parking at OHare International Airport The...Ch. 2.4 - Cost of natural gas In march 2014, Laclede Gas had...Ch. 2.4 -
Cost of natural gas In april ,Nicor Gas had the...Ch. 2.4 - Federal Income Tax Two 2014 Tax Rate Schedules are...Ch. 2.4 - Federal Income Tax Refer to the 2014 tax rate...Ch. 2.4 -
Cost of Transporting Goods. A trucking company...Ch. 2.4 - Car Rental Costs An economy car rented in Florida...Ch. 2.4 -
Mortgage Fees Fannie Mae charges a loan-level...Ch. 2.4 - Minimum Payments for Credit Cards Holders of a...Ch. 2.4 - Wind Chill The wind chill factor represents the...Ch. 2.4 - Wind Chill Redo Problem 61(a)-(d) for an air...Ch. 2.4 -
First-class mail In the U.S Postal Service...Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - In Problems 64-71, use a graphing utility....Ch. 2.4 - Consider the equation y={ 1ifxisrational...Ch. 2.4 - Define some functions that pass through ( 0,0...Ch. 2.4 - Prob. 72AYUCh. 2.4 - Problems are based on material learned earlier in...Ch. 2.4 - Prob. 74AYUCh. 2.4 - Prob. 75AYUCh. 2.5 - Suppose that the graph of a function f is known....Ch. 2.5 - Suppose that the graph of a function f is known....Ch. 2.5 - True or False The graph of y= 1 3 g( x ) is the...Ch. 2.5 - True or False The graph of y=f( x ) is the...Ch. 2.5 - Which of the following functions has a graph that...Ch. 2.5 - Which of the following functions has a graph that...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In problems 7-18, match each graph to one of the...Ch. 2.5 - In Problem 19-26, write the function whose graph...Ch. 2.5 - In Problem 19-26, write the function whose graph...Ch. 2.5 - In Problem 19-26, write the function whose graph...Ch. 2.5 - In Problem 19-26, write the function whose graph...Ch. 2.5 - In Problem 19-26, write the function whose graph...Ch. 2.5 - In Problem 19-26, write the function whose graph...Ch. 2.5 - Prob. 25AYUCh. 2.5 - In Problem 19-26, write the function whose graph...Ch. 2.5 - In Problem 27-30, find the function that is...Ch. 2.5 - In Problem 27-30, find the function that is...Ch. 2.5 - Prob. 29AYUCh. 2.5 - In Problem 27-30, find the function that is...Ch. 2.5 - In Problem 27-30, find the function that is...Ch. 2.5 - In Problem 27-30, find the function that is...Ch. 2.5 - In Problem 27-30, find the function that is...Ch. 2.5 - In Problem 27-30, find the function that is...Ch. 2.5 - Prob. 35AYUCh. 2.5 - Prob. 36AYUCh. 2.5 - Prob. 37AYUCh. 2.5 - Prob. 38AYUCh. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - Prob. 41AYUCh. 2.5 - Prob. 42AYUCh. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 39-62, graph each function using the...Ch. 2.5 - In Problems 63-66, the graph of a function f is...Ch. 2.5 - In Problems 63-66, the graph of a function f is...Ch. 2.5 - In Problems 63-66, the graph of a function f is...Ch. 2.5 - In Problems 63-66, the graph of a function f is...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - In Problems 69-76, complete the square of each...Ch. 2.5 - 77. The graph of a function f is illustrated in...Ch. 2.5 - 78. The graph of a function f is illustrated in...Ch. 2.5 - 79. Suppose ( 1,3 ) is a point on the graph of...Ch. 2.5 - 80. Suppose ( 3,5 ) is a point on the graph of...Ch. 2.5 - 81. Graph the following functions using...Ch. 2.5 - 82. Graph the following functions using...Ch. 2.5 - 83. (a) Graph f( x )=| x3 |3 using...Ch. 2.5 - 84. (a) Graph f( x )=2| x4 |+4 using...Ch. 2.5 - 85. Thermostat Control Energy conservation experts...Ch. 2.5 - Digital Music Revenues The total projected...Ch. 2.5 - 87. Temperature Measurements The relationship...Ch. 2.5 - 88. Period of a Pendulum The period T (in seconds)...Ch. 2.5 - 89. The equation y= ( xc ) 2 defines a family of...Ch. 2.5 - 90. Repeat Problem 89 for the family of parabolas...Ch. 2.5 - 91. Suppose that the graph of a function f is...Ch. 2.5 - 92. Suppose that the graph of a function f is...Ch. 2.5 - 93. The area under the curve y= x bounded front...Ch. 2.5 - 94. Explain how the range of the function f( x )=...Ch. 2.5 - 95. Explain how the domain of g( x )= x compares...Ch. 2.5 - Prob. 94AYUCh. 2.5 - Prob. 95AYUCh. 2.5 - Prob. 96AYUCh. 2.5 - Prob. 97AYUCh. 2.6 - 1. P=( x,y ) be a point on the graph of y= x 2 8 ....Ch. 2.6 - 2. P=( x,y ) be a point on the graph of y= x 2 8 ....Ch. 2.6 - Prob. 3AYUCh. 2.6 - Prob. 4AYUCh. 2.6 - 5. A right triangle has one vertex on the graph of...Ch. 2.6 - 6. A right triangle has one vertex on the graph of...Ch. 2.6 - A rectangle has one corner in quadrant I on the...Ch. 2.6 -
A rectangle is inscribed in a semicircle of...Ch. 2.6 - 9. A rectangle is inscribed in a semicircle of...Ch. 2.6 - 10. A circle of radius r is inscribed in a square....Ch. 2.6 - 11. Geometry A wire 10 meters long is to be cut...Ch. 2.6 - Prob. 12AYUCh. 2.6 - 13. Geometry A wire of length x is bent into the...Ch. 2.6 - Prob. 14AYUCh. 2.6 - 15. Geometry A semicircle of radius r is inscribed...Ch. 2.6 - 16. Geometry An equilateral triangle is inscribed...Ch. 2.6 - 17. Geometry An equilateral triangle is inscribed...Ch. 2.6 - 18. Uniform Motion Two cars leave an intersection...Ch. 2.6 - 19. Uniform Motion Two cars are approaching an...Ch. 2.6 - 20. Inscribing a Cylinder in a Sphere Inscribe a...Ch. 2.6 - 21. Inscribing a Cylinder in a Cone Inscribe a...Ch. 2.6 - 22. Installing Cable TV MetroMedia Cable is asked...Ch. 2.6 - 23. Time Required to Go from an Island to a Town...Ch. 2.6 - 24. Filling a Conical Tank Water is poured into a...Ch. 2.6 - Constructing an Open Box An open box with a square...Ch. 2.6 - Constructing an Open Box An open box with a...Ch. 2.6 - Prob. 27AYUCh. 2.6 - Write the function whose graph is the graph of...Ch. 2.6 - Problems are based on material learned earlier in...Ch. 2.6 - Find the domain of h(x)={2x1ifx23x2if2x5Ch. 2 - In Problems 1 and 2, determine whether each...Ch. 2 - In Problems and , Determine whether each relation...Ch. 2 - In Problems 3-5, find the following for each...Ch. 2 - In Problems 3-5, find the following for each...Ch. 2 - In Problems 3-5, find the following for each...Ch. 2 - In Problems 6-11, find the domain of each...Ch. 2 - In Problems 6-11, find the domain of each...Ch. 2 - In Problems 6-11, find the domain of each...Ch. 2 - In Problems 6-11, find the domain of each...Ch. 2 - In Problems 6-11, find the domain of each...Ch. 2 - In Problems 6-11, find the domain of each...Ch. 2 - In Problems 12-14, find f+g,fg,fg,and f g for each...Ch. 2 - In Problems 12-14, find f+g,fg,fg,and f g for each...Ch. 2 - In Problems 12-14, find f+g,fg,fg,and f g for each...Ch. 2 - Find the difference quotient of f( x )=2 x 2 +x+1...Ch. 2 - Consider the graph of the function f on the right....Ch. 2 - Use the graph of the function f shown to find: (a)...Ch. 2 - In Problems 18-21, determine (algebraically)...Ch. 2 - In Problems 18-21, determine (algebraically)...Ch. 2 - In Problems 18-21, determine (algebraically)...Ch. 2 - In Problems 18-21, determine (algebraically)...Ch. 2 - In problems 25 and 26, use a graphing utility to...Ch. 2 - In problems and use a graphing utility to graph...Ch. 2 - Find the average rate of change of f( x )=8 x 2 x...Ch. 2 - In Problems 25 and 26, find the average rate of...Ch. 2 - In Problems 25 and 26, find the average rate of...Ch. 2 - In Problems 27 and 28, is the graph shown the...Ch. 2 - In Problems 27 and 28, is the graph shown the...Ch. 2 - In Problems 29 and 30, graph each function. Be...Ch. 2 - In Problems 29 and 30, graph each function. Be...Ch. 2 - In Problems 31-36, graph each function using the...Ch. 2 - In Problems 31-36, graph each function using the...Ch. 2 - In Problems 31-36, graph each function using the...Ch. 2 - In Problems 31-36, graph each function using the...Ch. 2 - In Problems 31-36, graph each function using the...Ch. 2 - In Problems 31-36, graph each function using the...Ch. 2 - In Problems 37 and 38: (a) Find the domain of each...Ch. 2 - In Problems 37 and 38: (a) Find the domain of each...Ch. 2 - A function f is defined by f( x )= Ax+5 6x2 If f(...Ch. 2 - Constructing a Closed Box A closed box with a...Ch. 2 - Area of a Rectangle A rectangle has one vertex in...Ch. 2 - 1. Find the domain and range of each relation....Ch. 2 - In Problems find the domain of each function and...Ch. 2 - In Problems 24, find the domain of each function...Ch. 2 - In Problems 24, find the domain of each function...Ch. 2 - Consider the graph of the function f below. Find...Ch. 2 - 6. Graph the function on the interval using a...Ch. 2 - Consider the function g(x)={2x+1ifx1x4ifx1 Graph...Ch. 2 - For the function f(x)=3x22x+4, find the average...Ch. 2 - For the functions f(x)=2x2+1 and g(x)=3x2, find...Ch. 2 - Graph each function using the techniques of...Ch. 2 -
The variable interest rate on a student loan...Ch. 2 - Prob. 12CTCh. 2 - In Problems 1-6, find the real solutions of each...Ch. 2 - In Problems 1-6, find the real solutions of each...Ch. 2 - In Problems 1-6, find the real solutions of each...Ch. 2 - In Problems 1-6, find the real solutions of each...Ch. 2 - In Problems 1-6, find the real solutions of each...Ch. 2 - In Problems 1-6, find the real solutions of each...Ch. 2 - In Problems 7-9, solve each ineQuality. Graph the...Ch. 2 - In Problems 7-9, solve each ineQuality. Graph the...Ch. 2 - In Problems 7-9, solve each ineQuality. Graph the...Ch. 2 - (a) Find the distance from P 1 =( 2,3 )to P 2 =(...Ch. 2 - In problems 11-14, graph each equation. 3x2y=12Ch. 2 - In problems 11-14, graph each equation. x= y 2Ch. 2 - In problems 11-14, graph each equation. x 2 + ( y3...Ch. 2 - In problems 11-14, graph each equation. y= xCh. 2 - Prob. 15CRCh. 2 - Find the slope-intercept form of the equation of...Ch. 2 - In problems 17-19, graph each function. f( x )= (...Ch. 2 - In problems 17-19, graph each function. f( x )= 1...Ch. 2 - In problems 17-19, graph each function. f( x )={...
Additional Math Textbook Solutions
Find more solutions based on key concepts
A categorical variable has three categories, with the following frequencies of occurrence: a. Compute the perce...
Basic Business Statistics, Student Value Edition
CHECK POINT 1 In a survey on musical tastes, respondents were asked: Do you listed to classical music? Do you l...
Thinking Mathematically (6th Edition)
Express the side length of a square as a function of the length d of the square’s diagonal. Then express the ar...
University Calculus: Early Transcendentals (4th Edition)
Comparison tests Use the Comparison Test or Limit Comparison Test to determine whether the following series con...
Calculus: Early Transcendentals (2nd Edition)
For years, telephone area codes in the United States and Canada consisted of a sequence of three digits. The fi...
A First Course in Probability (10th Edition)
Fill in each blank so that the resulting statement is true. If n is a counting number, bn, read ______, indicat...
College Algebra (7th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- 4. Let F RNR be a mapping. (i) x ЄRN ? (ii) : What does it mean to say that F is differentiable at a point [1 Mark] In Theorem 5.4 in the Lecture Notes we proved that if F is differentiable at a point x E RN then F is continuous at x. Proof. Let (n) CRN be a sequence such that xn → x ЄERN as n → ∞. We want to show that F(xn) F(x), which means F is continuous at x. Denote hnxn - x, so that ||hn|| 0. Thus we find ||F(xn) − F(x)|| = ||F(x + hn) − F(x)|| * ||DF (x)hn + R(hn) || (**) ||DF(x)hn||+||R(hn)||| → 0, because the linear mapping DF(x) is continuous and for all large nЄ N, (***) ||R(hn) || ||R(hn) || ≤ → 0. ||hn|| (a) Explain in details why ||hn|| → 0. [3 Marks] (b) Explain the steps labelled (*), (**), (***). [6 Marks]arrow_forward4. In Theorem 5.4 in the Lecture Notes we proved that if F: RN → Rm is differentiable at x = RN then F is continuous at x. Proof. Let (xn) CRN be a sequence such that x → x Є RN as n → ∞. We want F(x), which means F is continuous at x. to show that F(xn) Denote hn xnx, so that ||hn||| 0. Thus we find ||F (xn) − F(x) || (*) ||F(x + hn) − F(x)|| = ||DF(x)hn + R(hn)|| (**) ||DF(x)hn|| + ||R(hn) || → 0, because the linear mapping DF(x) is continuous and for all large n = N, |||R(hn) || ≤ (***) ||R(hn)|| ||hn|| → 0. Explain the steps labelled (*), (**), (***) [6 Marks] (ii) Give an example of a function F: RR such that F is contin- Total marks 10 uous at x=0 but F is not differentiable at at x = 0. [4 Marks]arrow_forward3. Let f R2 R be a function. (i) Explain in your own words the relationship between the existence of all partial derivatives of f and differentiability of f at a point x = R². (ii) Consider R2 → R defined by : [5 Marks] f(x1, x2) = |2x1x2|1/2 Show that af af -(0,0) = 0 and -(0, 0) = 0, Jx1 მx2 but f is not differentiable at (0,0). [10 Marks]arrow_forward
- (1) Write the following quadratic equation in terms of the vertex coordinates.arrow_forwardThe final answer is 8/π(sinx) + 8/3π(sin 3x)+ 8/5π(sin5x)....arrow_forwardKeity x२ 1. (i) Identify which of the following subsets of R2 are open and which are not. (a) A = (2,4) x (1, 2), (b) B = (2,4) x {1,2}, (c) C = (2,4) x R. Provide a sketch and a brief explanation to each of your answers. [6 Marks] (ii) Give an example of a bounded set in R2 which is not open. [2 Marks] (iii) Give an example of an open set in R2 which is not bounded. [2 Marksarrow_forward
- 2. (i) Which of the following statements are true? Construct coun- terexamples for those that are false. (a) sequence. Every bounded sequence (x(n)) nEN C RN has a convergent sub- (b) (c) (d) Every sequence (x(n)) nEN C RN has a convergent subsequence. Every convergent sequence (x(n)) nEN C RN is bounded. Every bounded sequence (x(n)) EN CRN converges. nЄN (e) If a sequence (xn)nEN C RN has a convergent subsequence, then (xn)nEN is convergent. [10 Marks] (ii) Give an example of a sequence (x(n))nEN CR2 which is located on the parabola x2 = x², contains infinitely many different points and converges to the limit x = (2,4). [5 Marks]arrow_forward2. (i) What does it mean to say that a sequence (x(n)) nEN CR2 converges to the limit x E R²? [1 Mark] (ii) Prove that if a set ECR2 is closed then every convergent sequence (x(n))nen in E has its limit in E, that is (x(n)) CE and x() x x = E. [5 Marks] (iii) which is located on the parabola x2 = = x x4, contains a subsequence that Give an example of an unbounded sequence (r(n)) nEN CR2 (2, 16) and such that x(i) converges to the limit x = (2, 16) and such that x(i) # x() for any i j. [4 Marksarrow_forward1. (i) which are not. Identify which of the following subsets of R2 are open and (a) A = (1, 3) x (1,2) (b) B = (1,3) x {1,2} (c) C = AUB (ii) Provide a sketch and a brief explanation to each of your answers. [6 Marks] Give an example of a bounded set in R2 which is not open. (iii) [2 Marks] Give an example of an open set in R2 which is not bounded. [2 Marks]arrow_forward
- 2. if limit. Recall that a sequence (x(n)) CR2 converges to the limit x = R² lim ||x(n)x|| = 0. 818 - (i) Prove that a convergent sequence (x(n)) has at most one [4 Marks] (ii) Give an example of a bounded sequence (x(n)) CR2 that has no limit and has accumulation points (1, 0) and (0, 1) [3 Marks] (iii) Give an example of a sequence (x(n))neN CR2 which is located on the hyperbola x2 1/x1, contains infinitely many different Total marks 10 points and converges to the limit x = (2, 1/2). [3 Marks]arrow_forward3. (i) Consider a mapping F: RN Rm. Explain in your own words the relationship between the existence of all partial derivatives of F and dif- ferentiability of F at a point x = RN. (ii) [3 Marks] Calculate the gradient of the following function f: R2 → R, f(x) = ||x||3, Total marks 10 where ||x|| = √√√x² + x/2. [7 Marks]arrow_forward1. (i) (ii) which are not. What does it mean to say that a set ECR2 is closed? [1 Mark] Identify which of the following subsets of R2 are closed and (a) A = [-1, 1] × (1, 3) (b) B = [-1, 1] x {1,3} (c) C = {(1/n², 1/n2) ER2 | n EN} Provide a sketch and a brief explanation to each of your answers. [6 Marks] (iii) Give an example of a closed set which does not have interior points. [3 Marks]arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you

 Algebra for College StudentsAlgebraISBN:9781285195780Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning
Algebra for College StudentsAlgebraISBN:9781285195780Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning Intermediate AlgebraAlgebraISBN:9781285195728Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning
Intermediate AlgebraAlgebraISBN:9781285195728Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill


Algebra for College Students
Algebra
ISBN:9781285195780
Author:Jerome E. Kaufmann, Karen L. Schwitters
Publisher:Cengage Learning

Intermediate Algebra
Algebra
ISBN:9781285195728
Author:Jerome E. Kaufmann, Karen L. Schwitters
Publisher:Cengage Learning

College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning

Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
What is Ellipse?; Author: Don't Memorise;https://www.youtube.com/watch?v=nzwCInIMlU4;License: Standard YouTube License, CC-BY