
Concept explainers
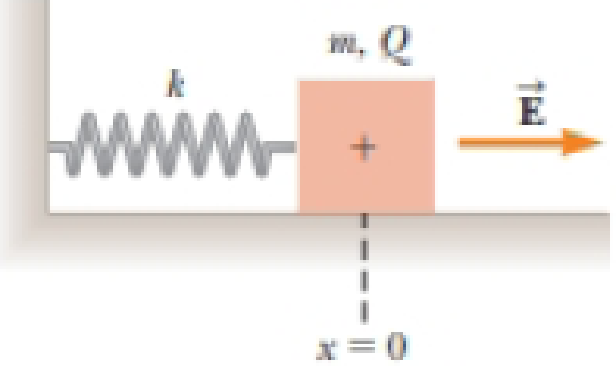
Review. A block having mass m and charge + Q is connected to an insulating spring having a force constant k. The block lies on a frictionless, insulating, horizontal track, and the system is immersed in a uniform electric field of magnitude E directed as shown in Figure P24.6. The block is released from rest when the spring is unstretched (at x = 0). We wish to show that the ensuing motion of the block is simple harmonic. (a) Consider the system of the block, the spring, and the electric field. Is this system isolated or nonisolated? (b) What kinds of potential energy exist within this system? (c) Call the initial configuration of the system that existing just as the block is released from rest. The final configuration is when the block momentarily comes to rest again. What is the value of x when the block comes to rest momentarily? (d) At some value of x we will call x = x0, the block has zero net force on it. What analysis model describes the particle in this situation? (c) What is the value of x0? (f) Define a new coordinate system x′ such that x′ = x − x0. Show that x′ satisfies a differential equation for
Figure P24.6
(a)
Whether the system is isolated or non-isolated.
Answer to Problem 6P
The system is non-isolated.
Explanation of Solution
Given info: The mass of the block is
The system of the block with a spring is isolated system but when the system of the block with a spring is placed in a electric or magnetic field then it experiences an external force that makes the system non-isolated.
Conclusion:
Therefore, the system is non-isolated.
(b)
The kinds of potential energy exist in the system.
Answer to Problem 6P
The kinds of potential energy exist in the system are elastic potential energy and electrostatic potential energy.
Explanation of Solution
Given info: The mass of the block is
There are two kinds of potential energy exist in the system one is elastic potential energy and other is electrostatic potential energy.
The formula to calculate the elastic potential energy is,
Here,
The formula to calculate the electrostatic potential energy is,
Here,
The formula to calculate electrostatic force is,
Here,
Substitute
Conclusion:
Therefore, the kinds of potential energy exist in the system are elastic potential energy and electrostatic potential energy.
(c)
The value of
Answer to Problem 6P
The value of
Explanation of Solution
Given info: The mass of the block is
The formula to calculate the elastic potential energy is,
Here,
The formula to calculate the electrostatic potential energy is,
Here,
The formula to calculate electrostatic force is,
Here,
Substitute
Equate the formula (1) and (2) as,
Conclusion:
Therefore, the value of
(d)
The analysis model that describes particle in this situation.
Answer to Problem 6P
The analysis model that describes the particle in this situation is in equilibrium.
Explanation of Solution
Given info: The mass of the block is
The above statement is explained as the net force acting on the block is zero at some value of
Conclusion:
Therefore, the particle in this situation is in equilibrium.
(e)
The value of
Answer to Problem 6P
The value of
Explanation of Solution
Given info: The mass of the block is
The formula to calculate the spring force is,
Here,
The formula to calculate electric force is,
Here,
The net force is zero so it is expressed as,
Here,
Rearrange the above expression to find
Conclusion:
Therefore, the value of
(f)
To show: The coordinate
Answer to Problem 6P
Thus, the coordinate
Explanation of Solution
The mass of the block is
Given info:
From part (e), the value of
The formula to calculate the force equation is,
Here,
The formula to calculate the acceleration is,
Here,
The summation of the force is,
Here,
The new coordinate is,

Substitute
Substitute
This is the required equation of S.H.M.
Conclusion:
Therefore, the coordinate
(g)
The period of the simple harmonic motion.
Answer to Problem 6P
The period of the simple harmonic motion is
Explanation of Solution
Given info: The mass of the block is
From part (f), the equation for the S.H.M is,
The general equation of S.H.M is,
Here,
Compare the equation (4) and (5) as,
The formula to calculate the time period is,
Here,
Substitute
Conclusion:
Therefore, the period of the simple harmonic motion is
(h)
The time period depend on the electric field magnitude or not.
Answer to Problem 6P
The time period does not depend on the electric field magnitude.
Explanation of Solution
Given info: The mass of the block is
From part (g),the time period is,
In the above formula, there is no term that signifies electric field magnitude. So the time period is independent of electric field magnitude.
Conclusion:
Therefore, the time period does not depend on the electric field magnitude.
Want to see more full solutions like this?
Chapter 24 Solutions
Bundle: Physics For Scientists And Engineers With Modern Physics, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Multi-term
Additional Science Textbook Solutions
College Physics: A Strategic Approach (3rd Edition)
Microbiology Fundamentals: A Clinical Approach
Fundamentals Of Thermodynamics
MARINE BIOLOGY
Campbell Essential Biology (7th Edition)
Cosmic Perspective Fundamentals
- A hollow, conducting sphere with an outer radius of 0.260 m and an inner radius of 0.200 m has a uniform surface charge density of +6.67 × 10−6 C/m2. A charge of -0.800 μC is now introduced into the cavity inside the sphere. What is the new charge density on the outside of the sphere? Calculate the strength of the electric field just outside the sphere. What is the electric flux through a spherical surface just inside the inner surface of the sphere?arrow_forwardA point charge of -3.00 μC is located in the center of a spherical cavity of radius 6.60 cm inside an insulating spherical charged solid. The charge density in the solid is 7.35 × 10−4 C/m3. Calculate the magnitude of the electric field inside the solid at a distance of 9.10 cm from the center of the cavity. Find the direction of this electric field.arrow_forwardAn infinitely long conducting cylindrical rod with a positive charge λ per unit length is surrounded by a conducting cylindrical shell (which is also infinitely long) with a charge per unit length of −2λ and radius r1, as shown in the figure. What is E(r), the radial component of the electric field between the rod and cylindrical shell as a function of the distance r from the axis of the cylindrical rod? Express your answer in terms of λ, r, and ϵ0, the permittivity of free space. What is σinner, the surface charge density (charge per unit area) on the inner surface of the conducting shell? What is σouterσouter, the surface charge density on the outside of the conducting shell? (Recall from the problem statement that the conducting shell has a total charge per unit length given by −2λ.) What is the radial component of the electric field, E(r), outside the shell?arrow_forward
- A very long conducting tube (hollow cylinder) has inner radius aa and outer radius b. It carries charge per unit length +α, where αα is a positive constant with units of C/m. A line of charge lies along the axis of the tube. The line of charge has charge per unit length +α. Calculate the electric field in terms of α and the distance r from the axis of the tube for r<a. Calculate the electric field in terms of α and the distance rr from the axis of the tube for a<r<b. Calculate the electric field in terms of αα and the distance r from the axis of the tube for r>b. What is the charge per unit length on the inner surface of the tube? What is the charge per unit length on the outer surface of the tube?arrow_forwardTwo small insulating spheres with radius 9.00×10−2 m are separated by a large center-to-center distance of 0.545 m . One sphere is negatively charged, with net charge -1.75 μC , and the other sphere is positively charged, with net charge 3.70 μC . The charge is uniformly distributed within the volume of each sphere. What is the magnitude E of the electric field midway between the spheres? Take the permittivity of free space to be ϵ0 = 8.85×10−12 C2/(N⋅m2) . What is the direction of the electric field midway between the spheres?arrow_forwardA conducting spherical shell with inner radius aa and outer radius bb has a positive point charge Q located at its center. The total charge on the shell is -3Q, and it is insulated from its surroundings. Derive the expression for the electric field magnitude in terms of the distance r from the center for the region r<a. Express your answer in terms of some or all of the variables Q, a, b, and appropriate constants. Derive the expression for the electric field magnitude in terms of the distance rr from the center for the region a<r<b. Derive the expression for the electric field magnitude in terms of the distance rr from the center for the region r>b. What is the surface charge density on the inner surface of the conducting shell? What is the surface charge density on the outer surface of the conducting shell?arrow_forward
- A small sphere with a mass of 3.00×10−3 g and carrying a charge of 4.80×10−8 C hangs from a thread near a very large, charged insulating sheet, as shown in the figure (Figure 1). The charge density on the sheet is −2.20×10−9 C/m2 . Find the angle of the thread.arrow_forwardA small conducting spherical shell with inner radius aa and outer radius bb is concentric with a larger conducting spherical shell with inner radius c and outer radius d (Figure 1). The inner shell has total charge +2q, and the outer shell has charge −2q. Calculate the magnitude of the electric field in terms of q and the distance rr from the common center of the two shells for r<a. Calculate the magnitude of the electric field for a<r<b. Calculate the magnitude of the electric field for b<r<c.arrow_forwardA cube has sides of length L = 0.800 m . It is placed with one corner at the origin as shown in the figure. The electric field is not uniform but is given by E→=αxi^+βzk^, where α=−3.90 and β= 7.10. What is the sum of the flux through the surface S5 and S6? What is the sum of the flux through the surface S2 and S4? Find the total electric charge inside the cube.arrow_forward
- In the figure, a proton is projected horizontally midway between two parallel plates that are separated by 0.6 cm. The electrical field due to the plates has magnitude 450000 N/C between the plates away from the edges. If the plates are 3 cm long, find the minimum speed of the proton if it just misses the lower plate as it emerges from the field.arrow_forwardA point charge of magnitude q is at the center of a cube with sides of length L. What is the electric flux Φ through each of the six faces of the cube? What would be the flux Φ1 through a face of the cube if its sides were of length L1? Please explain everything.arrow_forwardIf a 1/2 inch diameter drill bit spins at 3000 rotations per minute, how fast is the outer edge moving as it contacts a piece of metal while drilling a machine part?arrow_forward
 Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
 Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





