
Concept explainers
Mixing Suppose a brine containing
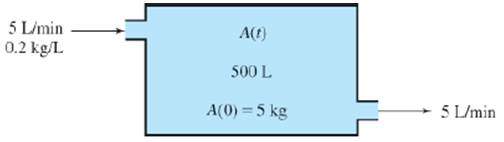
Figure 2.6 Mixing problem with equal flow rates
a. Find the concentration, in kilograms per liter, of salt in the tank after
A further discussion of mixing problems is given in Section 3.2.]
b. After
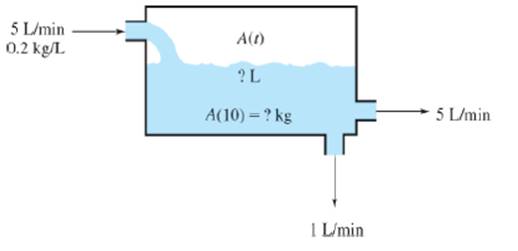 Figure 2.7 Mixing problem with unequal flow rates
Figure 2.7 Mixing problem with unequal flow rates
Want to see the full answer?
Check out a sample textbook solution
Chapter 2 Solutions
Fundamentals of Differential Equations and Boundary Value Problems
- 9. Spectral Theorem: Let A be a symmetric matrix A- (14) a) Find the eigenvalues and eigenvectors of A. b) Show that A is diagonalizable. c) Verify the spectral theorem by diagonalizing A.arrow_forwardGiven the system of linear equations 2xyz=1, x-3y+2z = 4, 3x+2y+3z = 5 a) Represent the system as Ax = b where A is a matrix, and solve for x using Cramer's rule. b) Compute the determinant of matrix A and discuss its implications on the solvability of the system.arrow_forward43 Game Theory: Nash Equilibrium Task: Refer to Question 43 in the provided document. Link: https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440AZF/view?usp=sharingarrow_forward
- For context, the image provided below is a quesion from a Sepetember, 2024 past paper in statistical modelingarrow_forwardFor context, the images attached below (the question and the related figure) is from a january 2024 past paperarrow_forwardFor context, the image attached below is a question from a June 2024 past paper in statisical modelingarrow_forward
- For context, the images attached below are a question from a June, 2024 past paper in statistical modelingarrow_forwardFor context, the images attached below (question and related graph) are from a February 2024 past paper in statistical modelingarrow_forwardFor context, the images attached below are from a February 2024 past paper in statistical modelingarrow_forward
- For context, the image provided below is a question from a September, 2024 past paper in statistical modelingarrow_forwardFor context, the image below is from a January 2024 past paper in statistical modelingarrow_forwardFor context, the image provided below is a question from a September, 2024 past paper in statistical modelingarrow_forward
 Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
 College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage





