
(a)
To show that the minimum spanning tree is unique but not the second best spanning tree.
(a)
Explanation of Solution
Suppose there are 4 vertices { p, q, r, s } in the following graph. Consider the edge weights and vertices as follows:
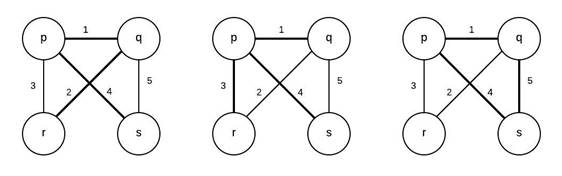
The minimum spanning tree has weight of 7 and there exists two second best minimum spanning tree having weight 8.
(b)
To prove that there exists edges ( u , v )
(b)
Explanation of Solution
T is the minimum spanning tree of graph G . Consider adding an edge that is ( u , v )
(c)
To describe an O (
(c)
Explanation of Solution
The algorithm is as follows:
Consider using dynamic
(d)
To give an efficient algorithm to compute the second-best minimum spanning tree of graph G.
(d)
Explanation of Solution
Firstly, compute the minimum spanning tree in O ( E + Vlog ( V )), this is in time O (
Want to see more full solutions like this?
- "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forwardSolve this "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forward"Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forward
- "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forwardSpecifications: Part-1Part-1: DescriptionIn this part of the lab you will build a single operation ALU. This ALU will implement a bitwise left rotation. Forthis lab assignment you are not allowed to use Digital's Arithmetic components.IF YOU ARE FOUND USING THEM, YOU WILL RECEIVE A ZERO FOR LAB2!The ALU you will be implementing consists of two 4-bit inputs (named inA and inB) and one 4-bit output (named out). Your ALU must rotate the bits in inA by the amount given by inB (i.e. 0-15).Part-1: User InterfaceYou are provided an interface file lab2_part1.dig; start Part-1 from this file.NOTE: You are not permitted to edit the content inside the dotted lines rectangle. Part-1: ExampleIn the figure above, the input values that we have selected to test are inA = {inA_3, inA_2, inA_1, inA_0} = {0, 1, 0,0} and inB = {inB_3, inB_2, inB_1, inB_0} = {0, 0, 1, 0}. Therefore, we must rotate the bus 0100 bitwise left by00102, or 2 in base 10, to get {0, 0, 0, 1}. Please note that a rotation left is…arrow_forwardSolve this "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forward
- Solve this "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forward"Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forwardSolve this "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forward
- Solve this "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forwardSpecifications: Part-2Part-2: DescriptionIn this part of the lab, we will illuminate two 7-segment displays. You will need to understand 2's Complement todetermine when the input 4-bit binary number corresponds to a negative or positive number. To understand how anLED display works in Digital, please refer to the playWithLED_Display.dig file provided. You should play withdifferent input combinations to see how it influences the LED Display value. In the screenshot below, note how I wasable to generate the display of “3” on the Hex display by lighting up only certain input wires to the unit. Here is a picture of how the different segments light up to produce the different displays: Note in the picture above that we showed displays only from 0-8 since in 4-bit 2s complement representation, 8 is thelargest modulus value you can represent (the range of integers would be -8 to +7).Your circuit in Part-2 must accept a 4-bit 2's complement input {in3, in2, in1, in0} where in3 is the most…arrow_forwardSolve this "Do not use AI tools. Solve the problem by hand on paper only and upload a photo of your handwritten solution."arrow_forward
 Operations Research : Applications and AlgorithmsComputer ScienceISBN:9780534380588Author:Wayne L. WinstonPublisher:Brooks Cole
Operations Research : Applications and AlgorithmsComputer ScienceISBN:9780534380588Author:Wayne L. WinstonPublisher:Brooks Cole Fundamentals of Information SystemsComputer ScienceISBN:9781305082168Author:Ralph Stair, George ReynoldsPublisher:Cengage Learning
Fundamentals of Information SystemsComputer ScienceISBN:9781305082168Author:Ralph Stair, George ReynoldsPublisher:Cengage Learning C++ Programming: From Problem Analysis to Program...Computer ScienceISBN:9781337102087Author:D. S. MalikPublisher:Cengage Learning
C++ Programming: From Problem Analysis to Program...Computer ScienceISBN:9781337102087Author:D. S. MalikPublisher:Cengage Learning C++ for Engineers and ScientistsComputer ScienceISBN:9781133187844Author:Bronson, Gary J.Publisher:Course Technology Ptr
C++ for Engineers and ScientistsComputer ScienceISBN:9781133187844Author:Bronson, Gary J.Publisher:Course Technology Ptr



