
Concept explainers
Expand Your knowledge: Decimal Data The fallowing data represent tonnes of wheat harvested each year (1894-1925) from Plot 19 at the Rothamsted Agricultural Experiment Stations, England.
| 2.71 | 1.62 | 2.60 | 1.64 | 2.20 | 2.02 | 1.67 | 1.99 | 2.34 | 1.26 | 1.31 |
| 1.80 | 2.82 | 2.15 | 2.07 | 1.62 | 1.47 | 2.19 | 0.59 | 1.48 | 0.77 | 1.04 |
| 1.32 | 0.89 | 1.35 | 0.95 | 0.94 | 1.39 | 1.19 | 1.18 | 0.46 | 0.70 |
(a) Multiply each data value by 100 to “clear" the decimals.
(b) Use the standard procedures of this section to make a frequency table and histogram with your whole-number data. Use six classes.
(c) Divide class limits, class boundaries, and class midpoints by 100 to get back to your original data values.
(a)
To find: The data that are multiply with 100 for each value in the data..
Answer to Problem 21P
Solution: The data multiply with 100 for each value in the data is as follows:
| data | data*100 | data | data*100 | data | data*100 | data | data*100 |
| 2.71 | 271 | 2.34 | 234 | 1.47 | 147 | 1.35 | 135 |
| 1.62 | 162 | 1.26 | 126 | 2.19 | 219 | 0.95 | 95 |
| 2.6 | 260 | 1.31 | 131 | 0.59 | 59 | 0.94 | 94 |
| 1.64 | 164 | 1.8 | 180 | 1.48 | 148 | 1.39 | 139 |
| 2.2 | 220 | 2.82 | 282 | 0.77 | 77 | 1.19 | 119 |
| 2.02 | 202 | 2.15 | 215 | 2.04 | 204 | 1.18 | 118 |
| 1.67 | 167 | 2.07 | 207 | 1.32 | 132 | 0.46 | 46 |
| 1.99 | 199 | 1.62 | 162 | 0.89 | 89 | 0.7 | 70 |
Explanation of Solution
Calculation: The data represent the tons of wheat harvested each year and there are 32 values in the data set. To find the decimal data in to “clear” data that multiply with each value in the data by 100. The calculation is as follows:
| Data | Data*100 | Data | Data*100 | Data | Data*100 | Data | Data*100 |
| 2.71 | 2.34 | 234 | 1.47 | 147 | 1.35 | 135 | |
| 1.62 | 1.26 | 126 | 2.19 | 219 | 0.95 | 95 | |
| 2.6 | 1.31 | 131 | 0.59 | 59 | 0.94 | 94 | |
| 1.64 | 164 | 1.8 | 180 | 1.48 | 148 | 1.39 | 139 |
| 2.2 | 220 | 2.82 | 282 | 0.77 | 77 | 1.19 | 119 |
| 2.02 | 202 | 2.15 | 215 | 2.04 | 204 | 1.18 | 118 |
| 1.67 | 167 | 2.07 | 207 | 1.32 | 132 | 0.46 | 46 |
| 1.99 | 199 | 1.62 | 162 | 0.89 | 89 | 0.7 | 70 |
Interpretation: Hence, the data multiplied with 100 is as follows:
| Data | Data*100 | Data | Data*100 | Data | Data*100 | Data | Data*100 |
| 2.71 | 271 | 2.34 | 234 | 1.47 | 147 | 1.35 | 135 |
| 1.62 | 162 | 1.26 | 126 | 2.19 | 219 | 0.95 | 95 |
| 2.6 | 260 | 1.31 | 131 | 0.59 | 59 | 0.94 | 94 |
| 1.64 | 164 | 1.8 | 180 | 1.48 | 148 | 1.39 | 139 |
| 2.2 | 220 | 2.82 | 282 | 0.77 | 77 | 1.19 | 119 |
| 2.02 | 202 | 2.15 | 215 | 2.04 | 204 | 1.18 | 118 |
| 1.67 | 167 | 2.07 | 207 | 1.32 | 132 | 0.46 | 46 |
| 1.99 | 199 | 1.62 | 162 | 0.89 | 89 | 0.7 | 70 |
(b)
To find: The class width, class limits, class boundaries, midpoint, frequency, relative frequency, and cumulative frequency of the data..
Answer to Problem 21P
Solution: The complete frequency table is as follows:
| class limits | class boundaries | midpoints | freq | relative freq | cumulative freq |
| 46-85 | 45.5-85.5 | 65.5 | 4 | 0.12 | 4 |
| 86-125 | 85.5-125.5 | 105.5 | 5 | 0.16 | 9 |
| 126-165 | 125.5-165.5 | 145.5 | 10 | 0.31 | 19 |
| 166-205 | 165.5-205.5 | 185.5 | 5 | 0.16 | 24 |
| 206-245 | 205.5-245.5 | 225.5 | 5 | 0.16 | 29 |
| 246-285 | 245.5-285.5 | 265.5 | 3 | 0.09 | 32 |
Explanation of Solution
Calculation: To find the class width for the whole data of 32 values, it is observed that largest value of the data set is 282 and the smallest value is 46 in the data. Using 6 classes, the class width calculated in the following way:
The value is round up to the nearest whole number. Hence, the class width of the data set is 40. The class width for the data is 40 and the lowest data value (46) will be the lower class limit of the first class. Because the class width is 40, it must add 40 to the lowest class limit in the first class to find the lowest class limit in the second class. There are 6 desired classes. Hence, the class limits are 46–85, 86–125, 126–165, 166–205, 206–245, and 246–285. Now, to find the class boundaries subtract 0.5 from lower limit of every class and add 0.5 to the upper limit of every class interval. Hence, the class boundaries are 45.5–85.5, 85.5–125.5, 125.5–165.5, 165.5–205.5, 205.5–245.5, and 245.5–285.5.
Next to find the midpoint of the class is calculated by using formula,
Midpoint of first class is calculated as:
The frequencies for respective classes are 4, 5, 10, 5, 5, and 3.
Relative frequency is calculated by using the formula
The frequency for 1st class is 4 and total frequencies are 32 so the relative frequency is
The calculated frequency table is as follows:
| Class limits | Class boundaries | Midpoints | Freq | Relative freq | Cumulative freq |
| 46-85 | 45.5-85.5 | 65.5 | 4 | 0.12 | 4 |
| 86-125 | 85.5-125.5 | 105.5 | 5 | 0.16 | 9 |
| 126-165 | 125.5-165.5 | 145.5 | 10 | 0.31 | 19 |
| 166-205 | 165.5-205.5 | 185.5 | 5 | 0.16 | 24 |
| 206-245 | 205.5-245.5 | 225.5 | 5 | 0.16 | 29 |
| 246-285 | 245.5-285.5 | 265.5 | 3 | 0.09 | 32 |
Graph: To construct the histogram by using the MINITAB, the steps are as follows:
Step 1: Enter the class boundaries in C1 and frequency in C2.
Step 2: Go to Graph > Histogram > Simple.
Step 3: Enter C1 in Graph variable then go to Data options > Frequency > C2.
Step 4: Click on OK.
The obtained histogram is as follows:
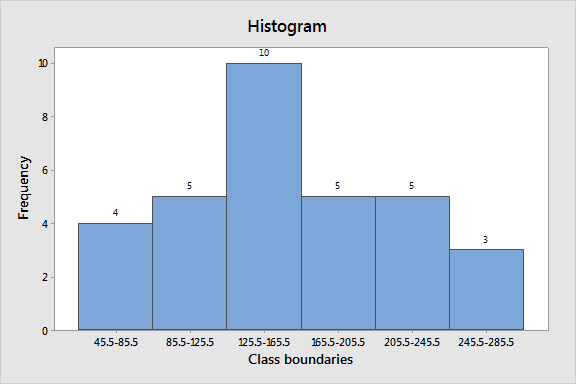
Interpretation: Hence, the complete frequency table is as follows:
| Class limits | Class boundaries | Midpoints | Freq | Relative freq | Cumulative freq |
| 46-85 | 45.5-85.5 | 65.5 | 4 | 0.12 | 4 |
| 86-125 | 85.5-125.5 | 105.5 | 5 | 0.16 | 9 |
| 126-165 | 125.5-165.5 | 145.5 | 10 | 0.31 | 19 |
| 166-205 | 165.5-205.5 | 185.5 | 5 | 0.16 | 24 |
| 206-245 | 205.5-245.5 | 225.5 | 5 | 0.16 | 29 |
| 246-285 | 245.5-285.5 | 265.5 | 3 | 0.09 | 32 |
(c)
To find: The class limits, class boundaries, and midpoints in the table by dividing 100..
Answer to Problem 21P
Solution: The frequency table of original data is as:
| class limits | class boundaries | Midpoints |
| 0.46-0.85 | 0.455-0.855 | 0.655 |
| 0.86-1.25 | 0.855-1.255 | 1.055 |
| 1.26-1.65 | 1.255-1.655 | 1.455 |
| 1.66-2.05 | 1.655-2.055 | 1.855 |
| 2.06-2.45 | 2.055-2.455 | 2.255 |
| 2.46-2.85 | 2.455-2.855 | 2.655 |
Explanation of Solution
Calculation: The frequency table for whole number is obtained in above part. It is the data that multiply each value by 100 to ‘clear’ decimals from the data. The frequency table for whole number is as follows:
| class limits | class boundaries | Midpoints |
| 46-85 | 45.5-85.5 | 65.5 |
| 86-125 | 85.5-125.5 | 105.5 |
| 126-165 | 125.5-165.5 | 145.5 |
| 166-205 | 165.5-205.5 | 185.5 |
| 206-245 | 205.5-245.5 | 225.5 |
| 246-285 | 245.5-285.5 | 265.5 |
To find the decimal or original data, divide the class limits, class boundaries and midpoints by 100. The calculation as follows:
| class limits | class boundaries | Midpoints |
| 0.46-0.85 | 0.455-0.855 | 0.655 |
| 0.86-1.25 | 0.855-1.255 | 1.055 |
| 1.26-1.65 | 1.255-1.655 | 1.455 |
| 1.66-2.05 | 1.655-2.055 | 1.855 |
| 2.06-2.45 | 2.055-2.455 | 2.255 |
| 2.46-2.85 | 2.455-2.855 | 2.655 |
Interpretation: Hence, the data divide by 100 is as:
| class limits | class boundaries | Midpoints |
| 0.46-0.85 | 0.455-0.855 | 0.655 |
| 0.86-1.25 | 0.855-1.255 | 1.055 |
| 1.26-1.65 | 1.255-1.655 | 1.455 |
| 1.66-2.05 | 1.655-2.055 | 1.855 |
| 2.06-2.45 | 2.055-2.455 | 2.255 |
| 2.46-2.85 | 2.455-2.855 | 2.655 |
Want to see more full solutions like this?
Chapter 2 Solutions
EBK UNDERSTANDING BASIC STATISTICS
- A marketing agency wants to determine whether different advertising platforms generate significantly different levels of customer engagement. The agency measures the average number of daily clicks on ads for three platforms: Social Media, Search Engines, and Email Campaigns. The agency collects data on daily clicks for each platform over a 10-day period and wants to test whether there is a statistically significant difference in the mean number of daily clicks among these platforms. Conduct ANOVA test. You can provide your answer by inserting a text box and the answer must include: also please provide a step by on getting the answers in excel Null hypothesis, Alternative hypothesis, Show answer (output table/summary table), and Conclusion based on the P value.arrow_forwardA company found that the daily sales revenue of its flagship product follows a normal distribution with a mean of $4500 and a standard deviation of $450. The company defines a "high-sales day" that is, any day with sales exceeding $4800. please provide a step by step on how to get the answers Q: What percentage of days can the company expect to have "high-sales days" or sales greater than $4800? Q: What is the sales revenue threshold for the bottom 10% of days? (please note that 10% refers to the probability/area under bell curve towards the lower tail of bell curve) Provide answers in the yellow cellsarrow_forwardBusiness Discussarrow_forward
- The following data represent total ventilation measured in liters of air per minute per square meter of body area for two independent (and randomly chosen) samples. Analyze these data using the appropriate non-parametric hypothesis testarrow_forwardeach column represents before & after measurements on the same individual. Analyze with the appropriate non-parametric hypothesis test for a paired design.arrow_forwardShould you be confident in applying your regression equation to estimate the heart rate of a python at 35°C? Why or why not?arrow_forward
 Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt





