Chapter 17, Problem 7QP

Agency Costs Fountain Corporation’s economists estimate that a good business environment and a bad business environment are equally likely for the coming year. The managers of the company must choose between two mutually exclusive projects. Assume that the project the company chooses will be the firm’s only activity and that the firm will close one year from today. The company is obligated to make a $3,500 payment to bondholders at the end of the year. The projects have the same systematic risk but different volatilities. Consider the following information pertaining to the two projects:

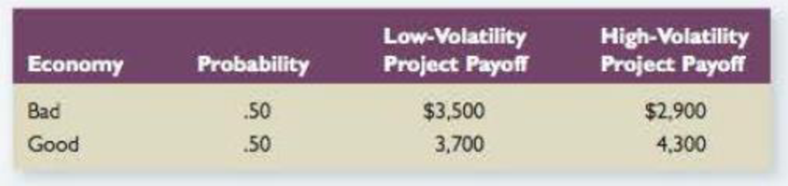
1. a. What is the expected value of the company if the low-volatility project is undertaken? What if the high-volatility project is undertaken? Which of the two strategies maximizes the expected value of the firm?
2. b. What is the expected value of the company’s equity if the low-volatility project is undertaken? What is it if the high-volatility project is undertaken?
3. c. Which project would the company’s stockholders prefer’? Explain.
4. d. Suppose bondholders arc fully aware that stockholders might choose to maximize equity value rather than total firm value and opt for the high-volatility project. To minimize this agency cost, the firm's bondholders decide to use a bond covenant to stipulate that the bondholders can demand a higher payment if the company chooses to take on the high-volatility project. What payment to bondholders would make stockholders indifferent between the two projects?

Summary Introduction

To determine: The expected value of the firm if the low volatility project is undertaken.

Introduction:

Cost of equity: It is a return that a company pays to its equity investors. A company’s equity cost signifies the compensation the market demands in substitute for owning the possessions and bearing the ownership risks

Explanation of Solution

The expected value of every project is the total of the probability of each state of the economy times the value in that economy state.

As this is only project for the firm, the firm value will be similar as the project value.

Calculate the low-volatility project value:

The probability of bad is 0.50, project payoff value (low volatility) for bad is $3,500 and project payoff value (low volatility) for good is $3,700.

$\begin{array}{c}\text{Low volatility project value}=\left[\begin{array}{l}\text{Probability}\left({\text{Bad payoff value}}_{\text{Low volatility}}\right)+\\ \text{Probability}\left({\text{Good payoff value}}_{\text{Low volatility}}\right)\end{array}\right]\\ =0.50\left(\$3,500\right)+0.50\left(\$3,700\right)\\ =\$1,750+\$1,850\\ =\$3,600\end{array}$

Therefore, the low volatility project value is $3,600.

Calculate the high-volatility project value:

The probability of good is 0.50, project payoff value (high volatility) for bad is $2,900 and project payoff value (high volatility) for good is $4,300.

$\begin{array}{c}\text{High volatility project value}=\left[\begin{array}{l}\text{Probability}\left({\text{Bad payoff value}}_{\text{High volatility}}\right)+\\ \text{Probability}\left({\text{Good payoff value}}_{\text{High volatility}}\right)\end{array}\right]\\ =0.50\left(\$2,900\right)+0.50\left(\$4,300\right)\\ =\$1,450+\$2,150\\ =\$3,600\end{array}$

Therefore, the high volatility project value is $3,600.

Summary Introduction

To determine: The expected value of the company’s equity if low volatility and high volatility project is undertaken.

Explanation of Solution

Explanation:

The equity value is the residual value of the firm after the company pays off bondholders. If the low-volatility project is decided, the company’s equity will be worth of $0 if the economy is bad and $200 if the economy is good. As these two scenarios are evenly probable, the anticipated value of the company’s equity is as follows:

Calculate the expected value of equity with low volatility project:

$\begin{array}{c}\text{Low volatility project value}=\left[\begin{array}{l}\text{Probability}\left({\text{Equity worth}}_{\text{Bad}}\right)+\\ \text{Probability}\left({\text{Equity worth}}_{\text{Good}}\right)\end{array}\right]\\ =0.50\left(\$0\right)+0.50\left(\$200\right)\\ =\$0+\$100\\ =\$100\end{array}$

Therefore, the expected value of equity with low volatility project value is $100.

Calculate the expected value of equity with high volatility project:

$\begin{array}{c}\text{High volatility project value}=\left[\begin{array}{l}\text{Probability}\left({\text{Equity worth}}_{\text{Bad}}\right)+\\ \text{Probability}\left({\text{Equity worth}}_{\text{Good}}\right)\end{array}\right]\\ =0.50\left(\$0\right)+0.50\left(\$800\right)\\ =\$0+\$400\\ =\$400\end{array}$

Therefore, the expected value of equity with high volatility project value is $400.

Summary Introduction

To determine: Which project should the company’s stockholder prefer.

Explanation of Solution

Risk-neutral investors should prefer the strategy with the greater expected value. Hence, the firm’s stockholders should choose the high-volatility project, since it maximize the anticipated worth of the firm’s equity.

Summary Introduction

To determine: The returns that bondholders would make stockholders indifferent among the two given projects.

Explanation of Solution

To make stockholders in-different among the lower-volatility project and the higher-volatility project, the bondholders will require increase their required debt-payment so that the anticipated equity worth if the high-volatility project is chosen is equal to the expected worth of equity if the lower-volatility project is chosen. As shown in part b, the expected value of equity when the lower-volatility project selected is $100.

If the high-volatility project is preferred, the value of the company will have around $2,900 if the economy is bad and $4,300 when the economy is good. When the economy is under bad, the whole $2,900 will go to the stockholders and bondholders will obtain nothing.

If the economy is under good, stockholders will obtain the dissimilarity among $4,300, the overall worth of the company, and the necessary debt payment. Assume, X as the debt payment that bondholders will need if the high-volatility project is preferred. For stockholders to be dissimilar among the two projects, the anticipated equity value if the high-volatility project is preferred must be equal to $100.

Determine the value of X:

$\begin{array}{c}{\text{Expected value of equity}}_{\text{Lower votality}}=\left(\begin{array}{l}{\text{Probability}}_1\left(\text{Equity worth}\right)\\ +{\text{Probaility}}_2\left({\text{Value of compay}}_{\text{Bad}}-\text{X}\right)\end{array}\right)\\ 100=0.5\left(0\right)+0.5\left(4,300-\text{X}\right)\\ 100=0+2,150-0.5\text{X}\\ \text{0}\text{.5X}=\$2,150-100\\ =\frac{2,050}{0.5}\\ =\$4,100\end{array}$

Therefore, the debt payment value will be $4,100,