Multivariable Calculus
8th Edition
ISBN: 9781305266643
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 16.1, Problem 30E
Match the functions f with the plots of their gradient
30. f(x, y) = x(x + y)
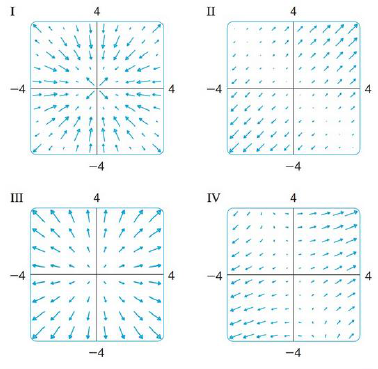
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
3.
Consider the sequences of functions f₁: [-π, π] → R,
sin(n²x)
An(2)
n
f pointwise as
(i) Find a function ƒ : [-T,π] → R such that fn
n∞. Further, show that fn →f uniformly on [-π,π] as n → ∞.
[20 Marks]
(ii) Does the sequence of derivatives f(x) has a pointwise limit on [-7, 7]?
Justify your answer.
[10 Marks]
1. (i) Give the definition of a metric on a set X.
[5 Marks]
(ii) Let X = {a, b, c} and let a function d : XxX → [0, ∞) be defined
as d(a, a) = d(b,b) = d(c, c) 0, d(a, c) = d(c, a) 1, d(a, b) = d(b, a) = 4,
d(b, c) = d(c,b) = 2. Decide whether d is a metric on X. Justify your answer.
=
(iii) Consider a metric space (R, d.), where
=
[10 Marks]
0
if x = y,
d* (x, y)
5
if xy.
In the metric space (R, d*), describe:
(a) open ball B2(0) of radius 2 centred at 0;
(b) closed ball B5(0) of radius 5 centred at 0;
(c) sphere S10 (0) of radius 10 centred at 0.
[5 Marks]
[5 Marks]
[5 Marks]
(c) sphere S10 (0) of radius 10 centred at 0.
[5 Marks]
2. Let C([a, b]) be the metric space of continuous functions on the interval
[a, b] with the metric
doo (f,g)
=
max f(x)g(x)|.
xЄ[a,b]
= 1x. Find:
Let f(x) = 1 - x² and g(x):
(i) do(f, g) in C'([0, 1]);
(ii) do(f,g) in C([−1, 1]).
[20 Marks]
[20 Marks]
Chapter 16 Solutions
Multivariable Calculus
Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...Ch. 16.1 - Sketch the vector field F by drawing a diagram...
Ch. 16.1 - Match the vector fields F with the plots labeled...Ch. 16.1 - Match the vector fields F with the plots labeled...Ch. 16.1 - Match the vector fields F with the plots labeled...Ch. 16.1 - Match the vector fields F with the plots labeled...Ch. 16.1 - Match the vector fields F on 3 with the plots...Ch. 16.1 - Match the vector fields F on 3 with the plots...Ch. 16.1 - Match the vector fields F on 3 with the plots...Ch. 16.1 - Match the vector fields F on 3 with the plots...Ch. 16.1 - Prob. 21ECh. 16.1 - Prob. 22ECh. 16.1 - Find the gradient vector field of f. 23. f(x, y,...Ch. 16.1 - Find the gradient vector field of f. 24. f(x, y,...Ch. 16.1 - Find the gradient vector field f of f and sketch...Ch. 16.1 - Find the gradient vector field f of f and sketch...Ch. 16.1 - Match the functions f with the plots of their...Ch. 16.1 - Match the functions f with the plots of their...Ch. 16.1 - Match the functions f with the plots of their...Ch. 16.1 - Match the functions f with the plots of their...Ch. 16.1 - A particle moves in a velocity field V(x, y) = x2,...Ch. 16.1 - At time t = 1, a particle is located at position...Ch. 16.1 - The flow lines (or streamlines) of a vector field...Ch. 16.1 - (a) Sketch the vector field F(x, y) = i + x j and...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Evaluate the line integral, where C is the given...Ch. 16.2 - Prob. 16ECh. 16.2 - Let F be the vector field shown in the figure. (a)...Ch. 16.2 - The figure shows a vector field F and two curves...Ch. 16.2 - Prob. 19ECh. 16.2 - Prob. 20ECh. 16.2 - Evaluate the line integral C F dr, where C is...Ch. 16.2 - Evaluate the line integral C F dr, where C is...Ch. 16.2 - Use a calculator to evaluate the line integral...Ch. 16.2 - Use a calculator to evaluate the line integral...Ch. 16.2 - Use a calculator to evaluate the line integral...Ch. 16.2 - Prob. 26ECh. 16.2 - Prob. 31ECh. 16.2 - (a) Find the work done by the force field F(x, y)...Ch. 16.2 - A thin wire is bent into the shape of a semicircle...Ch. 16.2 - A thin wire has the shape of the first-quadrant...Ch. 16.2 - Prob. 35ECh. 16.2 - Prob. 36ECh. 16.2 - If a wire with linear density (x, y) lies along a...Ch. 16.2 - If a wire with linear density (x, y, z) lies along...Ch. 16.2 - Find the work done by the force field F(x, y) = x...Ch. 16.2 - Find the work done by the force field F(x, y) = x2...Ch. 16.2 - Find the work done by the force field F(x, y, z) =...Ch. 16.2 - The force exerted by an electric charge at the...Ch. 16.2 - Prob. 43ECh. 16.2 - Prob. 44ECh. 16.2 - A 160-lb man carries a 25-lb can of paint up a...Ch. 16.2 - Suppose there is a hole in the can of paint in...Ch. 16.2 - (a) Show that a constant force field does zero...Ch. 16.2 - The base of a circular fence with radius 10 m is...Ch. 16.2 - Prob. 49ECh. 16.2 - Prob. 50ECh. 16.2 - An object moves along the curve C shown in the...Ch. 16.2 - Experiments show that a steady current I in a long...Ch. 16.3 - The figure shows a curve C and a contour map of a...Ch. 16.3 - A table of values of a function f with continuous...Ch. 16.3 - Determine whether or not F is a conservative...Ch. 16.3 - Determine whether or not F is a conservative...Ch. 16.3 - Determine whether or not F is a conservative...Ch. 16.3 - Determine whether or not F is a conservative...Ch. 16.3 - Determine whether or not F is a conservative...Ch. 16.3 - Determine whether or not F is a conservative...Ch. 16.3 - Determine whether or not F is a conservative...Ch. 16.3 - Prob. 10ECh. 16.3 - The figure shows the vector field F(x, y) = 2xy,...Ch. 16.3 - Prob. 12ECh. 16.3 - (a) Find a function f such that F = f and (b) use...Ch. 16.3 - (a) Find a function f such that F = f and (b) use...Ch. 16.3 - (a) Find a function f such that F = f and (b) use...Ch. 16.3 - Prob. 16ECh. 16.3 - Prob. 17ECh. 16.3 - (a) Find a function f such that F = f and (b) use...Ch. 16.3 - Show that the line integral is independent of path...Ch. 16.3 - Show that the line integral is independent of path...Ch. 16.3 - Prob. 21ECh. 16.3 - Prob. 22ECh. 16.3 - Find the work done by the force field F in moving...Ch. 16.3 - Find the work done by the force field F in moving...Ch. 16.3 - Is the vector field shown in the figure...Ch. 16.3 - Is the vector field shown in the figure...Ch. 16.3 - Let F = f, where f(x, y) = sin(x 2y). Find...Ch. 16.3 - Prob. 29ECh. 16.3 - Use Exercise 29 to show that the line integral C y...Ch. 16.3 - Prob. 31ECh. 16.3 - Prob. 32ECh. 16.3 - Prob. 33ECh. 16.3 - Prob. 34ECh. 16.3 - Let F(x, y) = yi+xjx2+y2 (a) Show that P/y=Q/x....Ch. 16.4 - Evaluate the line integral by two methods: (a)...Ch. 16.4 - Evaluate the line integral by two methods: (a)...Ch. 16.4 - Evaluate the line integral by two methods: (a)...Ch. 16.4 - Evaluate the line integral by two methods: (a)...Ch. 16.4 - Use Greens Theorem to evaluate the line integral...Ch. 16.4 - Use Greens Theorem to evaluate the line integral...Ch. 16.4 - Use Greens Theorem to evaluate the line integral...Ch. 16.4 - Use Greens Theorem to evaluate the line integral...Ch. 16.4 - Use Greens Theorem to evaluate the line integral...Ch. 16.4 - Use Greens Theorem to evaluate the line integral...Ch. 16.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 16.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 16.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 16.4 - Use Greens Theorem to evaluate C F dr. (Check the...Ch. 16.4 - Prob. 17ECh. 16.4 - A particle starts at the origin, moves along the...Ch. 16.4 - Use one of the formulas in (5) to find the area...Ch. 16.4 - If a circle C with radius 1 rolls along the...Ch. 16.4 - (a) If C is the line segment connecting the point...Ch. 16.4 - Let D be a region bounded by a simple closed path...Ch. 16.4 - Use Exercise 22 to find the centroid of a...Ch. 16.4 - Use Exercise 22 to find the centroid of the...Ch. 16.4 - A plane lamina with constant density (x, y) = ...Ch. 16.4 - Prob. 26ECh. 16.4 - Use the method of Example 5 to calculate C F dr,...Ch. 16.4 - Calculate C F dr, where F(x, y) = x2 + y, 3x y2...Ch. 16.4 - If F is the vector field of Example 5, show that C...Ch. 16.4 - Complete the proof of the special case of Greens...Ch. 16.4 - Use Greens Theorem to prove the change of...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - Find (a) the curl and (b) the divergence of the...Ch. 16.5 - The vector field F is shown in the xy-plane and...Ch. 16.5 - The vector field F is shown in the xy-plane and...Ch. 16.5 - The vector field F is shown in the xy-plane and...Ch. 16.5 - Let f be a scalar field and F a vector field....Ch. 16.5 - Prob. 13ECh. 16.5 - Determine whether or not the vector field is...Ch. 16.5 - Determine whether or not the vector field is...Ch. 16.5 - Determine whether or not the vector field is...Ch. 16.5 - Determine whether or not the vector field is...Ch. 16.5 - Determine whether or not the vector field is...Ch. 16.5 - Is there a vector field G on 3 such that curl G =...Ch. 16.5 - Is there a vector field G on 3 such that curl G =...Ch. 16.5 - Show that any vector field of the form F(x, y, z)...Ch. 16.5 - Show that any vector field of the form F(x, y, z)...Ch. 16.5 - Prove the identity, assuming that the appropriate...Ch. 16.5 - Prove the identity, assuming that the appropriate...Ch. 16.5 - Prove the identity, assuming that the appropriate...Ch. 16.5 - Prove the identity, assuming that the appropriate...Ch. 16.5 - Prove the identity, assuming that the appropriate...Ch. 16.5 - Prove the identity, assuming that the appropriate...Ch. 16.5 - Prove the identity, assuming that the appropriate...Ch. 16.5 - Let r = x i + y j + z k and r = |r|. 30. Verify...Ch. 16.5 - Let r = x i + y j + z k and r = |r|. 31. Verify...Ch. 16.5 - Let r = x i + y j + z k and r = |r|. 32. If F =...Ch. 16.5 - Use Greens Theorem in the form of Equation 13 to...Ch. 16.5 - Prob. 34ECh. 16.5 - Prob. 35ECh. 16.5 - Prob. 36ECh. 16.5 - This exercise demonstrates a connection between...Ch. 16.5 - Maxwells equations relating the electric field E...Ch. 16.5 - We have seen that all vector fields of the form F...Ch. 16.6 - Determine whether the points P and Q lie on the...Ch. 16.6 - Determine whether the points P and Q lie on the...Ch. 16.6 - Identify the surface with the given vector...Ch. 16.6 - Prob. 4ECh. 16.6 - Prob. 5ECh. 16.6 - Prob. 6ECh. 16.6 - Match the equations with the graphs labeled IVI...Ch. 16.6 - Match the equations with the graphs labeled IVI...Ch. 16.6 - Match the equations with the graphs labeled IVI...Ch. 16.6 - Match the equations with the graphs labeled IVI...Ch. 16.6 - Prob. 17ECh. 16.6 - Match the equations with the graphs labeled IVI...Ch. 16.6 - Find a parametric representation for the surface....Ch. 16.6 - Prob. 20ECh. 16.6 - Find a parametric representation for the surface....Ch. 16.6 - Find a parametric representation for the surface....Ch. 16.6 - Find a parametric representation for the surface....Ch. 16.6 - Find a parametric representation for the surface....Ch. 16.6 - Prob. 25ECh. 16.6 - Find a parametric representation for the surface....Ch. 16.6 - Prob. 29ECh. 16.6 - Find parametric equations for the surface obtained...Ch. 16.6 - Prob. 33ECh. 16.6 - Prob. 34ECh. 16.6 - Find an equation of the tangent plane to the given...Ch. 16.6 - Prob. 36ECh. 16.6 - Find an equation of the tangent plane to the given...Ch. 16.6 - Prob. 38ECh. 16.6 - Prob. 39ECh. 16.6 - Prob. 40ECh. 16.6 - Prob. 41ECh. 16.6 - Find the area of the surface. 42. The part of the...Ch. 16.6 - Prob. 43ECh. 16.6 - Prob. 44ECh. 16.6 - Find the area of the surface. 45. The part of the...Ch. 16.6 - Find the area of the surface. 46. The part of the...Ch. 16.6 - Find the area of the surface. 47. The part of the...Ch. 16.6 - Find the area of the surface. 48.The helicoid (or...Ch. 16.6 - Find the area of the surface. 49. The surface with...Ch. 16.6 - Prob. 50ECh. 16.6 - Prob. 51ECh. 16.6 - Find the area of the surface correct to four...Ch. 16.6 - Find the area of the surface correct to four...Ch. 16.6 - Prob. 54ECh. 16.6 - Prob. 56ECh. 16.6 - Prob. 59ECh. 16.6 - Prob. 60ECh. 16.6 - Prob. 61ECh. 16.6 - The figure shows the surface created when the...Ch. 16.6 - Prob. 63ECh. 16.7 - LetSbe the surface of the box enclosed by the...Ch. 16.7 - Prob. 2ECh. 16.7 - Prob. 3ECh. 16.7 - Prob. 4ECh. 16.7 - Evaluate the surface integral. 5. s (x + y + z)...Ch. 16.7 - Evaluate the surface integral. 6. s xyz dS, Sis...Ch. 16.7 - Evaluate the surface integral. 7. s y dS,Sis the...Ch. 16.7 - Evaluate the surface integral. 8.s (x2+ y2)dS, Sis...Ch. 16.7 - Evaluate the surface integral. 9. s x2yz dS, Sis...Ch. 16.7 - Evaluate the surface integral. 10. s xz dS, S is...Ch. 16.7 - Evaluate the surface integral. 11. s x dS, S is...Ch. 16.7 - Evaluate the surface integral. 12. s y dS, S is...Ch. 16.7 - Evaluate the surface integral. 13. s z2dS, S is...Ch. 16.7 - Evaluate the surface integral. 14. s y2z2 dS, S is...Ch. 16.7 - Evaluate the surface integral. 15. s x dS, S is...Ch. 16.7 - Evaluate the surface integral. 16 s y2 dS, S is...Ch. 16.7 - Evaluate the surface integral. 17. s (x2z +...Ch. 16.7 - Evaluate the surface integral. 18. s (x + y + z)...Ch. 16.7 - Evaluate the surface integral. 19. s xz dS, S is...Ch. 16.7 - Evaluate the surface integral. 20. s (x2 + y2 +...Ch. 16.7 - Evaluate the surface integral s F dS for the...Ch. 16.7 - Evaluate the surface integral s F dS for the...Ch. 16.7 - Evaluate the surface integral s F dS for the...Ch. 16.7 - Evaluate the surface integral s F dS for the...Ch. 16.7 - Evaluate the surface integral s F dS for the...Ch. 16.7 - Evaluate the surface integral s F dS for the...Ch. 16.7 - Evaluate the surface integral s F dS for the...Ch. 16.7 - Find a formula for s F dS similar to Formula 10...Ch. 16.7 - Find a formula for s F dS similar to Formula 10...Ch. 16.7 - Find the center of mass of the hemisphere x2 + y2...Ch. 16.7 - Find the mass of a thin funnel in the shape of a...Ch. 16.7 - (a) Give an integral expression for the moment of...Ch. 16.7 - Let S be the part of the sphere x2 + y2 + z2 = 25...Ch. 16.7 - Prob. 43ECh. 16.7 - Prob. 44ECh. 16.7 - Prob. 45ECh. 16.7 - Prob. 46ECh. 16.7 - The temperature at the point (x, y, z) in a...Ch. 16.7 - Prob. 48ECh. 16.7 - Prob. 49ECh. 16.8 - 1. A hemisphere H and a portion P of a paraboloid...Ch. 16.8 - Use Stokes Theorem to evaluate s curl F dS. 2....Ch. 16.8 - Use Stokes Theorem to evaluate s curl F dS. 3....Ch. 16.8 - Prob. 4ECh. 16.8 - (x, y, z) = xyz i + xy j + x2yz k. S consists of...Ch. 16.8 - Use Stokes Theorem to evaluate s curl F dS. 6....Ch. 16.8 - Use Stokes Theorem to evaluate c F dr. In each...Ch. 16.8 - Use Stokes Theorem to evaluate c F dr. In each...Ch. 16.8 - Use Stokes Theorem to evaluate c F dr. In each...Ch. 16.8 - Prob. 10ECh. 16.8 - (a) Use Stokes Theorem to evaluate c F dr, where...Ch. 16.8 - Prob. 12ECh. 16.8 - Verify that Stokes Theorem is true for the given...Ch. 16.8 - Verify that Stokes Theorem is true for the given...Ch. 16.8 - Verify that Stokes Theorem is true for the given...Ch. 16.8 - A particle moves along line segments from the...Ch. 16.8 - Evaluate c (y + sin x) dx + (z2 + cos y) dy + x3...Ch. 16.8 - If S is a sphere and F satisfies the hypotheses of...Ch. 16.8 - Prob. 20ECh. 16.9 - Verify that the Divergence Theorem is true for the...Ch. 16.9 - Verify that the Divergence Theorem is true for the...Ch. 16.9 - Verify that the Divergence Theorem is true for the...Ch. 16.9 - Verify that the Divergence Theorem is true for the...Ch. 16.9 - Use the Divergence Theorem to calculate the...Ch. 16.9 - Use the Divergence Theorem to calculate the...Ch. 16.9 - Use the Divergence Theorem to calculate the...Ch. 16.9 - Use the Divergence Theorem to calculate the...Ch. 16.9 - Use the Divergence Theorem to calculate the...Ch. 16.9 - Prob. 10ECh. 16.9 - Prob. 11ECh. 16.9 - Prob. 12ECh. 16.9 - Prob. 13ECh. 16.9 - Use the Divergence Theorem to calculate the...Ch. 16.9 - Prob. 17ECh. 16.9 - Let F(x, y, z) = z tan-1(y2) i + z3 ln(x2 + 1) j +...Ch. 16.9 - A vector field F is shown. Use the interpretation...Ch. 16.9 - (a) Are the points P1 and P2 sources or sinks for...Ch. 16.9 - Prob. 23ECh. 16.9 - Use the Divergence Theorem to evaluate...Ch. 16.9 - Prob. 25ECh. 16.9 - Prob. 26ECh. 16.9 - Prob. 27ECh. 16.9 - Prob. 28ECh. 16.9 - Prob. 29ECh. 16.9 - Prove each identity, assuming that S and E satisfy...Ch. 16.9 - Suppose S and E satisfy the conditions of the...Ch. 16.9 - Prob. 32ECh. 16 - What is a vector field? Give three examples that...Ch. 16 - Prob. 2RCCCh. 16 - Prob. 3RCCCh. 16 - (a) Define the line integral of a vector field F...Ch. 16 - Prob. 5RCCCh. 16 - (a) What does it mean to say that C F dris...Ch. 16 - Prob. 7RCCCh. 16 - Prob. 8RCCCh. 16 - Prob. 9RCCCh. 16 - Prob. 10RCCCh. 16 - Prob. 11RCCCh. 16 - Prob. 12RCCCh. 16 - Prob. 13RCCCh. 16 - Prob. 14RCCCh. 16 - Prob. 15RCCCh. 16 - In what ways are the Fundamental Theorem for Line...Ch. 16 - Prob. 1RQCh. 16 - Prob. 2RQCh. 16 - Prob. 3RQCh. 16 - Prob. 4RQCh. 16 - Prob. 5RQCh. 16 - Prob. 6RQCh. 16 - Prob. 7RQCh. 16 - Determine whether the statement is true or false....Ch. 16 - Prob. 9RQCh. 16 - Prob. 10RQCh. 16 - Prob. 11RQCh. 16 - Determine whether the statement is true or false....Ch. 16 - Prob. 13RQCh. 16 - Prob. 1RECh. 16 - Evaluate the line integral. 2. C x ds, C is the...Ch. 16 - Prob. 3RECh. 16 - Evaluate the line integral. 4. C y dx + (x + y2)...Ch. 16 - Prob. 5RECh. 16 - Evaluate the line integral. 6. C xy dx + ey dy +...Ch. 16 - Evaluate the line integral. 7. C xy dx + y2 dy +...Ch. 16 - Prob. 8RECh. 16 - Prob. 9RECh. 16 - Prob. 10RECh. 16 - Prob. 11RECh. 16 - Prob. 12RECh. 16 - Prob. 13RECh. 16 - Show that F is a conservative and use this fact to...Ch. 16 - Verify that Greens Theorem is true for the line...Ch. 16 - Prob. 16RECh. 16 - Prob. 17RECh. 16 - Prob. 18RECh. 16 - Prob. 19RECh. 16 - If F and G are vector fields whose component...Ch. 16 - Prob. 21RECh. 16 - If f and g are twice differentiable functions,...Ch. 16 - If f is a harmonic function, that is, 2f = 0, show...Ch. 16 - Prob. 24RECh. 16 - Prob. 25RECh. 16 - Prob. 27RECh. 16 - Prob. 28RECh. 16 - Prob. 29RECh. 16 - Prob. 30RECh. 16 - Prob. 31RECh. 16 - Prob. 32RECh. 16 - Use Stokes Theorem to evaluate C F dr, where F(x,...Ch. 16 - Prob. 34RECh. 16 - Prob. 35RECh. 16 - Compute the outward flux of F(x, y, z) =...Ch. 16 - Let F(x, y, z) = (3x2 yz 3y) i + (x3z 3x) j +...Ch. 16 - Prob. 38RECh. 16 - Find S F n dS, where F(x, y, z) = x i + y j + z k...Ch. 16 - Prob. 40RECh. 16 - Prob. 41RECh. 16 - 1. Let S be a smooth parametric surface and let P...Ch. 16 - Find the positively oriented simple closed curve C...Ch. 16 - Let C be a simple closed piecewise-smooth space...Ch. 16 - Prove the following identity: (F G) = (F )G + (G...Ch. 16 - The figure depicts the sequence of events in each...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Given lim x-4 f (x) = 1,limx-49 (x) = 10, and lim→-4 h (x) = -7 use the limit properties to find lim→-4 1 [2h (x) — h(x) + 7 f(x)] : - h(x)+7f(x) 3 O DNEarrow_forward17. Suppose we know that the graph below is the graph of a solution to dy/dt = f(t). (a) How much of the slope field can you sketch from this information? [Hint: Note that the differential equation depends only on t.] (b) What can you say about the solu- tion with y(0) = 2? (For example, can you sketch the graph of this so- lution?) y(0) = 1 y ANarrow_forward(b) Find the (instantaneous) rate of change of y at x = 5. In the previous part, we found the average rate of change for several intervals of decreasing size starting at x = 5. The instantaneous rate of change of fat x = 5 is the limit of the average rate of change over the interval [x, x + h] as h approaches 0. This is given by the derivative in the following limit. lim h→0 - f(x + h) − f(x) h The first step to find this limit is to compute f(x + h). Recall that this means replacing the input variable x with the expression x + h in the rule defining f. f(x + h) = (x + h)² - 5(x+ h) = 2xh+h2_ x² + 2xh + h² 5✔ - 5 )x - 5h Step 4 - The second step for finding the derivative of fat x is to find the difference f(x + h) − f(x). - f(x + h) f(x) = = (x² x² + 2xh + h² - ])- = 2x + h² - 5h ])x-5h) - (x² - 5x) = ]) (2x + h - 5) Macbook Proarrow_forward
- Evaluate the integral using integration by parts. Sx² cos (9x) dxarrow_forwardLet f be defined as follows. y = f(x) = x² - 5x (a) Find the average rate of change of y with respect to x in the following intervals. from x = 4 to x = 5 from x = 4 to x = 4.5 from x = 4 to x = 4.1 (b) Find the (instantaneous) rate of change of y at x = 4. Need Help? Read It Master Itarrow_forwardVelocity of a Ball Thrown into the Air The position function of an object moving along a straight line is given by s = f(t). The average velocity of the object over the time interval [a, b] is the average rate of change of f over [a, b]; its (instantaneous) velocity at t = a is the rate of change of f at a. A ball is thrown straight up with an initial velocity of 128 ft/sec, so that its height (in feet) after t sec is given by s = f(t) = 128t - 16t². (a) What is the average velocity of the ball over the following time intervals? [3,4] [3, 3.5] [3, 3.1] ft/sec ft/sec ft/sec (b) What is the instantaneous velocity at time t = 3? ft/sec (c) What is the instantaneous velocity at time t = 7? ft/sec Is the ball rising or falling at this time? O rising falling (d) When will the ball hit the ground? t = sec Need Help? Read It Watch Itarrow_forward
- Find the slope of the tangent line to the graph of the function at the given point. f(x) = -4x + 5 at (-1, 9) m Determine an equation of the tangent line. y = Need Help? Read It Watch It SUBMIT ANSWERarrow_forwardFind the slope of the tangent line to the graph of the function at the given point. f(x) = 5x-4x² at (-1, -9) m Determine an equation of the tangent line. y = Need Help? Read It Master It SUBMIT ANSWERarrow_forwardFor what value of A and B the function f(x) will be continuous everywhere for the given definition?..arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Basic Differentiation Rules For Derivatives; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=IvLpN1G1Ncg;License: Standard YouTube License, CC-BY