
Concept explainers
Income and Percent Audited. The Transactional Records Access Clearinghouse at Syracuse University reported data showing the odds of an Internal Revenue Service audit. The following table shows the average adjusted gross income reported and the percent of the returns that were audited for 20 selected IRS districts.
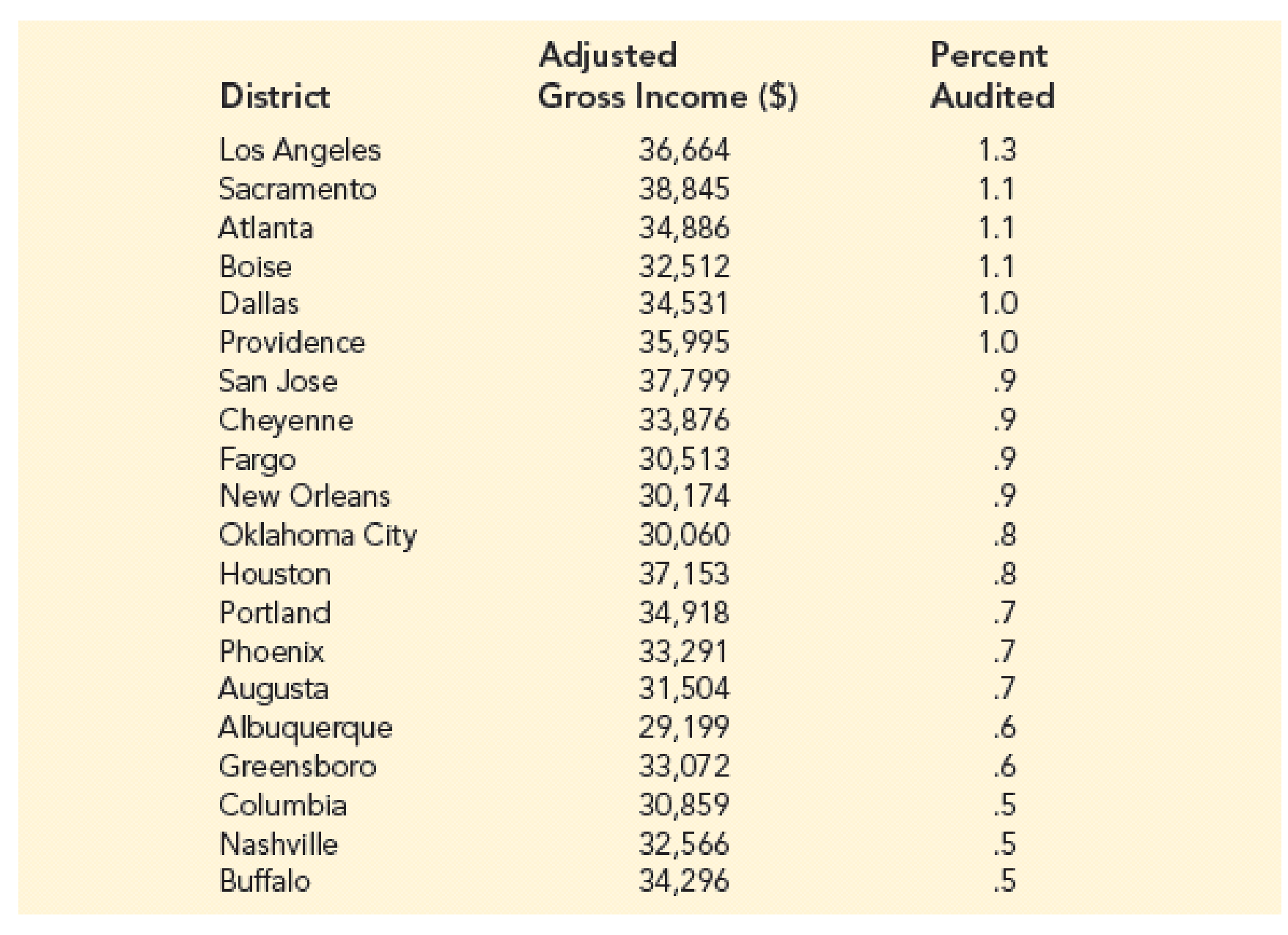
- a. Develop the estimated regression equation that could be used to predict the percent audited given the average adjusted gross income reported.
- b. At the .05 level of significance, determine whether the adjusted gross income and the percent audited are related.
- c. Did the estimated regression equation provide a good fit? Explain.
- d. Use the estimated regression equation developed in part (a) to calculate a 95% confidence interval for the expected percent audited for districts with an average adjusted gross income of $35,000.
a.
Find the estimated regression equation to predict the percent audited given the average adjusted gross income reported.
Answer to Problem 67SE
The estimated regression equation to predict the percent audited, given the average adjusted gross income reported is as follows:
Explanation of Solution
Calculation:
The data are related to the adjusted gross income ($) and percent audited for 20 selected IRS districts.
In the given problem, the percent audited is the dependent variable (y) and the adjusted gross income is the independent variable (x).
Regression:
Software procedure:
Step-by-step procedure to obtain the estimated regression equation using EXCEL:
- In Excel sheet, enter Adjusted Gross income and Percent Audited in different columns.
- In Data, select Data Analysis and choose Regression.
- In Input Y Range, select Percent Audited.
- In Input X Range, select Adjusted Gross income.
- Select Labels.
- Click OK.
Output obtained using EXCEL is given below:
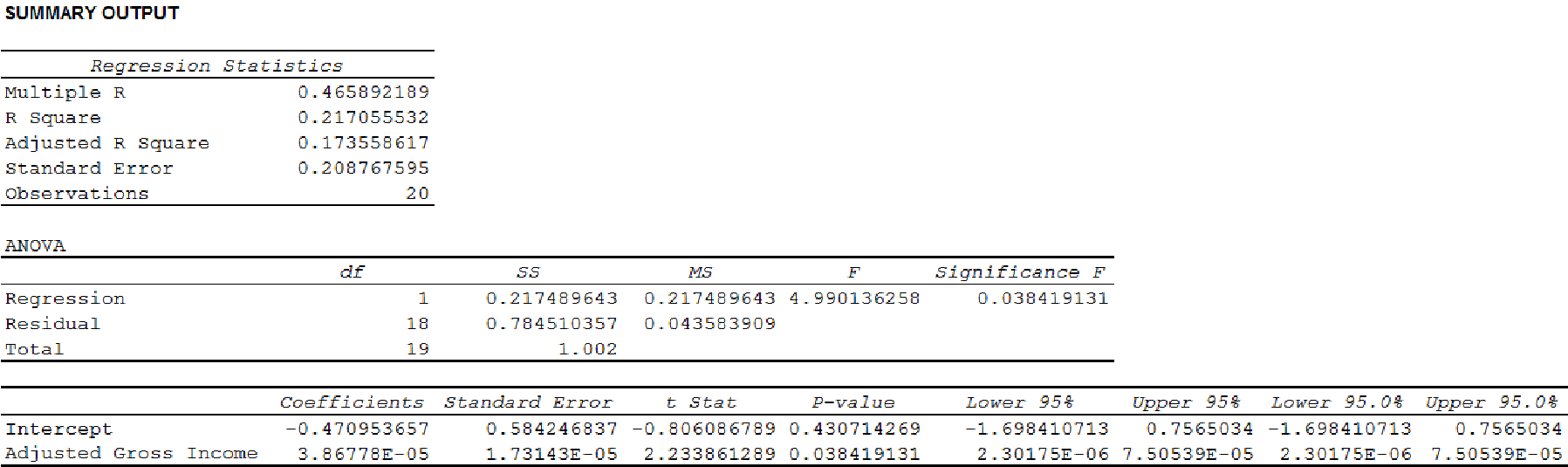
Thus, the estimated regression equation to predict the percent audited, given the average adjusted gross income reported is as follows:
b.
Use
Answer to Problem 67SE
There is a significant relationship between the adjusted gross income and the percent audited.
Explanation of Solution
Calculation:
State the test hypotheses.
Null hypothesis:
That is, there is no significant relationship between the adjusted gross income and the percent audited.
Alternative hypothesis:
That is, there is a significant relationship between the adjusted gross income and the percent audited.
From the output in Part (a), it is found that the F-test statistic is 4.99.
Level of significance:
The given level of significance is
p-value:
From Part (a) in the output, it is found that the p-value is 0.038.
Rejection rule:
If the
Conclusion:
Here, the p-value is less than the level of significance.
That is,
Thus, the decision is “reject the null hypothesis”.
Therefore, the data provide sufficient evidence to conclude that there is a significant relationship between the adjusted gross income and the percent audited.
Thus, the adjusted gross income and the percent audited are related.
c.
Explain whether the estimated regression equation provides a good fit to the data.
Answer to Problem 67SE
The estimated regression equation does not provide a good fit to the data.
Explanation of Solution
The coefficient of determination (
In the given output of Part (a),
Thus, the percentage of variation in the observed values of percent audited that is explained by the regression is 21.71%, which indicates that only 21.71% of the variability in percent audited is explained by the variability in the adjusted gross income using the linear regression model.
Thus, the estimated regression equation does not provide a good fit to the data.
d.
Find a 95% confidence interval for the expected percent audited for districts with an average adjusted gross income of $35,000.
Answer to Problem 67SE
The 95% confidence interval for the expected percent audited for districts with an average adjusted gross income of $35,000 is
Explanation of Solution
Calculation:
The estimate of standard deviation of
From Part (a), the estimated regression equation is as follows:
Also, the mean square error (MSE) is 0.0436.
According to the regression equation
Thus, the possible value of the dependent variable y when
The standard error of the estimate is obtained as follows:
Thus, the standard error of the estimate is 0.2088.
It is known that for a sample of size n, the mean of a random variable x can be obtained as follows:
Thus, the mean of the random variable x is obtained as follows:
The mean of the random variable x is
The value of
| 36,664 | 3,028 | 9,168,784 |
| 38,845 | 5,209 | 27,133,681 |
| 34,886 | 1,250 | 1,562,500 |
| 32,512 | –1,124 | 1,263,376 |
| 34,531 | 895 | 801,025 |
| 35,995 | 2,359 | 5,564,881 |
| 37,799 | 4,163 | 17,330,569 |
| 33,876 | 240 | 57,600 |
| 30,513 | –3,123 | 9,753,129 |
| 30,174 | –3,462 | 11,985,444 |
| 30,060 | –3,576 | 12,787,776 |
| 37,153 | 3,517 | 12,369,289 |
| 34,918 | 1,282 | 1,643,524 |
| 33,291 | –345 | 119,025 |
| 31,504 | –2,132 | 4,545,424 |
| 29,199 | –4,437 | 19,686,969 |
| 33,072 | –564 | 318,096 |
| 30,859 | –2,777 | 7,711,729 |
| 32,566 | –1,070 | 1,144,900 |
| 34,296 | 660 | 435,600 |
For the adjusted gross income of (35,000), the standard deviation of
Thus, the standard deviation of
The confidence interval for the expected value of y
Degrees of freedom:
For a sample of size n, the degrees of freedom is given as
In this given problem, for a sample size 20, the degrees of freedom is as follows:
Thus, the degrees of freedom is 18.
Level of significance:
The given level of significance is
For both tails distribution:
Form Table 2 of “t Distribution” in Appendix B, it is found that the value of t test statistic with the level of significance 0.025 and degrees of freedom 18 is
Therefore, the required confidence interval is obtained as follows:
Thus, the 95% confidence interval for the expected percent audited for districts with an average adjusted gross income of $35,000 is
Want to see more full solutions like this?
Chapter 14 Solutions
Essentials Of Statistics For Business & Economics
- At the same restaurant as in Question 19 with the same normal distribution, what's the chance of it taking no more than 15 minutes to get service?arrow_forwardClint, obviously not in college, sleeps an average of 8 hours per night with a standard deviation of 15 minutes. What's the chance of him sleeping between 7.5 and 8.5 hours on any given night? 0-(7-0) 200 91109s and doiw $20 (8-0) mol 8520 slang $199 galbrog seam side pide & D (newid se od poyesvig as PELEO PER AFTE editiw noudab temand van Czarrow_forwardTimes to complete a statistics exam have a normal distribution with a mean of 40 minutes and standard deviation of 6 minutes. Deshawn's time comes in at the 90th percentile. What percentage of the students are still working on their exams when Deshawn leaves?arrow_forward
- Suppose that the weights of cereal boxes have a normal distribution with a mean of 20 ounces and standard deviation of half an ounce. A box that has a standard score of o weighs how much? syed by ilog ni 21arrow_forwardBob scores 80 on both his math exam (which has a mean of 70 and standard deviation of 10) and his English exam (which has a mean of 85 and standard deviation of 5). Find and interpret Bob's Z-scores on both exams to let him know which exam (if either) he did bet- ter on. Don't, however, let his parents know; let them think he's just as good at both subjects. algas 70) sering digarrow_forwardSue's math class exam has a mean of 70 with a standard deviation of 5. Her standard score is-2. What's her original exam score?arrow_forward
- Clint sleeps an average of 8 hours per night with a standard deviation of 15 minutes. What's the chance he will sleep less than 7.5 hours tonight? nut bow visarrow_forwardSuppose that your score on an exam is directly at the mean. What's your standard score?arrow_forwardOne state's annual rainfall has a normal dis- tribution with a mean of 100 inches and standard deviation of 25 inches. Suppose that corn grows best when the annual rainfall is between 100 and 150 inches. What's the chance of achieving this amount of rainfall? wved now of sociarrow_forward
 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning




