
Concept explainers
Review. For the arrangement shown in Figure P14.60, the inclined plane and the small pulley are frictionless; the string supports the object of mass M at the bottom of the plane; and the string has mass m. The system is in equilibrium, and the vertical part of the string has a length h. We wish to study standing waves set up in the vertical section of the string. (a) What analysis model describes the object of mass M? (b) What analysis model describes the waves on the vertical part of the string? (c) Find the tension in the string. (d) Model the shape of the string as one leg and the hypotenuse of a right triangle. Find the whole length of the string. (e) Find the mass per unit length of the string. (f) Find the speed of waves on the string. (g) Find the lowest frequency for a standing wave on the vertical section of the string. (h) Evaluate this result for M = 1.50 kg, m = 0.750 g, h = 0.500 m, and θ = 30.0°. (i) Find the numerical value for the lowest frequency for a standing wave on the sloped section of the string.
Figure P14.60
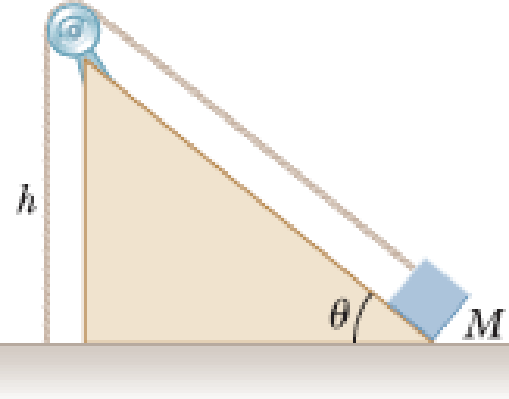
(a)
The analysis model
Answer to Problem 60P
The object is described using constant acceleration model.
Explanation of Solution
The mass of the object supported by the string is
The object of mass
Conclusion:
Therefore, the object of mass
(b)
The analysis model
Answer to Problem 60P
The waves on the vertical part of the string can be described using the waves under boundary conditions model.
Explanation of Solution
The mass of the object supported by the string is
The vertical portion of the string is fixed at both the ends hence the boundary conditions stand for the waves. Write the general equation for the wavelength on the string.
Here,
Conclusion:
Therefore, the waves on the vertical part of the string can be described using the waves under boundary conditions model.
(c)
The tension on the string
Answer to Problem 60P
The tension on the string is
Explanation of Solution
Figure.1 shows the arrangement of the mass-pulley system.
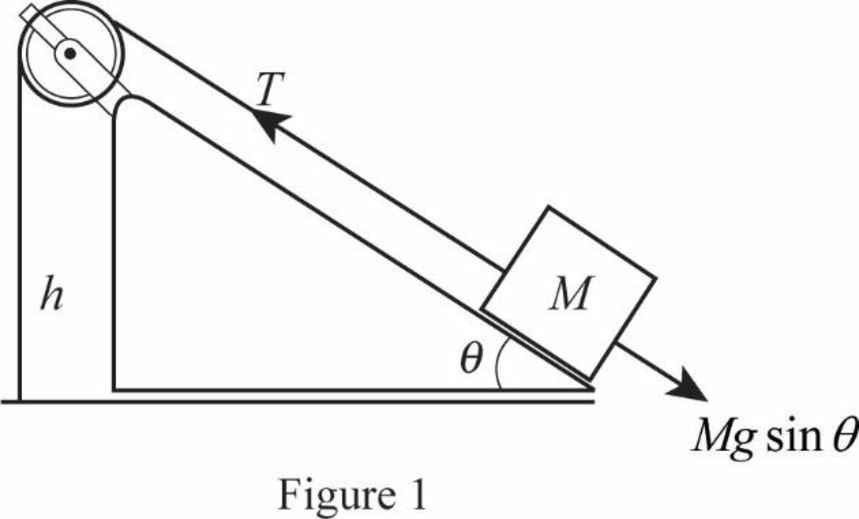
Write the equation for the net force acting on the mass.
Here,
Conclusion:
Re-write the equation (I) such that the net force is zero.
Therefore, the tension on the string is
(d)
The length of the string
Answer to Problem 60P
The length of the string is
Explanation of Solution
Write the equation for the sine of the angle of inclination.
Rewrite the equation (I) to find the equation for the
Write the equation for the total length of the string.
Conclusion:
Substitute equation (II) in equation (III).
Therefore, the length of the strings is
(e)
The mass per unit length of the string
Answer to Problem 60P
The mass per unit length of the string is
Explanation of Solution
Write the equation for the mass per unit length of the string.
Here,
Conclusion:
Substitute equation (V) in equation (VI).
Therefore, the mass per unit length of the string is
(f)
The speed of waves on the string
Answer to Problem 60P
The speed of waves on the string is
Explanation of Solution
Write the equation for the speed of the waves on the string.
Here,
Conclusion:
Substitute equation (II) and equation (VIII) in equation (IX).
Therefore, the speed of the waves on the string is
(g)
The lowest frequency for a standing wave
Answer to Problem 60P
The lowest frequency for the standing waves is
Explanation of Solution
The length
Write the equation for the frequency of the standing wave.
Here,
Conclusion:
Substitute equation (X) and equation (XI) in equation (XII).
Therefore, the lowest possible frequency of the standing waves is
(h)
The lowest frequency for a set of given values
Answer to Problem 60P
The lowest frequency for the standing waves is
Explanation of Solution
Write the equation for the lowest frequency of the standing wave from equation (XIII).
Conclusion:
Substitute
Therefore, the lowest frequency of the standing waves is
(i)
The lowest frequency on the sloped section
Answer to Problem 60P
The lowest frequency for the standing waves on the sloped section is
Explanation of Solution
The wavelength of the fundamental mode of vibration is twice the length of the sloped section.
Here,
Substitute equation (X) and equation (XIV) in equation (XII).
Substitute
Therefore, the lowest frequency of the standing waves is
Want to see more full solutions like this?
Chapter 14 Solutions
Principles of Physics: A Calculus-Based Text, Hybrid (with Enhanced WebAssign Printed Access Card)
- please answer this asap!!!!arrow_forwardRT = 4.7E-30 18V IT = 2.3E-3A+ 12 38Ω ли 56Ω ли r5 27Ω ли r3 28Ω r4 > 75Ω r6 600 0.343V 75.8A Now figure out how much current in going through the r4 resistor. |4 = unit And then use that current to find the voltage drop across the r resistor. V4 = unitarrow_forward7 Find the volume inside the cone z² = x²+y², above the (x, y) plane, and between the spheres x²+y²+z² = 1 and x² + y²+z² = 4. Hint: use spherical polar coordinates.arrow_forward
- ганм Two long, straight wires are oriented perpendicular to the page, as shown in the figure(Figure 1). The current in one wire is I₁ = 3.0 A, pointing into the page, and the current in the other wire is 12 4.0 A, pointing out of the page. = Find the magnitude and direction of the net magnetic field at point P. Express your answer using two significant figures. VO ΜΕ ΑΣΦ ? Figure P 5.0 cm 5.0 cm ₁ = 3.0 A 12 = 4.0 A B: μΤ You have already submitted this answer. Enter a new answer. No credit lost. Try again. Submit Previous Answers Request Answer 1 of 1 Part B X Express your answer using two significant figures. ΜΕ ΑΣΦ 0 = 0 ? below the dashed line to the right P You have already submitted this answer. Enter a new answer. No credit lost. Try again.arrow_forwardAn infinitely long conducting cylindrical rod with a positive charge λ per unit length is surrounded by a conducting cylindrical shell (which is also infinitely long) with a charge per unit length of −2λ and radius r1, as shown in the figure. What is σinner, the surface charge density (charge per unit area) on the inner surface of the conducting shell? What is σouter, the surface charge density on the outside of the conducting shell? (Recall from the problem statement that the conducting shell has a total charge per unit length given by −2λ.)arrow_forwardA small conducting spherical shell with inner radius aa and outer radius b is concentric with a larger conducting spherical shell with inner radius c and outer radius d (Figure 1). The inner shell has total charge +2q, and the outer shell has charge −2q. What's the total charge on the inner surface of the small shell? What's the total charge on the outer surface of the small shell? What's the total charge on the inner surface of the large shell? What's the total charge on the outer surface of the large shell?arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





