
Concept explainers
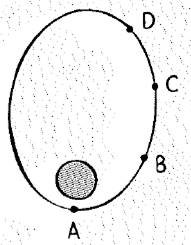
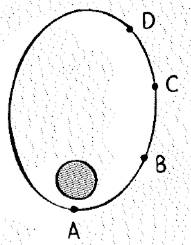
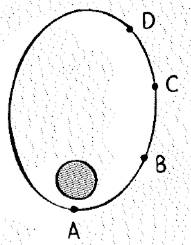
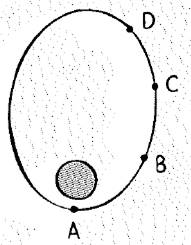
The positions of a satellite in elliptical orbit are indicated.
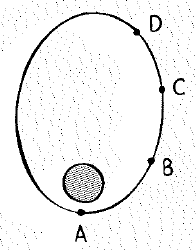
Rank these quantities from greatest to least.
a. gravitational force
b. speed
c. momentum
d. KE
e. PE
f. total energy
g. acceleration
(a)
To rank: Gravitational force at different points from greatest to least.
Answer to Problem 22A
Gravitational force, from greatest to least is, A>B>C>D
Explanation of Solution
Given:
The position of satellite in elliptical orbit are shown below.
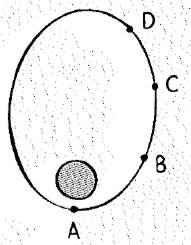
Formula used:
Gravitational force of attraction between two massive bodies of mass is
Where,
G = Gravitational constant =
r = distance between to body.
Calculation:
From above equation, gravitational force is inversely proportional to the square of the distance between two body.
From figure, distance of the satellite from the earth is given at different position is,
(Position A) < (Position B) < (Position C) < (Position D)
Since less be the distance, large will be the force of gravity
Conclusion:
Therefore, gravitational force, from greatest to least is, A > B > C > D.
(b)
To rank: Speed of satellite, from greatest to least.
Answer to Problem 22A
Speed of satellite, from greatest to least is, A > B > C > D
Explanation of Solution
Given:
The position of satellite in elliptical orbit are shown below.
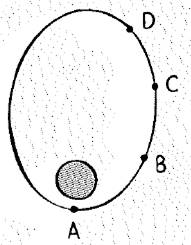
Formula:
The speed of the satellite in elliptical orbit is,
Where,
G = Gravitational constant = 6.67×10-11 N.m2/kg2
M = Mass of the satellite
r = The distance of the satellite from the earth
a = Semi-major axis
Calculation:
From given formula,
From the given formula, distance of the satellite increases as the satellite near to the earth. So, the speed is increases from position A to position D
Conclusion:
Therefore, speed of satellite, from greatest to least is, A>B>C>D
(c)
To rank: Momentum of satellite, from greatest to least.
Answer to Problem 22A
Momentum of satellite, from greatest to least is, A>B>C>D
Explanation of Solution
Given:
The position of satellite in elliptical orbit are shown below.
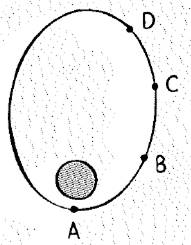
Formula:
The momentum of the satellite in elliptical orbit is,
Where,
M = Mass of the satellite
v = Speed of the satellite.
P = momentum of the satellite.
Calculation:
Since from the given formula, momentum of the satellite is directly proportional to the velocity of the satellite.
As from the given formula, speed of the satellite increases as the satellite near to the earth. So, the momentum is increases from D position A
Conclusion:
Therefore, momentum of satellite, from greatest to least is, A>B>C>D
(d)
To rank: Kinetic energy of satellite, from greatest to least.
Answer to Problem 22A
Kinetic energy of satellite, from greatest to least is, A>B>C>D
Explanation of Solution
Given:
The position of satellite in elliptical orbit are shown below.
Formula:
The kinetic energy of the satellite in elliptical orbit is,
Where,
KE = kinetic energy of satellite
M = Mass of the satellite
v = Speed of the satellite.
Calculation:
Since from the given formula, kinetic energy of the satellite is directly proportional to the velocity of the satellite.
From the given formula, speed of the satellite increases as the satellite near to the earth. So, the kinetic energy is increases from position D to position A
Conclusion:
Therefore, momentum of satellite, from greatest to least is, A>B>C>D
(e)
To rank: Potential energy of satellite, from greatest to least.
Answer to Problem 22A
Potential energy of satellite, from greatest to least is, D>C>B>A
Explanation of Solution
Given:
The position of satellite in elliptical orbit are shown below.
Formula:
The potential energy of the satellite in elliptical orbit is,
Where,
G = Gravitational constant= 6.67×10-11 N.m2/kg2
M = Mass of the satellite
m = Mass of the earth
r = The distance of the satellite from the earth
R = Radius of earth
Calculation:
From given formula,
From the given formula, distance of the satellite increases as the satellite near to the earth. Since the potential is negative. So, the potential energy is decreases from position A to position D
Conclusion:
Therefore, potential energy of satellite, from greatest to least is, D > C > B > A
(f)
To rank: Total energy ( E ) of satellite, from greatest to least.
Answer to Problem 22A
Total energy ( E ) of satellite, from greatest to least is, A = B = C = D
Explanation of Solution
Given:
The position of satellite in elliptical orbit are shown below.
Formula:
The total energy ( E ) of the satellite in elliptical orbit is,
Where,
PE = Potential energy of the satellite
KE = Kinetic energy of the satellite
Calculation:
According to conservation of energy, the total energy is constant in any process. When satellite move around the earth in elliptical orbit, depending on its position, velocity of the satellite get changed. When particle is move near to the earth due to gravitational pull, speed of the satellite increases. That increase in the speed of the satellite responsible for the higher kinetic energy. Similarly, as particle move away from the earth, its potential energy increases. Since the total energy of the satellite during its journey is remain constant.
Conclusion:
Therefore, total energy ( E ) of satellite, from greatest to least is, A = B = C = D
(g)
To rank: Acceleration of satellite, from greatest to least.
Answer to Problem 22A
Acceleration of satellite, from greatest to least is, A > B > C > D
Explanation of Solution
Given:
The position of satellite in elliptical orbit are shown below.
Formula:
The acceleration of the satellite in elliptical orbit is,
F= ma
Where,
F = Force acting on the satellite
m = mass of the satellite
a =Acceleration of the satellite
Calculation:
It is known from the part (a), as the distance between the satellite and Earth decreases gravitational force acting on the satellite increases. Since the acceleration of the satellite is directly proportional to the gravitational force on the satellite. Therefore, as distance between earth and satellite decreases, acceleration of the satellite increases.
Conclusion:
Therefore, acceleration of satellite, from greatest to least is, A > B > C > D
Chapter 14 Solutions
Conceptual Physics C2009 Guided Reading & Study Workbook Se
Additional Science Textbook Solutions
Introductory Chemistry (6th Edition)
Campbell Essential Biology with Physiology (5th Edition)
Chemistry: Structure and Properties (2nd Edition)
Organic Chemistry (8th Edition)
Microbiology: An Introduction
Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)
- How do I plot the force F in Matlba (of gravity pulling on the masses) versus spring displacement, and fit the data with a linear function to find the value for the spring constant. To get a linear fit, use polynomial order 1. Report the value of 'k' from the fit. What code is used?arrow_forwardOk im confused on this portion of the questions being asked. the first snip is the solution you gave which is correct. BUt now it is asking for this and im confused. The magnitude of the force F_11 is __________LB. The direction of the force F_11 is __________LB.arrow_forwardNo chatgpt pls will upvotearrow_forward
- The spring in the figure has a spring constant of 1300 N/m. It is compressed 17.0 cm, then launches a 200 g block. The horizontal surface is frictionless, but the block’s coefficient of kinetic friction on the incline is 0.200. What distance d does the block sail through the air?arrow_forwardSolve and answer the problem correctly and be sure to check your work. Thank you!!arrow_forwardSolve and answer the problem correctly and be sure to check your work. Thank you!!arrow_forward
- A 10-m-long glider with a mass of 680 kg (including the passengers) is gliding horizontally through the air at 28 m/s when a 60 kg skydiver drops out by releasing his grip on the glider. What is the glider's speed just after the skydiver lets go?arrow_forwardPROBLEM 2 A cube of mass m is placed in a rotating funnel. (The funnel is rotating around the vertical axis shown in the diagram.) There is no friction between the cube and the funnel but the funnel is rotating at just the right speed needed to keep the cube rotating with the funnel. The cube travels in a circular path of radius r, and the angle between the vertical and the wall of the funnel is 0. Express your answers to parts (b) and (c) in terms of m, r, g, and/or 0. (a) Sketch a free-body diagram for the cube. Show all the forces acting on it, and show the appropriate coordinate system to use for this problem. (b) What is the normal force acting on the cube? FN=mg58 (c) What is the speed v of the cube? (d) If the speed of the cube is different from what you determined in part (c), a force of friction is necessary to keep the cube from slipping in the funnel. If the funnel is rotating slower than it was above, draw a new free-body diagram for the cube to show which way friction…arrow_forwardCircular turns of radius r in a race track are often banked at an angle θ to allow the cars to achieve higher speeds around the turns. Assume friction is not present. Write an expression for the tan(θ) of a car going around the banked turn in terms of the car's speed v, the radius of the turn r, and g so that the car will not move up or down the incline of the turn. tan(θ) =arrow_forward
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON





