A Problem Solving Approach to Mathematics for Elementary School Teachers (12th Edition)
12th Edition
ISBN: 9780321987297
Author: Rick Billstein, Shlomo Libeskind, Johnny Lott
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 1.2A, Problem 1A
ASSESSEMENT
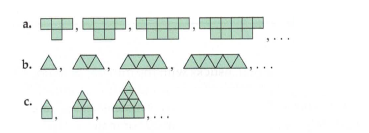
For each of the following sequences of figures, determine a possible pattern and draw the next figure according to that pattern:
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
9 AB is parallel to plane m and perpendicular to plane r. CD lies
in r. Which of the following must be true?
arim
br m
6 CD L m
d AB || CD
e AB and CD are skew.
a. A company is offering a job with a
salary of $35,000 for the first year and a
3% raise each year after that. If the 3%
raise continues every year, find the
amount of money you would earn in a
40-year career.
(6) Prove that the image of a polygon in R², under an isometry, is congruent to the
original polygon.
Chapter 1 Solutions
A Problem Solving Approach to Mathematics for Elementary School Teachers (12th Edition)
Ch. 1.1 - Mathematical Connections 1-2 a. If a fixed number...Ch. 1.1 - Mathematical Connections 1-2 A student says she...Ch. 1.1 - Mathematical Connections 1-2 Abby and Dan are...Ch. 1.1 - Consider a game where you have two distict piles...Ch. 1.1 - Mathematical Connections 1-2 A mathematician named...Ch. 1.1 - Mathematical Connections 1-2 The figure below...Ch. 1.1 - Mathematical Connections 1-1 The distance around...Ch. 1.1 - Work in pairs on the following versions of a game...Ch. 1.1 - John asks why the last step of Polyas four-step...Ch. 1.1 - Mathematical Connections 1-2 Connecting...
Ch. 1.1 - Mathematical Connections 1-2 Connecting...Ch. 1.1 - National Assessment of Educational Progress NAEP...Ch. 1.1 - A pattern of dots is shown above. How many dots...Ch. 1.1 - Susie said, I have 83c but fewer than 10 coins....Ch. 1.1 - An Introduction to Problem Solving Sam folds a...Ch. 1.1A - Use the approach in Gausss Problem to find the...Ch. 1.1A - Use the ideas in drawings a and b to find the...Ch. 1.1A - Find the sum 36+37+38+39+...+146+147.Ch. 1.1A - Assessment 1-1A Cookies are sold singly or in...Ch. 1.1A - Assessment 1-1A In a big red box, there are 7...Ch. 1.1A - Assessment 1-1A How many triangles are in the...Ch. 1.1A - Without computing each sum of the arithmetic...Ch. 1.1A - Assessment 1-1A Alababa, Bubba, Cory, and Dandy...Ch. 1.1A - Assessment 1-1A How many ways can you make change...Ch. 1.1A - Assessment 1-1A The following is a magic square...Ch. 1.1A - Assessment 1-1A Debbie and Amy began reading a...Ch. 1.1A - Assessment 1-1A The 14 digits of a credit card are...Ch. 1.1A - Assessment 1-1A Three closed boxes A, B, and C of...Ch. 1.1A - Assessment 1-1A An electrician charges 50 per hour...Ch. 1.1A - Assessment 1-1A Kathy stood on the middle rung of...Ch. 1.1B - Use the approach in Gausss Problem to find the...Ch. 1.1B - Use the diagram below to explain how to find the...Ch. 1.1B - Find the sum of 58+59+60+61+...+203.Ch. 1.1B - Eve Merriam " titled her childrens book...Ch. 1.1B - Prob. 5ACh. 1.1B - How many squares are in the following figure?Ch. 1.1B - If P=1+3+5+7+...+99 and Q=5+7+9...+101 are sums of...Ch. 1.1B - The sign says that you are leaving Missoula, Butte...Ch. 1.1B - Marc goes to the store with exactly 1.00 in...Ch. 1.1B - Find a 3-by-3 magic square using the numbers 3, 5,...Ch. 1.1B - Eight marbles look alike, but one is slightly...Ch. 1.1B - Recall the song "TheTwelveDaysofChristmas": On the...Ch. 1.1B - a. Suppose you have quarters, dimes and pennies...Ch. 1.1B - Suppose you buy lunch for the math club. You have...Ch. 1.1B - One winter night the temperature fell 15 degrees...Ch. 1.2 - a. If a fixed number is added to each term of an...Ch. 1.2 - A student says she read that Thomas Robert Malthus...Ch. 1.2 - MATHEMATICAL CONNECTIONS Abby to take place in 5...Ch. 1.2 - MATHEMATICAL CONNECTIONS The arithmetic average of...Ch. 1.2 - Prob. 5MCCh. 1.2 - Prob. 6MCCh. 1.2 - Prob. 7MCCh. 1.2 - Prob. 8MCCh. 1.2 - Prob. 9MCCh. 1.2 - Prob. 10MCCh. 1.2 - The following pattern is called Pascals triangle....Ch. 1.2 - Prob. 12MCCh. 1.2 - MATHEMATICAL CONNECTIONS Joey said that 4, 24, 44,...Ch. 1.2 - Mathematical Connections A1 and Betty were asked...Ch. 1.2 - MATHEMATICAL CONNECTIONS A student claims the...Ch. 1.2 - Prob. 16MCCh. 1.2 - Mathematical connections 12 In a baseball league...Ch. 1.2 - Mathematical connections 12 How many ways can you...Ch. 1.2 - Mathematical connections 12 Tents hold...Ch. 1.2 - Prob. 1NAEPCh. 1.2 - NATIONAL ASSESSMENT OF EDUCATIONAL PROGRESS NAEP...Ch. 1.2 - A car can seat c adults. A van can seat 4 more...Ch. 1.2 - Prob. 4NAEPCh. 1.2A - ASSESSEMENT For each of the following sequences of...Ch. 1.2A - ASSESSMENT Each of the following sequences is...Ch. 1.2A - Assessment Find the 100th term and the nth term...Ch. 1.2A - ASSESSMENT Use a traditional clock face to...Ch. 1.2A - ASSESSMENT The pattern 1,8,27,64,125,... is a...Ch. 1.2A - ASSESSMENT The first windmill has 5 matchstick...Ch. 1.2A - ASSESSMENT In the following sequence, the figures...Ch. 1.2A - ASSESSMENT The school population for a certain...Ch. 1.2A - Joes annual income has been increasing each year...Ch. 1.2A - The first difference of a sequence is the...Ch. 1.2A - List the next three terms to continue a pattern in...Ch. 1.2A - Assessment How many terms are there in each of the...Ch. 1.2A - ASSESSMENT Find the first five terms in sequences...Ch. 1.2A - Find a counterexample for each of the following:...Ch. 1.2A - Assume that the following patterns are built of...Ch. 1.2A - ASSESSMENT Consider the sequences given in the...Ch. 1.2A - ASSESSMENT A sheet of paper is cut into 5...Ch. 1.2A - Assessment Each of the following sequences is...Ch. 1.2A - Assessment A Fibonacci-type sequence is a sequence...Ch. 1.2A - Prob. 20ACh. 1.2A - ASSESSMENT A new pair of tennis shoes cost 80. If...Ch. 1.2B - ASSESSEMENT For each of the following sequences of...Ch. 1.2B - Assessment Each of the following sequences is...Ch. 1.2B - Find the 100th term and the nth term for each of...Ch. 1.2B - ASSESSMENT Use a traditional clock face to...Ch. 1.2B - Assessment Observe the following pattern:...Ch. 1.2B - In the following pattern, one hexagon takes 6...Ch. 1.2B - ASSESSMENT Each successive figure below is made of...Ch. 1.2B - ASSESSMENT A tank contains 15,360L of water. At...Ch. 1.2B - ASSESSMENT The Washington Middle School time is an...Ch. 1.2B - The first difference of a sequence is the...Ch. 1.2B - Prob. 11ACh. 1.2B - ASSESSMENT How many terms are there in a following...Ch. 1.2B - ASSESSMENT Find the first five terms in sequences...Ch. 1.2B - ASSESSMENT Find a counterexample for each of the...Ch. 1.2B - ASSESSMENT Assume the following pattern with terms...Ch. 1.2B - ASSESSMENT Consider the sequences given in the...Ch. 1.2B - Female bees are born fertilized eggs, and male...Ch. 1.2B - Assessment Each of the following sequences is...Ch. 1.2B - Prob. 19ACh. 1.2B - Starting with 1 and 1 as the first two terms of...Ch. 1.CR - CHAPTER 1 REVIEW If today is Sunday, July 4, and...Ch. 1.CR - CHAPTER 1 REVIEW Jackie spent 40 on two items. If...Ch. 1.CR - A nursery rhyme states: A diller, a dollar, a ten...Ch. 1.CR - CHAPTER 1 REVIEW List three more terms that...Ch. 1.CR - Find a possible nth term in each of the following:...Ch. 1.CR - Prob. 6CRCh. 1.CR - CHAPTER 1 REVIEW Find the following sums: a....Ch. 1.CR - Prob. 8CRCh. 1.CR - Prob. 9CRCh. 1.CR - Prob. 10CRCh. 1.CR - CHAPTER 1 REVIEW Solve the following equations: a....Ch. 1.CR - CHAPTER 1 REVIEW If fence posts are to be placed...Ch. 1.CR - If a complete rotation of a car tire moves car...Ch. 1.CR - CHAPTER 1 REVIEW The members of Mrs. Grants class...Ch. 1.CR - CHAPTER 1 REVIEW A carpenter has three large...Ch. 1.CR - Use differences to find the next term in the...Ch. 1.CR - CHAPTER 1 REVIEW An ant farm can hold 100,000...Ch. 1.CR - CHAPTER 1 REVIEW Tomas team entered a mathematics...Ch. 1.CR - CHAPTER 1 REVIEW Three pieces of wood are needed...Ch. 1.CR - CHAPTER 1 REVIEW How many four-digit numbers have...Ch. 1.CR - If n and m are natural numbers and nm, how many...Ch. 1.CR - Prob. 22CRCh. 1.CR - Prob. 23CRCh. 1.CR - Prob. 24CRCh. 1.CR - a. If every second term in an arithmetic sequence...Ch. 1.CR - Prob. 26CRCh. 1.CR - CHAPTER 1 REVIEW Each of the following is a...Ch. 1 - NOW TRY THIS Explain whether the approach in...Ch. 1 - NOW TRY THIS Find the sum of consecutive natural...Ch. 1 - NOW TRY THIS Each of 16 people in a round-robin...Ch. 1 - NOW TRY THIS An elevator stopped at the middle...Ch. 1 - Prob. 5NTCh. 1 - NOW TRY THIS A prime number is a natural number...Ch. 1 - In Figure 13, we want to know how many different...Ch. 1 - Prob. 8NTCh. 1 - NOW TRY THIS Consider the rectangular numbers in...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- The function f(x) is represented by the equation, f(x) = x³ + 8x² + x − 42. Part A: Does f(x) have zeros located at -7, 2, -3? Explain without using technology and show all work. Part B: Describe the end behavior of f(x) without using technology.arrow_forwardHow does the graph of f(x) = (x − 9)4 – 3 compare to the parent function g(x) = x²?arrow_forwardFind the x-intercepts and the y-intercept of the graph of f(x) = (x − 5)(x − 2)(x − 1) without using technology. Show all work.arrow_forward
- In a volatile housing market, the overall value of a home can be modeled by V(x) = 415x² - 4600x + 200000, where V represents the value of the home and x represents each year after 2020. Part A: Find the vertex of V(x). Show all work. Part B: Interpret what the vertex means in terms of the value of the home.arrow_forwardShow all work to solve 3x² + 5x - 2 = 0.arrow_forwardTwo functions are given below: f(x) and h(x). State the axis of symmetry for each function and explain how to find it. f(x) h(x) 21 5 4+ 3 f(x) = −2(x − 4)² +2 + -5 -4-3-2-1 1 2 3 4 5 -1 -2 -3 5arrow_forward
- The functions f(x) = (x + 1)² - 2 and g(x) = (x-2)² + 1 have been rewritten using the completing-the-square method. Apply your knowledge of functions in vertex form to determine if the vertex for each function is a minimum or a maximum and explain your reasoning.arrow_forwardTotal marks 15 3. (i) Let FRN Rm be a mapping and x = RN is a given point. Which of the following statements are true? Construct counterex- amples for any that are false. (a) If F is continuous at x then F is differentiable at x. (b) If F is differentiable at x then F is continuous at x. If F is differentiable at x then F has all 1st order partial (c) derivatives at x. (d) If all 1st order partial derivatives of F exist and are con- tinuous on RN then F is differentiable at x. [5 Marks] (ii) Let mappings F= (F1, F2) R³ → R² and G=(G1, G2) R² → R² : be defined by F₁ (x1, x2, x3) = x1 + x², G1(1, 2) = 31, F2(x1, x2, x3) = x² + x3, G2(1, 2)=sin(1+ y2). By using the chain rule, calculate the Jacobian matrix of the mapping GoF R3 R², i.e., JGoF(x1, x2, x3). What is JGOF(0, 0, 0)? (iii) [7 Marks] Give reasons why the mapping Go F is differentiable at (0, 0, 0) R³ and determine the derivative matrix D(GF)(0, 0, 0). [3 Marks]arrow_forward5. (i) Let f R2 R be defined by f(x1, x2) = x² - 4x1x2 + 2x3. Find all local minima of f on R². (ii) [10 Marks] Give an example of a function f: R2 R which is not bounded above and has exactly one critical point, which is a minimum. Justify briefly Total marks 15 your answer. [5 Marks]arrow_forward
- Total marks 15 4. : Let f R2 R be defined by f(x1, x2) = 2x²- 8x1x2+4x+2. Find all local minima of f on R². [10 Marks] (ii) Give an example of a function f R2 R which is neither bounded below nor bounded above, and has no critical point. Justify briefly your answer. [5 Marks]arrow_forward4. Let F RNR be a mapping. (i) x ЄRN ? (ii) : What does it mean to say that F is differentiable at a point [1 Mark] In Theorem 5.4 in the Lecture Notes we proved that if F is differentiable at a point x E RN then F is continuous at x. Proof. Let (n) CRN be a sequence such that xn → x ЄERN as n → ∞. We want to show that F(xn) F(x), which means F is continuous at x. Denote hnxn - x, so that ||hn|| 0. Thus we find ||F(xn) − F(x)|| = ||F(x + hn) − F(x)|| * ||DF (x)hn + R(hn) || (**) ||DF(x)hn||+||R(hn)||| → 0, because the linear mapping DF(x) is continuous and for all large nЄ N, (***) ||R(hn) || ||R(hn) || ≤ → 0. ||hn|| (a) Explain in details why ||hn|| → 0. [3 Marks] (b) Explain the steps labelled (*), (**), (***). [6 Marks]arrow_forward4. In Theorem 5.4 in the Lecture Notes we proved that if F: RN → Rm is differentiable at x = RN then F is continuous at x. Proof. Let (xn) CRN be a sequence such that x → x Є RN as n → ∞. We want F(x), which means F is continuous at x. to show that F(xn) Denote hn xnx, so that ||hn||| 0. Thus we find ||F (xn) − F(x) || (*) ||F(x + hn) − F(x)|| = ||DF(x)hn + R(hn)|| (**) ||DF(x)hn|| + ||R(hn) || → 0, because the linear mapping DF(x) is continuous and for all large n = N, |||R(hn) || ≤ (***) ||R(hn)|| ||hn|| → 0. Explain the steps labelled (*), (**), (***) [6 Marks] (ii) Give an example of a function F: RR such that F is contin- Total marks 10 uous at x=0 but F is not differentiable at at x = 0. [4 Marks]arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
what is Research Design, Research Design Types, and Research Design Methods; Author: Educational Hub;https://www.youtube.com/watch?v=LpmGSioXxdo;License: Standard YouTube License, CC-BY