Chapter 12.3, Problem 19PPS

a.

To determine

To identify: the sample and the population for each situation.

Then describe a statistic and a parameter.

Answer to Problem 19PPS

The pennies chosen by Theo, Lydia and Peter each represents a sample and the total pennies in the jar, that is, $30$ pennies represents the population.

The mean year of the pennies in the sample is a statistic and the mean year of the pennies in the population is the parameter.

Explanation of Solution

Given information :

There are $30$ pennies in Mr. Day’s jar. Theo looks at $5$ pennies from the jar and replaces them. Lydia looks at $10$ pennies and replaces them, and Peter looks at $20$ pennies and replaces them.

The year of pennies in jar:

  

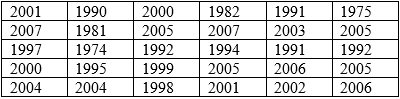
As per problem,

A sample is some portion of a larger group called population.

As per the given information, of the $30$ pennies in Mr. Day’s jar, Theo looks at $5$ pennies from the jar and replaces them. Lydia looks at $10$ pennies and replaces them, and Peter looks at $20$ pennies and replaces them.

The pennies chosen by Theo, Lydia and Peter each represents a sample and the total pennies in the jar, that is, $30$ pennies represents the population.

The mean year of the pennies in the sample is the statistic and the mean year of the pennies in the population is the parameter.

b.

To determine

To calculate: the mean year and mean absolute deviation of Theo’s pennies.

Answer to Problem 19PPS

The mean year of Theo’s pennies is $\underset{\_}{1984}$ .

The mean absolute deviation is $\underset{\_}{9}$ .

Explanation of Solution

Given:

There are $30$ pennies in Mr. Day’s jar. Theo looks at $5$ pennies from the jar and replaces them.

The years of Theo’s pennies are:

  $1974,1975,1981,1999,1992$

Formula used :

Step1: Find the mean, $\overline{x}$

Step2: Find the sum of the absolute values of the difference between each value in the set of data and the mean.

Step3: divide the sum by the number of values in the set of data.

Calculation:

As per the given problem,

The data set: $1974,1975,1981,1999,1992$

The number of values in the data set $\text{=5}$

Step1: To find the mean, add the values in the data and then divide by the number of values in the data set.

  $\begin{array}{l}\overline{x}=\frac{1974+1975+1981+1999+1992}{5}\\ \overline{x}=\frac{9921}{5}\approx 1984\end{array}$

Step2: To find the sum of the absolute values, add the difference between each value and the mean.

The sum of absolute values

  $\begin{array}{l}=\left|1974-1984\right|+\left|1975-1984\right|+\left|1981-1984\right|+\left|1999-1984\right|+\left|1992-1984\right|\\ =10+9+3+15+8\\ =45\end{array}$

Step 3: Divide the sum by the number of values

  $\text{mean absolute deviation =}\frac{45}{5}=9$

c.

To determine

To calculate:the mean year and mean absolute deviation for Lydia’s pennies.

Answer to Problem 19PPS

The mean year of Lydia’s pennies is $\underset{\_}{2001}$ .

The mean absolute deviation is $\underset{\_}{3.2}$ .

Explanation of Solution

Given:

There are $30$ pennies in Mr. Day’s jar. Lydia looks at $10$ pennies and replaces them.

The years of Lydia’s pennies are:

  $2004,1999,2004,2005,1991,2003,2005,2000,2001,1998$

Calculation:

As per the given problem,

The data set: $2004,1999,2004,2005,1991,2003,2005,2000,2001,1998$

The number of values in the data set $\text{=10}$

Step1: To find the mean, add the values in the data and then divide by the number of values in the data set.

  $\begin{array}{l}\overline{x}=\frac{2004+1999+2004+2005+1991+2003+2005+2000+2001+1998}{10}\\ \overline{x}=\frac{20010}{10}=2001\end{array}$

Step2: To find the sum of the absolute values, add the difference between each value and the mean.

The sum of absolute values

  $\begin{array}{l}=\left|2004-2001\right|+\left|1999-2001\right|+\left|2004-2001\right|+\left|2005-2001\right|+\left|1991-2001\right|+\\ \left|2003-2001\right|+\left|2005-2001\right|+\left|2000-2001\right|+\left|2001-2001\right|+\left|1998-2001\right|\\ =3+2+3+4+10+2+4+1+0+3\\ =32\end{array}$

Step 3: Divide the sum by the number of values

  $\text{mean absolute deviation =}\frac{32}{10}=3.2$

d.

To determine

To calculate: the mean year and mean absolute deviation for Peter’s pennies.

Answer to Problem 19PPS

The mean year of Peter’s pennies is $\underset{\_}{1998}$ .

The mean absolute deviation is $\underset{\_}{6.45}$ .

Explanation of Solution

Given:

There are $30$ pennies in Mr. Day’s jar. Peter looks at $20$ pennies and replaces them.

The years of Peter’s pennies are:

  $\begin{array}{l}2007,2005,1975,2003,2005,1997,1992,1994,1991,1992,\\ 2000,1999,2005,1982,2005,2004,1998,2001,2002,2006\end{array}$

Calculation:

As per the given problem,

The data set:

  $\begin{array}{l}2007,2005,1975,2003,2005,1997,1992,1994,1991,1992,\\ 2000,1999,2005,1982,2005,2004,1998,2001,2002,2006\end{array}$

The number of values in the data set $\text{=20}$

Step1: To find the mean, add the values in the data and then divide by the number of values in the data set.

  $\begin{array}{l}\overline{x}= [2007+2005+1975+2003+2005+1997+1992+1994+1991+1992+\\ 2000+1999+2005+1982+2005+2004+1998+2001+2002+2006]÷20\\ \overline{x}=\frac{39963}{20}\approx 1998\end{array}$

Step2: To find the sum of the absolute values, add the difference between each value and the mean.

The sum of absolute values

  $\begin{array}{l}=\left|2007-1998\right|+\left|2005-1998\right|+\left|1975-1998\right|+\left|2003-1998\right|+\left|2005-1998\right|+\\ \left|1997-1998\right|+\left|1992-1998\right|+\left|1994-1998\right|+\left|1991-1998\right|+\left|1992-1998\right|+\\ \left|2000-1998\right|+\left|1999-1998\right|+\left|2005-1998\right|+\left|1982-1998\right|+\left|2005-1998\right|+\\ \left|2004-1998\right|+\left|1998-1998\right|+\left|2001-1998\right|+\left|2002-1998\right|+\left|2006-1998\right|\\ =9+7+23+5+7+1+6+4+7+6+2+1+7+16+7+6+0+3+4+8\\ =129\end{array}$

Step 3: Divide the sum by the number of values

  $\text{mean absolute deviation =}\frac{129}{20}=6.45$

e.

To determine

To calculate: the mean year and mean absolute deviation for all the pennies in the jar.

To compare the samples with the full population.

Answer to Problem 19PPS

The mean year of all the pennies is $\underset{\_}{1997}$ .

The mean absolute deviation is $\underset{\_}{7.4}$ .

Peter’s sample is more similar to the full population than that of the others.

Explanation of Solution

Given:

There are $30$ pennies in Mr. Day’s jar.

The year of pennies in jar:

  

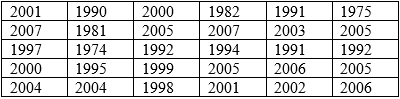
Calculation:

As per the given problem,

The data set:

  

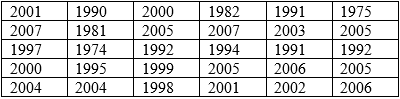
The number of values in the data set $\text{=30}$

Step1: To find the mean, add the values in the data and then divide by the number of values in the data set.

  $\begin{array}{l}\overline{x}= [2001+2007+1997+2000+2004+1990+1981+1974+1995+2004+\\ 2000+2005+1992+1999+1998+1982+2007+1994+2005+2001+\\ 1991+2003+1991+2006+2002+1975+2005+1992+2005+2006]÷30\\ \overline{x}=\frac{59912}{30}\approx 1997\end{array}$

Step2: To find the sum of the absolute values, add the difference between each value and the mean.

The sum of absolute values

  $\begin{array}{l}=\left|2001-1997\right|+\left|2007-1997\right|+\left|1997-1997\right|+\left|2000-1997\right|+\left|2004-1997\right|+\\ \left|1990-1997\right|+\left|1981-1997\right|+\left|1974-1997\right|+\left|1995-1997\right|+\left|2004-1997\right|+\\ \left|2000-1997\right|+\left|2005-1997\right|+\left|1992-1997\right|+\left|1999-1997\right|+\left|1998-1997\right|+\\ \left|1982-1997\right|+\left|2007-1997\right|+\left|1994-1997\right|+\left|2005-1997\right|+\left|2001-1997\right|+\\ \left|1991-1997\right|+\left|2003-1997\right|+\left|1991-1997\right|+\left|2006-1997\right|+\left|2002-1997\right|+\\ \left|1975-1997\right|+\left|2005-1997\right|+\left|1992-1997\right|+\left|2005-1997\right|+\left|2006-1997\right|\\ =4+10+0+3+7+7+16+23+2+7+3+8+5+2+1+15+10+3+8+\\ \text{}\text{}\text{}4+6+6+6+9+5+22+8+5+8+9\\ =222\end{array}$

Step 3: Divide the sum by the number of values

  $\text{mean absolute deviation =}\frac{222}{30}=7.4$

As, Peter’s sample (calculate in part (d)) has the minimum difference from the population’s mean year (one year) and mean absolute deviation ( $0.95$ ), then the populationis most accurately represented by Peter’s mean absolute deviation. This is because Peter’s sample constitute most of the pennies in the population.