
Concept explainers
(a)
Find the acceleration of block A for each system.
(a)
Answer to Problem 12.15P
The acceleration of block A for system 1 is
The acceleration of block A for system 2 is
The acceleration of block A for system 1 is
Explanation of Solution
Calculation:
Sketch the general diagram of systems as shown in Figure (1).
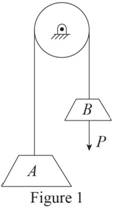
Write total length of cable connecting block A and block B.
Here,
Differentiate Equation (1) with respect to t to write velocity of the blocks.
Here,
Differentiate Equation (2) with respect to t to write acceleration of the blocks.
First of all check the required static friction with static friction to maintain equilibrium.
Sketch the free body diagram and kinetic diagram of block A as shown in Figure (2).
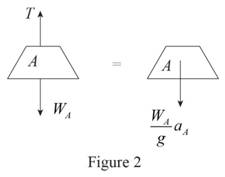
Refer Figure (2).
Consider downward direction as positive.
Apply Newton’s law of motion along y-axis.
Here, T is the tension in the cable,
Sketch the free body diagram and kinetic diagram of block B as shown in Figure (3).
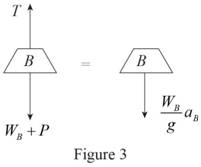
Refer Figure (3).
Consider downward direction as positive.
Apply Newton’s law of motion along y-axis.
Find the equation of acceleration of block A.
Here, T is the tension in the cable,
Substitute
The initial velocity of block A is zero.
Find the equation of velocity of block A using kinematics:
Here,
Substitute At
Find the equation of time required for block A to reach any velocity.
Find the acceleration of block A
Substitute 200 lb for
Therefore, the acceleration of block A for system 1 is
Find the acceleration of block A
Substitute 200 lb for
Therefore, the acceleration of block A for system 2 is
Find the acceleration of block A
Substitute 2200 lb for
Therefore, the acceleration of block A for system 2 is
(b)
Find the velocity of block A for each system after it has moved through 10 ft
(b)
Answer to Problem 12.15P
The velocity of block A for system 1 after it has moved through 10 ft is
The velocity of block A for system 2 after it has moved through 10 ft is
The velocity of block A for system 3 after it has moved through 10 ft is
Explanation of Solution
Calculation:
Find the velocity of block A for system 1
Substitute
Thus, the velocity of block A for system 1 after it has moved through 10 ft is
Find the velocity of block A for system 2
Substitute
Thus, the velocity of block A for system 2 after it has moved through 10 ft is
Find the velocity of block A for system 3
Substitute
Thus, the velocity of block A for system 3 after it has moved through 10 ft is
(c)
Find the time required for block A to reach a velocity of 20 ft/s
(c)
Answer to Problem 12.15P
The time required for block A for system 1 to reach a velocity of 20 ft/s is
The time required for block A for system 2 to reach a velocity of 20 ft/s is
The time required for block A for system 3 to reach a velocity of 20 ft/s is
Explanation of Solution
Calculation:
Find the time required for block A for system 1
Substitute
Thus, the time of required for block A for system 1 to reach a velocity of 20 ft/s is
Find the time required for block A for system 2
Substitute
Thus, the time of required for block A for system 2 to reach a velocity of 20 ft/s is
Find the time required for block A for system 3
Substitute
Thus, the time of required for block A for system 3 to reach a velocity of 20 ft/s is
Want to see more full solutions like this?
Chapter 12 Solutions
<LCPO> VECTOR MECH,STAT+DYNAMICS
- A gas turbine cycle has two stages of compression, with an intercooler between the stages. Air enters the first stage at 100 kPa, 300 K. The pressure efficiency of 82%. Air exits the intercooler at 330 K. Calculate the temperature at the exit of each compressor stage and the total specific work required.arrow_forwardFor problem 13, your answer should be the same as problem 12. Calculate the flow velocity and the heat transfer/area of the outer surfaces for both duct geometries to see the performance difference of the two designs.arrow_forwardOne end of a thin uniform rod of mass m and length 31 rests against a smooth vertical wall. The other end of the rod is attached by a string of length 1 to a fixed point O which is located a distance 21 from the wall. A horizontal force of magnitude F₁ is applied to the lower end of the rod as shown. Assuming the rod and the string remain in the same vertical plane perpendicular to the wall, find the angle 0 between the rod and the wall at the position of static equilibrium. Notes: This quiz is going to walk you through a sequence of steps to do this. It won't give you the answers, but it will hopefully get you to see how to approach problems like this so that you have a working reference/template in the future. This is actually a modified version of a problem from the textbook (6.3). Note that in that problem, is not actually given. It has been introduced for convenience as we move through solving the problem, and should not show up in the final answer. DO NOT DO PROBLEM 6.3. It is…arrow_forward
- varrow_forward13.64 The shaft shown in Sketch h transfers power between the two pulleys. The tension on the slack side (right pul- ley) is 30% of that on the tight side. The shaft rotates at 900 rpm and is supported uniformly by a radial ball bearing at points 0 and B. Select a pair of radial ball bear- ings with 99% reliability and 40,000 hr of life. Assume Eq. (13.83) can be used to account for lubricant clean- liness. All length dimensions are in millimeters. Ans. Cmin = 42,400 N.arrow_forwardA 4 inch wide, 12 inch tall cross section beam is subjected to an internal shear of 5.5 kips. What is the maximum transverse shear stress in the beam in psi if this bending is about the x axis?arrow_forward
- A Brayton cycle produces 14 MW with an inlet state of 17°C, 100 kPa, and a compression ratio of 16:1. The heat added in the combustion is 960 kJ/kg. 0.7 MW of heat transferred from the turbine to the environment. What are the highest temperature and the mass flow rate of air? Assume cold air properties.arrow_forward. A gas turbine with air enters the compressor at 300 K, 1 bar, and exits from the turbine at 750 K, 1 bar. The thermal efficiency of the cycle is 40.1% and the back work ratio (BWR) is 0.4. Find the pressure ratio of the cycle. Assume variable specific heat.arrow_forwardA regenerative gas turbine power plant is shown in Fig. below. Air enters the compressor at 1 bar, 27°C with a mass flow rate of 0.562 kg/s and is compressed to 4 bar. The isentropic efficiency of the compressor is 80%, and the regenerator effectiveness is 90%. All the power developed by the high-pressure turbine is used to run the compressor. The low-pressure turbine provides the net power output. Each turbine has an isentropic efficiency of 87% and the temperature at the inlet to the highpressure turbine is 1200 K. Assume cold air properties, determine: a. The net power output, in kW. b. The thermal efficiency of the cycle.arrow_forward
- For tixed inlet state and exit pressure, use a cold-air standard analysis to show that the pressure ratio across the two compressor stages that gives nunimum work input is:=)) k/(k-1) when Ta Ti, where Ta is the temperature of the air entering the second stage compressor and Pi is the intercooler pressure. Put the suitable assumptionsarrow_forwardDerive the equation below ah ap ax 12μ ax, +( ah ap ay 12μ ay Where P P (x, y) is the oil film pressure. 1..ah 2 axarrow_forwardCan you determine the eignevalues by hand?arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





