Concept explainers
Pascal’s Triangle The triangular pattern in the following figure is known as Pascal’s triangle. Pascal's triangle has intrigued mathematician for hundreds of years. Although it is named after the mathematician Blaise Pascal (1623-1662), there is evidence that it was first developed in China in the 1300s. The numbers in Pascal's triangle are created in the following manner. Each row begins and ends with the number 1. Any other number in a row is the sum of the two closest numbers about it. For instance, the first 10 in raw 5 is the sum of the first 4 and the 6 above it in raw 4.
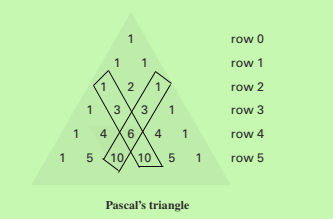
There are many patterns that can be discovered in Pascal's triangle.
a. Find the sum of the numbers in each row, except row 0, of the portion of Pascal's triangle shown above. What pattern do you observe concerning mesa sums? Predict the sum of the numbers in row 9 of Pascal’s triangle.
b. The numbers
Trending nowThis is a popular solution!

Chapter 1 Solutions
WebAssign Printed Access Card for Aufmann/Lockwood/Nation/Clegg's Mathematical Excursions, 4th Edition, Single-Term
- The position of a moving hockey puck after t seconds is s(t) = tan a. Find the velocity of the hockey puck at any time t. v(t) ===== b. Find the acceleration of the puck at any time t. -1 a (t) = (t) where s is in meters. c. Evaluate v(t) and a (t) for t = 1, 4, and 5 seconds. Round to 4 decimal places, if necessary. v (1) v (4) v (5) a (1) = = = = a (4) = a (5) = d. What conclusion can be drawn from the results in the previous part? ○ The hockey puck is decelerating/slowing down at 1, 4, and 5 seconds ○ The hockey puck has a constant velocity/speed at 1, 4, and 5 seconds ○ The hockey puck is accelerating/speeding up at 1, 4, and 5 secondsarrow_forwardIf the average price of a new one family home is $246,300 with a standard deviation of $15,000 find the minimum and maximum prices of the houses that a contractor will build to satisfy 88% of the market valuearrow_forwardT={(−7,1),(1,−1),(6,−8),(2,8)} Find the domain and range of the inverse. Express your answer as a set of numbers.arrow_forward
- T={(−7,1),(1,−1),(6,−8),(2,8)}. Find the inverse. Express your answer as a set of ordered pairs.arrow_forwardStarting with the finished version of Example 6.2, attached, change the decision criterion to "maximize expected utility," using an exponential utility function with risk tolerance $5,000,000. Display certainty equivalents on the tree. a. Keep doubling the risk tolerance until the company's best strategy is the same as with the EMV criterion—continue with development and then market if successful. The risk tolerance must reach $ 160,000,000 before the risk averse company acts the same as the EMV-maximizing company. b. With a risk tolerance of $320,000,000, the company views the optimal strategy as equivalent to receiving a sure $____________ , even though the EMV from the original strategy (with no risk tolerance) is $ 59,200.arrow_forwardComplete solutions need handwriting. For all only sure experts solve it correct complete solutionsarrow_forward
- The graph below shows the U.S. federal expenses for 2012. A) estimate the fraction of the total expenses that were spent on Medicare. Write your answer as the closest fraction whose denominator is 100. B) estimate the fraction of the total expenses that were spent on Medicare and Medicaid. Write your answer as the closest fraction, whose denominator is 100.arrow_forwardStarting with the finished version of Example 6.2, attached, change the decision criterion to "maximize expected utility," using an exponential utility function with risk tolerance $5,000,000. Display certainty equivalents on the tree. a. Keep doubling the risk tolerance until the company's best strategy is the same as with the EMV criterion—continue with development and then market if successful. The risk tolerance must reach $ ____________ before the risk averse company acts the same as the EMV-maximizing company. b. With a risk tolerance of $320,000,000, the company views the optimal strategy as equivalent to receiving a sure $____________ , even though the EMV from the original strategy (with no risk tolerance) is $ ___________ .arrow_forward2.8.1arrow_forward
- Do not use the Residue Theorem. Thank you.arrow_forwardA television network earns an average of $14 million each season from a hit program and loses an average of $8 million each season on a program that turns out to be a flop. Of all programs picked up by this network in recent years, 25% turn out to be hits and 75% turn out to be flops. At a cost of C dollars, a market research firm will analyze a pilot episode of a prospective program and issue a report predicting whether the given program will end up being a hit. If the program is actually going to be a hit, there is a 75% chance that the market researchers will predict the program to be a hit. If the program is actually going to be a flop, there is only a 30% chance that the market researchers will predict the program to be a hit. What is the maximum value of C that the network should be willing to pay the market research firm? Enter your answer in dollars, not in million dollars. $ __________ Calculate EVPI for this decision problem. Enter your answer in dollars, not in million…arrow_forwardEvaluate the line integral sin z dz, So sin where C is the portion of the curve y = x² from 0 to −1 + i.arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL


