Chapter 11, Problem 47DA

a.

To determine

Check whether there is evidence that there is a difference in the mean selling price of homes with a pool and without a pool.

Answer to Problem 47DA

The conclusion is that there is no evidence of a difference in the mean selling prices of homes with a pool and without a pool.

Explanation of Solution

Calculation:

In this context, let ${\mu }_1$ denotes the mean selling price of homes sold last year without a pool and ${\mu }_2$ denotes the mean selling price of homes sold last year with a pool.

The hypotheses are given below:

Null hypothesis:

$H_0:{\mu }_1={\mu }_2$

Alternative hypothesis:

$H_1:{\mu }_1\ne {\mu }_2$

Significance level, $\alpha$:

It is given that the significance level, $\alpha =0.05$.

Degrees of freedom:

The degrees of freedom is as follows:

$\begin{array}{c}df=n_1+n_2-2\\ =38+67-2\\ =103\end{array}$

Step-by-step procedure to obtain the critical values using MINITAB software:

• Choose Graph > Probability Distribution Plot choose View Probability > OK.
• From Distribution, choose ‘t’ distribution.
• In Degrees of freedom, enter 103.
• Click the Shaded Area tab.
• Choose Probability and Both Tails for the region of the curve to shade.
• Enter the Probability as 0.05.
• Click OK.

Output obtained using MINITAB software is given below:

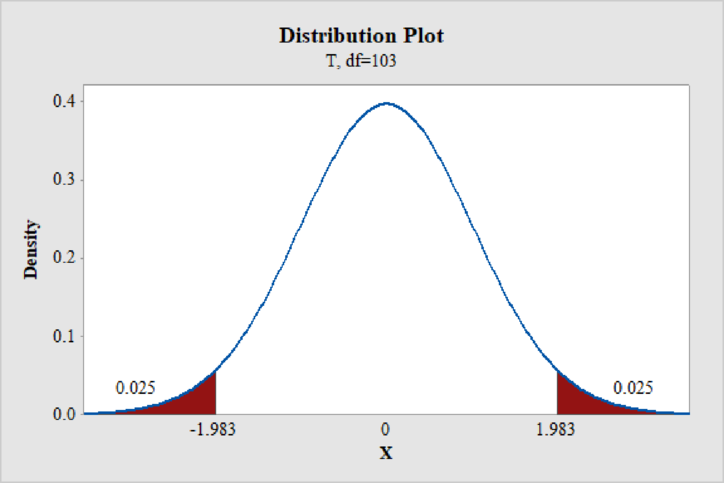
From the MINITAB output, the critical values are $\pm 1.983$

The decision rule is as follows:

If $t<-1.983$, then reject the null hypothesis $H_0$.

If $t\text{>}1.983$, then reject the null hypothesis $H_0$.

If $-1.983<t<1.983$, then fail to reject the null hypothesis $H_0$.

Test statistic:

Software procedure:

Step-by-step procedure to obtain the P-value and test statistic using MINITAB software:

• Choose Stat > Basic Statistics > 2 sample t.
• Choose Samples in different columns.
• In Sample 1, enter the column of Without pool.
• In Sample 2, enter the column of With pool.
• Choose Assume equal variance.
• Choose Options.
• In Confidence level, enter 95.
• In Alternative, select not equal.
• Click OK in all the dialogue boxes.

Output obtained using MINITAB software is given below:

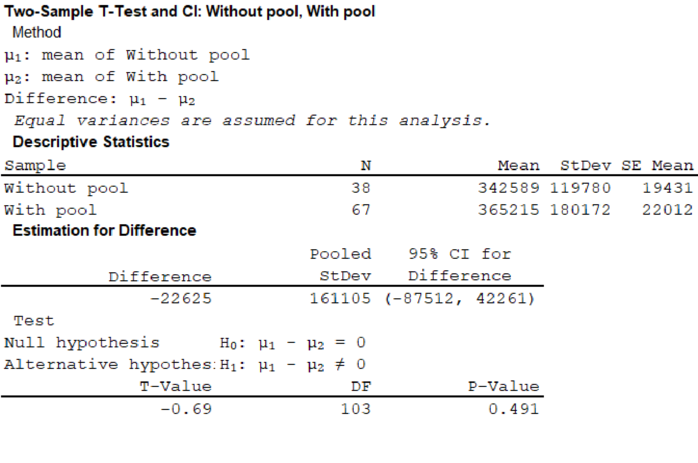
From the given MINITAB output, the value of the test statistic is –0.69.

Decision:

The critical values are ±1.983.

The value of test statistic is –0.69.

The value of test statistic lies between the critical values.

That is, $\text{Critical value}\left(=-1.983\right)<\text{Test statistic}\left(=-0.69\right)<\text{Critical value}\left(=1.983\right)$.

From the decision rule, fail to reject the null hypothesis.

Therefore, there is no evidence of a difference in the mean selling prices of homes with a pool and without a pool.

b.

To determine

Check whether there is evidence of a difference in the mean selling prices of homes with an attached garage and without an attached garage.

Answer to Problem 47DA

The conclusion is that there is evidence of difference in the mean selling prices of homes with an attached garage and homes without an attached garage.

Explanation of Solution

Calculation:

In this context, ${\mu }_1$ denotes the mean selling price of homes sold last year without an attached garage and ${\mu }_2$ denotes the mean selling price of homes sold last year with an attached garage.

The hypotheses are given below:

Null hypothesis:

$H_0:{\mu }_1={\mu }_2$

Alternative hypothesis:

$H_1:{\mu }_1\ne {\mu }_2$

Significance level, $\alpha$:

It is given that the significance level, $\alpha =0.05$.

Degrees of freedom:

The degrees of freedom is as follows:

$\begin{array}{c}df=n_1+n_2-2\\ =27+78-2\\ =103\end{array}$

From the Part a, the critical values are $\pm 1.983$.

Test statistic:

Software procedure:

Step-by-step procedure to obtain the P-value and test statistic using MINITAB software:

• Choose Stat > Basic Statistics > 2 sample t.
• Choose Samples in different columns.
• In Sample 1, enter the column of Without garage.
• In Sample 2, enter the column of With garage.
• Choose Assume equal variance.
• Choose Options.
• In Confidence level, enter 95.
• In Alternative, select not equal.
• Click OK in all the dialogue boxes.

Output obtained using MINITAB software is given below:

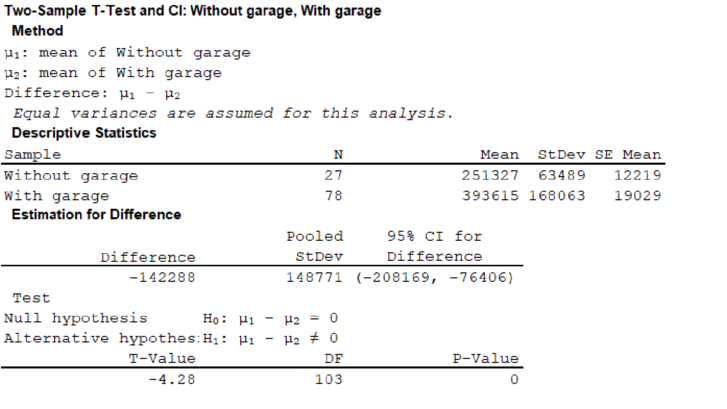
From the given MINITAB output, the value of the test statistic is –4.28.

Decision:

The critical values are ±1.983.

The value of test statistic is –4.28.

The value of test statistic is less than the critical value –1.983.

That is, $\text{Test statistic}\left(-4.28\right)<\text{Critical}\text{value}\left(\pm 1.983\right)$.

From the decision rule, reject the null hypothesis.

Therefore, there is evidence of difference in the mean selling prices of homes with an attached garage and without an attached garage.

c.

To determine

Check whether there is evidence of a difference in the mean selling price of homes that are in default on the mortgage and not in default on the mortgage.

Answer to Problem 47DA

The conclusion is that there is no evidence of difference in the mean selling prices of homes that are in default on the mortgage and not in default on the mortgage.

Explanation of Solution

Calculation:

In this context, ${\mu }_1$ denotes the mean selling price of homes sold last year that are not default on the mortgage and ${\mu }_2$ denotes the mean selling price of homes sold last year that are default on the mortgage.

The hypotheses are given below:

Null hypothesis:

$H_0:{\mu }_1={\mu }_2$

Alternative hypothesis:

$H_1:{\mu }_1\ne {\mu }_2$

Significance level, $\alpha$:

It is given that the significance level, $\alpha =0.05$.

Degrees of freedom:

The degrees of freedom is as follows:

$\begin{array}{c}df=n_1+n_2-2\\ =70+35-2\\ =103\end{array}$

From Part a, the critical values are $\pm 1.983$

Test statistic:

Software procedure:

Step-by-step procedure to obtain the P-value and test statistic using MINITAB software:

• Choose Stat > Basic Statistics > 2 sample t.
• Choose Samples in different columns.
• In Sample 1, enter the column of not default.
• In Sample 2, enter the column of default.
• Choose Assume equal variance.
• Choose Options.
• In Confidence level, enter 95.
• In Alternative, select not equal.
• Click OK in all the dialogue boxes.

Output obtained using MINITAB software is given below:

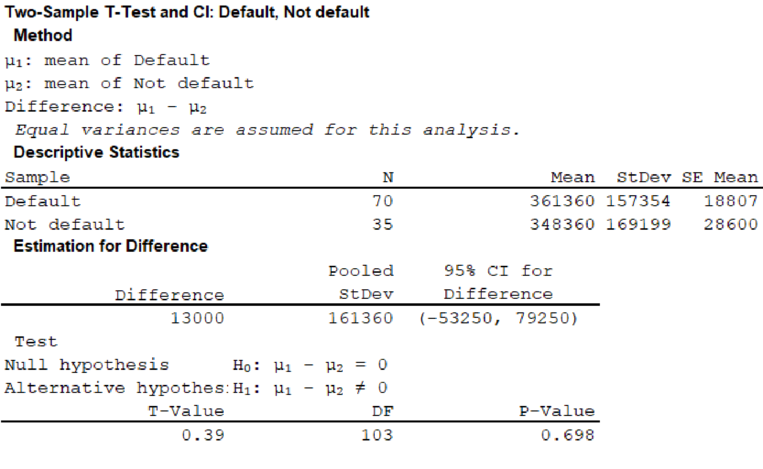
From the given MINITAB output, the value of the test statistic is 0.39.

Decision:

The critical values are ±1.983.

The value of test statistic is 0.39.

The value of test statistic lies between the critical values.

That is, $\text{Critical value}\left(=-1.983\right)<\text{Test statistic}\left(=0.39\right)<\text{Critical value}\left(=1.983\right)$.

From the decision rule, fail to reject the null hypothesis.

Therefore, there is no evidence that the difference in the mean selling price of homes that is in default on the mortgage and homes not default on the mortgage.