Chapter 11, Problem 21CE

To determine

Check whether there is a difference in the mean number of times men and women order take-out dinners in a month.

Find the p-value.

Answer to Problem 21CE

The conclusion is that there is no difference in the mean number of times men and women order take-out dinners in a month.

The p-value is 0.063.

Explanation of Solution

Calculation:

The null and alternative hypotheses are stated below:

Let ${\mu }_1$ is the average men who live alone buy take-out dinner in a month and ${\mu }_2$ the average women who live alone buy take-out dinner in a month.

Null hypothesis:

$H_0:{\mu }_1={\mu }_2$

Alternative hypothesis:

$H_1:{\mu }_1\ne {\mu }_2$

Significance level, $\alpha$:

It is given that the significance level, $\alpha =0.01$.

Degrees of freedom:

The degrees of freedom is as follows:

$\begin{array}{c}df=n_1+n_2-2\\ =35+40-2\\ =73\end{array}$

Thus, the number of degrees of freedom is 73.

Step-by-step procedure to obtain the critical value using MINITAB software:

• Choose Graph > Probability Distribution Plot choose View Probability > OK.
• From Distribution, choose ‘t’ distribution.
• In Degrees of freedom, enter 73.
• Click the Shaded Area tab.
• Choose P Value and both Tails for the region of the curve to shade.
• Enter the probability value as 0.01.
• Click OK.

Output obtained using MINITAB software is given below:

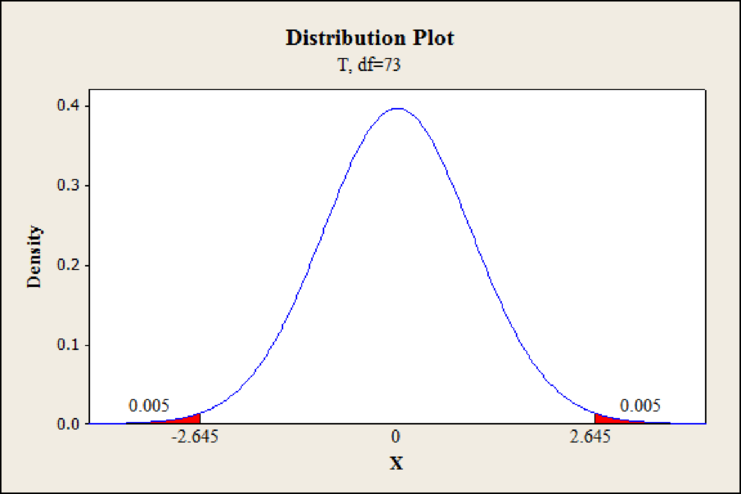
From the MINITAB output, the critical value is ±2.645.

The decision rule is as follows:

If $t\text{ <}-2.645$, then reject the null hypothesis $H_0$.

If $t\text{>}2.645$, then reject the null hypothesis $H_0$.

Pooled estimate:

The pooled estimate of the population variance is as follows:

$=\frac{\left(n_1-1\right)s_1^2+\left(n_2-1\right)s_2^2}{n_1+n_2-2}$

Substitute $s_1$ as 4.48, $s_2$ as 3.86, $n_1$ as 35, and $n_2$ as 40 in the formula given above as follows:

$\begin{array}{c}{s}_p^2=\frac{\left(35-1\right){\left(4.48\right)}^2+\left(40-1\right){\left(3.86\right)}^2}{35+40-2}\\ =\frac{\left(34\right)\left(20.0704\right)+\left(39\right)\left(14.8996\right)}{25}\\ =\frac{1,263.478}{73}\\ =17.31\end{array}$

Test statistic:

The test statistic for the hypothesis test of ${\mu }_1-{\mu }_2$ when ${\sigma }_1$ and ${\sigma }_2$ are unknown is as follows:

$t=\frac{\left({\overline{x}}_1-{\overline{x}}_2\right)}{\sqrt{s_p^2\left(\frac{1}{n_1}+\frac{1}{n_2}\right)}}$

Substitute ${\overline{x}}_1$ as 20.0704, ${\overline{x}}_2$ as 14.8996, $s_p^2$ as 17.31, $n_1$ as 35, and $n_2$ as 40 in the formula given above as follows:

$\begin{array}{c}t=\frac{24.51-22.69}{\sqrt{17.31\left(\frac{1}{35}+\frac{1}{40}\right)}}\\ =\frac{1.82}{\sqrt{0.9273}}\\ =\frac{1.82}{0.9630}\\ =1.890\end{array}$

Thus, the test statistic is 1.890.

Decision:

The critical value is 2.645, and the value of the test statistic is 1.890.

The value of the test statistic is less than the critical value.

That is, $\text{Test statistic}\left(1.890\right)<\text{Critical}\text{value}\left(2.645\right)$.

From the decision rule, fail to reject the null hypothesis.

Conclusion:

Therefore, there is no evidence that the mean number of times men and women order take-out dinners in a month.

p-value:

Step-by-step procedure to obtain the p-value using MINITAB software:

• Choose Graph > Probability Distribution Plot choose View Probability > OK.
• From Distribution, choose ‘t’ distribution.
• In Degrees of freedom, enter 73.
• Click the Shaded Area tab.
• Choose X Value and Two Tail for the region of the curve to shade.
• Enter the X value as 1.890.
• Click OK.

Output obtained using MINITAB software is given below:

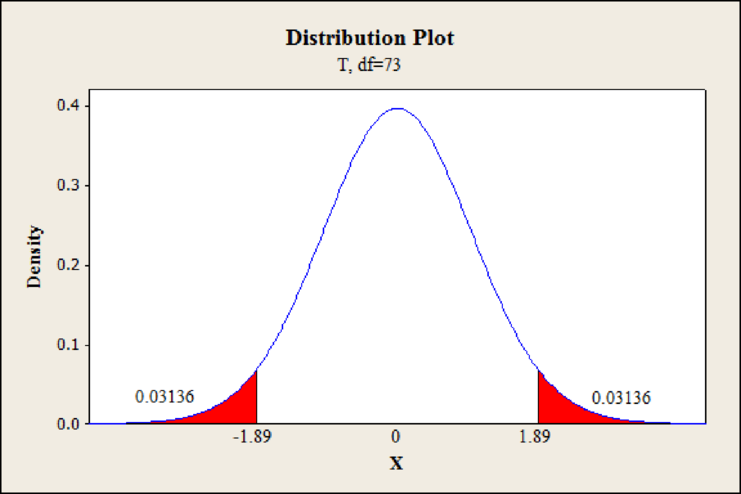
From the MINITAB output, the p-value is 0.03136.

$\begin{array}{c}p\text{-value = 2}\left(0.03136\right)\\ =0.063\end{array}$

Thus, the p-value is 0.063.