Chapter 11, Problem 2NT

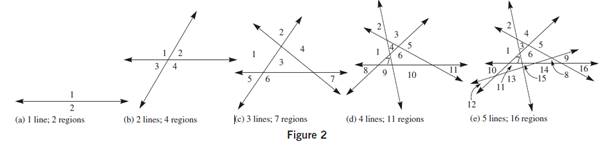
Just as a point separates a line into three parts, a line separates a plane into three parts: the line and two half-planes as shown in Table 4. Two lines can separate a plane into a maximum of 4 parts, and so on. Figure 2 shows the first few cases. Conjecture an expression for the maximum number of regions into which n lines in a plane separate the plane, if the lines themselves are not included.

To determine

To find:

The expression for the maximum numbers of regions for $n$ lines in a plane by using the figure

Answer to Problem 2NT

Solution:

$L\left(n\right)=\frac{n\left(n+1\right)}{2}$.

Explanation of Solution

Formula used:

The sum of first n numbers is $s=\left[\frac{n\left(n+1\right)}{2}\right]$

Calculation:

Given that, the one line can separate a plane into a maximum of 2 parts. The two lines can separate a plane into a maximum of 4 parts, three lines can separate a plane into a maximum of 7 parts, four lines can separate a plane into a maximum of 11 parts, and five lines can separate a plane into a maximum of 16 parts and so on.

If $n$ lines are added to a set of $n-1$ lines, then the maximum number of regions is equal to $n$.

Computethe equations by using the figure.

$L\left(2\right)-L\left(1\right)=2$. $\left(1\right)$

$L\left(3\right)-L\left(2\right)=3$. $\left(2\right)$

$L\left(4\right)-L\left(3\right)=4$. $\left(3\right)$

$L\left(5\right)-L\left(4\right)=5$. $\left(4\right)$

$⋮$

$L\left(n\right)-L\left(n-1\right)=n$. $\left(5\right)$

Add all the above equation as follows.

$\left\{\begin{array}{l}L\left(2\right)-L\left(1\right)+L\left(3\right)-L\left(2\right)+\\ L\left(4\right)-L\left(3\right)+L\left(5\right)-L\left(4\right)+\dots +L\left(n\right)-L\left(n-1\right)\end{array}\right\}=2+3+4+5+\dots +n$

Solve the equation as follws.

$\begin{array}{l}L\left(n\right)-L\left(1\right)=2+3+4+5+\dots +n\\ L\left(n\right)-2=2+3+4+5+\dots +n\left[\because L\left(1\right)=2\right]\\ L\left(n\right)=2+2+3+4+5+\dots +n\\ L\left(n\right)=\left(1+1\right)+2+3+4+5+\dots +n\\ L\left(n\right)=1+2+3+4+5+\dots +\left(n+1\right)\\ L\left(n\right)=\frac{n\left(n+1\right)}{2}\end{array}$

Therefore, the expression for the maximum numbers of regions for $n$ lines in a plane is $L\left(n\right)=\frac{n\left(n+1\right)}{2}$.