
Concept explainers
In Problems 1 and 2, is the matrix game strictly determined?
Whether the matrix game
Answer to Problem 1RE
The matrix game
Explanation of Solution
Consider the following matrix game.
Now, a saddle value is a payoff value which is both a row minimum and a column maximum of a payoff matrix.
Locate the saddle values.
Circle the minimum value in each row.
So, the matrix game will become,
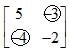
Place squares around the maximum payoff in each column of the matrix game.

In matrix game, none of the payoffs is both row minimum and column maximum.
So, the matrix game does not have a saddle value.
If the matrix game has a saddle value, then it is said to be strictly determined.
Hence, the matrix game is not strictly determined.
Want to see more full solutions like this?
Chapter 11 Solutions
FINITE MATHMATICS F/ BUSI...-ACCESS
Additional Math Textbook Solutions
College Algebra with Modeling & Visualization (5th Edition)
Calculus: Early Transcendentals (2nd Edition)
Calculus: Early Transcendentals (2nd Edition)
Basic Business Statistics, Student Value Edition
Elementary Statistics (13th Edition)
Algebra and Trigonometry (6th Edition)
- Q3: Solve the system x = A(t)x(t) where A = 1 1 -2 2 1 -1 01 - -1. (10M)arrow_forward17. Suppose that X1, X2,..., Xn are random variables, such that E|xk| < ∞ for all k, and set Yn = max1arrow_forward6. Show that, for any random variable, X, and a > 0, L P(x < X ≤ x+a) dx = a. 2015arrow_forward15. This problem extends Problem 20.6. Let X, Y be random variables with finite mean. Show that (P(X ≤ x ≤ Y) - P(Y < x ≤ X))dx = E Y — E X.arrow_forwardCould you please solve this question by sketching a graph to find the region of integration and the bounds of the integralarrow_forwardTheorem: Xo is critical point of x° = F(x) iff F(x)=0arrow_forward2. Which of the following statements are (not) true? lim sup{An U Bn} 818 lim sup{A, B} 818 lim inf{An U Bn} 818 818 lim inf{A, B} An An A, Bn- A, BnB →B = = = lim sup A, U lim sup Bn; 818 818 lim sup A, lim sup Bn; 818 81U lim inf A, U lim inf Bn; 818 818 lim inf A, lim inf Bn; n→X 818 An U BRAUB as no; An OBRANB as n→∞.arrow_forwardHelp me with the accurate answer and solution asap pls pls thank yo uarrow_forwardPls help me with accurate answer and solution as soon as possible pls thank youarrow_forwardHelp me with step by step solution and accurate answer as soon as possible plsarrow_forwardThroughout, A, B, (An, n≥ 1), and (Bn, n≥ 1) are subsets of 2. 1. Show that AAB (ANB) U (BA) = (AUB) (AB), Α' Δ Β = Α Δ Β, {A₁ U A2} A {B₁ U B2) C (A1 A B₁}U{A2 A B2).arrow_forward16. Show that, if X and Y are independent random variables, such that E|X|< ∞, and B is an arbitrary Borel set, then EXI{Y B} = EX P(YE B).arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
 Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education
Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
 Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON
Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning,
Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning, Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education
Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education





