
Concept explainers
(a)
Interpretation:
The probability for the particle having wavefunction
Concept introduction:
For the normalization of the wavefunction, the wavefunction is integrated as a product of its conjugate over the entire limits. It is expressed by the equation as given below.
Where,
•
•
•
Answer to Problem 10.27E
The probability for the particle having wavefunction
Explanation of Solution
For the probability of the wavefunction the expression is as follows.
Where,
•
•
•
•
Substitute the values in the above equation as follows.
The above equation is simplified as given below.
The probability for the particle having wavefunction
(b)
Interpretation:
The probability for the particle having wavefunction
Concept introduction:
For the normalization of the wavefunction, the wavefunction is integrated as a product of its conjugate over the entire limits. It is expressed by the equation as given below.
Where,
•
•
•
Answer to Problem 10.27E
The probability for the particle having wavefunction
Explanation of Solution
For the probability of the wavefunction the expression is as follows.
Where,
•
•
•
•
Substitute the values in the above equation as follows.
The above equation is simplified as follows.
The probability for the particle having wavefunction
(c)
Interpretation:
The probability for the particle having wavefunction
Concept introduction:
For the normalization of the wavefunction, the wavefunction is integrated as a product of its conjugate over the entire limits. It is expressed by the equation as given below.
Where,
•
•
•
Answer to Problem 10.27E
The probability for the particle having wavefunction
Explanation of Solution
For the probability of the wavefunction the expression is as follows.
Where,
•
•
•
•
Substitute the values in the above equation as follows.
The above equation is simplified as follows.
The probability for the particle having wavefunction
(d)
Interpretation:
The probability for the particle having wavefunction
Concept introduction:
For the normalization of the wavefunction, the wavefunction is integrated as a product of its conjugate over the entire limits. It is expressed by the equation as given below.
Where,
•
•
•
Answer to Problem 10.27E
The probability for the particle having wavefunction
Explanation of Solution
For the probability of the wavefunction the expression is as follows.
Where,
•
•
•
•
Substitute the values in the above equation as follows.
The above equation is simplified as given below.
The probability for the particle having wavefunction
(e)
Interpretation:
The probability for the particle having wavefunction
Concept introduction:
For the normalization of the wavefunction, the wavefunction is integrated as a product of its conjugate over the entire limits. It is expressed by the equation as given below.
Where,
•
•
•
Answer to Problem 10.27E
The probability for the particle having wavefunction
Explanation of Solution
For the probability of the wavefunction the expression is as follows.
Where,
•
•
•
•
Substitute the values in the above equation as follows.
The above equation is simplified as follows.
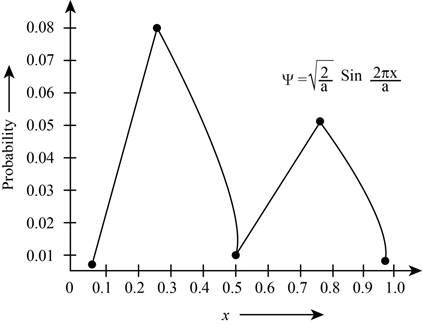
The plot the probabilities versus

Figure 1
The plot shows the probability for the given wave function. According to this plot, the probability of finding the particle is maximum in the range of
The probability for the particle having wavefunction
Want to see more full solutions like this?
Chapter 10 Solutions
Student Solutions Manual for Ball's Physical Chemistry, 2nd
- Explain how, based on physical gas adsorption isotherms, we can determine whether multi-walled C nanotubes are open at their ends. Explain this.arrow_forwardcan somone answer pleasearrow_forwardConstruct a molecular orbital energy-level diagram for BeH2. Sketch the MO pictures (schematic representation) for the HOMO and LUMO of BeH2 [Orbital Potential Energies, H (1s): -13.6 eV; Be (2s): -9.3 eV, Be (2p): -6.0 eV]arrow_forward
- Indicate the isomers of the A(H2O)6Cl3 complex. State the type of isomerism they exhibit and explain it briefly.arrow_forwardState the formula of the compound potassium μ-dihydroxydicobaltate (III) tetraoxalate.arrow_forwardConsider the reaction of the cyclopentanone derivative shown below. i) NaOCH2CH3 CH3CH2OH, 25°C ii) CH3!arrow_forward
- What constitutes a 'reference material', and why does its utilization play a critical role in the chemical analysis of food products? Provide examples.arrow_forwardExplain what calibration is and why it is essential in relation to food analysis. Provide examples.arrow_forwardThe cobalt mu-hydroxide complex cobaltate(III) of potassium is a dinuclear complex. Correct?arrow_forward
- The cobalt mi-hydroxide complex cobaltate(III) of potassium is a dinuclear complex. Correct?arrow_forward3. Arrange the different acids in Exercise B # 2 from the strongest (1) to the weakest acid (10). 1. 2. (strongest) 3. 4. 5. 6. 7. 8. 9. 10 10. (weakest)arrow_forwardName Section Score Date EXERCISE B pH, pOH, pка, AND PKD CALCULATIONS 1. Complete the following table. Solution [H+] [OH-] PH РОН Nature of Solution A 2 x 10-8 M B 1 x 10-7 M C D 12.3 6.8 2. The following table contains the names, formulas, ka or pka for some common acids. Fill in the blanks in the table. (17 Points) Acid Name Formula Dissociation reaction Ka pka Phosphoric acid H₂PO₁ H3PO4 H++ H₂PO 7.08 x 10-3 Dihydrogen H₂PO H₂PO H+ HPO 6.31 x 10-6 phosphate Hydrogen HPO₁ 12.4 phosphate Carbonic acid H2CO3 Hydrogen HCO 6.35 10.3 carbonate or bicarbonate Acetic acid CH,COOH 4.76 Lactic acid CH₂CHOH- COOH 1.38 x 10 Ammonium NH 5.63 x 10-10 Phenol CH₂OH 1 x 10-10 Protonated form CH3NH3* 3.16 x 10-11 of methylaminearrow_forward
 Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning,
Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning,
