Physics for Scientists and Engineers with Modern Physics, Technology Update
9th Edition
ISBN: 9781305401969
Author: SERWAY, Raymond A.; Jewett, John W.
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 1, Problem 7P
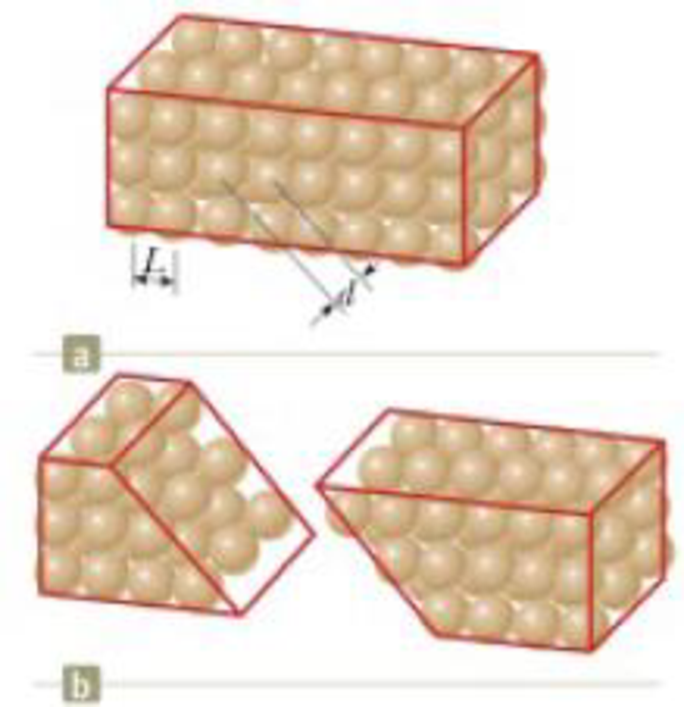
A crystalline solid consists of atoms stacked up in a repeating lattice structure. Consider a crystal as shown in Figure P1.7a. The atoms reside at the corners of cubes of side L = 0.200 nm. One piece of evidence for the regular arrangement of atoms comes from the flat surfaces along which a crystal separates, or cleaves, when it is broken. Suppose this crystal cleaves along a face diagonal as shown in Figure P1.7b. Calculate the spacing d between two adjacent atomic planes that separate when the crystal cleaves.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
E1
R₁
w
0.50
20 Ω
12
R₁₂
ww
ΒΩ
R₂
60
E3
C
RA
w
15 Ω
E2
0.25
E4
0.75 Ω
0.5 Ω
Solve plz
Solve plz
Chapter 1 Solutions
Physics for Scientists and Engineers with Modern Physics, Technology Update
Ch. 1.1 - In a machine shop, two cams are produced, one of...Ch. 1.3 - True or False: Dimensional analysis can give you...Ch. 1.4 - The distance between two cities is 100 mi. What is...Ch. 1 - Prob. 1OQCh. 1 - A house is advertised as having 1 420 square feet...Ch. 1 - Prob. 3OQCh. 1 - Prob. 4OQCh. 1 - Prob. 5OQCh. 1 - Prob. 6OQCh. 1 - Prob. 7OQ
Ch. 1 - Prob. 8OQCh. 1 - Prob. 9OQCh. 1 - Prob. 10OQCh. 1 - Prob. 1CQCh. 1 - Prob. 2CQCh. 1 - Prob. 3CQCh. 1 - Prob. 4CQCh. 1 - Prob. 1PCh. 1 - Prob. 2PCh. 1 - Prob. 3PCh. 1 - Prob. 4PCh. 1 - Prob. 5PCh. 1 - Prob. 6PCh. 1 - A crystalline solid consists of atoms stacked up...Ch. 1 - Prob. 8PCh. 1 - Prob. 9PCh. 1 - Prob. 10PCh. 1 - Prob. 11PCh. 1 - Prob. 12PCh. 1 - The position of a particle moving under uniform...Ch. 1 - (a) Assume the equation x = At3 + Bt describes the...Ch. 1 - A solid piece of lead has a mass of 23.94 g and a...Ch. 1 - Prob. 16PCh. 1 - Prob. 17PCh. 1 - Prob. 18PCh. 1 - Why is the following situation impossible? A...Ch. 1 - Prob. 20PCh. 1 - Prob. 21PCh. 1 - Prob. 22PCh. 1 - Prob. 23PCh. 1 - Prob. 24PCh. 1 - Prob. 25PCh. 1 - Let AI represent the density of aluminum and Fe...Ch. 1 - One gallon of paint (volume = 3.78 103 m3) covers...Ch. 1 - Prob. 28PCh. 1 - Prob. 29PCh. 1 - Prob. 30PCh. 1 - Prob. 31PCh. 1 - (a) Compute the order of magnitude of the mass of...Ch. 1 - To an order of magnitude, how many piano tuners...Ch. 1 - Prob. 34PCh. 1 - Prob. 35PCh. 1 - How many significant figures are in the following...Ch. 1 - The tropical year, the time interval from one...Ch. 1 - Prob. 38PCh. 1 - Review. In a community college parking lot, the...Ch. 1 - Prob. 40PCh. 1 - Prob. 41PCh. 1 - Prob. 42PCh. 1 - Review. The ratio of the number of sparrows...Ch. 1 - Prob. 44PCh. 1 - Prob. 45PCh. 1 - Prob. 46PCh. 1 - Prob. 47PCh. 1 - Prob. 48PCh. 1 - Prob. 49PCh. 1 - Prob. 50PCh. 1 - Prob. 51PCh. 1 - Prob. 52PCh. 1 - Prob. 53PCh. 1 - Prob. 54APCh. 1 - Prob. 55APCh. 1 - Prob. 56APCh. 1 - Prob. 57APCh. 1 - Prob. 58APCh. 1 - Prob. 59APCh. 1 - Prob. 60APCh. 1 - Prob. 61APCh. 1 - The distance from the Sun to the nearest star is...Ch. 1 - Prob. 63APCh. 1 - A spherical shell has an outside radius of 2.60 cm...Ch. 1 - Prob. 65APCh. 1 - Air is blown into a spherical balloon so that,...Ch. 1 - Prob. 67APCh. 1 - In physics, it is important to use mathematical...Ch. 1 - The consumption of natural gas by a company...Ch. 1 - A woman wishing to know the height of a mountain...Ch. 1 - Prob. 71APCh. 1 - Prob. 72APCh. 1 - Prob. 73AP
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- please help me solve this questions. show all calculations and a good graph too :)arrow_forwardWhat is the force (in N) on the 2.0 μC charge placed at the center of the square shown below? (Express your answer in vector form.) 5.0 με 4.0 με 2.0 με + 1.0 m 1.0 m -40 με 2.0 μCarrow_forwardWhat is the force (in N) on the 5.4 µC charge shown below? (Express your answer in vector form.) −3.1 µC5.4 µC9.2 µC6.4 µCarrow_forward
- An ideal gas in a sealed container starts out at a pressure of 8900 N/m2 and a volume of 5.7 m3. If the gas expands to a volume of 6.3 m3 while the pressure is held constant (still at 8900 N/m2), how much work is done by the gas? Give your answer as the number of Joules.arrow_forwardThe outside temperature is 25 °C. A heat engine operates in the environment (Tc = 25 °C) at 50% efficiency. How hot does it need to get the high temperature up to in Celsius?arrow_forwardGas is compressed in a cylinder creating 31 Joules of work on the gas during the isothermal process. How much heat flows from the gas into the cylinder in Joules?arrow_forward
- The heat engine gives 1100 Joules of energy of high temperature from the burning gasoline by exhausting 750 Joules to low-temperature . What is the efficiency of this heat engine in a percentage?arrow_forwardL₁ D₁ L₂ D2 Aluminum has a resistivity of p = 2.65 × 10 8 2. m. An aluminum wire is L = 2.00 m long and has a circular cross section that is not constant. The diameter of the wire is D₁ = 0.17 mm for a length of L₁ = 0.500 m and a diameter of D2 = 0.24 mm for the rest of the length. a) What is the resistance of this wire? R = Hint A potential difference of AV = 1.40 V is applied across the wire. b) What is the magnitude of the current density in the thin part of the wire? Hint J1 = c) What is the magnitude of the current density in the thick part of the wire? J₂ = d) What is the magnitude of the electric field in the thin part of the wire? E1 = Hint e) What is the magnitude of the electric field in the thick part of the wire? E2 =arrow_forwardplease helparrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781285737027
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781938168000
Author:Paul Peter Urone, Roger Hinrichs
Publisher:OpenStax College
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
Components of a Vector (Part 1) | Unit Vectors | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=fwMUELxZ0Pw;License: Standard YouTube License, CC-BY
02 - Learn Unit Conversions, Metric System & Scientific Notation in Chemistry & Physics; Author: Math and Science;https://www.youtube.com/watch?v=W_SMypXo7tc;License: Standard Youtube License