Thinking Mathematically (6th Edition)
6th Edition
ISBN: 9780321867322
Author: Robert F. Blitzer
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 1, Problem 27RE
(a)
To determine
The group in the village thathas a population that exceeds 100
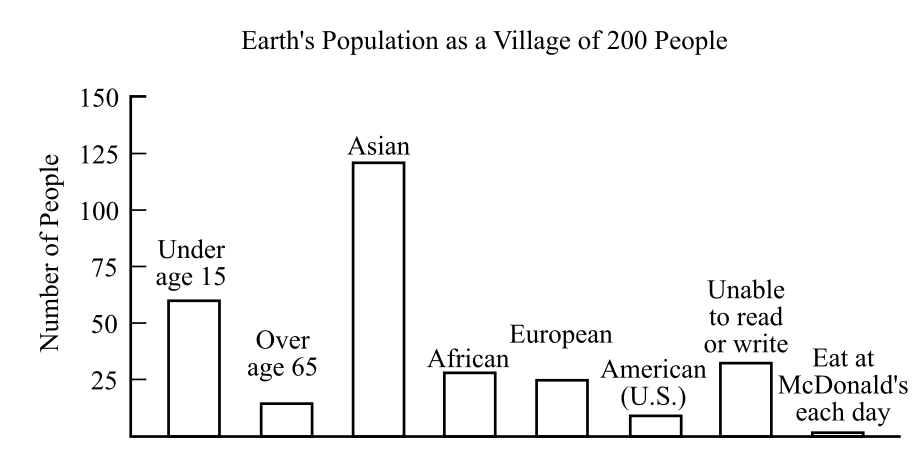
(b)
To determine
The estimate of the number of people in the world, in millions, unable to read or write from the given graph
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
9 AB is parallel to plane m and perpendicular to plane r. CD lies
in r. Which of the following must be true?
arim
br m
6 CD L m
d AB || CD
e AB and CD are skew.
a. A company is offering a job with a
salary of $35,000 for the first year and a
3% raise each year after that. If the 3%
raise continues every year, find the
amount of money you would earn in a
40-year career.
(6) Prove that the image of a polygon in R², under an isometry, is congruent to the
original polygon.
Chapter 1 Solutions
Thinking Mathematically (6th Edition)
Ch. 1.1 - CHECK POINT 1 Find a counterexample to show that...Ch. 1.1 - CHECK POINT 2 Identify a pattern in each list of...Ch. 1.1 - CHECK POINT 3 Identify a pattern in each list of...Ch. 1.1 - CHECK POINT 4 Describe two patterns in this...Ch. 1.1 - CHECK POINT 5 Consider the following procedure:...Ch. 1.1 - The statement 3 + 3 = 6 serves as a/an ________ to...Ch. 1.1 - Arriving at a specific conclusion from one or more...Ch. 1.1 - Arriving at a general conclusion based on...Ch. 1.1 - True or False: A theorem cannot have...Ch. 1.1 - In Exercises 1-8, find a counterexample to show...
Ch. 1.1 - In Exercises 1-8, find a counterexample to show...Ch. 1.1 - In Exercises 1-8, find a counterexample to show...Ch. 1.1 - In Exercises 1-8, find a counterexample to show...Ch. 1.1 - In Exercises 1-8, find a counterexample to show...Ch. 1.1 - In Exercises 1-8, find a counterexample to show...Ch. 1.1 - In Exercises 1-8, find a counterexample to show...Ch. 1.1 - In Exercises 1-8, find a counterexample to show...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, Identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 9-38, identify a pattern in each list...Ch. 1.1 - In Excises 9-38, identify a pattern in each list...Ch. 1.1 - In Exercises 39-42, identify a pattern in each...Ch. 1.1 - In Exercises 39-42, identify a pattern in each...Ch. 1.1 - In Exercises 39-42, identify a pattern in each...Ch. 1.1 - In Exercises 39-42, identify a pattern in each...Ch. 1.1 - Select a number. Multiply the number by 4. Add 8...Ch. 1.1 - Prob. 44ECh. 1.1 - Prob. 45ECh. 1.1 - Prob. 46ECh. 1.1 - In Exercises 47-52, use inductive reasoning to...Ch. 1.1 - In Exercises 47-52, use inductive reasoning to...Ch. 1.1 - In Exercises 47-52, use inductive reasoning to...Ch. 1.1 - Prob. 50ECh. 1.1 - In Exercises 47-52, use inductive reasoning to...Ch. 1.1 - In Exercises 47-52, use inductive reasoning to...Ch. 1.1 - In Exercises 53-54, use inductive reasoning to...Ch. 1.1 - In Exercises 53-54, use inductive reasoning to...Ch. 1.1 - Study the pattern in these examples: a 3 # a 4 = a...Ch. 1.1 - Study the pattern in these examples: a 5 * a 3 * a...Ch. 1.1 - In Exercises 57-60, identify the reasoning...Ch. 1.1 - In Exercises 57-60, identify the reasoning...Ch. 1.1 - Prob. 59ECh. 1.1 - In Exercises 57-60, identify the reasoning...Ch. 1.1 - The ancient Greeks studies figurate numbers, so...Ch. 1.1 - The triangular arrangement of numbers shown below...Ch. 1.1 - Writing about mathematics will help you to learn...Ch. 1.1 - Describe what is meant by deductive reasoning....Ch. 1.1 - Give an example of a decision that you made...Ch. 1.1 - Make Sense? In Exercises 66-69, determine whether...Ch. 1.1 - Make sense? In Exercises 66-69, determine whether...Ch. 1.1 - I used the data shown in the bar graph, which...Ch. 1.1 - I used the data shown in the bar graph for...Ch. 1.1 - If ( 6 − 2 ) 2 = 36 − 24 + 4 and ( 8 − 5 ) 2 = 64...Ch. 1.1 - 71. The rectangle shows an array of nine numbers...Ch. 1.1 - Write a list of numbers that has two patterns so...Ch. 1.1 - a. Repeat the following procedure with at least...Ch. 1.1 - a. Use a calculator to find 6 × 6 , 66 × 66 , 666...Ch. 1.1 - 75. a. Use a calculator to find , and .
b....Ch. 1.1 - Stereotyping refers to classifying people, places,...Ch. 1.2 - CHECKPOINT 1 Round world population...Ch. 1.2 - CHECK POINT 2 Round 3.141592, the first seven...Ch. 1.2 - CHECK POINT 3 You and a friend ate lunch at Ye...Ch. 1.2 - CHECK POINT 4 A Landscape architect who works full...Ch. 1.2 - Prob. 5CPCh. 1.2 - CHECK POINT 6 Use the date for women in Figure 1.5...Ch. 1.2 - Prob. 7CPCh. 1.2 - Prob. 8CPCh. 1.2 - The process of arriving at an approximate answer...Ch. 1.2 - Fill in each blank so that the resulting statement...Ch. 1.2 - Fill in each blank so that the resulting statement...Ch. 1.2 - Fill in each blank so that resulting statement is...Ch. 1.2 - Prob. 5CVCCh. 1.2 - Fill in each blank so that the resulting statement...Ch. 1.2 - Prob. 1ECh. 1.2 - The bar graph gives the 2011 populations of the...Ch. 1.2 - In Exercises 3-8, use this approximation to round...Ch. 1.2 - In Exercises 3-8, use this approximation to round...Ch. 1.2 - In Exercises 3-8, use this approximation to round...Ch. 1.2 - In Exercises 3-8, use this approximation to round...Ch. 1.2 - In Exercises 3-8, use this approximation to round...Ch. 1.2 - In Exercises 3-8, use this approximation to round...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 9-34, because different rounding...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - In Exercises 23-34, determine each estimate...Ch. 1.2 - The average life expectancy in Mozambique is 40.3...Ch. 1.2 - In Exercises 35-36, obtain an estimate for each...Ch. 1.2 - In Exercises 35-36, obtain an estimate for each...Ch. 1.2 - Ten people ordered calculators. The least...Ch. 1.2 - Ten people ordered calculators. The least...Ch. 1.2 - Travelling at an average rate of between 60 and 70...Ch. 1.2 - Prob. 40ECh. 1.2 - 41. Imagine that you counted 60 numbers per minute...Ch. 1.2 - Prob. 42ECh. 1.2 - 43. Without using a calculator, estimate the...Ch. 1.2 - Without using a calculator, estimate the number of...Ch. 1.2 - An online test of English spelling looked at how...Ch. 1.2 - An online test of English spelling looked at how...Ch. 1.2 - The percentage of U.S. college freshmen claiming...Ch. 1.2 - The percentage of U.S. college freshmen claiming...Ch. 1.2 - According to a Gallup poll, in 2012 almost a third...Ch. 1.2 - According to a Gallup poll, in 2012 almost a third...Ch. 1.2 - 51. a. Estimate the yearly increase in the average...Ch. 1.2 - a. Estimate the yearly increase in the average...Ch. 1.2 - Prob. 53ECh. 1.2 - Prob. 54ECh. 1.2 - Explain how to round 14.26841 to the nearest...Ch. 1.2 - Prob. 56ECh. 1.2 - 57. In this era of calculators and computers, why...Ch. 1.2 - Prob. 58ECh. 1.2 - Prob. 59ECh. 1.2 - Prob. 60ECh. 1.2 - Prob. 61ECh. 1.2 - Prob. 62ECh. 1.2 - Prob. 63ECh. 1.2 - Prob. 64ECh. 1.2 - 65. A forecaster at the National Hurricane Center...Ch. 1.2 - Prob. 66ECh. 1.2 - Prob. 67ECh. 1.2 - Prob. 68ECh. 1.2 - Prob. 69ECh. 1.2 - Prob. 70ECh. 1.2 - In Exercises 71-74, match the story with the...Ch. 1.2 - In Exercises 71-74, match the story with the...Ch. 1.2 - In Exercises 71-74, match the story with the...Ch. 1.2 - In Exercises 71-74, match the story with the...Ch. 1.2 - 75. American children ages 2 to 17 spend 19 hours...Ch. 1.2 - Prob. 76ECh. 1.2 - 77. Group members should devise an estimation...Ch. 1.2 - Group members should begin by consulting an...Ch. 1.3 - CHECK POINT 1 Which necessary piece of information...Ch. 1.3 - CHECK POINT 2 Solve the following problem. If the...Ch. 1.3 - CHECK POINT 3 By paying $350 cash up front and the...Ch. 1.3 - CHECK POINT 4 Suppose you are an engineer...Ch. 1.3 - CHECK POINT 5 Your “lecture wardrobe” is rather...Ch. 1.3 - CHECK POINT 6 As in Example 6, a sales director...Ch. 1.3 - The first step in problem solving is to read the...Ch. 1.3 - 2. The second step in problem solving is to ____...Ch. 1.3 - True or False: Polya’s four steps in problem...Ch. 1.3 - True or False: When making a choice between...Ch. 1.3 - In Exercises 1-4, what necessary piece of...Ch. 1.3 - In Exercises 1-4, what necessary piece of...Ch. 1.3 - In Exercises 1-4, what necessary piece of...Ch. 1.3 - In Exercises 1-4, what necessary piece of...Ch. 1.3 - In Exercises 5-8, one more piece of information is...Ch. 1.3 - In Exercises 5-8, one more piece of information is...Ch. 1.3 - In Exercises 5-8, one more piece of information is...Ch. 1.3 - In Exercises 5-8, one more piece of information is...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 14ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 16ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 20ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 23ECh. 1.3 - Prob. 24ECh. 1.3 - Prob. 25ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 30ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 32ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 34ECh. 1.3 - Prob. 35ECh. 1.3 - Prob. 36ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 38ECh. 1.3 - Use Polya’s four-step method in problem solving to...Ch. 1.3 - Prob. 40ECh. 1.3 - Prob. 41ECh. 1.3 - Prob. 42ECh. 1.3 - The map shows five western states. Trace a route...Ch. 1.3 - The layout of a city with land masses and bridges...Ch. 1.3 - Prob. 45ECh. 1.3 - The figure represents a map of 13 countries. If...Ch. 1.3 - Prob. 47ECh. 1.3 - Prob. 48ECh. 1.3 - As in Sudoku, fill in the missing numbers in the...Ch. 1.3 - The missing numbers in the 4-by-4 array are...Ch. 1.3 - Prob. 51ECh. 1.3 - Prob. 52ECh. 1.3 - Prob. 53ECh. 1.3 - In Exercises 52-54, explain the plan needed to...Ch. 1.3 - Prob. 55ECh. 1.3 - Make Sense? In Exercises 56-59, determine whether...Ch. 1.3 - Make Sense? In Exercises 56-59, determine whether...Ch. 1.3 - Make Sense? In Exercises 56-59, determine whether...Ch. 1.3 - Make Sense? In Exercises 56-59, determine whether...Ch. 1.3 - 60. Gym lockers are to be numbered from 1 through...Ch. 1.3 - Prob. 61ECh. 1.3 - Prob. 62ECh. 1.3 - 63. One person tells the truth on Monday, Tuesday,...Ch. 1.3 - Prob. 64ECh. 1.3 - 65. As in Sudoku, fill in the missing numbers...Ch. 1.3 - Prob. 66ECh. 1.3 - Prob. 67ECh. 1.3 - Prob. 68ECh. 1.3 - Prob. 69ECh. 1.3 - Prob. 70ECh. 1.3 - Prob. 71ECh. 1.3 - Prob. 72ECh. 1.3 - Prob. 73ECh. 1.3 - Prob. 74ECh. 1.3 - Group members should describe a problem in need of...Ch. 1 - 1. Which reasoning process is shown in the...Ch. 1 - Prob. 2TCh. 1 - Prob. 3TCh. 1 - Prob. 4TCh. 1 - Prob. 5TCh. 1 - Prob. 6TCh. 1 - Consider the following procedure: Select a number....Ch. 1 - Round 3,279,425 to the nearest hundred thousand.Ch. 1 - Prob. 9TCh. 1 - Prob. 10TCh. 1 - In Exercises 10-13, determine each estimate...Ch. 1 - In Exercises 10-13, determine each estimate...Ch. 1 - Prob. 13TCh. 1 - Prob. 14TCh. 1 - 15. The stated intent of the 1994 “don’t ask,...Ch. 1 - The bar graph shows a dramatic change in the high...Ch. 1 - The cost of renting a boat from Estes Rental is $9...Ch. 1 - Prob. 18TCh. 1 - Prob. 19TCh. 1 - Prob. 20TCh. 1 - 1. Which reasoning process is shown in the...Ch. 1 - 2. Which reasoning process is shown in the...Ch. 1 - In Exercises 3-10, identify a pattern in each list...Ch. 1 - Prob. 4RECh. 1 - In Exercises 3-10, identify a pattern in each list...Ch. 1 - Prob. 6RECh. 1 - Prob. 7RECh. 1 - In Exercises 3-10, identify a pattern in each list...Ch. 1 - Prob. 9RECh. 1 - Prob. 10RECh. 1 - Identify a pattern in the following sequence of...Ch. 1 - In Exercises 12-13, use inductive reasoning to...Ch. 1 - Prob. 13RECh. 1 - Consider the following procedure: Select a number....Ch. 1 - Prob. 15RECh. 1 - Prob. 16RECh. 1 - In Exercises 17-20, obtain an estimate for each...Ch. 1 - In Exercises 17-20, obtain an estimate for each...Ch. 1 - Prob. 19RECh. 1 - Prob. 20RECh. 1 - In Exercises 21-24, determine each estimate...Ch. 1 - In Exercises 21-24, determine each estimate...Ch. 1 - In Exercises 21-24, determine each estimate...Ch. 1 - Prob. 24RECh. 1 - A small private school employs 10 teachers with...Ch. 1 - Prob. 26RECh. 1 - Prob. 27RECh. 1 - 28. The bar graph shows the percentage of people...Ch. 1 - Prob. 29RECh. 1 - The bar graph shows the population of the Unites...Ch. 1 - Prob. 31RECh. 1 - Prob. 32RECh. 1 - Prob. 33RECh. 1 - Use the four-step method in problem solving to...Ch. 1 - Prob. 35RECh. 1 - Prob. 36RECh. 1 - Use the four-step method in problem solving to...Ch. 1 - Use the four-step method in problem solving to...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- The function f(x) is represented by the equation, f(x) = x³ + 8x² + x − 42. Part A: Does f(x) have zeros located at -7, 2, -3? Explain without using technology and show all work. Part B: Describe the end behavior of f(x) without using technology.arrow_forwardHow does the graph of f(x) = (x − 9)4 – 3 compare to the parent function g(x) = x²?arrow_forwardFind the x-intercepts and the y-intercept of the graph of f(x) = (x − 5)(x − 2)(x − 1) without using technology. Show all work.arrow_forward
- In a volatile housing market, the overall value of a home can be modeled by V(x) = 415x² - 4600x + 200000, where V represents the value of the home and x represents each year after 2020. Part A: Find the vertex of V(x). Show all work. Part B: Interpret what the vertex means in terms of the value of the home.arrow_forwardShow all work to solve 3x² + 5x - 2 = 0.arrow_forwardTwo functions are given below: f(x) and h(x). State the axis of symmetry for each function and explain how to find it. f(x) h(x) 21 5 4+ 3 f(x) = −2(x − 4)² +2 + -5 -4-3-2-1 1 2 3 4 5 -1 -2 -3 5arrow_forward
- The functions f(x) = (x + 1)² - 2 and g(x) = (x-2)² + 1 have been rewritten using the completing-the-square method. Apply your knowledge of functions in vertex form to determine if the vertex for each function is a minimum or a maximum and explain your reasoning.arrow_forwardTotal marks 15 3. (i) Let FRN Rm be a mapping and x = RN is a given point. Which of the following statements are true? Construct counterex- amples for any that are false. (a) If F is continuous at x then F is differentiable at x. (b) If F is differentiable at x then F is continuous at x. If F is differentiable at x then F has all 1st order partial (c) derivatives at x. (d) If all 1st order partial derivatives of F exist and are con- tinuous on RN then F is differentiable at x. [5 Marks] (ii) Let mappings F= (F1, F2) R³ → R² and G=(G1, G2) R² → R² : be defined by F₁ (x1, x2, x3) = x1 + x², G1(1, 2) = 31, F2(x1, x2, x3) = x² + x3, G2(1, 2)=sin(1+ y2). By using the chain rule, calculate the Jacobian matrix of the mapping GoF R3 R², i.e., JGoF(x1, x2, x3). What is JGOF(0, 0, 0)? (iii) [7 Marks] Give reasons why the mapping Go F is differentiable at (0, 0, 0) R³ and determine the derivative matrix D(GF)(0, 0, 0). [3 Marks]arrow_forward5. (i) Let f R2 R be defined by f(x1, x2) = x² - 4x1x2 + 2x3. Find all local minima of f on R². (ii) [10 Marks] Give an example of a function f: R2 R which is not bounded above and has exactly one critical point, which is a minimum. Justify briefly Total marks 15 your answer. [5 Marks]arrow_forward
- Total marks 15 4. : Let f R2 R be defined by f(x1, x2) = 2x²- 8x1x2+4x+2. Find all local minima of f on R². [10 Marks] (ii) Give an example of a function f R2 R which is neither bounded below nor bounded above, and has no critical point. Justify briefly your answer. [5 Marks]arrow_forward4. Let F RNR be a mapping. (i) x ЄRN ? (ii) : What does it mean to say that F is differentiable at a point [1 Mark] In Theorem 5.4 in the Lecture Notes we proved that if F is differentiable at a point x E RN then F is continuous at x. Proof. Let (n) CRN be a sequence such that xn → x ЄERN as n → ∞. We want to show that F(xn) F(x), which means F is continuous at x. Denote hnxn - x, so that ||hn|| 0. Thus we find ||F(xn) − F(x)|| = ||F(x + hn) − F(x)|| * ||DF (x)hn + R(hn) || (**) ||DF(x)hn||+||R(hn)||| → 0, because the linear mapping DF(x) is continuous and for all large nЄ N, (***) ||R(hn) || ||R(hn) || ≤ → 0. ||hn|| (a) Explain in details why ||hn|| → 0. [3 Marks] (b) Explain the steps labelled (*), (**), (***). [6 Marks]arrow_forward4. In Theorem 5.4 in the Lecture Notes we proved that if F: RN → Rm is differentiable at x = RN then F is continuous at x. Proof. Let (xn) CRN be a sequence such that x → x Є RN as n → ∞. We want F(x), which means F is continuous at x. to show that F(xn) Denote hn xnx, so that ||hn||| 0. Thus we find ||F (xn) − F(x) || (*) ||F(x + hn) − F(x)|| = ||DF(x)hn + R(hn)|| (**) ||DF(x)hn|| + ||R(hn) || → 0, because the linear mapping DF(x) is continuous and for all large n = N, |||R(hn) || ≤ (***) ||R(hn)|| ||hn|| → 0. Explain the steps labelled (*), (**), (***) [6 Marks] (ii) Give an example of a function F: RR such that F is contin- Total marks 10 uous at x=0 but F is not differentiable at at x = 0. [4 Marks]arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Use of ALGEBRA in REAL LIFE; Author: Fast and Easy Maths !;https://www.youtube.com/watch?v=9_PbWFpvkDc;License: Standard YouTube License, CC-BY
Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=P182Abv3fOk;License: Standard YouTube License, CC-BY
Applications of Algebra (Digit, Age, Work, Clock, Mixture and Rate Problems); Author: EngineerProf PH;https://www.youtube.com/watch?v=Y8aJ_wYCS2g;License: Standard YouTube License, CC-BY