
Using Radian or Degree Measure In Exercises 1-4, (a) sketch the angle in standard position, (b) determine the quadrant in which the angle lies, and (c) determine two coterminal
(a)
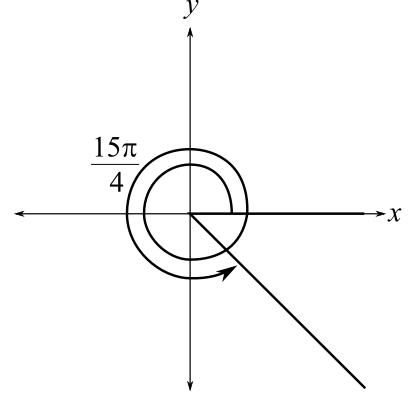
To graph: The angle,
Explanation of Solution
Graph:
Consider, the provided angle,
Now, in order to sketch the angle,
Since, the angle,
Therefore,
(b)
The quadrant in which, the angle,
Answer to Problem 1RE
The quadrant in which, the angle,
Explanation of Solution
Consider, the provided angle,
The co-ordinate system consists of four quadrants numbered as
Quadrant
Quadrant
Quadrant
Quadrant
Since, the angle
Therefore, the angle,
Hence, the quadrant in which, the angle,
(c)
The (one positive and one negative) co-terminal angle, for the angle,
Answer to Problem 1RE
The (one positive and one negative) co-terminal angle, for the angle,
Explanation of Solution
Co-terminal angle:
If two angles are made by the same initial and terminal side, then, such angles are called co-terminal angles. The positive and negative co-terminal angle of an angle can be obtained by subtracting or adding
Consider, the provided angle,
Now, in order to get the positive and negative co-terminal angles, add and subtract
For the positive angle,
And,
For the negative angle,
Hence, the (one positive and one negative) co-terminal angle, for the angle,
Want to see more full solutions like this?
Chapter 1 Solutions
Trigonometry (MindTap Course List)
- 11) The letters r and 0 represent polar coordinates. Write the equation r sine = 10 using rectangular coordinates (x, y). A) x = 10y B) y = 10 C) x = 10 D) y = 10xarrow_forward18) Find all the complex cube roots of - 8i. Leave your answers in polar form with the argument in degrees.arrow_forwardWrite the complex number √3 - i in polar form.arrow_forward
- 2 10) The letters x and y represent rectangular coordinates. Write the equation x² + 4y = 4 using polar coordinates (r, e). A) 4 cos² 0 + sin² 0 = 4r C) r²(4 cos² 0 + sin² 0) = 4 B) cos² 0 + 4 sin² 0 = 4r D) r² (cos20 + 4 sin² 0) = 4arrow_forward2) A radio transmission tower is 130 feet tall. How long should a guy wire be if it is to be attached 6 feet from the top and is to make an angle of 20° with the ground? Give your answer to the nearest tenth of a foot.arrow_forward4) Two sides and an angle are given. Determine whether the given information results in one triangle, two triangles, or no triangle at all. Solve any triangle(s) that results. b=6, c=7, B = 80° A) one triangle B=40°, A = 60°, a = 13 C) one triangle C 39°, A 61°, a = 15 = B) one triangle C=41°, A = 59°, a = 17 D) no trianglearrow_forward
- 7) A painter needs to cover a triangular region 63 meters by 67 meters by 74 meters. A can of paint covers 70 square meters. How many cans will be needed?arrow_forward12) Match the graph to one of the equations. 71 5+ 4+ 2. 32 ←IIIIIII -8 -7 -6 -5 -4-3-2-11 1 2 3 4 5 6 7 8 r -2+ -3+ -4+ -5+ -6+ -7⋅ A) r=3+4 sin 0 B) r 4+3 cos C) r=4+3 sin 0 D) r=3+4 cos 0arrow_forwardᏃ Find ✓. Leave your answer in polar form. 7π 7π Z = 3 cos + i sin 4 4 9π 9π w W = 6 cos + i sin 4 4arrow_forward
- Write the complex number in rectangular form. 11π 117 COS + i sin 6 6arrow_forward8) The polar coordinates of a point are given. Find the rectangular coordinates of the point. (-7, 3157) 2πarrow_forward3) Solve the triangle. 7 20° B с 110° A) C 55°, a = 19.23, c = 15.68 B) C = C) C=45°, a 15.68, c = 19.23 D) C 50°, a 19.23, c = 15.68 = 50%, a= 15.68, c = 19.23arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning  Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning





